Geometry

2021

• Paper 1, Section I, F

  Geometry | Part IB, 2021

  Let $f: \mathbb{R}^{3} \rightarrow \mathbb{R}$ be a smooth function and let $\Sigma=f^{-1}(0)$ (assumed not empty). Show that if the differential $D f_{p} \neq 0$ for all $p \in \Sigma$, then $\Sigma$ is a smooth surface in $\mathbb{R}^{3}$.

  Is $\left\{(x, y, z) \in \mathbb{R}^{3}: x^{2}+y^{2}=\cosh \left(z^{2}\right)\right\}$ a smooth surface? Is every surface $\Sigma \subset \mathbb{R}^{3}$ of the form $f^{-1}(0)$ for some smooth $f: \mathbb{R}^{3} \rightarrow \mathbb{R}$ ? Justify your answers.

  comment

• Paper 1, Section II, F

  Geometry | Part IB, 2021

  Let $S \subset \mathbb{R}^{3}$ be an oriented surface. Define the Gauss map $N$ and show that the differential $D N_{p}$ of the Gauss map at any point $p \in S$ is a self-adjoint linear map. Define the Gauss curvature $\kappa$ and compute $\kappa$ in a given parametrisation.

  A point $p \in S$ is called umbilic if $D N_{p}$ has a repeated eigenvalue. Let $S \subset \mathbb{R}^{3}$ be a surface such that every point is umbilic and there is a parametrisation $\phi: \mathbb{R}^{2} \rightarrow S$ such that $S=\phi\left(\mathbb{R}^{2}\right)$. Prove that $S$ is part of a plane or part of a sphere. $[$ Hint: consider the symmetry of the mixed partial derivatives $n_{u v}=n_{v u}$, where $n(u, v)=N(\phi(u, v))$ for $\left.(u, v) \in \mathbb{R}^{2} .\right]$

  comment

• Paper 2, Section II, E

  Geometry | Part IB, 2021

  Define $\mathbb{H}$, the upper half plane model for the hyperbolic plane, and show that $\operatorname{PSL}_{2}(\mathbb{R})$ acts on $\mathbb{H}$ by isometries, and that these isometries preserve the orientation of $\mathbb{H}$.

  Show that every orientation preserving isometry of $\mathbb{H}$ is in $P S L_{2}(\mathbb{R})$, and hence the full group of isometries of $\mathbb{H}$ is $G=P S L_{2}(\mathbb{R}) \cup P S L_{2}(\mathbb{R}) \tau$, where $\tau z=-\bar{z}$.

  Let $\ell$ be a hyperbolic line. Define the reflection $\sigma_{\ell}$ in $\ell$. Now let $\ell, \ell^{\prime}$ be two hyperbolic lines which meet at a point $A \in \mathbb{H}$ at an angle $\theta$. What are the possibilities for the group $G$ generated by $\sigma_{\ell}$ and $\sigma_{\ell^{\prime}}$ ? Carefully justify your answer.

  comment

• Paper 3, Section I, E

  Geometry | Part IB, 2021

  State the local Gauss-Bonnet theorem for geodesic triangles on a surface. Deduce the Gauss-Bonnet theorem for closed surfaces. [Existence of a geodesic triangulation can be assumed.]

  Let $S_{r} \subset \mathbb{R}^{3}$ denote the sphere with radius $r$ centred at the origin. Show that the Gauss curvature of $S_{r}$ is $1 / r^{2}$. An octant is any of the eight regions in $S_{r}$ bounded by arcs of great circles arising from the planes $x=0, y=0, z=0$. Verify directly that the local Gauss-Bonnet theorem holds for an octant. [You may assume that the great circles on $S_{r}$ are geodesics.]

  comment

• Paper 3, Section II, E

  Geometry | Part IB, 2021

  Let $S \subset \mathbb{R}^{3}$ be an embedded smooth surface and $\gamma:[0,1] \rightarrow S$ a parameterised smooth curve on $S$. What is the energy of $\gamma$ ? By applying the Euler-Lagrange equations for stationary curves to the energy function, determine the differential equations for geodesics on $S$ explicitly in terms of a parameterisation of $S$.

  If $S$ contains a straight line $\ell$, prove from first principles that each segment $[P, Q] \subset \ell$ (with some parameterisation) is a geodesic on $S$.

  Let $H \subset \mathbb{R}^{3}$ be the hyperboloid defined by the equation $x^{2}+y^{2}-z^{2}=1$ and let $P=\left(x_{0}, y_{0}, z_{0}\right) \in H$. By considering appropriate isometries, or otherwise, display explicitly three distinct (as subsets of $H$ ) geodesics $\gamma: \mathbb{R} \rightarrow H$ through $P$ in the case when $z_{0} \neq 0$ and four distinct geodesics through $P$ in the case when $z_{0}=0$. Justify your answer.

  Let $\gamma: \mathbb{R} \rightarrow H$ be a geodesic, with coordinates $\gamma(t)=(x(t), y(t), z(t))$. Clairaut's relation asserts $\rho(t) \sin \psi(t)$ is constant, where $\rho(t)=\sqrt{x(t)^{2}+y(t)^{2}}$ and $\psi(t)$ is the angle between $\dot{\gamma}(t)$ and the plane through the point $\gamma(t)$ and the $z$-axis. Deduce from Clairaut's relation that there exist infinitely many geodesics $\gamma(t)$ on $H$ which stay in the half-space $\{z>0\}$ for all $t \in \mathbb{R}$.

  [You may assume that if $\gamma(t)$ satisfies the geodesic equations on $H$ then $\gamma$ is defined for all $t \in \mathbb{R}$ and the Euclidean norm $\|\dot{\gamma}(t)\|$ is constant. If you use a version of the geodesic equations for a surface of revolution, then that should be proved.]

  comment

• Paper 4, Section II, F

  Geometry | Part IB, 2021

  Define an abstract smooth surface and explain what it means for the surface to be orientable. Given two smooth surfaces $S_{1}$ and $S_{2}$ and a map $f: S_{1} \rightarrow S_{2}$, explain what it means for $f$ to be smooth

  For the cylinder

  $$C=\left\{(x, y, z) \in \mathbb{R}^{3}: x^{2}+y^{2}=1\right\},$$

  let $a: C \rightarrow C$ be the orientation reversing diffeomorphism $a(x, y, z)=(-x,-y,-z)$. Let $S$ be the quotient of $C$ by the equivalence relation $p \sim a(p)$ and let $\pi: C \rightarrow S$ be the canonical projection map. Show that $S$ can be made into an abstract smooth surface so that $\pi$ is smooth. Is $S$ orientable? Justify your answer.

  comment

2020

• Paper 1, Section I, E

  Geometry | Part IB, 2020

  Define the Gauss map of a smooth embedded surface. Consider the surface of revolution $S$ with points

  $$\left(\begin{array}{c} (2+\cos v) \cos u \\ (2+\cos v) \sin u \\ \sin v \end{array}\right) \in \mathbb{R}^{3}$$

  for $u, v \in[0,2 \pi]$. Let $f$ be the Gauss map of $S$. Describe $f$ on the $\{y=0\}$ cross-section of $S$, and use this to write down an explicit formula for $f$.

  Let $U$ be the upper hemisphere of the 2 -sphere $S^{2}$, and $K$ the Gauss curvature of $S$. Calculate $\int_{f^{-1}(U)} K d A$.

  comment

• Paper 1, Section II, E

  Geometry | Part IB, 2020

  Let $\mathcal{C}$ be the curve in the $(x, z)$-plane defined by the equation

  $$\left(x^{2}-1\right)^{2}+\left(z^{2}-1\right)^{2}=5 .$$

  Sketch $\mathcal{C}$, taking care with inflection points.

  Let $S$ be the surface of revolution in $\mathbb{R}^{3}$ given by spinning $\mathcal{C}$ about the $z$-axis. Write down an equation defining $S$. Stating any result you use, show that $S$ is a smooth embedded surface.

  Let $r$ be the radial coordinate on the $(x, y)$-plane. Show that the Gauss curvature of $S$ vanishes when $r=1$. Are these the only points at which the Gauss curvature of $S$ vanishes? Briefly justify your answer.

  comment

• Paper 2, Section II, F

  Geometry | Part IB, 2020

  Let $H=\{z=x+i y \in \mathbb{C}: y>0\}$ be the hyperbolic half-plane with the metric $g_{H}=\left(d x^{2}+d y^{2}\right) / y^{2}$. Define the length of a continuously differentiable curve in $H$ with respect to $g_{H}$.

  What are the hyperbolic lines in $H$ ? Show that for any two distinct points $z, w$ in $H$, the infimum $\rho(z, w)$ of the lengths (with respect to $g_{H}$ ) of curves from $z$ to $w$ is attained by the segment $[z, w]$ of the hyperbolic line with an appropriate parameterisation.

  The 'hyperbolic Pythagoras theorem' asserts that if a hyperbolic triangle $A B C$ has angle $\pi / 2$ at $C$ then

  $$\cosh c=\cosh a \cosh b,$$

  where $a, b, c$ are the lengths of the sides $B C, A C, A B$, respectively.

  Let $l$ and $m$ be two hyperbolic lines in $H$ such that

  $$\inf \{\rho(z, w): z \in l, w \in m\}=d>0$$

  Prove that the distance $d$ is attained by the points of intersection with a hyperbolic line $h$ that meets each of $l, m$ orthogonally. Give an example of two hyperbolic lines $l$ and $m$ such that the infimum of $\rho(z, w)$ is not attained by any $z \in l, w \in m$.

  [You may assume that every Möbius transformation that maps H onto itself is an isometry of $\left.g_{H} \cdot\right]$

  comment

2019

• Paper 1, Section I, E

  Geometry | Part IB, 2019

  Describe the Poincaré disc model $D$ for the hyperbolic plane by giving the appropriate Riemannian metric.

  Calculate the distance between two points $z_{1}, z_{2} \in D$. You should carefully state any results about isometries of $D$ that you use.

  comment

• Paper 2, Section II, E

  Geometry | Part IB, 2019

  Define a smooth embedded surface in $\mathbb{R}^{3}$. Sketch the surface $C$ given by

  $$\left(\sqrt{2 x^{2}+2 y^{2}}-4\right)^{2}+2 z^{2}=2$$

  and find a smooth parametrisation for it. Use this to calculate the Gaussian curvature of $C$ at every point.

  Hence or otherwise, determine which points of the embedded surface

  $$\left(\sqrt{x^{2}+2 x z+z^{2}+2 y^{2}}-4\right)^{2}+(z-x)^{2}=2$$

  have Gaussian curvature zero. [Hint: consider a transformation of $\mathbb{R}^{3}$.]

  [You should carefully state any result that you use.]

  comment

• Paper 3, Section I, E

  Geometry | Part IB, 2019

  State a formula for the area of a spherical triangle with angles $\alpha, \beta, \gamma$.

  Let $n \geqslant 3$. What is the area of a convex spherical $n$-gon with interior angles $\alpha_{1}, \ldots, \alpha_{n}$ ? Justify your answer.

  Find the range of possible values for the interior angle of a regular convex spherical $n-g \mathrm{gn}$

  comment

• Paper 3, Section II, E

  Geometry | Part IB, 2019

  Define a geodesic triangulation of an abstract closed smooth surface. Define the Euler number of a triangulation, and state the Gauss-Bonnet theorem for closed smooth surfaces. Given a vertex in a triangulation, its valency is defined to be the number of edges incident at that vertex.

  (a) Given a triangulation of the torus, show that the average valency of a vertex of the triangulation is 6 .

  (b) Consider a triangulation of the sphere.

  (i) Show that the average valency of a vertex is strictly less than 6 .

  (ii) A triangulation can be subdivided by replacing one triangle $\Delta$ with three sub-triangles, each one with vertices two of the original ones, and a fixed interior point of $\Delta$.

  Using this, or otherwise, show that there exist triangulations of the sphere with average vertex valency arbitrarily close to 6 .

  (c) Suppose $S$ is a closed abstract smooth surface of everywhere negative curvature. Show that the average vertex valency of a triangulation of $S$ is bounded above and below.

  comment

• Paper 4, Section II, E

  Geometry | Part IB, 2019

  Let $H=\{x+i y \mid x, y \in \mathbb{R}, y>0\}$ be the upper-half plane with hyperbolic metric $\frac{d x^{2}+d y^{2}}{y^{2}}$. Define the group $P S L(2, \mathbb{R})$, and show that it acts by isometries on $H$. [If you use a generation statement you must carefully state it.]

  (a) Prove that $P S L(2, \mathbb{R})$ acts transitively on the collection of pairs $(l, P)$, where $l$ is a hyperbolic line in $H$ and $P \in l$.

  (b) Let $l^{+} \subset H$ be the imaginary half-axis. Find the isometries of $H$ which fix $l^{+}$ pointwise. Hence or otherwise find all isometries of $H$.

  (c) Describe without proof the collection of all hyperbolic lines which meet $l^{+}$with (signed) angle $\alpha, 0<\alpha<\pi$. Explain why there exists a hyperbolic triangle with angles $\alpha, \beta$ and $\gamma$ whenever $\alpha+\beta+\gamma<\pi$.

  (d) Is this triangle unique up to isometry? Justify your answer. [You may use without proof the fact that Möbius maps preserve angles.]

  comment

2018

• Paper 1, Section I, G

  Geometry | Part IB, 2018

  (a) State the Gauss-Bonnet theorem for spherical triangles.

  (b) Prove that any geodesic triangulation of the sphere has Euler number equal to $2 .$

  (c) Prove that there is no geodesic triangulation of the sphere in which every vertex is adjacent to exactly 6 triangles.

  comment

• Paper 2, Section II, G

  Geometry | Part IB, 2018

  For any matrix

  $$A=\left(\begin{array}{ll} a & b \\ c & d \end{array}\right) \in S L(2, \mathbb{R})$$

  the corresponding Möbius transformation is

  $$z \mapsto A z=\frac{a z+b}{c z+d},$$

  which acts on the upper half-plane $\mathbb{H}$, equipped with the hyperbolic metric $\rho$.

  (a) Assuming that $|\operatorname{tr} A|>2$, prove that $A$ is conjugate in $S L(2, \mathbb{R})$ to a diagonal matrix $B$. Determine the relationship between $|\operatorname{tr} A|$ and $\rho(i, B i)$.

  (b) For a diagonal matrix $B$ with $|\operatorname{tr} B|>2$, prove that

  $$\rho(x, B x)>\rho(i, B i)$$

  for all $x \in \mathbb{H}$ not on the imaginary axis.

  (c) Assume now that $|\operatorname{tr} A|<2$. Prove that $A$ fixes a point in $\mathbb{H}$.

  (d) Give an example of a matrix $A$ in $S L(2, \mathbb{R})$ that does not preserve any point or hyperbolic line in $\mathbb{H}$. Justify your answer.

  comment

• Paper 3, Section I, G

  Geometry | Part IB, 2018

  Consider a quadrilateral $A B C D$ in the hyperbolic plane whose sides are hyperbolic line segments. Suppose angles $A B C, B C D$ and $C D A$ are right-angles. Prove that $A D$ is longer than $B C$.

  [You may use without proof the distance formula in the upper-half-plane model

  $$\left.\rho\left(z_{1}, z_{2}\right)=2 \tanh ^{-1}\left|\frac{z_{1}-z_{2}}{z_{1}-\bar{z}_{2}}\right| \cdot\right]$$

  comment

• Paper 3, Section II, G

  Geometry | Part IB, 2018

  Let $U$ be an open subset of the plane $\mathbb{R}^{2}$, and let $\sigma: U \rightarrow S$ be a smooth parametrization of a surface $S$. A coordinate curve is an arc either of the form

  $$\alpha_{v_{0}}(t)=\sigma\left(t, v_{0}\right)$$

  for some constant $v_{0}$ and $t \in\left[u_{1}, u_{2}\right]$, or of the form

  $$\beta_{u_{0}}(t)=\sigma\left(u_{0}, t\right)$$

  for some constant $u_{0}$ and $t \in\left[v_{1}, v_{2}\right]$. A coordinate rectangle is a rectangle in $S$ whose sides are coordinate curves.

  Prove that all coordinate rectangles in $S$ have opposite sides of the same length if and only if $\frac{\partial E}{\partial v}=\frac{\partial G}{\partial u}=0$ at all points of $S$, where $E$ and $G$ are the usual components of the first fundamental form, and $(u, v)$ are coordinates in $U$.

  comment

• Paper 4, Section II, G

  Geometry | Part IB, 2018

  A Möbius strip in $\mathbb{R}^{3}$ is parametrized by

  $$\sigma(u, v)=(Q(u, v) \sin u, Q(u, v) \cos u, v \cos (u / 2))$$

  for $(u, v) \in U=(0,2 \pi) \times \mathbb{R}$, where $Q \equiv Q(u, v)=2-v \sin (u / 2)$. Show that the Gaussian curvature is

  $$K=\frac{-1}{\left(v^{2} / 4+Q^{2}\right)^{2}}$$

  at $(u, v) \in U$

  comment

2017

• Paper 1, Section I, G

  Geometry | Part IB, 2017

  Give the definition for the area of a hyperbolic triangle with interior angles $\alpha, \beta, \gamma$.

  Let $n \geqslant 3$. Show that the area of a convex hyperbolic $n$-gon with interior angles $\alpha_{1}, \ldots, \alpha_{n}$ is $(n-2) \pi-\sum \alpha_{i}$.

  Show that for every $n \geqslant 3$ and for every $A$ with $0<A<(n-2) \pi$ there exists a regular hyperbolic $n$-gon with area $A$.

  comment

• Paper 2, Section II, G

  Geometry | Part IB, 2017

  Let $H=\left\{\mathbf{x} \in \mathbb{R}^{n} \mid \mathbf{u} \cdot \mathbf{x}=c\right\}$ be a hyperplane in $\mathbb{R}^{n}$, where $\mathbf{u}$ is a unit vector and $c$ is a constant. Show that the reflection map

  $$\mathbf{x} \mapsto \mathbf{x}-2(\mathbf{u} \cdot \mathbf{x}-c) \mathbf{u}$$

  is an isometry of $\mathbb{R}^{n}$ which fixes $H$ pointwise.

  Let $\mathbf{p}, \mathbf{q}$ be distinct points in $\mathbb{R}^{n}$. Show that there is a unique reflection $R$ mapping $\mathbf{p}$ to $\mathbf{q}$, and that $R \in O(n)$ if and only if $\mathbf{p}$ and $\mathbf{q}$ are equidistant from the origin.

  Show that every isometry of $\mathbb{R}^{n}$ can be written as a product of at most $n+1$ reflections. Give an example of an isometry of $\mathbb{R}^{2}$ which cannot be written as a product of fewer than 3 reflections.

  comment

• Paper 3, Section I, G

  Geometry | Part IB, 2017

  Let

  $$\pi(x, y, z)=\frac{x+i y}{1-z}$$

  be stereographic projection from the unit sphere $S^{2}$ in $\mathbb{R}^{3}$ to the Riemann sphere $\mathbb{C}_{\infty}$. Show that if $r$ is a rotation of $S^{2}$, then $\pi r \pi^{-1}$ is a Möbius transformation of $\mathbb{C}_{\infty}$ which can be represented by an element of $S U(2)$. (You may assume without proof any result about generation of $S O(3)$ by a particular set of rotations, but should state it carefully.)

  comment

• Paper 3, Section II, G

  Geometry | Part IB, 2017

  Let $\sigma: U \rightarrow \mathbb{R}^{3}$ be a parametrised surface, where $U \subset \mathbb{R}^{2}$ is an open set.

  (a) Explain what are the first and second fundamental forms of the surface, and what is its Gaussian curvature. Compute the Gaussian curvature of the hyperboloid $\sigma(x, y)=(x, y, x y)$.

  (b) Let $\mathbf{a}(x)$ and $\mathbf{b}(x)$ be parametrised curves in $\mathbb{R}^{3}$, and assume that

  $$\sigma(x, y)=\mathbf{a}(x)+y \mathbf{b}(x)$$

  Find a formula for the first fundamental form, and show that the Gaussian curvature vanishes if and only if

  $$\mathbf{a}^{\prime} \cdot\left(\mathbf{b} \times \mathbf{b}^{\prime}\right)=0$$

  comment

• Paper 4, Section II, G

  Geometry | Part IB, 2017

  What is a hyperbolic line in (a) the disc model (b) the upper half-plane model of the hyperbolic plane? What is the hyperbolic distance $d(P, Q)$ between two points $P, Q$ in the hyperbolic plane? Show that if $\gamma$ is any continuously differentiable curve with endpoints $P$ and $Q$ then its length is at least $d(P, Q)$, with equality if and only if $\gamma$ is a monotonic reparametrisation of the hyperbolic line segment joining $P$ and $Q$.

  What does it mean to say that two hyperbolic lines $L, L^{\prime}$ are (a) parallel (b) ultraparallel? Show that $L$ and $L^{\prime}$ are ultraparallel if and only if they have a common perpendicular, and if so, then it is unique.

  A horocycle is a curve in the hyperbolic plane which in the disc model is a Euclidean circle with exactly one point on the boundary of the disc. Describe the horocycles in the upper half-plane model. Show that for any pair of horocycles there exists a hyperbolic line which meets both orthogonally. For which pairs of horocycles is this line unique?

  comment

2016

• Paper 1, Section I, F

  Geometry | Part IB, 2016

  (a) Describe the Poincaré disc model $D$ for the hyperbolic plane by giving the appropriate Riemannian metric.

  (b) Let $a \in D$ be some point. Write down an isometry $f: D \rightarrow D$ with $f(a)=0$.

  (c) Using the Poincaré disc model, calculate the distance from 0 to re $e^{i \theta}$ with $0 \leqslant r<1$

  (d) Using the Poincaré disc model, calculate the area of a disc centred at a point $a \in D$ and of hyperbolic radius $\rho>0$.

  comment

• Paper 2, Section II, F

  Geometry | Part IB, 2016

  (a) Let $A B C$ be a hyperbolic triangle, with the angle at $A$ at least $\pi / 2$. Show that the side $B C$ has maximal length amongst the three sides of $A B C$.

  [You may use the hyperbolic cosine formula without proof. This states that if $a, b$ and $c$ are the lengths of $B C, A C$, and $A B$ respectively, and $\alpha, \beta$ and $\gamma$ are the angles of the triangle at $A, B$ and $C$ respectively, then

  $$\cosh a=\cosh b \cosh c-\sinh b \sinh c \cos \alpha .]$$

  (b) Given points $z_{1}, z_{2}$ in the hyperbolic plane, let $w$ be any point on the hyperbolic line segment joining $z_{1}$ to $z_{2}$, and let $w^{\prime}$ be any point not on the hyperbolic line passing through $z_{1}, z_{2}, w$. Show that

  $$\rho\left(w^{\prime}, w\right) \leqslant \max \left\{\rho\left(w^{\prime}, z_{1}\right), \rho\left(w^{\prime}, z_{2}\right)\right\}$$

  where $\rho$ denotes hyperbolic distance.

  (c) The diameter of a hyperbolic triangle $\Delta$ is defined to be

  $$\sup \{\rho(P, Q) \mid P, Q \in \Delta\}$$

  Show that the diameter of $\Delta$ is equal to the length of its longest side.

  comment

• Paper 3, Section I, $5 F$

  Geometry | Part IB, 2016

  (a) State Euler's formula for a triangulation of a sphere.

  (b) A sphere is decomposed into hexagons and pentagons with precisely three edges at each vertex. Determine the number of pentagons.

  comment

• Paper 3, Section II, F

  Geometry | Part IB, 2016

  (a) Define the cross-ratio $\left[z_{1}, z_{2}, z_{3}, z_{4}\right]$ of four distinct points $z_{1}, z_{2}, z_{3}, z_{4} \in \mathbb{C} \cup\{\infty\}$. Show that the cross-ratio is invariant under Möbius transformations. Express $\left[z_{2}, z_{1}, z_{3}, z_{4}\right]$ in terms of $\left[z_{1}, z_{2}, z_{3}, z_{4}\right]$.

  (b) Show that $\left[z_{1}, z_{2}, z_{3}, z_{4}\right]$ is real if and only if $z_{1}, z_{2}, z_{3}, z_{4}$ lie on a line or circle in $\mathbb{C} \cup\{\infty\}$.

  (c) Let $z_{1}, z_{2}, z_{3}, z_{4}$ lie on a circle in $\mathbb{C}$, given in anti-clockwise order as depicted.

  

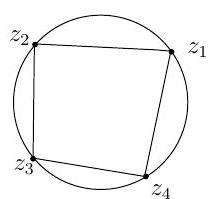
  Show that $\left[z_{1}, z_{2}, z_{3}, z_{4}\right]$ is a negative real number, and that $\left[z_{2}, z_{1}, z_{3}, z_{4}\right]$ is a positive real number greater than 1 . Show that $\left|\left[z_{1}, z_{2}, z_{3}, z_{4}\right]\right|+1=\left|\left[z_{2}, z_{1}, z_{3}, z_{4}\right]\right|$. Use this to deduce Ptolemy's relation on lengths of edges and diagonals of the inscribed 4-gon:

  $$\left|z_{1}-z_{3}\right|\left|z_{2}-z_{4}\right|=\left|z_{1}-z_{2}\right|\left|z_{3}-z_{4}\right|+\left|z_{2}-z_{3}\right|\left|z_{4}-z_{1}\right|$$

  comment

• Paper 4, Section II, F

  Geometry | Part IB, 2016

  Let $\alpha(s)=(f(s), g(s))$ be a simple curve in $\mathbb{R}^{2}$ parameterised by arc length with $f(s)>0$ for all $s$, and consider the surface of revolution $S$ in $\mathbb{R}^{3}$ defined by the parameterisation

  $$\sigma(u, v)=(f(u) \cos v, f(u) \sin v, g(u))$$

  (a) Calculate the first and second fundamental forms for $S$. Show that the Gaussian curvature of $S$ is given by

  $$K=-\frac{f^{\prime \prime}(u)}{f(u)}$$

  (b) Now take $f(s)=\cos s+2, g(s)=\sin s, 0 \leqslant s<2 \pi$. What is the integral of the Gaussian curvature over the surface of revolution $S$ determined by $f$ and $g$ ?

  [You may use the Gauss-Bonnet theorem without proof.]

  (c) Now suppose $S$ has constant curvature $K \equiv 1$, and suppose there are two points $P_{1}, P_{2} \in \mathbb{R}^{3}$ such that $S \cup\left\{P_{1}, P_{2}\right\}$ is a smooth closed embedded surface. Show that $S$ is a unit sphere, minus two antipodal points.

  [Do not attempt to integrate an expression of the form $\sqrt{1-C^{2} \sin ^{2} u}$ when $C \neq 1$. Study the behaviour of the surface at the largest and smallest possible values of $u$.]

  comment

2015

• Paper 1, Section I, F

  Geometry | Part IB, 2015

  (i) Give a model for the hyperbolic plane. In this choice of model, describe hyperbolic lines.

  Show that if $\ell_{1}, \ell_{2}$ are two hyperbolic lines and $p_{1} \in \ell_{1}, p_{2} \in \ell_{2}$ are points, then there exists an isometry $g$ of the hyperbolic plane such that $g\left(\ell_{1}\right)=\ell_{2}$ and $g\left(p_{1}\right)=p_{2}$.

  (ii) Let $T$ be a triangle in the hyperbolic plane with angles $30^{\circ}, 30^{\circ}$ and $45^{\circ}$. What is the area of $T$ ?

  comment

• Paper 2, Section II, F

  Geometry | Part IB, 2015

  (a) For each of the following subsets of $\mathbb{R}^{3}$, explain briefly why it is a smooth embedded surface or why it is not.

  $$\begin{aligned} S_{1} &=\{(x, y, z) \mid x=y, z=3\} \cup\{(2,3,0)\} \\ S_{2} &=\left\{(x, y, z) \mid x^{2}+y^{2}-z^{2}=1\right\} \\ S_{3} &=\left\{(x, y, z) \mid x^{2}+y^{2}-z^{2}=0\right\} \end{aligned}$$

  (b) Let $f: U=\{(u, v) \mid v>0\} \rightarrow \mathbb{R}^{3}$ be given by

  $$f(u, v)=\left(u^{2}, u v, v\right),$$

  and let $S=f(U) \subseteq \mathbb{R}^{3}$. You may assume that $S$ is a smooth embedded surface.

  Find the first fundamental form of this surface.

  Find the second fundamental form of this surface.

  Compute the Gaussian curvature of this surface.

  comment

• Paper 3, Section $I$, F

  Geometry | Part IB, 2015

  State the sine rule for spherical triangles.

  Let $\Delta$ be a spherical triangle with vertices $A, B$, and $C$, with angles $\alpha, \beta$ and $\gamma$ at the respective vertices. Let $a, b$, and $c$ be the lengths of the edges $B C, A C$ and $A B$ respectively. Show that $b=c$ if and only if $\beta=\gamma$. [You may use the cosine rule for spherical triangles.] Show that this holds if and only if there exists a reflection $M$ such that $M(A)=A, M(B)=C$ and $M(C)=B$.

  Are there equilateral triangles on the sphere? Justify your answer.

  comment

• Paper 3, Section II, F

  Geometry | Part IB, 2015

  Let $T: \mathbb{C}_{\infty} \rightarrow \mathbb{C}_{\infty}$ be a Möbius transformation on the Riemann sphere $\mathbb{C}_{\infty}$.

  (i) Show that $T$ has either one or two fixed points.

  (ii) Show that if $T$ is a Möbius transformation corresponding to (under stereographic projection) a rotation of $S^{2}$ through some fixed non-zero angle, then $T$ has two fixed points, $z_{1}, z_{2}$, with $z_{2}=-1 / \bar{z}_{1}$.

  (iii) Suppose $T$ has two fixed points $z_{1}, z_{2}$ with $z_{2}=-1 / \bar{z}_{1}$. Show that either $T$ corresponds to a rotation as in (ii), or one of the fixed points, say $z_{1}$, is attractive, i.e. $T^{n} z \rightarrow z_{1}$ as $n \rightarrow \infty$ for any $z \neq z_{2}$.

  comment

• Paper 4, Section II, F

  Geometry | Part IB, 2015

  Let $\alpha(s)=(f(s), g(s))$ be a curve in $\mathbb{R}^{2}$ parameterized by arc length, and consider the surface of revolution $S$ in $\mathbb{R}^{3}$ defined by the parameterization

  $$\sigma(u, v)=(f(u) \cos v, f(u) \sin v, g(u))$$

  In what follows, you may use that a curve $\sigma \circ \gamma$ in $S$, with $\gamma(t)=(u(t), v(t))$, is a geodesic if and only if

  $$\ddot{u}=f(u) \frac{d f}{d u} \dot{v}^{2}, \quad \frac{d}{d t}\left(f(u)^{2} \dot{v}\right)=0$$

  (i) Write down the first fundamental form for $S$, and use this to write down a formula which is equivalent to $\sigma \circ \gamma$ being a unit speed curve.

  (ii) Show that for a given $u_{0}$, the circle on $S$ determined by $u=u_{0}$ is a geodesic if and only if $\frac{d f}{d u}\left(u_{0}\right)=0$.

  (iii) Let $\gamma(t)=(u(t), v(t))$ be a curve in $\mathbb{R}^{2}$ such that $\sigma \circ \gamma$ parameterizes a unit speed curve that is a geodesic in $S$. For a given time $t_{0}$, let $\theta\left(t_{0}\right)$ denote the angle between the curve $\sigma \circ \gamma$ and the circle on $S$ determined by $u=u\left(t_{0}\right)$. Derive Clairault's relation that

  $$f(u(t)) \cos (\theta(t))$$

  is independent of $t$.

  comment

2014

• Paper 1, Section I, F

  Geometry | Part IB, 2014

  Determine the second fundamental form of a surface in $\mathbb{R}^{3}$ defined by the parametrisation

  $$\sigma(u, v)=((a+b \cos u) \cos v,(a+b \cos u) \sin v, b \sin u)$$

  for $0<u<2 \pi, 0<v<2 \pi$, with some fixed $a>b>0$. Show that the Gaussian curvature $K(u, v)$ of this surface takes both positive and negative values.

  comment

• Paper 2, Section II, F

  Geometry | Part IB, 2014

  Let $H=\{x+i y: x, y \in \mathbb{R}, y>0\} \subset \mathbb{C}$ be the upper half-plane with a hyperbolic metric $g=\frac{d x^{2}+d y^{2}}{y^{2}}$. Prove that every hyperbolic circle $C$ in $H$ is also a Euclidean circle. Is the centre of $C$ as a hyperbolic circle always the same point as the centre of $C$ as a Euclidean circle? Give a proof or counterexample as appropriate.

  Let $A B C$ and $A^{\prime} B^{\prime} C^{\prime}$ be two hyperbolic triangles and denote the hyperbolic lengths of their sides by $a, b, c$ and $a^{\prime}, b^{\prime}, c^{\prime}$, respectively. Show that if $a=a^{\prime}, b=b^{\prime}$ and $c=c^{\prime}$, then there is a hyperbolic isometry taking $A B C$ to $A^{\prime} B^{\prime} C^{\prime}$. Is there always such an isometry if instead the triangles have one angle the same and $a=a^{\prime}, b=b^{\prime} ?$ Justify your answer.

  [Standard results on hyperbolic isometries may be assumed, provided they are clearly stated.]

  comment

• Paper 3, Section I, F

  Geometry | Part IB, 2014

  Let $f(x)=A x+b$ be an isometry $\mathbb{R}^{n} \rightarrow \mathbb{R}^{n}$, where $A$ is an $n \times n$ matrix and $b \in \mathbb{R}^{n}$. What are the possible values of $\operatorname{det} A$ ?

  Let $I$ denote the $n \times n$ identity matrix. Show that if $n=2$ and $\operatorname{det} A>0$, but $A \neq I$, then $f$ has a fixed point. Must $f$ have a fixed point if $n=3$ and $\operatorname{det} A>0$, but $A \neq I ?$ Justify your answer.

  comment

• Paper 3, Section II, F

  Geometry | Part IB, 2014

  Let $\mathcal{T}$ be a decomposition of the two-dimensional sphere into polygonal domains, with every polygon having at least three edges. Let $V, E$, and $F$ denote the numbers of vertices, edges and faces of $\mathcal{T}$, respectively. State Euler's formula. Prove that $2 E \geqslant 3 F$.

  Suppose that at least three edges meet at every vertex of $\mathcal{T}$. Let $F_{n}$ be the number of faces of $\mathcal{T}$ that have exactly $n$ edges $(n \geqslant 3)$ and let $V_{m}$ be the number of vertices at which exactly $m$ edges meet $(m \geqslant 3)$. Is it possible for $\mathcal{T}$ to have $V_{3}=F_{3}=0$ ? Justify your answer.

  By expressing $6 F-\sum_{n} n F_{n}$ in terms of the $V_{j}$, or otherwise, show that $\mathcal{T}$ has at least four faces that are triangles, quadrilaterals and/or pentagons.

  comment

• Paper 4, Section II, F

  Geometry | Part IB, 2014

  Define an embedded parametrised surface in $\mathbb{R}^{3}$. What is the Riemannian metric induced by a parametrisation? State, in terms of the Riemannian metric, the equations defining a geodesic curve $\gamma:(0,1) \rightarrow S$, assuming that $\gamma$ is parametrised by arc-length.

  Let $S$ be a conical surface

  $$S=\left\{(x, y, z) \in \mathbb{R}^{3}: 3\left(x^{2}+y^{2}\right)=z^{2}, \quad z>0\right\}$$

  Using an appropriate smooth parametrisation, or otherwise, prove that $S$ is locally isometric to the Euclidean plane. Show that any two points on $S$ can be joined by a geodesic. Is this geodesic always unique (up to a reparametrisation)? Justify your answer.

  [The expression for the Euclidean metric in polar coordinates on $\mathbb{R}^{2}$ may be used without proof.]

  comment

2013

• Paper 1, Section I, F

  Geometry | Part IB, 2013

  Let $l_{1}$ and $l_{2}$ be ultraparallel geodesics in the hyperbolic plane. Prove that the $l_{i}$ have a unique common perpendicular.

  Suppose now $l_{1}, l_{2}, l_{3}$ are pairwise ultraparallel geodesics in the hyperbolic plane. Can the three common perpendiculars be pairwise disjoint? Must they be pairwise disjoint? Briefly justify your answers.

  comment

• Paper 2, Section II, F

  Geometry | Part IB, 2013

  Let $A$ and $B$ be disjoint circles in $\mathbb{C}$. Prove that there is a Möbius transformation which takes $A$ and $B$ to two concentric circles.

  A collection of circles $X_{i} \subset \mathbb{C}, 0 \leqslant i \leqslant n-1$, for which

  1. $X_{i}$ is tangent to $A, B$ and $X_{i+1}$, where indices are $\bmod n$;

  2. the circles are disjoint away from tangency points;

  is called a constellation on $(A, B)$. Prove that for any $n \geqslant 2$ there is some pair $(A, B)$ and a constellation on $(A, B)$ made up of precisely $n$ circles. Draw a picture illustrating your answer.

  Given a constellation on $(A, B)$, prove that the tangency points $X_{i} \cap X_{i+1}$ for $0 \leqslant i \leqslant n-1$ all lie on a circle. Moreover, prove that if we take any other circle $Y_{0}$ tangent to $A$ and $B$, and then construct $Y_{i}$ for $i \geqslant 1$ inductively so that $Y_{i}$ is tangent to $A, B$ and $Y_{i-1}$, then we will have $Y_{n}=Y_{0}$, i.e. the chain of circles will again close up to form a constellation.

  comment

• Paper 3, Section I, F

  Geometry | Part IB, 2013

  Let $S$ be a surface with Riemannian metric having first fundamental form $d u^{2}+G(u, v) d v^{2}$. State a formula for the Gauss curvature $K$ of $S$.

  Suppose that $S$ is flat, so $K$ vanishes identically, and that $u=0$ is a geodesic on $S$ when parametrised by arc-length. Using the geodesic equations, or otherwise, prove that $G(u, v) \equiv 1$, i.e. $S$ is locally isometric to a plane.

  comment

• Paper 3, Section II, F

  Geometry | Part IB, 2013

  Show that the set of all straight lines in $\mathbb{R}^{2}$ admits the structure of an abstract smooth surface $S$. Show that $S$ is an open Möbius band (i.e. the Möbius band without its boundary circle), and deduce that $S$ admits a Riemannian metric with vanishing Gauss curvature.

  Show that there is no metric $d: S \times S \rightarrow \mathbb{R}_{\geqslant 0}$, in the sense of metric spaces, which

  1. induces the locally Euclidean topology on $S$ constructed above;

  2. is invariant under the natural action on $S$ of the group of translations of $\mathbb{R}^{2}$.

  Show that the set of great circles on the two-dimensional sphere admits the structure of a smooth surface $S^{\prime}$. Is $S^{\prime}$ homeomorphic to $S$ ? Does $S^{\prime}$ admit a Riemannian metric with vanishing Gauss curvature? Briefly justify your answers.

  comment

• Paper 4, Section II, F

  Geometry | Part IB, 2013

  Let $\eta$ be a smooth curve in the $x z$-plane $\eta(s)=(f(s), 0, g(s))$, with $f(s)>0$ for every $s \in \mathbb{R}$ and $f^{\prime}(s)^{2}+g^{\prime}(s)^{2}=1$. Let $S$ be the surface obtained by rotating $\eta$ around the $z$-axis. Find the first fundamental form of $S$.

  State the equations for a curve $\gamma:(a, b) \rightarrow S$ parametrised by arc-length to be a geodesic.

  A parallel on $S$ is the closed circle swept out by rotating a single point of $\eta$. Prove that for every $n \in \mathbb{Z}_{>0}$ there is some $\eta$ for which exactly $n$ parallels are geodesics. Sketch possible such surfaces $S$ in the cases $n=1$ and $n=2$.

  If every parallel is a geodesic, what can you deduce about $S$ ? Briefly justify your answer.

  comment

2012

• Paper 1, Section I, G

  Geometry | Part IB, 2012

  Describe a collection of charts which cover a circular cylinder of radius $R$. Compute the first fundamental form, and deduce that the cylinder is locally isometric to the plane.

  comment

• Paper 2, Section II, G

  Geometry | Part IB, 2012

  Let $S$ be a closed surface, equipped with a triangulation. Define the Euler characteristic $\chi(S)$ of $S$. How does $\chi(S)$ depend on the triangulation?

  Let $V, E$ and $F$ denote the number of vertices, edges and faces of the triangulation. Show that $2 E=3 F$.

  Suppose now the triangulation is tidy, meaning that it has the property that no two vertices are joined by more than one edge. Deduce that $V$ satisfies

  $$V \geqslant \frac{7+\sqrt{49-24 \chi(S)}}{2} .$$

  Hence compute the minimal number of vertices of a tidy triangulation of the real projective plane. [Hint: it may be helpful to consider the icosahedron as a triangulation of the sphere $\left.S^{2} .\right]$

  comment

• Paper 3, Section I, $5 G$

  Geometry | Part IB, 2012

  State a formula for the area of a hyperbolic triangle.

  Hence, or otherwise, prove that if $l_{1}$ and $l_{2}$ are disjoint geodesics in the hyperbolic plane, there is at most one geodesic which is perpendicular to both $l_{1}$ and $l_{2}$.

  comment

• Paper 3, Section II, G

  Geometry | Part IB, 2012

  Define the first and second fundamental forms of a smooth surface $\Sigma \subset \mathbb{R}^{3}$, and explain their geometrical significance.

  Write down the geodesic equations for a smooth curve $\gamma:[0,1] \rightarrow \Sigma$. Prove that $\gamma$ is a geodesic if and only if the derivative of the tangent vector to $\gamma$ is always orthogonal to $\Sigma$.

  A plane $\Pi \subset \mathbb{R}^{3}$ cuts $\Sigma$ in a smooth curve $C \subset \Sigma$, in such a way that reflection in the plane $\Pi$ is an isometry of $\Sigma$ (in particular, preserves $\Sigma$ ). Prove that $C$ is a geodesic.

  comment

• Paper 4, Section II, G

  Geometry | Part IB, 2012

  Let $\Sigma \subset \mathbb{R}^{3}$ be a smooth closed surface. Define the principal curvatures $\kappa_{\max }$ and $\kappa_{\min }$ at a point $p \in \Sigma$. Prove that the Gauss curvature at $p$ is the product of the two principal curvatures.

  A point $p \in \Sigma$ is called a parabolic point if at least one of the two principal curvatures vanishes. Suppose $\Pi \subset \mathbb{R}^{3}$ is a plane and $\Sigma$ is tangent to $\Pi$ along a smooth closed curve $C=\Pi \cap \Sigma \subset \Sigma$. Show that $C$ is composed of parabolic points.

  Can both principal curvatures vanish at a point of $C$ ? Briefly justify your answer.

  comment

2011

• Paper 1, Section I, F

  Geometry | Part IB, 2011

  Suppose that $H \subseteq \mathbb{C}$ is the upper half-plane, $H=\{x+i y \mid x, y \in \mathbb{R}, y>0\}$. Using the Riemannian metric $d s^{2}=\frac{d x^{2}+d y^{2}}{y^{2}}$, define the length of a curve $\gamma$ and the area of a region $\Omega$ in $H$.

  Find the area of

  $$\Omega=\left\{x+i y|| x \mid \leqslant \frac{1}{2}, x^{2}+y^{2} \geqslant 1\right\}$$

  comment

• Paper 2, Section II, F

  Geometry | Part IB, 2011

  Suppose that $\pi: S^{2} \rightarrow \mathbb{C}_{\infty}$ is stereographic projection. Show that, via $\pi$, every rotation of $S^{2}$ corresponds to a Möbius transformation in $P S U(2)$.

  comment

• Paper 3, Section I, F

  Geometry | Part IB, 2011

  Let $R(x, \theta)$ denote anti-clockwise rotation of the Euclidean plane $\mathbb{R}^{2}$ through an angle $\theta$ about a point $x$.

  Show that $R(x, \theta)$ is a composite of two reflexions.

  Assume $\theta, \phi \in(0, \pi)$. Show that the composite $R(y, \phi) \cdot R(x, \theta)$ is also a rotation $R(z, \psi)$. Find $z$ and $\psi$.

  comment

• Paper 3, Section II, F

  Geometry | Part IB, 2011

  Suppose that $\eta(u)=(f(u), 0, g(u))$ is a unit speed curve in $\mathbb{R}^{3}$. Show that the corresponding surface of revolution $S$ obtained by rotating this curve about the $z$-axis has Gaussian curvature $K=-\left(d^{2} f / d u^{2}\right) / f$.

  comment

• Paper 4, Section II, F

  Geometry | Part IB, 2011

  Suppose that $P$ is a point on a Riemannian surface $S$. Explain the notion of geodesic polar co-ordinates on $S$ in a neighbourhood of $P$, and prove that if $C$ is a geodesic circle centred at $P$ of small positive radius, then the geodesics through $P$ meet $C$ at right angles.

  comment

2010

• Paper 1, Section I, F

  Geometry | Part IB, 2010

  (i) Define the notion of curvature for surfaces embedded in $\mathbb{R}^{3}$.

  (ii) Prove that the unit sphere in $\mathbb{R}^{3}$ has curvature $+1$ at all points.

  comment

• Paper 2, Section II, F

  Geometry | Part IB, 2010

  Suppose that $a>0$ and that $S \subset \mathbb{R}^{3}$ is the half-cone defined by $z^{2}=a\left(x^{2}+y^{2}\right)$, $z>0$. By using an explicit smooth parametrization of $S$, calculate the curvature of $S$.

  Describe the geodesics on $S$. Show that for $a=3$, no geodesic intersects itself, while for $a>3$ some geodesic does so.

  comment

• Paper 3, Section I, F

  Geometry | Part IB, 2010

  (i) Write down the Poincaré metric on the unit disc model $D$ of the hyperbolic plane. Compute the hyperbolic distance $\rho$ from $(0,0)$ to $(r, 0)$, with $0<r<1$.

  (ii) Given a point $P$ in $D$ and a hyperbolic line $L$ in $D$ with $P$ not on $L$, describe how the minimum distance from $P$ to $L$ is calculated. Justify your answer.

  comment

• Paper 3, Section II, F

  Geometry | Part IB, 2010

  Describe the hyperbolic metric on the upper half-plane $H$. Show that any Möbius transformation that preserves $H$ is an isometry of this metric.

  Suppose that $z_{1}, z_{2} \in H$ are distinct and that the hyperbolic line through $z_{1}$ and $z_{2}$ meets the real axis at $w_{1}, w_{2}$. Show that the hyperbolic distance $\rho\left(z_{1}, z_{2}\right)$ between $z_{1}$ and $z_{2}$ is given by $\rho\left(z_{1}, z_{2}\right)=\log r$, where $r$ is the cross-ratio of the four points $z_{1}, z_{2}, w_{1}, w_{2}$, taken in an appropriate order.

  comment

• Paper 4, Section II, F

  Geometry | Part IB, 2010

  Suppose that $D$ is the unit disc, with Riemannian metric

  $$d s^{2}=\frac{d x^{2}+d y^{2}}{1-\left(x^{2}+y^{2}\right)}$$

  [Note that this is not a multiple of the Poincaré metric.] Show that the diameters of $D$ are, with appropriate parametrization, geodesics.

  Show that distances between points in $D$ are bounded, but areas of regions in $D$ are unbounded.

  comment

2009

• Paper 1, Section I, G

  Geometry | Part IB, 2009

  What is an ideal hyperbolic triangle? State a formula for its area.

  Compute the area of a hyperbolic disk of hyperbolic radius $\rho$. Hence, or otherwise, show that no hyperbolic triangle completely contains a hyperbolic circle of hyperbolic radius $2 .$

  comment

• Paper 2, Section II, G

  Geometry | Part IB, 2009

  What is meant by stereographic projection from the unit sphere in $\mathbb{R}^{3}$ to the complex plane? Briefly explain why a spherical triangle cannot map to a Euclidean triangle under stereographic projection.

  Derive an explicit formula for stereographic projection. Hence, or otherwise, prove that if a Möbius map corresponds via stereographic projection to a rotation of the sphere, it has two fixed points $p$ and $q$ which satisfy $p \bar{q}=-1$. Give, with justification:

  (i) a Möbius transformation which fixes a pair of points $p, q \in \mathbb{C}$ satisfying $p \bar{q}=-1$ but which does not arise from a rotation of the sphere;

  (ii) an isometry of the sphere (for the spherical metric) which does not correspond to any Möbius transformation under stereographic projection.

  comment

• Paper 3, Section I, G

  Geometry | Part IB, 2009

  Write down the equations for geodesic curves on a surface. Use these to describe all the geodesics on a circular cylinder, and draw a picture illustrating your answer.

  comment

• Paper 3, Section II, G

  Geometry | Part IB, 2009

  Consider a tessellation of the two-dimensional sphere, that is to say a decomposition of the sphere into polygons each of which has at least three sides. Let $E, V$ and $F$ denote the numbers of edges, vertices and faces in the tessellation, respectively. State Euler's formula. Prove that $2 E \geqslant 3 F$. Deduce that not all the vertices of the tessellation have valence $\geqslant 6$.

  By considering the plane $\{z=1\} \subset \mathbb{R}^{3}$, or otherwise, deduce the following: if $\Sigma$ is a finite set of straight lines in the plane $\mathbb{R}^{2}$ with the property that every intersection point of two lines is an intersection point of at least three, then all the lines in $\Sigma$ meet at a single point.

  comment

• Paper 4, Section II, G

  Geometry | Part IB, 2009

  Let $U \subset \mathbb{R}^{2}$ be an open set. Let $\Sigma \subset \mathbb{R}^{3}$ be a surface locally given as the graph of an infinitely-differentiable function $f: U \rightarrow \mathbb{R}$. Compute the Gaussian curvature of $\Sigma$ in terms of $f$.

  Deduce that if $\widehat{\Sigma} \subset \mathbb{R}^{3}$ is a compact surface without boundary, its Gaussian curvature is not everywhere negative.

  Give, with brief justification, a compact surface $\widehat{\Sigma} \subset \mathbb{R}^{3}$ without boundary whose Gaussian curvature must change sign.

  comment

2008

• 1.I.2G

  Geometry | Part IB, 2008

  Show that any element of $S O(3, \mathbb{R})$ is a rotation, and that it can be written as the product of two reflections.

  comment

• 2.II.12G

  Geometry | Part IB, 2008

  Show that the area of a spherical triangle with angles $\alpha, \beta, \gamma$ is $\alpha+\beta+\gamma-\pi$. Hence derive the formula for the area of a convex spherical $n$-gon.

  Deduce Euler's formula $F-E+V=2$ for a decomposition of a sphere into $F$ convex polygons with a total of $E$ edges and $V$ vertices.

  A sphere is decomposed into convex polygons, comprising $m$ quadrilaterals, $n$ pentagons and $p$ hexagons, in such a way that at each vertex precisely three edges meet. Show that there are at most 7 possibilities for the pair $(m, n)$, and that at least 3 of these do occur.

  comment

• 3.I.2G

  Geometry | Part IB, 2008

  A smooth surface in $\mathbb{R}^{3}$ has parametrization

  $$\sigma(u, v)=\left(u-\frac{u^{3}}{3}+u v^{2}, v-\frac{v^{3}}{3}+u^{2} v, u^{2}-v^{2}\right) .$$

  Show that a unit normal vector at the point $\sigma(u, v)$ is

  $$\left(\frac{-2 u}{1+u^{2}+v^{2}}, \frac{2 v}{1+u^{2}+v^{2}}, \frac{1-u^{2}-v^{2}}{1+u^{2}+v^{2}}\right)$$

  and that the curvature is $\frac{-4}{\left(1+u^{2}+v^{2}\right)^{4}}$.

  comment

• 3.II.12G

  Geometry | Part IB, 2008

  Let $D$ be the unit disc model of the hyperbolic plane, with metric

  $$\frac{4|d \zeta|^{2}}{\left(1-|\zeta|^{2}\right)^{2}}$$

  (i) Show that the group of Möbius transformations mapping $D$ to itself is the group of transformations

  $$\zeta \mapsto \omega \frac{\zeta-\lambda}{\bar{\lambda} \zeta-1},$$

  where $|\lambda|<1$ and $|\omega|=1$.

  (ii) Assuming that the transformations in (i) are isometries of $D$, show that any hyperbolic circle in $D$ is a Euclidean circle.

  (iii) Let $P$ and $Q$ be points on the unit circle with $\angle P O Q=2 \alpha$. Show that the hyperbolic distance from $O$ to the hyperbolic line $P Q$ is given by

  $$2 \tanh ^{-1}\left(\frac{1-\sin \alpha}{\cos \alpha}\right)$$

  (iv) Deduce that if $a>2 \tanh ^{-1}(2-\sqrt{3})$ then no hyperbolic open disc of radius $a$ is contained in a hyperbolic triangle.

  comment

• 4.II.12G

  Geometry | Part IB, 2008

  Let $\gamma:[a, b] \rightarrow S$ be a curve on a smoothly embedded surface $S \subset \mathbf{R}^{3}$. Define the energy of $\gamma$. Show that if $\gamma$ is a stationary point for the energy for proper variations of $\gamma$, then $\gamma$ satisfies the geodesic equations

  $$\begin{aligned} \frac{d}{d t}\left(E \dot{\gamma}_{1}+F \dot{\gamma}_{2}\right) &=\frac{1}{2}\left(E_{u} \dot{\gamma}_{1}^{2}+2 F_{u} \dot{\gamma}_{1} \dot{\gamma}_{2}+G_{u} \dot{\gamma}_{2}^{2}\right) \\ \frac{d}{d t}\left(F \dot{\gamma}_{1}+G \dot{\gamma}_{2}\right) &=\frac{1}{2}\left(E_{v} \dot{\gamma}_{1}^{2}+2 F_{v} \dot{\gamma}_{1} \dot{\gamma}_{2}+G_{v} \dot{\gamma}_{2}^{2}\right) \end{aligned}$$

  where $\gamma=\left(\gamma_{1}, \gamma_{2}\right)$ in terms of a smooth parametrization $(u, v)$ for $S$, with first fundamental form $E d u^{2}+2 F d u d v+G d v^{2}$.

  Now suppose that for every $c, d$ the curves $u=c, v=d$ are geodesics.

  (i) Show that $(F / \sqrt{G})_{v}=(\sqrt{G})_{u}$ and $(F / \sqrt{E})_{u}=(\sqrt{E})_{v}$.

  (ii) Suppose moreover that the angle between the curves $u=c, v=d$ is independent of $c$ and $d$. Show that $E_{v}=0=G_{u}$.

  comment

2007

• 1.I.2A

  Geometry | Part IB, 2007

  State the Gauss-Bonnet theorem for spherical triangles, and deduce from it that for each convex polyhedron with $F$ faces, $E$ edges, and $V$ vertices, $F-E+V=2$.

  comment

• 2.II.12A

  Geometry | Part IB, 2007

  (i) The spherical circle with centre $P \in S^{2}$ and radius $r, 0<r<\pi$, is the set of all points on the unit sphere $S^{2}$ at spherical distance $r$ from $P$. Find the circumference of a spherical circle with spherical radius $r$. Compare, for small $r$, with the formula for a Euclidean circle and comment on the result.

  (ii) The cross ratio of four distinct points $z_{i}$ in $\mathbf{C}$ is

  $$\frac{\left(z_{4}-z_{1}\right)\left(z_{2}-z_{3}\right)}{\left(z_{4}-z_{3}\right)\left(z_{2}-z_{1}\right)} .$$

  Show that the cross-ratio is a real number if and only if $z_{1}, z_{2}, z_{3}, z_{4}$ lie on a circle or a line.

  [You may assume that Möbius transformations preserve the cross-ratio.]

  comment

• 3.I $2 \mathrm{~A} \quad$

  Geometry | Part IB, 2007

  Let $l$ be a line in the Euclidean plane $\mathbf{R}^{2}$ and $P$ a point on $l$. Denote by $\rho$ the reflection in $l$ and by $\tau$ the rotation through an angle $\alpha$ about $P$. Describe, in terms of $l, P$, and $\alpha$, a line fixed by the composition $\tau \rho$ and show that $\tau \rho$ is a reflection.

  comment

• 3.II.12A

  Geometry | Part IB, 2007

  For a parameterized smooth embedded surface $\sigma: V \rightarrow U \subset \mathbf{R}^{3}$, where $V$ is an open domain in $\mathbf{R}^{2}$, define the first fundamental form, the second fundamental form, and the Gaussian curvature $K$. State the Gauss-Bonnet formula for a compact embedded surface $S \subset \mathbf{R}^{3}$ having Euler number $e(S)$.

  Let $S$ denote a surface defined by rotating a curve

  $$\eta(u)=(r+a \sin u, 0, b \cos u) \quad 0 \leq u \leq 2 \pi$$

  about the $z$-axis. Here $a, b, r$ are positive constants, such that $a^{2}+b^{2}=1$ and $a<r$. By considering a smooth parameterization, find the first fundamental form and the second fundamental form of $S$.

  comment

• 4.II.12A

  Geometry | Part IB, 2007

  Write down the Riemannian metric for the upper half-plane model $\mathbf{H}$ of the hyperbolic plane. Describe, without proof, the group of isometries of $\mathbf{H}$ and the hyperbolic lines (i.e. the geodesics) on $\mathbf{H}$.

  Show that for any two hyperbolic lines $\ell_{1}, \ell_{2}$, there is an isometry of $\mathbf{H}$ which maps $\ell_{1}$ onto $\ell_{2}$.

  Suppose that $g$ is a composition of two reflections in hyperbolic lines which are ultraparallel (i.e. do not meet either in the hyperbolic plane or at its boundary). Show that $g$ cannot be an element of finite order in the group of isometries of $\mathbf{H}$.

  [Existence of a common perpendicular to two ultraparallel hyperbolic lines may be assumed. You might like to choose carefully which hyperbolic line to consider as a common perpendicular.]

  comment

2006

• 1.I $2 \mathrm{H} \quad$

  Geometry | Part IB, 2006

  Define the hyperbolic metric in the upper half-plane model $H$ of the hyperbolic plane. How does one define the hyperbolic area of a region in $H$ ? State the Gauss-Bonnet theorem for hyperbolic triangles.

  Let $R$ be the region in $H$ defined by

  $$0<x<\frac{1}{2}, \quad \sqrt{1-x^{2}}<y<1$$

  Calculate the hyperbolic area of $R$.

  comment

• 2.II.12H

  Geometry | Part IB, 2006

  Let $\sigma: V \rightarrow U \subset \mathbf{R}^{3}$ denote a parametrized smooth embedded surface, where $V$ is an open ball in $\mathbf{R}^{2}$ with coordinates $(u, v)$. Explain briefly the geometric meaning of the second fundamental form

  $$L d u^{2}+2 M d u d v+N d v^{2},$$

  where $L=\sigma_{u u} \cdot \mathbf{N}, M=\sigma_{u v} \cdot \mathbf{N}, N=\sigma_{v v} \cdot \mathbf{N}$, with $\mathbf{N}$ denoting the unit normal vector to the surface $U$.

  Prove that if the second fundamental form is identically zero, then $\mathbf{N}_{u}=\mathbf{0}=\mathbf{N}_{v}$ as vector-valued functions on $V$, and hence that $\mathbf{N}$ is a constant vector. Deduce that $U$ is then contained in a plane given by $\mathbf{x} \cdot \mathbf{N}=$ constant.

  comment

• 3.I.2H

  Geometry | Part IB, 2006

  Show that the Gaussian curvature $K$ at an arbitrary point $(x, y, z)$ of the hyperboloid $z=x y$, as an embedded surface in $\mathbf{R}^{3}$, is given by the formula

  $$K=-1 /\left(1+x^{2}+y^{2}\right)^{2} .$$

  comment

• 3.II.12H

  Geometry | Part IB, 2006

  Describe the stereographic projection map from the sphere $S^{2}$ to the extended complex plane $\mathbf{C}_{\infty}$, positioned equatorially. Prove that $w, z \in \mathbf{C}_{\infty}$ correspond to antipodal points on $S^{2}$ if and only if $w=-1 / \bar{z}$. State, without proof, a result which relates the rotations of $S^{2}$ to a certain group of Möbius transformations on $\mathbf{C}_{\infty}$.

  Show that any circle in the complex plane corresponds, under stereographic projection, to a circle on $S^{2}$. Let $C$ denote any circle in the complex plane other than the unit circle; show that $C$ corresponds to a great circle on $S^{2}$ if and only if its intersection with the unit circle consists of two points, one of which is the negative of the other.

  [You may assume the result that a Möbius transformation on the complex plane sends circles and straight lines to circles and straight lines.]

  comment

• 4.II.12H

  Geometry | Part IB, 2006

  Describe the hyperbolic lines in both the disc and upper half-plane models of the hyperbolic plane. Given a hyperbolic line $l$ and a point $P \notin l$, we define

  $$d(P, l):=\inf _{Q \in l} \rho(P, Q)$$

  where $\rho$ denotes the hyperbolic distance. Show that $d(P, l)=\rho\left(P, Q^{\prime}\right)$, where $Q^{\prime}$ is the unique point of $l$ for which the hyperbolic line segment $P Q^{\prime}$ is perpendicular to $l$.

  Suppose now that $L_{1}$ is the positive imaginary axis in the upper half-plane model of the hyperbolic plane, and $L_{2}$ is the semicircle with centre $a>0$ on the real line, and radius $r$, where $0<r<a$. For any $P \in L_{2}$, show that the hyperbolic line through $P$ which is perpendicular to $L_{1}$ is a semicircle centred on the origin of radius $\leqslant a+r$, and prove that

  $$d\left(P, L_{1}\right) \geqslant \frac{a-r}{a+r} .$$

  For arbitrary hyperbolic lines $L_{1}, L_{2}$ in the hyperbolic plane, we define

  $$d\left(L_{1}, L_{2}\right):=\inf _{P \in L_{1}, Q \in L_{2}} \rho(P, Q)$$

  If $L_{1}$ and $L_{2}$ are ultraparallel (i.e. hyperbolic lines which do not meet, either inside the hyperbolic plane or at its boundary), prove that $d\left(L_{1}, L_{2}\right)$ is strictly positive.

  [The equivalence of the disc and upper half-plane models of the hyperbolic plane, and standard facts about the metric and isometries of these models, may be quoted without proof.]

  comment

2005

• 1.I $2 \mathrm{~A} \quad$

  Geometry | Part IB, 2005

  Let $\sigma: \mathbf{R}^{2} \rightarrow \mathbf{R}^{3}$ be the map defined by

  $$\sigma(u, v)=((a+b \cos u) \cos v,(a+b \cos u) \sin v, b \sin u)$$

  where $0<b<a$. Describe briefly the image $T=\sigma\left(\mathbf{R}^{2}\right) \subset \mathbf{R}^{3}$. Let $V$ denote the open subset of $\mathbf{R}^{2}$ given by $0<u<2 \pi, 0<v<2 \pi$; prove that the restriction $\sigma_{V}$ defines a smooth parametrization of a certain open subset (which you should specify) of $T$. Hence, or otherwise, prove that $T$ is a smooth embedded surface in $\mathbf{R}^{3}$.

  [You may assume that the image under $\sigma$ of any open set $B \subset \mathbf{R}^{2}$ is open in $T$.]

  comment

• 2.II.12A

  Geometry | Part IB, 2005

  Let $U$ be an open subset of $\mathbf{R}^{2}$ equipped with a Riemannian metric. For $\gamma:[0,1] \rightarrow U$ a smooth curve, define what is meant by its length and energy. Prove that length $(\gamma)^{2} \leq \operatorname{energy}(\gamma)$, with equality if and only if $\dot{\gamma}$ has constant norm with respect to the metric.

  Suppose now $U$ is the upper half plane model of the hyperbolic plane, and $P, Q$ are points on the positive imaginary axis. Show that a smooth curve $\gamma$ joining $P$ and $Q$ represents an absolute minimum of the length of such curves if and only if $\gamma(t)=i v(t)$, with $v$ a smooth monotonic real function.

  Suppose that a smooth curve $\gamma$ joining the above points $P$ and $Q$ represents a stationary point for the energy under proper variations; deduce from an appropriate form of the Euler-Lagrange equations that $\gamma$ must be of the above form, with $\dot{v} / v$ constant.

  comment

• 3.I.2A

  Geometry | Part IB, 2005

  Write down the Riemannian metric on the disc model $\Delta$ of the hyperbolic plane. Given that the length minimizing curves passing through the origin correspond to diameters, show that the hyperbolic circle of radius $\rho$ centred on the origin is just the Euclidean circle centred on the origin with Euclidean $\operatorname{radius~} \tanh (\rho / 2)$. Prove that the hyperbolic area is $2 \pi(\cosh \rho-1)$.

  State the Gauss-Bonnet theorem for the area of a hyperbolic triangle. Given a hyperbolic triangle and an interior point $P$, show that the distance from $P$ to the nearest side is at most $\cosh ^{-1}(3 / 2)$.

  comment

• 3.II.12A

  Geometry | Part IB, 2005

  Describe geometrically the stereographic projection map $\pi$ from the unit sphere $S^{2}$ to the extended complex plane $\mathbf{C}_{\infty}=\mathbf{C} \cup\{\infty\}$, positioned equatorially, and find a formula for $\pi$.

  Show that any Möbius transformation $T \neq 1$ on $\mathbf{C}_{\infty}$ has one or two fixed points. Show that the Möbius transformation corresponding (under the stereographic projection map) to a rotation of $S^{2}$ through a non-zero angle has exactly two fixed points $z_{1}$ and $z_{2}$, where $z_{2}=-1 / \bar{z}_{1}$. If now $T$ is a Möbius transformation with two fixed points $z_{1}$ and $z_{2}$ satisfying $z_{2}=-1 / \bar{z}_{1}$, prove that either $T$ corresponds to a rotation of $S^{2}$, or one of the fixed points, say $z_{1}$, is an attractive fixed point, i.e. for $z \neq z_{2}, T^{n} z \rightarrow z_{1}$ as $n \rightarrow \infty$.

  [You may assume the fact that any rotation of $S^{2}$ corresponds to some Möbius transformation of $\mathbf{C}_{\infty}$ under the stereographic projection map.]

  comment

• 4.II.12A

  Geometry | Part IB, 2005

  Given a parametrized smooth embedded surface $\sigma: V \rightarrow U \subset \mathbf{R}^{3}$, where $V$ is an open subset of $\mathbf{R}^{2}$ with coordinates $(u, v)$, and a point $P \in U$, define what is meant by the tangent space at $P$, the unit normal $\mathbf{N}$ at $P$, and the first fundamental form

  $$E d u^{2}+2 F d u d v+G d v^{2} .$$

  [You need not show that your definitions are independent of the parametrization.]

  The second fundamental form is defined to be

  $$L d u^{2}+2 M d u d v+N d v^{2},$$

  where $L=\sigma_{u u} \cdot \mathbf{N}, M=\sigma_{u v} \cdot \mathbf{N}$ and $N=\sigma_{v v} \cdot \mathbf{N}$. Prove that the partial derivatives of $\mathbf{N}$ (considered as a vector-valued function of $u, v$ ) are of the form $\mathbf{N}_{u}=a \sigma_{u}+b \sigma_{v}$, $\mathbf{N}_{v}=c \sigma_{u}+d \sigma_{v}$, where

  $$-\left(\begin{array}{cc} L & M \\ M & N \end{array}\right)=\left(\begin{array}{ll} a & b \\ c & d \end{array}\right)\left(\begin{array}{ll} E & F \\ F & G \end{array}\right)$$

  Explain briefly the significance of the determinant $a d-b c$.

  comment

2004

• 1.I.3G

  Geometry | Part IB, 2004

  Using the Riemannian metric

  $$d s^{2}=\frac{d x^{2}+d y^{2}}{y^{2}}$$

  define the length of a curve and the area of a region in the upper half-plane $H=\{x+i y: y>0\}$.

  Find the hyperbolic area of the region $\{(x, y) \in H: 0<x<1, y>1\}$.

  comment

• 1.II.14G

  Geometry | Part IB, 2004

  Show that for every hyperbolic line $L$ in the hyperbolic plane $H$ there is an isometry of $H$ which is the identity on $L$ but not on all of $H$. Call it the reflection $R_{L}$.

  Show that every isometry of $H$ is a composition of reflections.

  comment

• 3.I.3G

  Geometry | Part IB, 2004

  State Euler's formula for a convex polyhedron with $F$ faces, $E$ edges, and $V$ vertices.

  Show that any regular polyhedron whose faces are pentagons has the same number of vertices, edges and faces as the dodecahedron.

  comment

• 3.II.15G

  Geometry | Part IB, 2004

  Let $a, b, c$ be the lengths of a right-angled triangle in spherical geometry, where $c$ is the hypotenuse. Prove the Pythagorean theorem for spherical geometry in the form

  $$\cos c=\cos a \cos b$$

  Now consider such a spherical triangle with the sides $a, b$ replaced by $\lambda a, \lambda b$ for a positive number $\lambda$. Show that the above formula approaches the usual Pythagorean theorem as $\lambda$ approaches zero.

  comment

2003

• 1.I.4F

  Geometry | Part IB, 2003

  Describe the geodesics (that is, hyperbolic straight lines) in either the disc model or the half-plane model of the hyperbolic plane. Explain what is meant by the statements that two hyperbolic lines are parallel, and that they are ultraparallel.

  Show that two hyperbolic lines $l$ and $l^{\prime}$ have a unique common perpendicular if and only if they are ultraparallel.

  [You may assume standard results about the group of isometries of whichever model of the hyperbolic plane you use.]

  comment

• 1.II.13F

  Geometry | Part IB, 2003

  Write down the Riemannian metric in the half-plane model of the hyperbolic plane. Show that Möbius transformations mapping the upper half-plane to itself are isometries of this model.

  Calculate the hyperbolic distance from $i b$ to $i c$, where $b$ and $c$ are positive real numbers. Assuming that the hyperbolic circle with centre $i b$ and radius $r$ is a Euclidean circle, find its Euclidean centre and radius.

  Suppose that $a$ and $b$ are positive real numbers for which the points $i b$ and $a+i b$ of the upper half-plane are such that the hyperbolic distance between them coincides with the Euclidean distance. Obtain an expression for $b$ as a function of $a$. Hence show that, for any $b$ with $0<b<1$, there is a unique positive value of $a$ such that the hyperbolic distance between $i b$ and $a+i b$ coincides with the Euclidean distance.

  comment

• 3.I.4F

  Geometry | Part IB, 2003

  Show that any isometry of Euclidean 3 -space which fixes the origin can be written as a composite of at most three reflections in planes through the origin, and give (with justification) an example of an isometry for which three reflections are necessary.

  comment

• 3.II.14F

  Geometry | Part IB, 2003

  State and prove the Gauss-Bonnet formula for the area of a spherical triangle. Deduce a formula for the area of a spherical $n$-gon with angles $\alpha_{1}, \alpha_{2}, \ldots, \alpha_{n}$. For what range of values of $\alpha$ does there exist a (convex) regular spherical $n$-gon with angle $\alpha$ ?

  Let $\Delta$ be a spherical triangle with angles $\pi / p, \pi / q$ and $\pi / r$ where $p, q, r$ are integers, and let $G$ be the group of isometries of the sphere generated by reflections in the three sides of $\Delta$. List the possible values of $(p, q, r)$, and in each case calculate the order of the corresponding group $G$. If $(p, q, r)=(2,3,5)$, show how to construct a regular dodecahedron whose group of symmetries is $G$.

  [You may assume that the images of $\Delta$ under the elements of $G$ form a tessellation of the sphere.]

  comment

2002

• 1.I.4E

  Geometry | Part IB, 2002

  Show that any finite group of orientation-preserving isometries of the Euclidean plane is cyclic.

  Show that any finite group of orientation-preserving isometries of the hyperbolic plane is cyclic.

  [You may assume that given any non-empty finite set $E$ in the hyperbolic plane, or the Euclidean plane, there is a unique smallest closed disc that contains E. You may also use any general fact about the hyperbolic plane without proof providing that it is stated carefully.]

  comment

• 1.II.13E

  Geometry | Part IB, 2002

  Let $\mathbb{H}=\{x+i y \in \mathbb{C}: y>0\}$, and let $\mathbb{H}$ have the hyperbolic metric $\rho$ derived from the line element $|d z| / y$. Let $\Gamma$ be the group of Möbius maps of the form $z \mapsto(a z+b) /(c z+d)$, where $a, b, c$ and $d$ are real and $a d-b c=1$. Show that every $g$ in $\Gamma$ is an isometry of the metric space $(\mathbb{H}, \rho)$. For $z$ and $w$ in $\mathbb{H}$, let

  $$h(z, w)=\frac{|z-w|^{2}}{\operatorname{Im}(z) \operatorname{Im}(w)}$$

  Show that for every $g$ in $\Gamma, h(g(z), g(w))=h(z, w)$. By considering $z=i y$, where $y>1$, and $w=i$, or otherwise, show that for all $z$ and $w$ in $\mathbb{H}$,

  $$\cosh \rho(z, w)=1+\frac{|z-w|^{2}}{2 \operatorname{Im}(z) \operatorname{Im}(w)}$$

  By considering points $i, i y$, where $y>1$ and $s+i t$, where $s^{2}+t^{2}=1$, or otherwise, derive Pythagoras' Theorem for hyperbolic geometry in the form $\cosh a \cosh b=\cosh c$, where $a, b$ and $c$ are the lengths of sides of a right-angled triangle whose hypotenuse has length $c$.

  comment

• 3.I.4E

  Geometry | Part IB, 2002

  State Euler's formula for a graph $\mathcal{G}$ with $F$ faces, $E$ edges and $V$ vertices on the surface of a sphere.

  Suppose that every face in $\mathcal{G}$ has at least three edges, and that at least three edges meet at every vertex of $\mathcal{G}$. Let $F_{n}$ be the number of faces of $\mathcal{G}$ that have exactly $n$ edges $(n \geqslant 3)$, and let $V_{m}$ be the number of vertices at which exactly $m$ edges meet $(m \geqslant 3)$. By expressing $6 F-\sum_{n} n F_{n}$ in terms of the $V_{j}$, or otherwise, show that every convex polyhedron has at least four faces each of which is a triangle, a quadrilateral or a pentagon.

  comment

• 3.II.14E

  Geometry | Part IB, 2002

  Show that every isometry of Euclidean space $\mathbb{R}^{3}$ is a composition of reflections in planes

  What is the smallest integer $N$ such that every isometry $f$ of $\mathbb{R}^{3}$ with $f(0)=0$ can be expressed as the composition of at most $N$ reflections? Give an example of an isometry that needs this number of reflections and justify your answer.

  Describe (geometrically) all twelve orientation-reversing isometries of a regular tetrahedron.

  comment

2001

• 1.I.4B

  Geometry | Part IB, 2001

  Write down the Riemannian metric on the disc model $\Delta$ of the hyperbolic plane. What are the geodesics passing through the origin? Show that the hyperbolic circle of radius $\rho$ centred on the origin is just the Euclidean circle centred on the origin with Euclidean radius $\tanh (\rho / 2)$.

  Write down an isometry between the upper half-plane model $H$ of the hyperbolic plane and the disc model $\Delta$, under which $i \in H$ corresponds to $0 \in \Delta$. Show that the hyperbolic circle of radius $\rho$ centred on $i$ in $H$ is a Euclidean circle with centre $i \cosh \rho$ and of radius $\sinh \rho$.

  comment

• 1.II.13B

  Geometry | Part IB, 2001

  Describe geometrically the stereographic projection map $\phi$ from the unit sphere $S^{2}$ to the extended complex plane $\mathbb{C}_{\infty}=\mathbb{C} \cup \infty$, and find a formula for $\phi$. Show that any rotation of $S^{2}$ about the $z$-axis corresponds to a Möbius transformation of $\mathbb{C}_{\infty}$. You are given that the rotation of $S^{2}$ defined by the matrix

  $$\left(\begin{array}{rrr} 0 & 0 & 1 \\ 0 & 1 & 0 \\ -1 & 0 & 0 \end{array}\right)$$

  corresponds under $\phi$ to a Möbius transformation of $\mathbb{C}_{\infty}$; deduce that any rotation of $S^{2}$ about the $x$-axis also corresponds to a Möbius transformation.

  Suppose now that $u, v \in \mathbb{C}$ correspond under $\phi$ to distinct points $P, Q \in S^{2}$, and let $d$ denote the angular distance from $P$ to $Q$ on $S^{2}$. Show that $-\tan ^{2}(d / 2)$ is the cross-ratio of the points $u, v,-1 / \bar{u},-1 / \bar{v}$, taken in some order (which you should specify). [You may assume that the cross-ratio is invariant under Möbius transformations.]

  comment

• 3.I.4B

  Geometry | Part IB, 2001

  State and prove the Gauss-Bonnet theorem for the area of a spherical triangle.

  Suppose $\mathbf{D}$ is a regular dodecahedron, with centre the origin. Explain how each face of $\mathbf{D}$ gives rise to a spherical pentagon on the 2 -sphere $S^{2}$. For each such spherical pentagon, calculate its angles and area.

  comment

• 3.II.14B

  Geometry | Part IB, 2001

  Describe the hyperbolic lines in the upper half-plane model $H$ of the hyperbolic plane. The group $G=\mathrm{SL}(2, \mathbb{R}) /\{\pm I\}$ acts on $H$ via Möbius transformations, which you may assume are isometries of $H$. Show that $G$ acts transitively on the hyperbolic lines. Find explicit formulae for the reflection in the hyperbolic line $L$ in the cases (i) $L$ is a vertical line $x=a$, and (ii) $L$ is the unit semi-circle with centre the origin. Verify that the composite $T$ of a reflection of type (ii) followed afterwards by one of type (i) is given by $T(z)=-z^{-1}+2 a$.

  Suppose now that $L_{1}$ and $L_{2}$ are distinct hyperbolic lines in the hyperbolic plane, with $R_{1}, R_{2}$ denoting the corresponding reflections. By considering different models of the hyperbolic plane, or otherwise, show that

  (a) $R_{1} R_{2}$ has infinite order if $L_{1}$ and $L_{2}$ are parallel or ultraparallel, and

  (b) $R_{1} R_{2}$ has finite order if and only if $L_{1}$ and $L_{2}$ meet at an angle which is a rational multiple of $\pi$.

  comment