Applications Of Quantum Mechanics

2021

• Paper 1, Section II, B

  Applications of Quantum Mechanics | Part II, 2021

  (a) Discuss the variational principle that allows one to derive an upper bound on the energy $E_{0}$ of the ground state for a particle in one dimension subject to a potential $V(x)$.

  If $V(x)=V(-x)$, how could you adapt the variational principle to derive an upper bound on the energy $E_{1}$ of the first excited state?

  (b) Consider a particle of mass $2 m=\hbar^{2}$ (in certain units) subject to a potential

  $$V(x)=-V_{0} e^{-x^{2}} \quad \text { with } \quad V_{0}>0$$

  (i) Using the trial wavefunction

  $$\psi(x)=e^{-\frac{1}{2} x^{2} a}$$

  with $a>0$, derive the upper bound $E_{0} \leqslant E(a)$, where

  $$E(a)=\frac{1}{2} a-V_{0} \frac{\sqrt{a}}{\sqrt{1+a}}$$

  (ii) Find the zero of $E(a)$ in $a>0$ and show that any extremum must obey

  $$(1+a)^{3}=\frac{V_{0}^{2}}{a} .$$

  (iii) By sketching $E(a)$ or otherwise, deduce that there must always be a minimum in $a>0$. Hence deduce the existence of a bound state.

  (iv) Working perturbatively in $0<V_{0} \ll 1$, show that

  $$-V_{0}<E_{0} \leqslant-\frac{1}{2} V_{0}^{2}+\mathcal{O}\left(V_{0}^{3}\right)$$

  [Hint: You may use that $\int_{-\infty}^{\infty} e^{-b x^{2}} d x=\sqrt{\frac{\pi}{b}}$ for $\left.b>0 .\right]$

  comment

• Paper 2, Section II, 36B

  Applications of Quantum Mechanics | Part II, 2021

  (a) The $s$-wave solution $\psi_{0}$ for the scattering problem of a particle of mass $m$ and momentum $\hbar k$ has the asymptotic form

  $$\psi_{0}(r) \sim \frac{A}{r}[\sin (k r)+g(k) \cos (k r)]$$

  Define the phase shift $\delta_{0}$ and verify that $\tan \delta_{0}=g(k)$.

  (b) Define the scattering amplitude $f$. For a spherically symmetric potential of finite range, starting from $\sigma_{T}=\int|f|^{2} d \Omega$, derive the expression

  $$\sigma_{T}=\frac{4 \pi}{k^{2}} \sum_{l=0}^{\infty}(2 l+1) \sin ^{2} \delta_{l}$$

  giving the cross-section $\sigma_{T}$ in terms of the phase shifts $\delta_{l}$ of the partial waves.

  (c) For $g(k)=-k / K$ with $K>0$, show that a bound state exists and compute its energy. Neglecting the contributions from partial waves with $l>0$, show that

  $$\sigma_{T} \approx \frac{4 \pi}{K^{2}+k^{2}}$$

  (d) For $g(k)=\gamma /\left(K_{0}-k\right)$ with $K_{0}>0, \gamma>0$ compute the $s$-wave contribution to $\sigma_{T}$. Working to leading order in $\gamma \ll K_{0}$, show that $\sigma_{T}$ has a local maximum at $k=K_{0}$. Interpret this fact in terms of a resonance and compute its energy and decay width.

  comment

• Paper 3, Section II, 34B

  Applications of Quantum Mechanics | Part II, 2021

  (a) In three dimensions, define a Bravais lattice $\Lambda$ and its reciprocal lattice $\Lambda^{*}$.

  A particle is subject to a potential $V(\mathbf{x})$ with $V(\mathbf{x})=V(\mathbf{x}+\mathbf{r})$ for $\mathbf{x} \in \mathbb{R}^{3}$ and $\mathbf{r} \in \Lambda$. State and prove Bloch's theorem and specify how the Brillouin zone is related to the reciprocal lattice.

  (b) A body-centred cubic lattice $\Lambda_{B C C}$ consists of the union of the points of a cubic lattice $\Lambda_{1}$ and all the points $\Lambda_{2}$ at the centre of each cube:

  $$\begin{aligned} \Lambda_{B C C} & \equiv \Lambda_{1} \cup \Lambda_{2}, \\ \Lambda_{1} & \equiv\left\{\mathbf{r} \in \mathbb{R}^{3}: \mathbf{r}=n_{1} \hat{\mathbf{i}}+n_{2} \hat{\mathbf{j}}+n_{3} \hat{\mathbf{k}}, \text { with } n_{1,2,3} \in \mathbb{Z}\right\}, \\ \Lambda_{2} & \equiv\left\{\mathbf{r} \in \mathbb{R}^{3}: \mathbf{r}=\frac{1}{2}(\hat{\mathbf{i}}+\hat{\mathbf{j}}+\hat{\mathbf{k}})+\mathbf{r}^{\prime}, \text { with } \mathbf{r}^{\prime} \in \Lambda_{1}\right\}, \end{aligned}$$

  where $\hat{\mathbf{i}}, \hat{\mathbf{j}}$ and $\hat{\mathbf{k}}$ are unit vectors parallel to the Cartesian coordinates in $\mathbb{R}^{3}$. Show that $\Lambda_{B C C}$ is a Bravais lattice and determine the primitive vectors $\mathbf{a}_{1}, \mathbf{a}_{2}$ and $\mathbf{a}_{3}$.

  Find the reciprocal lattice $\Lambda_{B C C}^{*} .$ Briefly explain what sort of lattice it is.

  $\left[\right.$ Hint: The matrix $M=\frac{1}{2}\left(\begin{array}{ccc}-1 & 1 & 1 \\ 1 & -1 & 1 \\ 1 & 1 & -1\end{array}\right)$ has inverse $M^{-1}=\left(\begin{array}{lll}0 & 1 & 1 \\ 1 & 0 & 1 \\ 1 & 1 & 0\end{array}\right)$.

  comment

• Paper 4, Section II, B

  Applications of Quantum Mechanics | Part II, 2021

  (a) Consider the nearly free electron model in one dimension with mass $m$ and periodic potential $V(x)=\lambda U(x)$ with $0<\lambda \ll 1$ and

  $$U(x)=\sum_{l=-\infty}^{\infty} U_{l} \exp \left(\frac{2 \pi i}{a} l x\right)$$

  Ignoring degeneracies, the energy spectrum of Bloch states with wavenumber $k$ is

  $$E(k)=E_{0}(k)+\lambda\langle k|U| k\rangle+\lambda^{2} \sum_{k^{\prime} \neq k} \frac{\left\langle k|U| k^{\prime}\right\rangle\left\langle k^{\prime}|U| k\right\rangle}{E_{0}(k)-E_{0}\left(k^{\prime}\right)}+\mathcal{O}\left(\lambda^{3}\right)$$

  where $\{|k\rangle\}$ are normalized eigenstates of the free Hamiltonian with wavenumber $k$. What is $E_{0}$ in this formula?

  If we impose periodic boundary conditions on the wavefunctions, $\psi(x)=\psi(x+L)$ with $L=N a$ and $N$ a positive integer, what are the allowed values of $k$ and $k^{\prime}$ ? Determine $\left\langle k|U| k^{\prime}\right\rangle$ for these allowed values.

  (b) State when the above expression for $E(k)$ ceases to be a good approximation and explain why. Quoting any result you need from degenerate perturbation theory, calculate to $\mathcal{O}(\lambda)$ the location and width of the band gaps.

  (c) Determine the allowed energy bands for each of the potentials

  $$\begin{aligned} &\text { (i) } V(x)=2 \lambda \cos \left(\frac{2 \pi x}{a}\right) \text {, } \\ &\text { (ii) } V(x)=\lambda a \sum_{n=-\infty}^{\infty} \delta(x-n a) \text {. } \end{aligned}$$

  (d) Briefly discuss a macroscopic physical consequence of the existence of energy bands.

  comment

2020

• Paper 1, Section II, C

  Applications of Quantum Mechanics | Part II, 2020

  Consider the quantum mechanical scattering of a particle of mass $m$ in one dimension off a parity-symmetric potential, $V(x)=V(-x)$. State the constraints imposed by parity, unitarity and their combination on the components of the $S$-matrix in the parity basis,

  $$S=\left(\begin{array}{cc} S_{++} & S_{+-} \\ S_{-+} & S_{--} \end{array}\right)$$

  For the specific potential

  $$V=\hbar^{2} \frac{U_{0}}{2 m}\left[\delta_{D}(x+a)+\delta_{D}(x-a)\right]$$

  show that

  $$S_{--}=e^{-i 2 k a}\left[\frac{\left(2 k-i U_{0}\right) e^{i k a}+i U_{0} e^{-i k a}}{\left(2 k+i U_{0}\right) e^{-i k a}-i U_{0} e^{i k a}}\right]$$

  For $U_{0}<0$, derive the condition for the existence of an odd-parity bound state. For $U_{0}>0$ and to leading order in $U_{0} a \gg 1$, show that an odd-parity resonance exists and discuss how it evolves in time.

  comment

• Paper 2, Section II, $35 \mathrm{C}$

  Applications of Quantum Mechanics | Part II, 2020

  a) Consider a particle moving in one dimension subject to a periodic potential, $V(x)=V(x+a)$. Define the Brillouin zone. State and prove Bloch's theorem.

  b) Consider now the following periodic potential

  $$V=V_{0}(\cos (x)-\cos (2 x))$$

  with positive constant $V_{0}$.

  i) For very small $V_{0}$, use the nearly-free electron model to compute explicitly the lowest-energy band gap to leading order in degenerate perturbation theory.

  ii) For very large $V_{0}$, the electron is localised very close to a minimum of the potential. Estimate the two lowest energies for such localised eigenstates and use the tight-binding model to estimate the lowest-energy band gap.

  comment

• Paper 3, Section II, C

  Applications of Quantum Mechanics | Part II, 2020

  (a) For the quantum scattering of a beam of particles in three dimensions off a spherically symmetric potential $V(r)$ that vanishes at large $r$, discuss the boundary conditions satisfied by the wavefunction $\psi$ and define the scattering amplitude $f(\theta)$. Assuming the asymptotic form

  $$\psi=\sum_{l=0}^{\infty} \frac{2 l+1}{2 i k}\left[(-1)^{l+1} \frac{e^{-i k r}}{r}+\left(1+2 i f_{l}\right) \frac{e^{i k r}}{r}\right] P_{l}(\cos \theta),$$

  state the constraints on $f_{l}$ imposed by the unitarity of the $S$-matrix and define the phase shifts $\delta_{l}$.

  (b) For $V_{0}>0$, consider the specific potential

  $$V(r)=\left\{\begin{array}{lc} \infty, & r \leqslant a \\ -V_{0}, & a<r \leqslant 2 a \\ 0, & r>2 a \end{array}\right.$$

  (i) Show that the s-wave phase shift $\delta_{0}$ obeys

  $$\tan \left(\delta_{0}\right)=\frac{k \cos (2 k a)-\kappa \cot (\kappa a) \sin (2 k a)}{k \sin (2 k a)+\kappa \cot (\kappa a) \cos (2 k a)},$$

  where $\kappa^{2}=k^{2}+2 m V_{0} / \hbar^{2}$.

  (ii) Compute the scattering length $a_{s}$ and find for which values of $\kappa$ it diverges. Discuss briefly the physical interpretation of the divergences. [Hint: you may find this trigonometric identity useful

  $$\left.\tan (A+B)=\frac{\tan A+\tan B}{1-\tan A \tan B} .\right]$$

  comment

• Paper 4, Section II, $34 C$

  Applications of Quantum Mechanics | Part II, 2020

  (a) For a particle of charge $q$ moving in an electromagnetic field with vector potential $\boldsymbol{A}$ and scalar potential $\phi$, write down the classical Hamiltonian and the equations of motion.

  (b) Consider the vector and scalar potentials

  $$\boldsymbol{A}=\frac{B}{2}(-y, x, 0), \quad \phi=0$$

  (i) Solve the equations of motion. Define and compute the cyclotron frequency $\omega_{B}$.

  (ii) Write down the quantum Hamiltonian of the system in terms of the angular momentum operator

  $$L_{z}=x p_{y}-y p_{x}$$

  Show that the states

  $$\psi(x, y)=f(x+i y) e^{-\left(x^{2}+y^{2}\right) q B / 4 \hbar}$$

  for any function $f$, are energy eigenstates and compute their energy. Define Landau levels and discuss this result in relation to them.

  (iii) Show that for $f(w)=w^{M}$, the wavefunctions in ( $\dagger$ ) are eigenstates of angular momentum and compute the corresponding eigenvalue. These wavefunctions peak in a ring around the origin. Estimate its radius. Using these two facts or otherwise, estimate the degeneracy of Landau levels.

  comment

2019

• Paper 1, Section II, B

  Applications of Quantum Mechanics | Part II, 2019

  A particle of mass $m$ and charge $q$ moving in a uniform magnetic field $\mathbf{B}=\nabla \times \mathbf{A}=$ $(0,0, B)$ and electric field $\mathbf{E}=-\nabla \phi$ is described by the Hamiltonian

  $$H=\frac{1}{2 m}|\mathbf{p}-q \mathbf{A}|^{2}+q \phi$$

  where $\mathbf{p}$ is the canonical momentum.

  [ In the following you may use without proof any results concerning the spectrum of the harmonic oscillator as long as they are stated clearly.]

  (a) Let $\mathbf{E}=\mathbf{0}$. Choose a gauge which preserves translational symmetry in the $y$ direction. Determine the spectrum of the system, restricted to states with $p_{z}=0$. The system is further restricted to lie in a rectangle of area $A=L_{x} L_{y}$, with sides of length $L_{x}$ and $L_{y}$ parallel to the $x$ - and $y$-axes respectively. Assuming periodic boundary conditions in the $y$-direction, estimate the degeneracy of each Landau level.

  (b) Consider the introduction of an additional electric field $\mathbf{E}=(\mathcal{E}, 0,0)$. Choosing a suitable gauge (with the same choice of vector potential $\mathbf{A}$ as in part (a)), write down the resulting Hamiltonian. Find the energy spectrum for a particle on $\mathbb{R}^{3}$ again restricted to states with $p_{z}=0$.

  Define the group velocity of the electron and show that its $y$-component is given by $v_{y}=-\mathcal{E} / B$.

  When the system is further restricted to a rectangle of area $A$ as above, show that the previous degeneracy of the Landau levels is lifted and determine the resulting energy gap $\Delta E$ between the ground-state and the first excited state.

  comment

• Paper 2, Section II, B

  Applications of Quantum Mechanics | Part II, 2019

  Give an account of the variational principle for establishing an upper bound on the ground state energy of a Hamiltonian $H$.

  A particle of mass $m$ moves in one dimension and experiences the potential $V=A|x|^{n}$ with $n$ an integer. Use a variational argument to prove the virial theorem,

  $$2\langle T\rangle_{0}=n\langle V\rangle_{0}$$

  where $\langle\cdot\rangle_{0}$ denotes the expectation value in the true ground state. Deduce that there is no normalisable ground state for $n \leqslant-3$.

  For the case $n=1$, use the ansatz $\psi(x) \propto e^{-\alpha^{2} x^{2}}$ to find an estimate for the energy of the ground state.

  comment

• Paper 3, Section II, B

  Applications of Quantum Mechanics | Part II, 2019

  A Hamiltonian $H$ is invariant under the discrete translational symmetry of a Bravais lattice $\Lambda$. This means that there exists a unitary translation operator $T_{\mathbf{r}}$ such that $\left[H, T_{\mathbf{r}}\right]=0$ for all $\mathbf{r} \in \Lambda$. State and prove Bloch's theorem for $H$.

  Consider the two-dimensional Bravais lattice $\Lambda$ defined by the basis vectors

  $$\mathbf{a}_{1}=\frac{a}{2}(\sqrt{3}, 1), \quad \mathbf{a}_{2}=\frac{a}{2}(\sqrt{3},-1)$$

  Find basis vectors $\mathbf{b}_{1}$ and $\mathbf{b}_{2}$ for the reciprocal lattice. Sketch the Brillouin zone. Explain why the Brillouin zone has only two physically distinct corners. Show that the positions of these corners may be taken to be $\mathbf{K}=\frac{1}{3}\left(2 \mathbf{b}_{1}+\mathbf{b}_{2}\right)$ and $\mathbf{K}^{\prime}=\frac{1}{3}\left(\mathbf{b}_{1}+2 \mathbf{b}_{2}\right)$.

  The dynamics of a single electron moving on the lattice $\Lambda$ is described by a tightbinding model with Hamiltonian

  $$H=\sum_{\mathbf{r} \in \Lambda}\left[E_{0}|\mathbf{r}\rangle\langle\mathbf{r}|-\lambda\left(|\mathbf{r}\rangle\left\langle\mathbf{r}+\mathbf{a}_{1}|+| \mathbf{r}\right\rangle\left\langle\mathbf{r}+\mathbf{a}_{2}|+| \mathbf{r}+\mathbf{a}_{1}\right\rangle\left\langle\mathbf{r}|+| \mathbf{r}+\mathbf{a}_{2}\right\rangle\langle\mathbf{r}|\right)\right]$$

  where $E_{0}$ and $\lambda$ are real parameters. What is the energy spectrum as a function of the wave vector $\mathbf{k}$ in the Brillouin zone? How does the energy vary along the boundary of the Brillouin zone between $\mathbf{K}$ and $\mathbf{K}^{\prime}$ ? What is the width of the band?

  In a real material, each site of the lattice $\Lambda$ contains an atom with a certain valency. Explain how the conducting properties of the material depend on the valency.

  Suppose now that there is a second band, with minimum $E=E_{0}+\Delta$. For what values of $\Delta$ and the valency is the material an insulator?

  comment

• Paper 4, Section II, B

  Applications of Quantum Mechanics | Part II, 2019

  (a) A classical beam of particles scatters off a spherically symmetric potential $V(r)$. Draw a diagram to illustrate the differential cross-section $d \sigma / d \Omega$, and use this to derive an expression for $d \sigma / d \Omega$ in terms of the impact parameter $b$ and the scattering angle $\theta$.

  A quantum beam of particles of mass $m$ and momentum $p=\hbar k$ is incident along the $z$-axis and scatters off a spherically symmetric potential $V(r)$. Write down the asymptotic form of the wavefunction $\psi$ in terms of the scattering amplitude $f(\theta)$. By considering the probability current $\mathbf{J}=-i(\hbar / 2 m)\left(\psi^{\star} \nabla \psi-\left(\nabla \psi^{\star}\right) \psi\right)$, derive an expression for the differential cross-section $d \sigma / d \Omega$ in terms of $f(\theta)$.

  (b) The solution $\psi(\mathbf{r})$ of the radial Schrödinger equation for a particle of mass $m$ and wave number $k$ moving in a spherically symmetric potential $V(r)$ has the asymptotic form

  $$\psi(\mathbf{r}) \sim \sum_{l=0}^{\infty}\left[A_{l}(k) \frac{\sin \left(k r-\frac{l \pi}{2}\right)}{k r}-B_{l}(k) \frac{\cos \left(k r-\frac{l \pi}{2}\right)}{k r}\right] P_{l}(\cos \theta)$$

  valid for $k r \gg 1$, where $A_{l}(k)$ and $B_{l}(k)$ are constants and $P_{l}$ denotes the $l$ th Legendre polynomial. Define the S-matrix element $S_{l}$ and the corresponding phase shift $\delta_{l}$ for the partial wave of angular momentum $l$, in terms of $A_{l}(k)$ and $B_{l}(k)$. Define also the scattering length $a_{s}$ for the potential $V$.

  Outside some core region, $r>r_{0}$, the Schrödinger equation for some such potential is solved by the s-wave (i.e. $l=0$ ) wavefunction $\psi(\mathbf{r})=\psi(r)$ with,

  $$\psi(r)=\frac{e^{-i k r}}{r}+\frac{k+i \lambda \tanh (\lambda r)}{k-i \lambda} \frac{e^{i k r}}{r}$$

  where $\lambda>0$ is a constant. Extract the S-matrix element $S_{0}$, the phase shift $\delta_{0}$ and the scattering length $a_{s}$. Deduce that the potential $V(r)$ has a bound state of zero angular momentum and compute its energy. Give the form of the (un-normalised) bound state wavefunction in the region $r>r_{0}$.

  comment

2018

• Paper 1, Section II, A

  Applications of Quantum Mechanics | Part II, 2018

  A particle of mass $m$ moves in one dimension in a periodic potential $V(x)$ satisfying $V(x+a)=V(x)$. Define the Floquet matrix $F$. Show that $\operatorname{det} F=1$ and explain why Tr $F$ is real. Show that allowed bands occur for energies such that $(\operatorname{Tr} F)^{2}<4$. Consider the potential

  $$V(x)=-\frac{\hbar^{2} \lambda}{m} \sum_{n=-\infty}^{+\infty} \delta(x-n a)$$

  For states of negative energy, construct the Floquet matrix with respect to the basis of states $e^{\pm \mu x}$. Derive an inequality for the values of $\mu$ in an allowed energy band.

  For states of positive energy, construct the Floquet matrix with respect to the basis of states $e^{\pm i k x}$. Derive an inequality for the values of $k$ in an allowed energy band.

  Show that the state with zero energy lies in a forbidden region for $\lambda a>2$.

  comment

• Paper 2, Section II, A

  Applications of Quantum Mechanics | Part II, 2018

  Consider a one-dimensional chain of $2 N \gg 1$ atoms, each of mass $m$. Impose periodic boundary conditions. The forces between neighbouring atoms are modelled as springs, with alternating spring constants $\lambda$ and $\alpha \lambda$. In equilibrium, the separation between the atoms is $a$.

  Denote the position of the $n^{\text {th }}$atom as $x_{n}(t)$. Let $u_{n}(t)=x_{n}(t)-n a$ be the displacement from equilibrium. Write down the equations of motion of the system.

  Show that the longitudinal modes of vibration are labelled by a wavenumber $k$ that is restricted to lie in a Brillouin zone. Find the frequency spectrum. What is the frequency gap at the edge of the Brillouin zone? Show that the gap vanishes when $\alpha=1$. Determine approximations for the frequencies near the centre of the Brillouin zone. Plot the frequency spectrum. What is the speed of sound in this system?

  comment

• Paper 3, Section II, A

  Applications of Quantum Mechanics | Part II, 2018

  A beam of particles of mass $m$ and momentum $p=\hbar k$ is incident along the $z$-axis. The beam scatters off a spherically symmetric potential $V(r)$. Write down the asymptotic form of the wavefunction in terms of the scattering amplitude $f(\theta)$.

  The incoming plane wave and the scattering amplitude can be expanded in partial waves as,

  $$\begin{gathered} e^{i k r \cos \theta} \sim \frac{1}{2 i k r} \sum_{l=0}^{\infty}(2 l+1)\left(e^{i k r}-(-1)^{l} e^{-i k r}\right) P_{l}(\cos \theta) \\ f(\theta)=\sum_{l=0}^{\infty} \frac{2 l+1}{k} f_{l} P_{l}(\cos \theta) \end{gathered}$$

  where $P_{l}$ are Legendre polynomials. Define the $S$-matrix. Assuming that the S-matrix is unitary, explain why we can write

  $$f_{l}=e^{i \delta_{l}} \sin \delta_{l}$$

  for some real phase shifts $\delta_{l}$. Obtain an expression for the total cross-section $\sigma_{T}$ in terms of the phase shifts $\delta_{l}$.

  [Hint: You may use the orthogonality of Legendre polynomials:

  $$\left.\int_{-1}^{+1} d w P_{l}(w) P_{l^{\prime}}(w)=\frac{2}{2 l+1} \delta_{l l^{\prime}} .\right]$$

  Consider the repulsive, spherical potential

  $$V(r)=\left\{\begin{array}{cc} +V_{0} & r<a \\ 0 & r>a \end{array}\right.$$

  where $V_{0}=\hbar^{2} \gamma^{2} / 2 m$. By considering the s-wave solution to the Schrödinger equation, show that

  $$\frac{\tan \left(k a+\delta_{0}\right)}{k a}=\frac{\tanh \left(\sqrt{\gamma^{2}-k^{2}} a\right)}{\sqrt{\gamma^{2}-k^{2}} a}$$

  For low momenta, $k a \ll 1$, compute the s-wave contribution to the total cross-section. Comment on the physical interpretation of your result in the limit $\gamma a \rightarrow \infty$.

  comment

• Paper 4, Section II, A

  Applications of Quantum Mechanics | Part II, 2018

  Define a Bravais lattice $\Lambda$ in three dimensions. Define the reciprocal lattice $\Lambda^{\star}$. Define the Brillouin zone.

  An FCC lattice has a basis of primitive vectors given by

  $$\mathbf{a}_{1}=\frac{a}{2}\left(\mathbf{e}_{2}+\mathbf{e}_{3}\right), \quad \mathbf{a}_{2}=\frac{a}{2}\left(\mathbf{e}_{1}+\mathbf{e}_{3}\right), \quad \mathbf{a}_{3}=\frac{a}{2}\left(\mathbf{e}_{1}+\mathbf{e}_{2}\right),$$

  where $\mathbf{e}_{i}$ is an orthonormal basis of $\mathbb{R}^{3}$. Find a basis of reciprocal lattice vectors. What is the volume of the Brillouin zone?

  The asymptotic wavefunction for a particle, of wavevector $\mathbf{k}$, scattering off a potential $V(\mathbf{r})$ is

  $$\psi(\mathbf{r}) \sim e^{i \mathbf{k} \cdot \mathbf{r}}+f_{\mathrm{V}}\left(\mathbf{k} ; \mathbf{k}^{\prime}\right) \frac{e^{i k r}}{r}$$

  where $\mathbf{k}^{\prime}=k \hat{\mathbf{r}}$ and $f_{\mathrm{V}}\left(\mathbf{k} ; \mathbf{k}^{\prime}\right)$ is the scattering amplitude. Give a formula for the Born approximation to the scattering amplitude.

  Scattering of a particle off a single atom is modelled by a potential $V(\mathbf{r})=V_{0} \delta(r-d)$ with $\delta$-function support on a spherical shell, $r=|\mathbf{r}|=d$ centred at the origin. Calculate the Born approximation to the scattering amplitude, denoting the resulting expression as $\tilde{f}_{V}\left(\mathbf{k} ; \mathbf{k}^{\prime}\right)$.

  Scattering of a particle off a crystal consisting of atoms located at the vertices of a lattice $\Lambda$ is modelled by a potential

  $$V_{\Lambda}=\sum_{\mathbf{R} \in \Lambda} V(\mathbf{r}-\mathbf{R})$$

  where $V(\mathbf{r})=V_{0} \delta(r-d)$ as above. Calculate the Born approximation to the scattering amplitude giving your answer in terms of your approximate expression $\tilde{f}_{\mathrm{V}}$ for scattering off a single atom. Show that the resulting amplitude vanishes unless the momentum transfer $\mathbf{q}=\mathbf{k}-\mathbf{k}^{\prime}$ lies in the reciprocal lattice $\Lambda^{\star}$.

  For the particular FCC lattice considered above, show that, when $k=|\mathbf{k}|>2 \pi / a$, scattering occurs for two values of the scattering angle, $\theta_{1}$ and $\theta_{2}$, related by

  $$\frac{\sin \left(\frac{\theta_{1}}{2}\right)}{\sin \left(\frac{\theta_{2}}{2}\right)}=\frac{2}{\sqrt{3}}$$

  comment

2017

• Paper 1, Section II, C

  Applications of Quantum Mechanics | Part II, 2017

  A one-dimensional lattice has $N$ sites with lattice spacing $a$. In the tight-binding approximation, the Hamiltonian describing a single electron is given by

  $$H=E_{0} \sum_{n}|n\rangle\langle n|-J \sum_{n}(|n\rangle\langle n+1|+| n+1\rangle\langle n|)$$

  where $|n\rangle$ is the normalised state of the electron localised on the $n^{\text {th }}$lattice site. Using periodic boundary conditions $|N+1\rangle \equiv|1\rangle$, solve for the spectrum of this Hamiltonian to derive the dispersion relation

  $$E(k)=E_{0}-2 J \cos (k a)$$

  Define the Brillouin zone. Determine the number of states in the Brillouin zone.

  Calculate the velocity $v$ and effective mass $m^{\star}$ of the particle. For which values of $k$ is the effective mass negative?

  In the semi-classical approximation, derive an expression for the time-dependence of the position of the electron in a constant electric field.

  Describe how the concepts of metals and insulators arise in the model above.

  comment

• Paper 2, Section II, C

  Applications of Quantum Mechanics | Part II, 2017

  Give an account of the variational method for establishing an upper bound on the ground-state energy of a Hamiltonian $H$ with a discrete spectrum $H|n\rangle=E_{n}|n\rangle$, where $E_{n} \leqslant E_{n+1}, n=0,1,2 \ldots$

  A particle of mass $m$ moves in the three-dimensional potential

  $$V(r)=-\frac{A e^{-\mu r}}{r}$$

  where $A, \mu>0$ are constants and $r$ is the distance to the origin. Using the normalised variational wavefunction

  $$\psi(r)=\sqrt{\frac{\alpha^{3}}{\pi}} e^{-\alpha r}$$

  show that the expected energy is given by

  $$E(\alpha)=\frac{\hbar^{2} \alpha^{2}}{2 m}-\frac{4 A \alpha^{3}}{(\mu+2 \alpha)^{2}}$$

  Explain why there is necessarily a bound state when $\mu<A m / \hbar^{2}$. What can you say about the existence of a bound state when $\mu \geqslant A m / \hbar^{2}$ ?

  [Hint: The Laplacian in spherical polar coordinates is

  $$\left.\nabla^{2}=\frac{1}{r^{2}} \frac{\partial}{\partial r}\left(r^{2} \frac{\partial}{\partial r}\right)+\frac{1}{r^{2} \sin \theta} \frac{\partial}{\partial \theta}\left(\sin \theta \frac{\partial}{\partial \theta}\right)+\frac{1}{r^{2} \sin ^{2} \theta} \frac{\partial^{2}}{\partial \phi^{2}}\right]$$

  comment

• Paper 3, Section II, C

  Applications of Quantum Mechanics | Part II, 2017

  A particle of mass $m$ and charge $q$ moving in a uniform magnetic field $\mathbf{B}=\boldsymbol{\nabla} \times \mathbf{A}=$ $(0,0, B)$ is described by the Hamiltonian

  $$H=\frac{1}{2 m}(\mathbf{p}-q \mathbf{A})^{2}$$

  where $\mathbf{p}$ is the canonical momentum, which obeys $\left[x_{i}, p_{j}\right]=i \hbar \delta_{i j}$. The mechanical momentum is defined as $\boldsymbol{\pi}=\mathbf{p}-q \mathbf{A}$. Show that

  $$\left[\pi_{x}, \pi_{y}\right]=i q \hbar B$$

  Define

  $$a=\frac{1}{\sqrt{2 q \hbar B}}\left(\pi_{x}+i \pi_{y}\right) \quad \text { and } \quad a^{\dagger}=\frac{1}{\sqrt{2 q \hbar B}}\left(\pi_{x}-i \pi_{y}\right) \text {. }$$

  Derive the commutation relation obeyed by $a$ and $a^{\dagger}$. Write the Hamiltonian in terms of $a$ and $a^{\dagger}$ and hence solve for the spectrum.

  In symmetric gauge, states in the lowest Landau level with $k_{z}=0$ have wavefunctions

  $$\psi(x, y)=(x+i y)^{M} e^{-q B r^{2} / 4 \hbar}$$

  where $r^{2}=x^{2}+y^{2}$ and $M$ is a positive integer. By considering the profiles of these wavefunctions, estimate how many lowest Landau level states can fit in a disc of radius $R$.

  comment

• Paper 4, Section II, C

  Applications of Quantum Mechanics | Part II, 2017

  (a) In one dimension, a particle of mass $m$ is scattered by a potential $V(x)$ where $V(x) \rightarrow 0$ as $|x| \rightarrow \infty$. For wavenumber $k>0$, the incoming $(\mathcal{I})$ and outgoing $(\mathcal{O})$ asymptotic plane wave states with positive $(+)$ and negative $(-)$ parity are given by

  $$\begin{array}{rr} \mathcal{I}_{+}(x)=e^{-i k|x|}, & \mathcal{I}_{-}(x)=\operatorname{sign}(x) e^{-i k|x|} \\ \mathcal{O}_{+}(x)=e^{+i k|x|}, & \mathcal{O}_{-}(x)=-\operatorname{sign}(x) e^{+i k|x|} \end{array}$$

  (i) Explain how this basis may be used to define the $S$-matrix,

  $$\mathcal{S}^{P}=\left(\begin{array}{cc} S_{++} & S_{+-} \\ S_{-+} & S_{--} \end{array}\right)$$

  (ii) For what choice of potential would you expect $S_{+-}=S_{-+}=0$ ? Why?

  (b) The potential $V(x)$ is given by

  $$V(x)=V_{0}[\delta(x-a)+\delta(x+a)]$$

  with $V_{0}$ a constant.

  (i) Show that

  $$S_{--}(k)=e^{-2 i k a}\left[\frac{\left(2 k-i U_{0}\right) e^{i k a}+i U_{0} e^{-i k a}}{\left(2 k+i U_{0}\right) e^{-i k a}-i U_{0} e^{i k a}}\right]$$

  where $U_{0}=2 m V_{0} / \hbar^{2}$. Verify that $\left|S_{--}\right|^{2}=1$. Explain the physical meaning of this result.

  (ii) For $V_{0}<0$, by considering the poles or zeros of $S_{--}(k)$, show that there exists one bound state of negative parity if $a U_{0}<-1$.

  (iii) For $V_{0}>0$ and $a U_{0} \gg 1$, show that $S_{--}(k)$ has a pole at

  $$k a=\pi+\alpha-i \gamma$$

  where $\alpha$ and $\gamma$ are real and

  $$\alpha=-\frac{\pi}{a U_{0}}+O\left(\frac{1}{\left(a U_{0}\right)^{2}}\right) \quad \text { and } \quad \gamma=\left(\frac{\pi}{a U_{0}}\right)^{2}+O\left(\frac{1}{\left(a U_{0}\right)^{3}}\right)$$

  Explain the physical significance of this result.

  comment

2016

• Paper 1, Section II, A

  Applications of Quantum Mechanics | Part II, 2016

  A particle in one dimension of mass $m$ and energy $E=\hbar^{2} k^{2} / 2 m(k>0)$ is incident from $x=-\infty$ on a potential $V(x)$ with $V(x) \rightarrow 0$ as $x \rightarrow-\infty$ and $V(x)=\infty$ for $x>0$. The relevant solution of the time-independent Schrödinger equation has the asymptotic form

  $$\psi(x) \sim \exp (i k x)+r(k) \exp (-i k x), \quad x \rightarrow-\infty$$

  Explain briefly why a pole in the reflection amplitude $r(k)$ at $k=i \kappa$ with $\kappa>0$ corresponds to the existence of a stable bound state in this potential. Indicate why a pole in $r(k)$ just below the real $k$-axis, at $k=k_{0}-i \rho$ with $k_{0} \gg \rho>0$, corresponds to a quasi-stable bound state. Find an approximate expression for the lifetime $\tau$ of such a quasi-stable state.

  Now suppose that

  $$V(x)= \begin{cases}\left(\hbar^{2} U / 2 m\right) \delta(x+a) & \text { for } x<0 \\ \infty & \text { for } x>0\end{cases}$$

  where $U>0$ and $a>0$ are constants. Compute the reflection amplitude $r(k)$ in this case and deduce that there are quasi-stable bound states if $U$ is large. Give expressions for the wavefunctions and energies of these states and compute their lifetimes, working to leading non-vanishing order in $1 / U$ for each expression.

  [ You may assume $\psi=0$ for $x \geqslant 0$ and $\lim _{\epsilon \rightarrow 0+}\left\{\psi^{\prime}(-a+\epsilon)-\psi^{\prime}(-a-\epsilon)\right\}=U \psi(-a)$.]

  comment

• Paper 2, Section II, A

  Applications of Quantum Mechanics | Part II, 2016

  A particle of mass $m$ moves in three dimensions subject to a potential $V(\mathbf{r})$ localised near the origin. The wavefunction for a scattering process with incident particle of wavevector $\mathbf{k}$ is denoted $\psi(\mathbf{k}, \mathbf{r})$. With reference to the asymptotic form of $\psi$, define the scattering amplitude $f\left(\mathbf{k}, \mathbf{k}^{\prime}\right)$, where $\mathbf{k}^{\prime}$ is the wavevector of the outgoing particle with $\left|\mathbf{k}^{\prime}\right|=|\mathbf{k}|=k$.

  By recasting the Schrödinger equation for $\psi(\mathbf{k}, \mathbf{r})$ as an integral equation, show that

  $$f\left(\mathbf{k}, \mathbf{k}^{\prime}\right)=-\frac{m}{2 \pi \hbar^{2}} \int d^{3} \mathbf{r}^{\prime} \exp \left(-i \mathbf{k}^{\prime} \cdot \mathbf{r}^{\prime}\right) V\left(\mathbf{r}^{\prime}\right) \psi\left(\mathbf{k}, \mathbf{r}^{\prime}\right)$$

  [You may assume that

  $$\mathcal{G}(k ; \mathbf{r})=-\frac{1}{4 \pi|\mathbf{r}|} \exp (i k|\mathbf{r}|)$$

  is the Green's function for $\nabla^{2}+k^{2}$ which obeys the appropriate boundary conditions for a scattering solution.]

  Now suppose $V(\mathbf{r})=\lambda U(\mathbf{r})$, where $\lambda \ll 1$ is a dimensionless constant. Determine the first two non-zero terms in the expansion of $f\left(\mathbf{k}, \mathbf{k}^{\prime}\right)$ in powers of $\lambda$, giving each term explicitly as an integral over one or more position variables $\mathbf{r}, \mathbf{r}^{\prime}, \ldots$

  Evaluate the contribution to $f\left(\mathbf{k}, \mathbf{k}^{\prime}\right)$ of order $\lambda$ in the case $U(\mathbf{r})=\delta(|\mathbf{r}|-a)$, expressing the answer as a function of $a, k$ and the scattering angle $\theta$ (defined so that $\left.\mathbf{k} \cdot \mathbf{k}^{\prime}=k^{2} \cos \theta\right)$.

  comment

• Paper 3, Section II, A

  Applications of Quantum Mechanics | Part II, 2016

  (a) A spinless charged particle moves in an electromagnetic field defined by vector and scalar potentials $\mathbf{A}(\mathbf{x}, t)$ and $\phi(\mathbf{x}, t)$. The wavefunction $\psi(\mathbf{x}, t)$ for the particle satisfies the time-dependent Schrödinger equation with Hamiltonian

  $$\hat{H}_{0}=\frac{1}{2 m}(-i \hbar \boldsymbol{\nabla}+e \mathbf{A}) \cdot(-i \hbar \boldsymbol{\nabla}+e \mathbf{A})-e \phi .$$

  Consider a gauge transformation

  $$\mathbf{A} \rightarrow \tilde{\mathbf{A}}=\mathbf{A}+\nabla f, \quad \phi \rightarrow \tilde{\phi}=\phi-\frac{\partial f}{\partial t}, \quad \psi \rightarrow \tilde{\psi}=\exp \left(-\frac{i e f}{\hbar}\right) \psi$$

  for some function $f(\mathbf{x}, t)$. Define covariant derivatives with respect to space and time, and show that $\tilde{\psi}$ satisfies the Schrödinger equation with potentials $\tilde{\mathbf{A}}$ and $\tilde{\phi}$.

  (b) Suppose that in part (a) the magnetic field has the form $\mathbf{B}=\boldsymbol{\nabla} \times \mathbf{A}=(0,0, B)$, where $B$ is a constant, and that $\phi=0$. Find a suitable $\mathbf{A}$ with $A_{y}=A_{z}=0$ and determine the energy levels of the Hamiltonian $\hat{H}_{0}$ when the $z$-component of the momentum of the particle is zero. Suppose in addition that the particle is constrained to lie in a rectangular region of area $\mathcal{A}$ in the $(x, y)$-plane. By imposing periodic boundary conditions in the $x$-direction, estimate the degeneracy of each energy level. [You may use without proof results for a quantum harmonic oscillator, provided they are clearly stated.]

  (c) An electron is a charged particle of spin $\frac{1}{2}$ with a two-component wavefunction $\psi(\mathbf{x}, t)$ governed by the Hamiltonian

  $$\hat{H}=\hat{H}_{0} \mathbb{I}_{2}+\frac{e \hbar}{2 m} \mathbf{B} \cdot \boldsymbol{\sigma}$$

  where $\mathbb{I}_{2}$ is the $2 \times 2$ unit matrix and $\sigma=\left(\sigma_{1}, \sigma_{2}, \sigma_{3}\right)$ denotes the Pauli matrices. Find the energy levels for an electron in the constant magnetic field defined in part (b), assuming as before that the $z$-component of the momentum of the particle is zero.

  Consider $N$ such electrons confined to the rectangular region defined in part (b). Ignoring interactions between the electrons, show that the ground state energy of this system vanishes for $N$ less than some integer $N_{\max }$ which you should determine. Find the ground state energy for $N=(2 p+1) N_{\max }$, where $p$ is a positive integer.

  comment

• Paper 4, Section II, A

  Applications of Quantum Mechanics | Part II, 2016

  Let $\Lambda \subset \mathbb{R}^{2}$ be a Bravais lattice. Define the dual lattice $\Lambda^{*}$ and show that

  $$V(\mathbf{x})=\sum_{\mathbf{q} \in \Lambda^{*}} V_{\mathbf{q}} \exp (i \mathbf{q} \cdot \mathbf{x})$$

  obeys $V(\mathbf{x}+l)=V(\mathbf{x})$ for all $l \in \Lambda$, where $V_{\mathbf{q}}$ are constants. Suppose $V(\mathbf{x})$ is the potential for a particle of mass $m$ moving in a two-dimensional crystal that contains a very large number of lattice sites of $\Lambda$ and occupies an area $\mathcal{A}$. Adopting periodic boundary conditions, plane-wave states $|\mathbf{k}\rangle$ can be chosen such that

  $$\langle\mathbf{x} \mid \mathbf{k}\rangle=\frac{1}{\mathcal{A}^{1 / 2}} \exp (i \mathbf{k} \cdot \mathbf{x}) \quad \text { and } \quad\left\langle\mathbf{k} \mid \mathbf{k}^{\prime}\right\rangle=\delta_{\mathbf{k} \mathbf{k}^{\prime}}$$

  The allowed wavevectors $\mathbf{k}$ are closely spaced and include all vectors in $\Lambda^{*}$. Find an expression for the matrix element $\left\langle\mathbf{k}|V(\mathbf{x})| \mathbf{k}^{\prime}\right\rangle$ in terms of the coefficients $V_{\mathbf{q}}$. [You need not discuss additional details of the boundary conditions.]

  Now suppose that $V(\mathbf{x})=\lambda U(\mathbf{x})$, where $\lambda \ll 1$ is a dimensionless constant. Find the energy $E(\mathbf{k})$ for a particle with wavevector $\mathbf{k}$ to order $\lambda^{2}$ in non-degenerate perturbation theory. Show that this expansion in $\lambda$ breaks down on the Bragg lines in k-space defined by the condition

  $$\mathbf{k} \cdot \mathbf{q}=\frac{1}{2}|\mathbf{q}|^{2} \quad \text { for } \quad \mathbf{q} \in \Lambda^{*}$$

  and explain briefly, without additional calculations, the significance of this for energy levels in the crystal.

  Consider the particular case in which $\Lambda$ has primitive vectors

  $$\mathbf{a}_{1}=2 \pi\left(\mathbf{i}+\frac{1}{\sqrt{3}} \mathbf{j}\right), \quad \mathbf{a}_{2}=2 \pi \frac{2}{\sqrt{3}} \mathbf{j}$$

  where $\mathbf{i}$ and $\mathbf{j}$ are orthogonal unit vectors. Determine the polygonal region in $\mathbf{k}$-space corresponding to the lowest allowed energy band.

  comment

2015

• Paper 1, Section II, A

  Applications of Quantum Mechanics | Part II, 2015

  Define the Rayleigh-Ritz quotient $R[\psi]$ for a normalisable state $|\psi\rangle$ of a quantum system with Hamiltonian $H$. Given that the spectrum of $H$ is discrete and that there is a unique ground state of energy $E_{0}$, show that $R[\psi] \geqslant E_{0}$ and that equality holds if and only if $|\psi\rangle$ is the ground state.

  A simple harmonic oscillator (SHO) is a particle of mass $m$ moving in one dimension subject to the potential

  $$V(x)=\frac{1}{2} m \omega^{2} x^{2}$$

  Estimate the ground state energy $E_{0}$ of the SHO by using the ground state wavefunction for a particle in an infinite potential well of width $a$, centred on the origin (the potential is $U(x)=0$ for $|x|<a / 2$ and $U(x)=\infty$ for $|x|>a / 2)$. Take $a$ as the variational parameter.

  Perform a similar estimate for the energy $E_{1}$ of the first excited state of the SHO by using the first excited state of the infinite potential well as a trial wavefunction.

  Is the estimate for $E_{1}$ necessarily an upper bound? Justify your answer.

  $\left[\right.$ You may use : $\int_{-\pi / 2}^{\pi / 2} y^{2} \cos ^{2} y d y=\frac{\pi}{4}\left(\frac{\pi^{2}}{6}-1\right) \quad$ and $\left.\quad \int_{-\pi}^{\pi} y^{2} \sin ^{2} y d y=\pi\left(\frac{\pi^{2}}{3}-\frac{1}{2}\right) \cdot\right]$

  comment

• Paper 2, Section II, A

  Applications of Quantum Mechanics | Part II, 2015

  A beam of particles of mass $m$ and energy $\hbar^{2} k^{2} / 2 m$ is incident on a target at the origin described by a spherically symmetric potential $V(r)$. Assuming the potential decays rapidly as $r \rightarrow \infty$, write down the asymptotic form of the wavefunction, defining the scattering amplitude $f(\theta)$.

  Consider a free particle with energy $\hbar^{2} k^{2} / 2 m$. State, without proof, the general axisymmetric solution of the Schrödinger equation for $r>0$ in terms of spherical Bessel and Neumann functions $j_{\ell}$ and $n_{\ell}$, and Legendre polynomials $P_{\ell}(\ell=0,1,2, \ldots)$. Hence define the partial wave phase shifts $\delta_{\ell}$ for scattering from a potential $V(r)$ and derive the partial wave expansion for $f(\theta)$ in terms of phase shifts.

  Now suppose

  $$V(r)=\left\{\begin{array}{cc} \hbar^{2} \gamma^{2} / 2 m & r<a \\ 0 & r>a \end{array}\right.$$

  with $\gamma>k$. Show that the S-wave phase shift $\delta_{0}$ obeys

  $$\frac{\tanh (\kappa a)}{\kappa a}=\frac{\tan \left(k a+\delta_{0}\right)}{k a}$$

  where $\kappa^{2}=\gamma^{2}-k^{2}$. Deduce that for an S-wave solution

  $$f \rightarrow \frac{\tanh \gamma a-\gamma a}{\gamma} \quad \text { as } \quad k \rightarrow 0$$

  [You may assume : $\quad \exp (i k r \cos \theta)=\sum_{\ell=0}^{\infty}(2 \ell+1) i^{\ell} j_{\ell}(k r) P_{\ell}(\cos \theta)$

  and $j_{\ell}(\rho) \sim \frac{1}{\rho} \sin (\rho-\ell \pi / 2), \quad n_{\ell}(\rho) \sim-\frac{1}{\rho} \cos (\rho-\ell \pi / 2) \quad$ as $\left.\quad \rho \rightarrow \infty .\right]$

  comment

• Paper 3, Section II, A

  Applications of Quantum Mechanics | Part II, 2015

  A particle of mass $m$ and energy $E=-\hbar^{2} \kappa^{2} / 2 m<0$ moves in one dimension subject to a periodic potential

  $$V(x)=-\frac{\hbar^{2} \lambda}{m} \sum_{\ell=-\infty}^{\infty} \delta(x-\ell a) \quad \text { with } \quad \lambda>0 .$$

  Determine the corresponding Floquet matrix $\mathcal{M}$. [You may assume without proof that for the Schrödinger equation with potential $\alpha \delta(x)$ the wavefunction $\psi(x)$ is continuous at $x=0$ and satisfies $\left.\psi^{\prime}(0+)-\psi^{\prime}(0-)=\left(2 m \alpha / \hbar^{2}\right) \psi(0) .\right]$

  Explain briefly, with reference to Bloch's theorem, how restrictions on the energy of a Bloch state can be derived from $\mathcal{M}$. Deduce that for the potential $V(x)$ above, $\kappa$ is confined to a range whose boundary values are determined by

  $$\tanh \left(\frac{\kappa a}{2}\right)=\frac{\kappa}{\lambda} \quad \text { and } \quad \operatorname{coth}\left(\frac{\kappa a}{2}\right)=\frac{\kappa}{\lambda} .$$

  Sketch the left-hand and right-hand sides of each of these equations as functions of $y=\kappa a / 2$. Hence show that there is exactly one allowed band of negative energies with either (i) $E_{-} \leqslant E<0$ or (ii) $E_{-} \leqslant E \leqslant E_{+}<0$ and determine the values of $\lambda a$ for which each of these cases arise. [You should not attempt to evaluate the constants $E_{\pm} .$]

  Comment briefly on the limit $a \rightarrow \infty$ with $\lambda$ fixed.

  comment

• Paper 4, Section II,

  Applications of Quantum Mechanics | Part II, 2015

  Let $\Lambda$ be a Bravais lattice with basis vectors $\mathbf{a}_{1}, \mathbf{a}_{2}, \mathbf{a}_{3}$. Define the reciprocal lattice $\Lambda^{*}$ and write down basis vectors $\mathbf{b}_{1}, \mathbf{b}_{2}, \mathbf{b}_{3}$ for $\Lambda^{*}$ in terms of the basis for $\Lambda$.

  A finite crystal consists of identical atoms at sites of $\Lambda$ given by

  $$\ell=n_{1} \mathbf{a}_{1}+n_{2} \mathbf{a}_{2}+n_{3} \mathbf{a}_{3} \quad \text { with } \quad 0 \leqslant n_{i}<N_{i}$$

  A particle of mass $m$ scatters off the crystal; its wavevector is $\mathbf{k}$ before scattering and $\mathbf{k}^{\prime}$ after scattering, with $|\mathbf{k}|=\left|\mathbf{k}^{\prime}\right|$. Show that the scattering amplitude in the Born approximation has the form

  $$-\frac{m}{2 \pi \hbar^{2}} \Delta(\mathbf{q}) \tilde{U}(\mathbf{q}), \quad \mathbf{q}=\mathbf{k}^{\prime}-\mathbf{k}$$

  where $U(\mathbf{x})$ is the potential due to a single atom at the origin and $\Delta(\mathbf{q})$ depends on the crystal structure. [You may assume that in the Born approximation the amplitude for scattering off a potential $V(\mathbf{x})$ is $-\left(m / 2 \pi \hbar^{2}\right) \tilde{V}(\mathbf{q})$ where tilde denotes the Fourier transform.]

  Derive an expression for $|\Delta(\mathbf{q})|$ that is valid when $e^{-i \mathbf{q} \cdot \mathbf{a}_{i}} \neq 1$. Show also that when $\mathbf{q}$ is a reciprocal lattice vector $|\Delta(\mathbf{q})|$ is equal to the total number of atoms in the crystal. Comment briefly on the significance of these results.

  Now suppose that $\Lambda$ is a face-centred-cubic lattice:

  $$\mathbf{a}_{1}=\frac{a}{2}(\hat{\mathbf{y}}+\hat{\mathbf{z}}), \quad \mathbf{a}_{2}=\frac{a}{2}(\hat{\mathbf{z}}+\hat{\mathbf{x}}), \quad \mathbf{a}_{3}=\frac{a}{2}(\hat{\mathbf{x}}+\hat{\mathbf{y}})$$

  where $a$ is a constant. Show that for a particle incident with $|\mathbf{k}|>2 \pi / a$, enhanced scattering is possible for at least two values of the scattering angle, $\theta_{1}$ and $\theta_{2}$, related by

  $$\frac{\sin \left(\theta_{1} / 2\right)}{\sin \left(\theta_{2} / 2\right)}=\frac{\sqrt{3}}{2}$$

  comment

2014

• Paper 1, Section II, A

  Applications of Quantum Mechanics | Part II, 2014

  A particle of mass $m$ scatters on a localised potential well $V(x)$ in one dimension. With reference to the asymptotic behaviour of the wavefunction as $x \rightarrow \pm \infty$, define the reflection and transmission amplitudes, $r$ and $t$, for a right-moving incident particle of wave number $k$. Define also the corresponding amplitudes, $r^{\prime}$ and $t^{\prime}$, for a left-moving incident particle of wave number $k$. Derive expressions for $r^{\prime}$ and $t^{\prime}$ in terms of $r$ and $t$.

  (a) Define the $S$-matrix, giving its elements in terms of $r$ and $t$. Using the relation

  $$|r|^{2}+|t|^{2}=1$$

  (which you need not derive), show that the S-matrix is unitary. How does the S-matrix simplify if the potential well satisfies $V(-x)=V(x)$ ?

  (b) Consider the potential well

  $$V(x)=-\frac{3 \hbar^{2}}{m} \frac{1}{\cosh ^{2}(x)}$$

  The corresponding Schrödinger equation has an exact solution

  $$\psi_{k}(x)=\exp (i k x)\left[3 \tanh ^{2}(x)-3 i k \tanh (x)-\left(1+k^{2}\right)\right]$$

  with energy $E=\hbar^{2} k^{2} / 2 m$, for every real value of $k$. [You do not need to verify this.] Find the S-matrix for scattering on this potential. What special feature does the scattering have in this case?

  (c) Explain the connection between singularities of the S-matrix and bound states of the potential well. By analytic continuation of the solution $\psi_{k}(x)$ to appropriate complex values of $k$, find the wavefunctions and energies of the bound states of the well. [You do not need to normalise the wavefunctions.]

  comment

• Paper 2, Section II, A

  Applications of Quantum Mechanics | Part II, 2014

  (a) A classical particle of mass $m$ scatters on a central potential $V(r)$ with energy $E$, impact parameter $b$, and scattering angle $\theta$. Define the corresponding differential cross-section.

  For particle trajectories in the Coulomb potential,

  $$V_{C}(r)=\frac{e^{2}}{4 \pi \epsilon_{0} r}$$

  the impact parameter is given by

  $$b=\frac{e^{2}}{8 \pi \epsilon_{0} E} \cot \left(\frac{\theta}{2}\right)$$

  Find the differential cross-section as a function of $E$ and $\theta$.

  (b) A quantum particle of mass $m$ and energy $E=\hbar^{2} k^{2} / 2 m$ scatters in a localised potential $V(\mathbf{r})$. With reference to the asymptotic form of the wavefunction at large $|\mathbf{r}|$, define the scattering amplitude $f\left(\mathbf{k}, \mathbf{k}^{\prime}\right)$ as a function of the incident and outgoing wavevectors $\mathbf{k}$ and $\mathbf{k}^{\prime}$ (where $|\mathbf{k}|=\left|\mathbf{k}^{\prime}\right|=k$ ). Define the differential cross-section for this process and express it in terms of $f\left(\mathbf{k}, \mathbf{k}^{\prime}\right)$.

  Now consider a potential of the form $V(\mathbf{r})=\lambda U(\mathbf{r})$, where $\lambda \ll 1$ is a dimensionless coupling and $U$ does not depend on $\lambda$. You may assume that the Schrödinger equation for the wavefunction $\psi(\mathbf{k} ; \mathbf{r})$ of a scattering state with incident wavevector $\mathbf{k}$ may be written as the integral equation

  $$\psi(\mathbf{k} ; \mathbf{r})=\exp (i \mathbf{k} \cdot \mathbf{r})+\frac{2 m \lambda}{\hbar^{2}} \int d^{3} r^{\prime} \mathcal{G}_{0}^{(+)}\left(k ; \mathbf{r}-\mathbf{r}^{\prime}\right) U\left(\mathbf{r}^{\prime}\right) \psi\left(\mathbf{k} ; \mathbf{r}^{\prime}\right)$$

  where

  $$\mathcal{G}_{0}^{(+)}(k ; \mathbf{r})=-\frac{1}{4 \pi} \frac{\exp (i k|\mathbf{r}|)}{|\mathbf{r}|}$$

  Show that the corresponding scattering amplitude is given by

  $$f\left(\mathbf{k}, \mathbf{k}^{\prime}\right)=-\frac{m \lambda}{2 \pi \hbar^{2}} \int d^{3} r^{\prime} \exp \left(-i \mathbf{k}^{\prime} \cdot \mathbf{r}^{\prime}\right) U\left(\mathbf{r}^{\prime}\right) \psi\left(\mathbf{k} ; \mathbf{r}^{\prime}\right)$$

  By expanding the wavefunction in powers of $\lambda$ and keeping only the leading term, calculate the leading-order contribution to the differential cross-section, and evaluate it for the case of the Yukawa potential

  $$V(\mathbf{r})=\lambda \frac{\exp (-\mu r)}{r}$$

  By taking a suitable limit, obtain the differential cross-section for quantum scattering in the Coulomb potential $V_{C}(r)$ defined in Part (a) above, correct to leading order in an expansion in powers of the constant $\tilde{\alpha}=e^{2} / 4 \pi \epsilon_{0}$. Express your answer as a function of the particle energy $E$ and scattering angle $\theta$, and compare it to the corresponding classical cross-section calculated in Part (a).

  Part II, $2014 \quad$ List of Questions

  [TURN OVER

  comment

• Paper 3, Section II, A

  Applications of Quantum Mechanics | Part II, 2014

  In the nearly-free electron model a particle of mass $m$ moves in one dimension in a periodic potential of the form $V(x)=\lambda U(x)$, where $\lambda \ll 1$ is a dimensionless coupling and $U(x)$ has a Fourier series

  $$U(x)=\sum_{l=-\infty}^{+\infty} U_{l} \exp \left(\frac{2 \pi i}{a} l x\right)$$

  with coefficients obeying $U_{-l}=U_{l}^{*}$ for all $l$.

  Ignoring any degeneracies in the spectrum, the exact energy $E(k)$ of a Bloch state with wavenumber $k$ can be expanded in powers of $\lambda$ as

  $$E(k)=E_{0}(k)+\lambda\langle k|U| k\rangle+\lambda^{2} \sum_{k^{\prime} \neq k} \frac{\left\langle k|U| k^{\prime}\right\rangle\left\langle k^{\prime}|U| k\right\rangle}{E_{0}(k)-E_{0}\left(k^{\prime}\right)}+O\left(\lambda^{3}\right)$$

  where $|k\rangle$ is a normalised eigenstate of the free Hamiltonian $\hat{H}_{0}=\hat{p}^{2} / 2 m$ with momentum $p=\hbar k$ and energy $E_{0}(k)=\hbar^{2} k^{2} / 2 m$.

  Working on a finite interval of length $L=N a$, where $N$ is a positive integer, we impose periodic boundary conditions on the wavefunction:

  $$\psi(x+N a)=\psi(x)$$

  What are the allowed values of the wavenumbers $k$ and $k^{\prime}$ which appear in (1)? For these values evaluate the matrix element $\left\langle k|U| k^{\prime}\right\rangle$.

  For what values of $k$ and $k^{\prime}$ does (1) cease to be a good approximation? Explain your answer. Quoting any results you need from degenerate perturbation theory, calculate to $O(\lambda)$ the location and width of the gaps between allowed energy bands for the periodic potential $V(x)$, in terms of the Fourier coefficients $U_{l}$.

  Hence work out the allowed energy bands for the following potentials:

  $$\begin{aligned} &\text { (i) } \quad V(x)=2 \lambda \cos \left(\frac{2 \pi x}{a}\right), \\ &\text { (ii) } \quad V(x)=\lambda a \sum_{n=-\infty}^{+\infty} \delta(x-n a) . \end{aligned}$$

  comment

• Paper 4, Section II, A

  Applications of Quantum Mechanics | Part II, 2014

  Let $\Lambda$ be a Bravais lattice in three dimensions. Define the reciprocal lattice $\Lambda^{*}$.

  State and prove Bloch's theorem for a particle moving in a potential $V(\mathbf{x})$ obeying

  $$V(\mathbf{x}+\ell)=V(\mathbf{x}) \quad \forall \ell \in \Lambda, \mathbf{x} \in \mathbb{R}^{3}$$

  Explain what is meant by a Brillouin zone for this potential and how it is related to the reciprocal lattice.

  A simple cubic lattice $\Lambda_{1}$ is given by the set of points

  $$\Lambda_{1}=\left\{\ell \in \mathbb{R}^{3}: \ell=n_{1} \hat{\mathbf{i}}+n_{2} \hat{\mathbf{j}}+n_{3} \hat{\mathbf{k}}, n_{1}, n_{2}, n_{3} \in \mathbb{Z}\right\}$$

  where $\hat{\mathbf{i}}, \hat{\mathbf{j}}$ and $\hat{\mathbf{k}}$ are unit vectors parallel to the Cartesian coordinate axes in $\mathbb{R}^{3}$. A bodycentred cubic $\left(\mathrm{BCC}\right.$ ) lattice $\Lambda_{B C C}$ is obtained by adding to $\Lambda_{1}$ the points at the centre of each cube, i.e. all points of the form

  $$\ell+\frac{1}{2}(\hat{\mathbf{i}}+\hat{\mathbf{j}}+\hat{\mathbf{k}}), \quad \ell \in \Lambda_{1}$$

  Show that $\Lambda_{B C C}$ is Bravais with primitive vectors

  $$\begin{aligned} \mathbf{a}_{1} &=\frac{1}{2}(\hat{\mathbf{j}}+\hat{\mathbf{k}}-\hat{\mathbf{i}}) \\ \mathbf{a}_{2} &=\frac{1}{2}(\hat{\mathbf{k}}+\hat{\mathbf{i}}-\hat{\mathbf{j}}) \\ \mathbf{a}_{3} &=\frac{1}{2}(\hat{\mathbf{i}}+\hat{\mathbf{j}}-\hat{\mathbf{k}}) \end{aligned}$$

  Find the reciprocal lattice $\Lambda_{B C C}^{*}$. Hence find a consistent choice for the first Brillouin zone of a potential $V(\mathbf{x})$ obeying

  $$\begin{aligned} & V(\mathbf{x}+\ell)=V(\mathbf{x}) \quad \forall \ell \in \Lambda_{B C C}, \mathbf{x} \in \mathbb{R}^{3} \\ & \text { [Hint: The matrix } \left.M=\frac{1}{2}\left(\begin{array}{rrr}-1 & 1 & 1 \\1 & -1 & 1 \\1 & 1 & -1\end{array}\right) \text { has inverse } M^{-1}=\left(\begin{array}{lll}0 & 1 & 1 \\1 & 0 & 1 \\1 & 1 & 0\end{array}\right) .\right] \end{aligned}$$

  comment

2013

• Paper 1, Section II, D

  Applications of Quantum Mechanics | Part II, 2013

  Consider a quantum system with Hamiltonian $\widehat{H}$and energy levels

  $$E_{0}<E_{1}<E_{2}<\ldots$$

  For any state $|\psi\rangle$ define the Rayleigh-Ritz quotient $R[\psi]$ and show the following:

  (i) the ground state energy $E_{0}$ is the minimum value of $R[\psi]$;

  (ii) all energy eigenstates are stationary points of $R[\psi]$ with respect to variations of $|\psi\rangle$.

  Under what conditions can the value of $R\left[\psi_{\alpha}\right]$ for a trial wavefunction $\psi_{\alpha}$ (depending on some parameter $\alpha$ ) be used as an estimate of the energy $E_{1}$ of the first excited state? Explain your answer.

  For a suitably chosen trial wavefunction which is the product of a polynomial and a Gaussian, use the Rayleigh-Ritz quotient to estimate $E_{1}$ for a particle of mass $m$ moving in a potential $V(x)=g|x|$, where $g$ is a constant.

  [You may use the integral formulae,

  $$\begin{aligned} \int_{0}^{\infty} x^{2 n} \exp \left(-p x^{2}\right) d x &=\frac{(2 n-1) ! !}{2(2 p)^{n}} \sqrt{\frac{\pi}{p}} \\ \int_{0}^{\infty} x^{2 n+1} \exp \left(-p x^{2}\right) d x &=\frac{n !}{2 p^{n+1}} \end{aligned}$$

  where $n$ is a non-negative integer and $p$ is a constant. ]

  comment

• Paper 2, Section II, D

  Applications of Quantum Mechanics | Part II, 2013

  (i) A particle of momentum $\hbar k$ and energy $E=\hbar^{2} k^{2} / 2 m$ scatters off a sphericallysymmetric target in three dimensions. Define the corresponding scattering amplitude $f$ as a function of the scattering angle $\theta$. Expand the scattering amplitude in partial waves of definite angular momentum $l$, and determine the coefficients of this expansion in terms of the phase shifts $\delta_{l}(k)$ appearing in the following asymptotic form of the wavefunction, valid at large distance from the target,

  $$\psi(\mathbf{r}) \sim \sum_{l=0}^{\infty} \frac{2 l+1}{2 i k}\left[e^{2 i \delta_{l}} \frac{e^{i k r}}{r}-(-1)^{l} \frac{e^{-i k r}}{r}\right] P_{l}(\cos \theta) .$$

  Here, $r=|\mathbf{r}|$ is the distance from the target and $P_{l}$ are the Legendre polynomials.

  [You may use without derivation the following approximate relation between plane and spherical waves (valid asymptotically for large $r$ ):

  $$\exp (i k z) \sim \sum_{l=0}^{\infty}(2 l+1) i^{l} \frac{\sin \left(k r-\frac{1}{2} l \pi\right)}{k r} P_{l}(\cos \theta) .$$

  (ii) Suppose that the potential energy takes the form $V(r)=\lambda U(r)$ where $\lambda \ll 1$ is a dimensionless coupling. By expanding the wavefunction in a power series in $\lambda$, derive the Born Approximation to the scattering amplitude in the form

  $$f(\theta)=-\frac{2 m \lambda}{\hbar^{2}} \int_{0}^{\infty} U(r) \frac{\sin q r}{q} r d r$$

  up to corrections of order $\lambda^{2}$, where $q=2 k \sin (\theta / 2)$. [You may quote any results you need for the Green's function for the differential operator $\nabla^{2}+k^{2}$ provided they are stated clearly.]

  (iii) Derive the corresponding order $\lambda$ contribution to the phase shift $\delta_{l}(k)$ of angular momentum $l$.

  [You may use the orthogonality relations

  $$\int_{-1}^{+1} P_{l}(w) P_{m}(w) d w=\frac{2}{(2 l+1)} \delta_{l m}$$

  and the integral formula

  $$\int_{0}^{1} P_{l}\left(1-2 x^{2}\right) \sin (a x) d x=\frac{a}{2}\left[j_{l}\left(\frac{a}{2}\right)\right]^{2}$$

  where $j_{l}(z)$ is a spherical Bessel function.]

  comment

• Paper 3, Section II, D

  Applications of Quantum Mechanics | Part II, 2013

  Write down the classical Hamiltonian for a particle of mass $m$, electric charge $-e$ and momentum p moving in the background of an electromagnetic field with vector and scalar potentials $\mathbf{A}(\mathbf{x}, t)$ and $\phi(\mathbf{x}, t)$.

  Consider the case of a constant uniform magnetic field, $\mathbf{B}=(0,0, B)$ and $\mathbf{E}=0$. Working in the gauge with $\mathbf{A}=(-B y, 0,0)$ and $\phi=0$, show that Hamilton's equations,

  $$\dot{\mathbf{x}}=\frac{\partial H}{\partial \mathbf{p}}, \quad \dot{\mathbf{p}}=-\frac{\partial H}{\partial \mathbf{x}},$$

  admit solutions corresponding to circular motion in the $x-y$ plane with angular frequency $\omega_{B}=e B / m$.

  Show that, in the same gauge, the coordinates $\left(x_{0}, y_{0}, 0\right)$ of the centre of the circle are related to the instantaneous position $\mathbf{x}=(x, y, z)$ and momentum $\mathbf{p}=\left(p_{x}, p_{y}, p_{z}\right)$ of the particle by

  $$x_{0}=x-\frac{p_{y}}{e B}, \quad y_{0}=\frac{p_{x}}{e B} .$$

  Write down the quantum Hamiltonian $\hat{H}$ for the system. In the case of a uniform constant magnetic field discussed above, find the allowed energy levels. Working in the gauge specified above, write down quantum operators corresponding to the classical quantities $x_{0}$ and $y_{0}$ defined in (1) above and show that they are conserved.

  [In this question you may use without derivation any facts relating to the energy spectrum of the quantum harmonic oscillator provided they are stated clearly.]

  comment

• Paper 4, Section II, D

  Applications of Quantum Mechanics | Part II, 2013

  Define the Floquet matrix for a particle moving in a periodic potential in one dimension and explain how it determines the allowed energy bands of the system.

  A potential barrier in one dimension has the form

  $$V(x)= \begin{cases}V_{0}(x), & |x|<a / 4 \\ 0, & |x|>a / 4\end{cases}$$

  where $V_{0}(x)$ is a smooth, positive function of $x$. The reflection and transmission amplitudes for a particle of wavenumber $k>0$, incident from the left, are $r(k)$ and $t(k)$ respectively. For a particle of wavenumber $-k$, incident from the right, the corresponding amplitudes are $r^{\prime}(k)$ and $t^{\prime}(k)=t(k)$. In the following, for brevity, we will suppress the $k$-dependence of these quantities.

  Consider the periodic potential $\tilde{V}$, defined by $\tilde{V}(x)=V(x)$ for $|x|<a / 2$ and by $\tilde{V}(x+a)=\widetilde{V}(x)$ elsewhere. Write down two linearly independent solutions of the corresponding Schrödinger equation in the region $-3 a / 4<x<-a / 4$. Using the scattering data given above, extend these solutions to the region $a / 4<x<3 a / 4$. Hence find the Floquet matrix of the system in terms of the amplitudes $r, r^{\prime}$ and $t$ defined above.

  Show that the edges of the allowed energy bands for this potential lie at $E=\hbar^{2} k^{2} / 2 m$, where

  $$k a=i \log \left(t \pm \sqrt{r r^{\prime}}\right)$$

  comment

2012

• Paper 1, Section II, E

  Applications of Quantum Mechanics | Part II, 2012

  Give an account of the variational principle for establishing an upper bound on the ground-state energy $E_{0}$ of a particle moving in a potential $V(x)$ in one dimension.

  A particle of unit mass moves in the potential

  $$V(x)= \begin{cases}\infty & x \leqslant 0 \\ \lambda x & x>0\end{cases}$$

  with $\lambda$ a positive constant. Explain why it is important that any trial wavefunction used to derive an upper bound on $E_{0}$ should be chosen to vanish for $x \leqslant 0$.

  Use the trial wavefunction

  $$\psi(x)= \begin{cases}0 & x \leqslant 0 \\ x e^{-a x} & x>0\end{cases}$$

  where $a$ is a positive real parameter, to establish an upper bound $E_{0} \leqslant E(a, \lambda)$ for the energy of the ground state, and hence derive the lowest upper bound on $E_{0}$ as a function of $\lambda$.

  Explain why the variational method cannot be used in this case to derive an upper bound for the energy of the first excited state.

  comment

• Paper 2, Section II, E

  Applications of Quantum Mechanics | Part II, 2012

  A solution of the $S$-wave Schrödinger equation at large distances for a particle of mass $m$ with momentum $\hbar k$ and energy $E=\hbar^{2} k^{2} / 2 m$, has the form

  $$\psi_{0}(\boldsymbol{r}) \sim \frac{A}{r}[\sin k r+g(k) \cos k r] .$$

  Define the phase shift $\delta_{0}$ and verify that $\tan \delta_{0}(k)=g(k)$.

  Write down a formula for the cross-section $\sigma$, for a particle of momentum $\hbar k$ scattering on a radially symmetric potential of finite range, as a function of the phase shifts $\delta_{l}$ for the partial waves with quantum number $l$.

  (i) Suppose that $g(k)=-k / K$ for $K>0$. Show that there is a bound state of energy $E_{B}=-\hbar^{2} K^{2} / 2 m$. Neglecting the contribution from partial waves with $l>0$ show that the cross section is

  $$\sigma=\frac{4 \pi}{K^{2}+k^{2}} .$$

  (ii) Suppose now that $g(k)=\gamma /\left(K_{0}-k\right)$ with $K_{0}>0, \gamma>0$ and $\gamma \ll K_{0}$. Neglecting the contribution from partial waves with $l>0$, derive an expression for the cross section $\sigma$, and show that it has a local maximum when $E \approx \hbar^{2} K_{0}^{2} / 2 \mathrm{~m}$. Discuss the interpretation of this phenomenon in terms of resonant behaviour and derive an expression for the decay width of the resonant state.

  comment

• Paper 3, Section II, E

  Applications of Quantum Mechanics | Part II, 2012

  A simple model of a crystal consists of a 1D linear array of sites at positions $x=n a$, for all integer $n$ and separation $a$, each occupied by a similar atom. The potential due to the atom at the origin is $U(x)$, which is symmetric: $U(-x)=U(x)$. The Hamiltonian, $H_{0}$, for the atom at the $n$-th site in isolation has electron eigenfunction $\psi_{n}(x)$ with energy $E_{0}$. Write down $H_{0}$ and state the relationship between $\psi_{n}(x)$ and $\psi_{0}(x)$.

  The Hamiltonian $H$ for an electron moving in the crystal is $H=H_{0}+V(x)$. Give an expression for $V(x)$.

  In the tight-binding approximation for this model the $\psi_{n}$ are assumed to be orthonormal, $\left(\psi_{n}, \psi_{m}\right)=\delta_{n m}$, and the only non-zero matrix elements of $H_{0}$ and $V$ are

  $$\left(\psi_{n}, H_{0} \psi_{n}\right)=E_{0}, \quad\left(\psi_{n}, V \psi_{n}\right)=\alpha, \quad\left(\psi_{n}, V \psi_{n \pm 1}\right)=-A$$

  where $A>0$. By considering the trial wavefunction $\Psi(x, t)=\sum_{n} c_{n}(t) \psi_{n}(x)$, show that the time-dependent Schrödinger equation governing the amplitudes $c_{n}(t)$ is

  $$i \hbar \dot{c}_{n}=\left(E_{0}+\alpha\right) c_{n}-A\left(c_{n+1}+c_{n-1}\right)$$

  By examining a solution of the form

  $$c_{n}=e^{i(k n a-E t / \hbar)}$$

  show that $E$, the energy of the electron in the crystal, lies in a band given by

  $$E=E_{0}+\alpha-2 A \cos k a$$

  Using the fact that $\psi_{0}(x)$ is a parity eigenstate show that

  $$\left(\psi_{n}, x \psi_{n}\right)=n a .$$

  The electron in this model is now subject to an electric field $\mathcal{E}$ in the direction of increasing $x$, so that $V(x)$ is replaced by $V(x)-e \mathcal{E} x$, where $-e$ is the charge on the electron. Assuming that $\left(\psi_{n}, x \psi_{m}\right)=0, n \neq m$, write down the new form of the time-dependent Schrödinger equation for the probability amplitudes $c_{n}$. Verify that it has solutions of the form

  $$c_{n}=\exp \left[-\frac{i}{\hbar} \int_{0}^{t} \epsilon\left(t^{\prime}\right) d t^{\prime}+i\left(k+\frac{e \mathcal{E} t}{\hbar}\right) n a\right]$$

  where

  $$\epsilon(t)=E_{0}+\alpha-2 A \cos \left[\left(k+\frac{e \mathcal{E} t}{\hbar}\right) a\right]$$

  Use this result to show that the dynamical behaviour of an electron near the bottom of an energy band is the same as that for a free particle in the presence of an electric field with an effective mass $m^{*}=\hbar^{2} /\left(2 A a^{2}\right)$.

  comment

• Paper 4, Section II, $33 E$

  Applications of Quantum Mechanics | Part II, 2012

  Consider a one-dimensional crystal lattice of lattice spacing $a$ with the $n$-th atom having position $r_{n}=n a+x_{n}$ and momentum $p_{n}$, for $n=0,1, \ldots, N-1$. The atoms interact with their nearest neighbours with a harmonic force and the classical Hamiltonian is

  $$H=\sum_{n} \frac{p_{n}^{2}}{2 m}+\frac{1}{2} \lambda\left(x_{n}-x_{n-1}\right)^{2}$$

  where we impose periodic boundary conditions: $x_{N}=x_{0}$. Show that the normal mode frequencies for the classical harmonic vibrations of the system are given by

  $$\omega_{l}=2 \sqrt{\frac{\lambda}{m}}\left|\sin \left(\frac{k_{l} a}{2}\right)\right|,$$

  where $k_{l}=2 \pi l / N a$, with $l$ integer and (for $N$ even, which you may assume) $-N / 2<l \leqslant$ $N / 2$. What is the velocity of sound in this crystal?

  Show how the system may be quantized to give the quantum operator

  $$x_{n}(t)=X_{0}(t)+\sum_{l \neq 0} \sqrt{\frac{\hbar}{2 N m \omega_{l}}}\left[a_{l} e^{-i\left(\omega_{l} t-k_{l} n a\right)}+a_{l}^{\dagger} e^{i\left(\omega_{l} t-k_{l} n a\right)}\right],$$

  where $a_{l}^{\dagger}$ and $a_{l}$ are creation and annihilation operators, respectively, whose commutation relations should be stated. Briefly describe the spectrum of energy eigenstates for this system, stating the definition of the ground state $|0\rangle$ and giving the expression for the energy eigenvalue of any eigenstate.

  The Debye-Waller factor $e^{-W(Q)}$ associated with Bragg scattering from this crystal is defined by the matrix element

  $$e^{-W(Q)}=\left\langle 0\left|e^{i Q x_{0}(0)}\right| 0\right\rangle$$

  In the case where $\left\langle 0\left|X_{0}\right| 0\right\rangle=0$, calculate $W(Q)$.

  comment

2011

• Paper 1, Section II, E

  Applications of Quantum Mechanics | Part II, 2011

  In one dimension a particle of mass $m$ and momentum $\hbar k, k>0$, is scattered by a potential $V(x)$ where $V(x) \rightarrow 0$ as $|x| \rightarrow \infty$. Incoming and outgoing plane waves of positive $(+)$ and negative $(-)$ parity are given, respectively, by

  $$\begin{array}{ll} I_{+}(k, x)=e^{-i k|x|}, & I_{-}(k, x)=\operatorname{sgn}(x) e^{-i k|x|} \\ O_{+}(k, x)=e^{i k|x|}, & O_{-}(k, x)=-\operatorname{sgn}(x) e^{i k|x|} \end{array}$$

  The scattering solutions to the time-independent Schrödinger equation with positive and negative parity incoming waves are $\psi_{+}(x)$ and $\psi_{-}(x)$, respectively. State how the asymptotic behaviour of $\psi_{+}$and $\psi_{-}$can be expressed in terms of $I_{+}, I_{-}, O_{+}, O_{-}$and the S-matrix denoted by

  $$\boldsymbol{S}=\left(\begin{array}{cc} S_{++} & S_{+-} \\ S_{-+} & S_{--} \end{array}\right)$$

  In the case where $V(x)=V(-x)$ explain briefly why you expect $S_{+-}=S_{-+}=0$.

  The potential $V(x)$ is given by

  $$V(x)=V_{0}[\delta(x-a)+\delta(x+a)]$$

  where $V_{0}$ is a constant. In this case, show that

  $$S_{--}(k)=e^{-2 i k a}\left[\frac{\left(2 k-i U_{0}\right) e^{i k a}+i U_{0} e^{-i k a}}{\left(2 k+i U_{0}\right) e^{-i k a}-i U_{0} e^{i k a}}\right]$$

  where $U_{0}=2 m V_{0} / \hbar^{2}$. Verify that $\left|S_{--}\right|^{2}=1$ and explain briefly the physical meaning of this result.

  For $V_{0}<0$, by considering the poles or zeros of $S_{--}(k)$ show that there exists one bound state of negative parity in this potential if $U_{0} a<-1$.

  For $V_{0}>0$ and $U_{0} a \gg 1$, show that $S_{--}(k)$ has a pole at

  $$k a=\pi+\alpha-i \gamma$$

  where, to leading order in $1 /\left(U_{0} a\right)$,

  $$\alpha=-\frac{\pi}{U_{0} a}, \quad \gamma=\left(\frac{\pi}{U_{0} a}\right)^{2}$$

  Explain briefy the physical meaning of this result, and why you expect that $\gamma>0$.

  comment

• Paper 2, Section II, E

  Applications of Quantum Mechanics | Part II, 2011

  A beam of particles of mass $m$ and momentum $p=\hbar k$, incident along the $z$-axis, is scattered by a spherically symmetric potential $V(r)$, where $V(r)=0$ for large $r$. State the boundary conditions on the wavefunction as $r \rightarrow \infty$ and hence define the scattering amplitude $f(\theta)$, where $\theta$ is the scattering angle.

  Given that, for large $r$,

  $$e^{i k r \cos \theta}=\frac{1}{2 i k r} \sum_{l=0}^{\infty}(2 l+1)\left(e^{i k r}-(-1)^{l} e^{-i k r}\right) P_{l}(\cos \theta)$$

  explain how the partial-wave expansion can be used to define the phase shifts $\delta_{l}(k)(l=$ $0,1,2, \ldots)$. Furthermore, given that $d \sigma / d \Omega=|f(\theta)|^{2}$, derive expressions for $f(\theta)$ and the total cross-section $\sigma$ in terms of the $\delta_{l}$.

  In a particular case $V(r)$ is given by

  $$V(r)=\left\{\begin{array}{cl} \infty, & r<a \\ -V_{0}, & a<r<2 a \\ 0, & r>2 a \end{array}\right.$$

  where $V_{0}>0$. Show that the $\mathrm{S}$-wave phase shift $\delta_{0}$ satisfies

  $$\tan \left(\delta_{0}\right)=\frac{k \cos (2 k a)-\kappa \cot (\kappa a) \sin (2 k a)}{k \sin (2 k a)+\kappa \cot (\kappa a) \cos (2 k a)},$$

  where $\kappa^{2}=2 m V_{0} / \hbar^{2}+k^{2}$.

  Derive an expression for the scattering length $a_{s}$ in terms of $\kappa$. Find the values of $\kappa$ for which $\left|a_{s}\right|$ diverges and briefly explain their physical significance.

  comment

• Paper 3, Section II, E

  Applications of Quantum Mechanics | Part II, 2011

  An electron of mass $m$ moves in a $D$-dimensional periodic potential that satisfies the periodicity condition

  $$V(\boldsymbol{r}+\boldsymbol{l})=V(\boldsymbol{r}) \quad \forall l \in \Lambda,$$

  where $\Lambda$ is a D-dimensional Bravais lattice. State Bloch's theorem for the energy eigenfunctions of the electron.

  For a one-dimensional potential $V(x)$ such that $V(x+a)=V(x)$, give a full account of how the "nearly free electron model" leads to a band structure for the energy levels.

  Explain briefly the idea of a Fermi surface and its rôle in explaining the existence of conductors and insulators.

  comment

• Paper 4, Section II, E

  Applications of Quantum Mechanics | Part II, 2011

  A particle of charge $-e$ and mass $m$ moves in a magnetic field $\boldsymbol{B}(\boldsymbol{x}, t)$ and in an electric potential $\phi(\boldsymbol{x}, t)$. The time-dependent Schrödinger equation for the particle's wavefunction $\Psi(\boldsymbol{x}, t)$ is

  $$i \hbar\left(\frac{\partial}{\partial t}-\frac{i e}{\hbar} \phi\right) \Psi=-\frac{\hbar^{2}}{2 m}\left(\nabla+\frac{i e}{\hbar} \boldsymbol{A}\right)^{2} \Psi$$

  where $\boldsymbol{A}$ is the vector potential with $\boldsymbol{B}=\boldsymbol{\nabla} \wedge \boldsymbol{A}$. Show that this equation is invariant under the gauge transformations

  $$\begin{array}{ll} \boldsymbol{A}(\boldsymbol{x}, t) & \rightarrow \boldsymbol{A}(\boldsymbol{x}, t)+\boldsymbol{\nabla} f(\boldsymbol{x}, t) \\ \phi(\boldsymbol{x}, t) & \rightarrow \quad \phi(\boldsymbol{x}, t)-\frac{\partial}{\partial t} f(\boldsymbol{x}, t) \end{array}$$

  where $f$ is an arbitrary function, together with a suitable transformation for $\Psi$ which should be stated.

  Assume now that $\partial \Psi / \partial z=0$, so that the particle motion is only in the $x$ and $y$ directions. Let $\boldsymbol{B}$ be the constant field $\boldsymbol{B}=(0,0, B)$ and let $\phi=0$. In the gauge where $\boldsymbol{A}=(-B y, 0,0)$ show that the stationary states are given by

  $$\Psi_{k}(\boldsymbol{x}, t)=\psi_{k}(\boldsymbol{x}) e^{-i E t / \hbar}$$

  with

  $$\psi_{k}(\boldsymbol{x})=e^{i k x} \chi_{k}(y)$$

  Show that $\chi_{k}(y)$ is the wavefunction for a simple one-dimensional harmonic oscillator centred at position $y_{0}=\hbar k / e B$. Deduce that the stationary states lie in infinitely degenerate levels (Landau levels) labelled by the integer $n \geqslant 0$, with energy

  $$E_{n}=(2 n+1) \frac{\hbar e B}{2 m}$$

  A uniform electric field $\mathcal{E}$ is applied in the $y$-direction so that $\phi=-\mathcal{E} y$. Show that the stationary states are given by $(*)$, where $\chi_{k}(y)$ is a harmonic oscillator wavefunction centred now at

  $$y_{0}=\frac{1}{e B}\left(\hbar k-m \frac{\mathcal{E}}{B}\right)$$

  Show also that the eigen-energies are given by

  $$E_{n, k}=(2 n+1) \frac{\hbar e B}{2 m}+e \mathcal{E} y_{0}+\frac{m \mathcal{E}^{2}}{2 B^{2}} .$$

  Why does this mean that the Landau energy levels are no longer degenerate in two dimensions?

  comment

2010

• Paper 1, Section II, B

  Applications of Quantum Mechanics | Part II, 2010

  Give an account of the variational principle for establishing an upper bound on the ground-state energy, $E_{0}$, of a particle moving in a potential $V(x)$ in one dimension.

  Explain how an upper bound on the energy of the first excited state can be found in the case that $V(x)$ is a symmetric function.

  A particle of mass $2 m=\hbar^{2}$ moves in the potential

  $$V(x)=-V_{0} e^{-x^{2}}, \quad V_{0}>0$$

  Use the trial wavefunction

  $$\psi(x)=e^{-\frac{1}{2} a x^{2}}$$

  where $a$ is a positive real parameter, to establish the upper bound $E_{0} \leqslant E(a)$ for the energy of the ground state, where

  $$E(a)=\frac{1}{2} a-V_{0} \frac{\sqrt{a}}{\sqrt{1+a}} .$$

  Show that, for $a>0, E(a)$ has one zero and find its position.

  Show that the turning points of $E(a)$ are given by

  $$(1+a)^{3}=\frac{V_{0}^{2}}{a}$$

  and deduce that there is one turning point in $a>0$ for all $V_{0}>0$.

  Sketch $E(a)$ for $a>0$ and hence deduce that $V(x)$ has at least one bound state for all $V_{0}>0$.

  For $0<V_{0} \ll 1$ show that

  $$-V_{0}<E_{0} \leqslant \epsilon\left(V_{0}\right),$$

  where $\epsilon\left(V_{0}\right)=-\frac{1}{2} V_{0}^{2}+\mathrm{O}\left(V_{0}^{4}\right)$.

  [You may use the result that $\int_{-\infty}^{\infty} e^{-b x^{2}} d x=\sqrt{\frac{\pi}{b}}$ for $b>0 .$ ]

  comment

• Paper 2, Section II, B

  Applications of Quantum Mechanics | Part II, 2010

  A beam of particles of mass $m$ and momentum $p=\hbar k$ is incident along the $z$-axis. Write down the asymptotic form of the wave function which describes scattering under the influence of a spherically symmetric potential $V(r)$ and which defines the scattering amplitude $f(\theta)$.

  Given that, for large $r$,

  $$e^{i k r \cos \theta} \sim \frac{1}{2 i k r} \sum_{l=0}^{\infty}(2 l+1)\left(e^{i k r}-(-1)^{l} e^{-i k r}\right) P_{l}(\cos \theta),$$

  show how to derive the partial-wave expansion of the scattering amplitude in the form

  $$f(\theta)=\frac{1}{k} \sum_{l=0}^{\infty}(2 l+1) e^{i \delta_{l}} \sin \delta_{l} P_{l}(\cos \theta)$$

  Obtain an expression for the total cross-section, $\sigma$.

  Let $V(r)$ have the form

  $$V(r)=\left\{\begin{array}{cl} -V_{0}, & r<a \\ 0, & r>a \end{array}\right.$$

  where $V_{0}=\frac{\hbar^{2}}{2 m} \gamma^{2}$

  Show that the $l=0$ phase-shift $\delta_{0}$ satisfies

  $$\frac{\tan \left(k a+\delta_{0}\right)}{k a}=\frac{\tan \kappa a}{\kappa a},$$

  where $\kappa^{2}=k^{2}+\gamma^{2}$.

  Assume $\gamma$ to be large compared with $k$ so that $\kappa$ may be approximated by $\gamma$. Show, using graphical methods or otherwise, that there are values for $k$ for which $\delta_{0}(k)=n \pi$ for some integer $n$, which should not be calculated. Show that the smallest value, $k_{0}$, of $k$ for which this condition holds certainly satisfies $k_{0}<3 \pi / 2 a$.

  comment

• Paper 3, Section II, B

  Applications of Quantum Mechanics | Part II, 2010

  State Bloch's theorem for a one dimensional lattice which is invariant under translations by $a$.

  A simple model of a crystal consists of a one-dimensional linear array of identical sites with separation $a$. At the $n$th site the Hamiltonian, neglecting all other sites, is $H_{n}$ and an electron may occupy either of two states, $\phi_{n}(x)$ and $\chi_{n}(x)$, where

  $$H_{n} \phi_{n}(x)=E_{0} \phi_{n}(x), \quad H_{n} \chi_{n}(x)=E_{1} \chi_{n}(x),$$

  and $\phi_{n}$ and $\chi_{n}$ are orthonormal. How are $\phi_{n}(x)$ and $\chi_{n}(x)$ related to $\phi_{0}(x)$ and $\chi_{0}(x)$ ?

  The full Hamiltonian is $H$ and is invariant under translations by $a$. Write trial wavefunctions $\psi(x)$ for the eigenstates of this model appropriate to a tight binding approximation if the electron has probability amplitudes $b_{n}$ and $c_{n}$ to be in the states $\phi_{n}$ and $\chi_{n}$ respectively.

  Assume that the only non-zero matrix elements in this model are, for all $n$,

  $$\begin{aligned} &\left(\phi_{n}, H_{n} \phi_{n}\right)=E_{0}, \quad\left(\chi_{n}, H_{n} \chi_{n}\right)=E_{1} \\ &\left(\phi_{n}, V \phi_{n \pm 1}\right)=\left(\chi_{n}, V \chi_{n \pm 1}\right)=\left(\phi_{n}, V \chi_{n \pm 1}\right)=\left(\chi_{n}, V \phi_{n \pm 1}\right)=-A, \end{aligned}$$

  where $H=H_{n}+V$ and $A>0$. Show that the time-dependent Schrödinger equation governing the amplitudes becomes

  $$\begin{aligned} i \hbar \dot{b}_{n} &=E_{0} b_{n}-A\left(b_{n+1}+b_{n-1}+c_{n+1}+c_{n-1}\right) \\ i \hbar \dot{c}_{n} &=E_{1} c_{n}-A\left(c_{n+1}+c_{n-1}+b_{n+1}+b_{n-1}\right) \end{aligned}$$

  By examining solutions of the form

  $$\left(\begin{array}{l} b_{n} \\ c_{n} \end{array}\right)=\left(\begin{array}{l} B \\ C \end{array}\right) e^{i(k n a-E t / \hbar)}$$

  show that the allowed energies of the electron are two bands given by

  $$E=\frac{1}{2}\left(E_{0}+E_{1}-4 A \cos k a\right) \pm \frac{1}{2} \sqrt{\left(E_{0}-E_{1}\right)^{2}+16 A^{2} \cos ^{2} k a}$$

  Define the Brillouin zone for this system and find the energies at the top and bottom of both bands. Hence, show that the energy gap between the bands is

  $$\Delta E=-4 A+\sqrt{\left(E_{1}-E_{0}\right)^{2}+16 A^{2}}$$

  Show that the wavefunctions $\psi(x)$ satisfy Bloch's theorem.

  Describe briefly what are the crucial differences between insulators, conductors and semiconductors.

  comment

• Paper 4, Section II, B

  Applications of Quantum Mechanics | Part II, 2010

  The scattering amplitude for electrons of momentum $\hbar \mathbf{k}$ incident on an atom located at the origin is $f(\hat{\mathbf{r}})$ where $\hat{\mathbf{r}}=\mathbf{r} / r$. Explain why, if the atom is displaced by a position vector a, the asymptotic form of the scattering wave function becomes

  $$\psi_{\mathbf{k}}(\mathbf{r}) \sim e^{i \mathbf{k} \cdot \mathbf{r}}+e^{i \mathbf{k} \cdot \mathbf{a}} \frac{e^{i k r^{\prime}}}{r^{\prime}} f\left(\hat{\mathbf{r}}^{\prime}\right) \sim e^{i \mathbf{k} \cdot \mathbf{r}}+e^{i\left(\mathbf{k}-\mathbf{k}^{\prime}\right) \cdot \mathbf{a}} \frac{e^{i k r}}{r} f(\hat{\mathbf{r}}),$$

  where $\mathbf{r}^{\prime}=\mathbf{r}-\mathbf{a}, r^{\prime}=\left|\mathbf{r}^{\prime}\right|, \hat{\mathbf{r}}^{\prime}=\mathbf{r}^{\prime} / r^{\prime}$ and $k=|\mathbf{k}|, \mathbf{k}^{\prime}=k \hat{\mathbf{r}}$. For electrons incident on $N$ atoms in a regular Bravais crystal lattice show that the differential cross-section for scattering in the direction $\hat{\mathbf{r}}$ is

  $$\frac{d \sigma}{d \Omega}=N|f(\hat{\mathbf{r}})|^{2} \Delta\left(\mathbf{k}-\mathbf{k}^{\prime}\right) .$$

  Derive an explicit form for $\Delta(\mathbf{Q})$ and show that it is strongly peaked when $\mathbf{Q} \approx \mathbf{b}$ for $\mathbf{b}$ a reciprocal lattice vector.

  State the Born approximation for $f(\hat{\mathbf{r}})$ when the scattering is due to a potential $V(\mathbf{r})$. Calculate the Born approximation for the case $V(\mathbf{r})=-a \delta(\mathbf{r}) .$

  Electrons with de Broglie wavelength $\lambda$ are incident on a target composed of many randomly oriented small crystals. They are found to be scattered strongly through an angle of $60^{\circ}$. What is the likely distance between planes of atoms in the crystal responsible for the scattering?

  comment

2009

• Paper 1, Section II, $34 \mathrm{D}$

  Applications of Quantum Mechanics | Part II, 2009

  Consider the scaled one-dimensional Schrödinger equation with a potential $V(x)$ such that there is a complete set of real, normalized bound states $\psi_{n}(x), n=0,1,2, \ldots$, with discrete energies $E_{0}<E_{1}<E_{2}<\ldots$, satisfying

  $$-\frac{d^{2} \psi_{n}}{d x^{2}}+V(x) \psi_{n}=E_{n} \psi_{n}$$

  Show that the quantity

  $$\langle E\rangle=\int_{-\infty}^{\infty}\left(\left(\frac{d \psi}{d x}\right)^{2}+V(x) \psi^{2}\right) d x$$

  where $\psi(x)$ is a real, normalized trial function depending on one or more parameters $\alpha$, can be used to estimate $E_{0}$, and show that $\langle E\rangle \geqslant E_{0}$.

  Let the potential be $V(x)=|x|$. Using a suitable one-parameter family of either Gaussian or piecewise polynomial trial functions, find a good estimate for $E_{0}$ in this case.

  How could you obtain a good estimate for $E_{1}$ ? [ You should suggest suitable trial functions, but DO NOT carry out any further integration.]

  comment

• Paper 2, Section II, D

  Applications of Quantum Mechanics | Part II, 2009

  A particle scatters quantum mechanically off a spherically symmetric potential $V(r)$. In the $l=0$ sector, and assuming $\hbar^{2} / 2 m=1$, the radial wavefunction $u(r)$ satisfies

  $$-\frac{d^{2} u}{d r^{2}}+V(r) u=k^{2} u$$

  and $u(0)=0$. The asymptotic behaviour of $u$, for large $r$, is

  $$u(r) \sim C\left(S(k) e^{i k r}-e^{-i k r}\right)$$

  where $C$ is a constant. Show that if $S(k)$ is analytically continued to complex $k$, then

  $$S(k) S(-k)=1 \quad \text { and } \quad S(k)^{*} S\left(k^{*}\right)=1$$

  Deduce that for real $k, S(k)=e^{2 i \delta_{0}(k)}$ for some real function $\delta_{0}(k)$, and that $\delta_{0}(k)=-\delta_{0}(-k) .$

  For a certain potential,

  $$S(k)=\frac{(k+i \lambda)(k+3 i \lambda)}{(k-i \lambda)(k-3 i \lambda)}$$

  where $\lambda$ is a real, positive constant. Evaluate the scattering length $a$ and the total cross section $4 \pi a^{2}$.

  Briefly explain the significance of the zeros of $S(k)$.

  comment

• Paper 3, Section II, D

  Applications of Quantum Mechanics | Part II, 2009

  An electron of charge $-e$ and mass $m$ is subject to a magnetic field of the form $\mathbf{B}=(0,0, B(y))$, where $B(y)$ is everywhere greater than some positive constant $B_{0}$. In a stationary state of energy $E$, the electron's wavefunction $\Psi$ satisfies

  $$-\frac{\hbar^{2}}{2 m}\left(\boldsymbol{\nabla}+\frac{i e}{\hbar} \mathbf{A}\right)^{2} \Psi+\frac{e \hbar}{2 m} \mathbf{B} \cdot \boldsymbol{\sigma} \Psi=E \Psi,$$

  where $\mathbf{A}$ is the vector potential and $\sigma_{1}, \sigma_{2}$ and $\sigma_{3}$ are the Pauli matrices.

  Assume that the electron is in a spin down state and has no momentum along the $z$-axis. Show that with a suitable choice of gauge, and after separating variables, equation (*) can be reduced to

  $$-\frac{d^{2} \chi}{d y^{2}}+(k+a(y))^{2} \chi-b(y) \chi=\epsilon \chi,$$

  where $\chi$ depends only on $y, \epsilon$ is a rescaled energy, and $b(y)$ a rescaled magnetic field strength. What is the relationship between $a(y)$ and $b(y)$ ?

  Show that $(* *)$ can be factorized in the form $M^{\dagger} M \chi=\epsilon \chi$ where

  $$M=\frac{d}{d y}+W(y)$$

  for some function $W(y)$, and deduce that $\epsilon$ is non-negative.

  Show that zero energy states exist for all $k$ and are therefore infinitely degenerate.

  comment

• Paper 4, Section II, D

  Applications of Quantum Mechanics | Part II, 2009

  What are meant by Bloch states and the Brillouin zone for a quantum mechanical particle moving in a one-dimensional periodic potential?

  Derive an approximate value for the lowest-lying energy gap for the Schrödinger equation

  $$-\frac{d^{2} \psi}{d x^{2}}-V_{0}(\cos x+\cos 2 x) \psi=E \psi$$

  when $V_{0}$ is small and positive.

  Estimate the width of this gap in the case that $V_{0}$ is large and positive.

  comment

2008

• 1.II.33E

  Applications of Quantum Mechanics | Part II, 2008

  A beam of particles each of mass $m$ and energy $\hbar^{2} k^{2} /(2 m)$ scatters off an axisymmetric potential $V$. In the first Born approximation the scattering amplitude is

  $$f(\theta)=-\frac{m}{2 \pi \hbar^{2}} \int e^{-i\left(\mathbf{k}-\mathbf{k}_{0}\right) \cdot \mathbf{x}^{\prime}} V\left(\mathbf{x}^{\prime}\right) d^{3} x^{\prime}$$

  where $\mathbf{k}_{0}=(0,0, k)$ is the wave vector of the incident particles and $\mathbf{k}=(k \sin \theta, 0, k \cos \theta)$ is the wave vector of the outgoing particles at scattering angle $\theta$ (and $\phi=0$ ). Let $\mathbf{q}=\mathbf{k}-\mathbf{k}_{0}$ and $q=|\mathbf{q}|$. Show that when the scattering potential $V$ is spherically symmetric the expression $(*)$ simplifies to

  $$f(\theta)=-\frac{2 m}{\hbar^{2} q} \int_{0}^{\infty} r^{\prime} V\left(r^{\prime}\right) \sin \left(q r^{\prime}\right) d r^{\prime}$$

  and find the relation between $q$ and $\theta$.

  Calculate this scattering amplitude for the potential $V(r)=V_{0} e^{-r}$ where $V_{0}$ is a constant, and show that at high energies the particles emerge predominantly in a narrow cone around the forward beam direction. Estimate the angular width of the cone.

  comment

• 2.II.33E

  Applications of Quantum Mechanics | Part II, 2008

  Consider a large, essentially two-dimensional, rectangular sample of conductor of area $A$, and containing $2 N$ electrons of charge $-e$. Suppose a magnetic field of strength $B$ is applied perpendicularly to the sample. Write down the Landau Hamiltonian for one of the electrons assuming that the electron interacts just with the magnetic field.

  [You may ignore the interaction of the electron spin with the magnetic field.]

  Find the allowed energy levels of the electron.

  Find the total energy of the $2 N$ electrons at absolute zero temperature as a function of $B$, assuming that $B$ is in the range

  $$\frac{\pi \hbar N}{e A} \leqslant B \leqslant \frac{2 \pi \hbar N}{e A} .$$

  Comment on the values of the total energy when $B$ takes the values at the two ends of this range.

  comment

• 3.II.33E

  Applications of Quantum Mechanics | Part II, 2008

  Consider the body-centred cuboidal lattice $L$ with lattice points $\left(n_{1} a, n_{2} a, n_{3} b\right)$ and $\left(\left(n_{1}+\frac{1}{2}\right) a,\left(n_{2}+\frac{1}{2}\right) a,\left(n_{3}+\frac{1}{2}\right) b\right)$, where $a$ and $b$ are positive and $n_{1}, n_{2}$ and $n_{3}$ take all possible integer values. Find the reciprocal lattice $\widetilde{L}$and describe its geometrical form. Calculate the volumes of the unit cells of the lattices $L$ and $\widetilde{L}$.

  Find the reciprocal lattice vector associated with the lattice planes parallel to the plane containing the points $(0,0, b),(0, a, b),\left(\frac{1}{2} a, \frac{1}{2} a, \frac{1}{2} b\right),(a, 0,0)$ and $(a, a, 0)$. Deduce the allowed Bragg scattering angles of X-rays off these planes, assuming that $b=\frac{4}{3} a$ and that the X-rays have wavelength $\lambda=\frac{1}{2} a$.

  comment

• 4.II.33E

  Applications of Quantum Mechanics | Part II, 2008

  Explain why the allowed energies of electrons in a three-dimensional crystal lie in energy bands. What quantum numbers can be used to classify the electron energy eigenstates?

  Describe the effect on the energy level structure of adding a small density of impurity atoms randomly to the crystal.

  comment

2007

• 1.II.33A

  Applications of Quantum Mechanics | Part II, 2007

  In a certain spherically symmetric potential, the radial wavefunction for particle scattering in the $l=0$ sector ( $S$-wave), for wavenumber $k$ and $r \gg 0$, is

  $$R(r, k)=\frac{A}{k r}\left(g(-k) e^{-i k r}-g(k) e^{i k r}\right)$$

  where

  $$g(k)=\frac{k+i \kappa}{k-i \alpha}$$

  with $\kappa$ and $\alpha$ real, positive constants. Scattering in sectors with $l \neq 0$ can be neglected. Deduce the formula for the $S$-matrix in this case and show that it satisfies the expected symmetry and reality properties. Show that the phase shift is

  $$\delta(k)=\tan ^{-1} \frac{k(\kappa+\alpha)}{k^{2}-\kappa \alpha}$$

  What is the scattering length for this potential?

  From the form of the radial wavefunction, deduce the energies of the bound states, if any, in this system. If you were given only the $S$-matrix as a function of $k$, and no other information, would you reach the same conclusion? Are there any resonances here?

  [Hint: Recall that $S(k)=e^{2 i \delta(k)}$ for real $k$, where $\delta(k)$ is the phase shift.]

  comment

• 2.II.33A

  Applications of Quantum Mechanics | Part II, 2007

  Describe the variational method for estimating the ground state energy of a quantum system. Prove that an error of order $\epsilon$ in the wavefunction leads to an error of order $\epsilon^{2}$ in the energy.

  Explain how the variational method can be generalized to give an estimate of the energy of the first excited state of a quantum system.

  Using the variational method, estimate the energy of the first excited state of the anharmonic oscillator with Hamiltonian

  $$H=-\frac{d^{2}}{d x^{2}}+x^{2}+x^{4}$$

  How might you improve your estimate?

  [Hint: If $I_{2 n}=\int_{-\infty}^{\infty} x^{2 n} e^{-a x^{2}} d x$ then

  $$\left.I_{0}=\sqrt{\frac{\pi}{a}}, \quad I_{2}=\sqrt{\frac{\pi}{a}} \frac{1}{2 a}, \quad I_{4}=\sqrt{\frac{\pi}{a}} \frac{3}{4 a^{2}}, \quad I_{6}=\sqrt{\frac{\pi}{a}} \frac{15}{8 a^{3}}\right]$$

  comment

• 3.II.33A

  Applications of Quantum Mechanics | Part II, 2007

  Consider the Hamiltonian

  $$H=\mathbf{B}(t) \cdot \mathbf{S}$$

  for a particle of spin $\frac{1}{2}$ fixed in space, in a rotating magnetic field, where

  $$S_{1}=\frac{\hbar}{2}\left(\begin{array}{ll} 0 & 1 \\ 1 & 0 \end{array}\right), \quad S_{2}=\frac{\hbar}{2}\left(\begin{array}{cc} 0 & -i \\ i & 0 \end{array}\right), \quad S_{3}=\frac{\hbar}{2}\left(\begin{array}{cc} 1 & 0 \\ 0 & -1 \end{array}\right)$$

  and

  $$\mathbf{B}(t)=B(\sin \alpha \cos \omega t, \sin \alpha \sin \omega t, \cos \alpha)$$

  with $B, \alpha$ and $\omega$ constant, and $B>0, \omega>0$.

  There is an exact solution of the time-dependent Schrödinger equation for this Hamiltonian,

  $$\chi(t)=\left(\cos \left(\frac{1}{2} \lambda t\right)-i \frac{B-\omega \cos \alpha}{\lambda} \sin \left(\frac{1}{2} \lambda t\right)\right) e^{-i \omega t / 2} \chi_{+}+i\left(\frac{\omega}{\lambda} \sin \alpha \sin \left(\frac{1}{2} \lambda t\right)\right) e^{i \omega t / 2} \chi_{-}$$

  where $\lambda \equiv\left(\omega^{2}-2 \omega B \cos \alpha+B^{2}\right)^{1 / 2}$ and

  $$\chi_{+}=\left(\begin{array}{c} \cos \frac{\alpha}{2} \\ e^{i \omega t} \sin \frac{\alpha}{2} \end{array}\right), \quad \chi_{-}=\left(\begin{array}{c} e^{-i \omega t} \sin \frac{\alpha}{2} \\ -\cos \frac{\alpha}{2} \end{array}\right)$$

  Show that, for $\omega \ll B$, this exact solution simplifies to a form consistent with the adiabatic approximation. Find the dynamic phase and the geometric phase in the adiabatic regime. What is the Berry phase for one complete cycle of $\mathbf{B}$ ?

  The Berry phase can be calculated as an integral of the form

  $$\Gamma=i \oint\left\langle\psi \mid \nabla_{\mathbf{R}} \psi\right\rangle \cdot d \mathbf{R}$$

  Evaluate $\Gamma$ for the adiabatic evolution described above.

  comment

• 4.II.33A

  Applications of Quantum Mechanics | Part II, 2007

  Consider a 1-dimensional chain of $2 N$ atoms of mass $m$ (with $N$ large and with periodic boundary conditions). The interactions between neighbouring atoms are modelled by springs with alternating spring constants $K$ and $G$, with $K>G$.

  

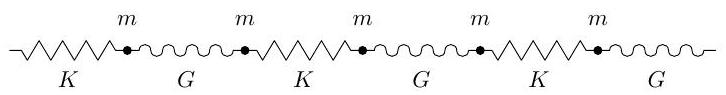
  In equilibrium, the separation of the atoms is $a$, the natural length of the springs.

  Find the frequencies of the longitudinal modes of vibration for this system, and show that they are labelled by a wavenumber $q$ that is restricted to a Brillouin zone. Identify the acoustic and optical bands of the vibration spectrum, and determine approximations for the frequencies near the centre of the Brillouin zone. What is the frequency gap between the acoustic and optical bands at the zone boundary?

  Describe briefly the properties of the phonons in this system.

  comment

2006

• 1.II.33A

  Applications of Quantum Mechanics | Part II, 2006

  Consider a particle of mass $m$ and momentum $\hbar k$ moving under the influence of a spherically symmetric potential $V(r)$ such that $V(r)=0$ for $r \geqslant a$. Define the scattering amplitude $f(\theta)$ and the phase shift $\delta_{\ell}(k)$. Here $\theta$ is the scattering angle. How is $f(\theta)$ related to the differential cross section?

  Obtain the partial-wave expansion

  $$f(\theta)=\frac{1}{k} \sum_{\ell=0}^{\infty}(2 \ell+1) e^{i \delta_{\ell}} \sin \delta_{\ell} P_{\ell}(\cos \theta) .$$

  Let $R_{\ell}(r)$ be a solution of the radial Schrödinger equation, regular at $r=0$, for energy $\hbar^{2} k^{2} / 2 m$ and angular momentum $\ell$. Let

  $$Q_{\ell}(k)=a \frac{R_{\ell}^{\prime}(a)}{R_{\ell}(a)}-k a \frac{j_{\ell}^{\prime}(k a)}{j_{\ell}(k a)}$$

  Obtain the relation

  $$\tan \delta_{\ell}=\frac{Q_{\ell}(k) j_{\ell}^{2}(k a) k a}{Q_{\ell}(k) n_{\ell}(k a) j_{\ell}(k a) k a-1} .$$

  Suppose that

  $$\tan \delta_{\ell} \approx \frac{\gamma}{k_{0}-k},$$

  for some $\ell$, with all other $\delta_{\ell}$ small for $k \approx k_{0}$. What does this imply for the differential cross section when $k \approx k_{0}$ ?

  [For $V=0$, the two independent solutions of the radial Schrödinger equation are $j_{\ell}(k r)$ and $n_{\ell}(k r)$ with

  $$\begin{aligned} j_{\ell}(\rho) & \sim \frac{1}{\rho} \sin \left(\rho-\frac{1}{2} \ell \pi\right), \quad n_{\ell}(\rho) \sim-\frac{1}{\rho} \cos \left(\rho-\frac{1}{2} \ell \pi\right) \quad \text { as } \quad \rho \rightarrow \infty \\ e^{i \rho \cos \theta} &=\sum_{\ell=0}^{\infty}(2 \ell+1) i^{\ell} j_{\ell}(\rho) P_{\ell}(\cos \theta) \end{aligned}$$

  Note that the Wronskian $\rho^{2}\left(j_{\ell}(\rho) n_{\ell}^{\prime}(\rho)-j_{\ell}^{\prime}(\rho) n_{\ell}(\rho)\right)$ is independent of $\left.\rho .\right]$

  comment

• 2.II.33D

  Applications of Quantum Mechanics | Part II, 2006

  State and prove Bloch's theorem for the electron wave functions for a periodic potential $V(\mathbf{r})=V(\mathbf{r}+\mathbf{l})$ where $\mathbf{l}=\sum_{i} n_{i} \mathbf{a}_{i}$ is a lattice vector.

  What is the reciprocal lattice? Explain why the Bloch wave-vector $\mathbf{k}$ is arbitrary up to $\mathbf{k} \rightarrow \mathbf{k}+\mathbf{g}$, where $\mathbf{g}$ is a reciprocal lattice vector.

  Describe in outline why one can expect energy bands $E_{n}(\mathbf{k})=E_{n}(\mathbf{k}+\mathbf{g})$. Explain how $\mathbf{k}$ may be restricted to a Brillouin zone $B$ and show that the number of states in volume $d^{3} k$ is

  $$\frac{2}{(2 \pi)^{3}} \mathrm{~d}^{3} k$$

  Assuming that the velocity of an electron in the energy band with Bloch wave-vector $\mathbf{k}$ is

  $$\mathbf{v}(\mathbf{k})=\frac{1}{\hbar} \frac{\partial}{\partial \mathbf{k}} E_{n}(\mathbf{k})$$

  show that the contribution to the electric current from a full energy band is zero. Given that $n(\mathbf{k})=1$ for each occupied energy level, show that the contribution to the current density is then

  $$\mathbf{j}=-e \frac{2}{(2 \pi)^{3}} \int_{B} \mathrm{~d}^{3} k n(\mathbf{k}) \mathbf{v}(\mathbf{k})$$

  where $-e$ is the electron charge.

  comment

• 3.II.33A

  Applications of Quantum Mechanics | Part II, 2006

  Consider a one-dimensional crystal of lattice space $b$, with atoms having positions $x_{s}$ and momenta $p_{s}, s=0,1,2, \ldots, N-1$, such that the classical Hamiltonian is

  $$H=\sum_{s=0}^{N-1}\left(\frac{p_{s}^{2}}{2 m}+\frac{1}{2} m \lambda^{2}\left(x_{s+1}-x_{s}-b\right)^{2}\right)$$

  where we identify $x_{N}=x_{0}$. Show how this may be quantized to give the energy eigenstates consisting of a ground state $|0\rangle$ together with free phonons with energy $\hbar \omega\left(k_{r}\right)$ where $k_{r}=2 \pi r / N b$ for suitable integers $r$. Obtain the following expression for the quantum operator $x_{s}$

  $$x_{s}=s b+\left(\frac{\hbar}{2 m N}\right)^{\frac{1}{2}} \sum_{r} \frac{1}{\sqrt{\omega\left(k_{r}\right)}}\left(a_{r} e^{i k_{r} s b}+a_{r}^{\dagger} e^{-i k_{r} s b}\right)$$

  where $a_{r}, a_{r}^{\dagger}$ are annihilation and creation operators, respectively.

  An interaction involves the matrix element

  $$M=\sum_{s=0}^{N-1}\left\langle 0\left|e^{i q x_{s}}\right| 0\right\rangle .$$

  Calculate this and show that $|M|^{2}$ has its largest value when $q=2 \pi n / b$ for integer $n$.

  Disregard the case $\omega\left(k_{r}\right)=0$.

  [You may use the relations

  $$\sum_{s=0}^{N-1} e^{i k_{r} s b}= \begin{cases}N, & r=N b \\ 0 & \text { otherwise }\end{cases}$$

  and $e^{A+B}=e^{A} e^{B} e^{-\frac{1}{2}[A, B]}$ if $[A, B]$ commutes with $A$ and with $\left.B .\right]$

  comment

• 4.II.33D

  Applications of Quantum Mechanics | Part II, 2006

  For the one-dimensional potential

  $$V(x)=-\frac{\hbar^{2} \lambda}{m} \sum_{n} \delta(x-n a)$$

  solve the Schrödinger equation for negative energy and obtain an equation that determines possible energy bands. Show that the results agree with the tight-binding model in appropriate limits.

  [It may be useful to note that $\left.V(x)=-\frac{\hbar^{2} \lambda}{m a} \sum_{n} e^{2 \pi i n x / a} .\right]$

  comment

2005

• 1.II.33B

  Applications of Quantum Mechanics | Part II, 2005

  A beam of particles is incident on a central potential $V(r)(r=|\mathbf{x}|)$ that vanishes for $r>R$. Define the differential cross-section $d \sigma / d \Omega$.

  Given that each incoming particle has momentum $\hbar \mathbf{k}$, explain the relevance of solutions to the time-independent Schrödinger equation with the asymptotic form

  $$\psi(\mathbf{x}) \sim e^{i \mathbf{k} \cdot \mathbf{x}}+f(\hat{\mathbf{x}}) \frac{e^{i k r}}{r}$$

  as $r \rightarrow \infty$, where $k=|\mathbf{k}|$ and $\hat{\mathbf{x}}=\mathbf{x} / r$. Write down a formula that determines $d \sigma / d \Omega$ in this case.

  Write down the time-independent Schrödinger equation for a particle of mass $m$ and energy $E=\frac{\hbar^{2} k^{2}}{2 m}$ in a central potential $V(r)$, and show that it allows a solution of the form

  $$\psi(\mathbf{x})=e^{i \mathbf{k} \cdot \mathbf{x}}-\frac{m}{2 \pi \hbar^{2}} \int d^{3} x^{\prime} \frac{e^{i k\left|\mathbf{x}-\mathbf{x}^{\prime}\right|}}{\left|\mathbf{x}-\mathbf{x}^{\prime}\right|} V\left(r^{\prime}\right) \psi\left(\mathbf{x}^{\prime}\right) .$$

  Show that this is consistent with $(*)$ and deduce an expression for $f(\hat{\mathbf{x}})$. Obtain the Born approximation for $f(\hat{\mathbf{x}})$, and show that $f(\hat{\mathbf{x}})=F(k \hat{\mathbf{x}}-\mathbf{k})$, where

  $$F(\mathbf{q})=-\frac{m}{2 \pi \hbar^{2}} \int d^{3} x e^{-i \mathbf{q} \cdot \mathbf{x}} V(r)$$

  Under what conditions is the Born approximation valid?

  Obtain a formula for $f(\hat{\mathbf{x}})$ in terms of the scattering angle $\theta$ in the case that

  $$V(r)=K \frac{e^{-\mu r}}{r},$$

  for constants $K$ and $\mu$. Hence show that $f(\hat{\mathbf{x}})$ is independent of $\hbar$ in the limit $\mu \rightarrow 0$, when expressed in terms of $\theta$ and the energy $E$.

  [You may assume that $\left.\left(\nabla^{2}+k^{2}\right)\left(\frac{e^{i k r}}{r}\right)=-4 \pi \delta^{3}(\mathbf{x}) .\right]$

  comment

• 2.II.33B

  Applications of Quantum Mechanics | Part II, 2005

  Describe briefly the variational approach to the determination of an approximate ground state energy $E_{0}$ of a Hamiltonian $H$.

  Let $\left|\psi_{1}\right\rangle$ and $\left|\psi_{2}\right\rangle$ be two states, and consider the trial state

  $$|\psi\rangle=a_{1}\left|\psi_{1}\right\rangle+a_{2}\left|\psi_{2}\right\rangle$$

  for real constants $a_{1}$ and $a_{2}$. Given that

  $$\begin{aligned} \left\langle\psi_{1} \mid \psi_{1}\right\rangle &=\left\langle\psi_{2} \mid \psi_{2}\right\rangle=1, &\left\langle\psi_{2} \mid \psi_{1}\right\rangle=\left\langle\psi_{1} \mid \psi_{2}\right\rangle=s, \\ \left\langle\psi_{1}|H| \psi_{1}\right\rangle &=\left\langle\psi_{2}|H| \psi_{2}\right\rangle=\mathcal{E}, &\left\langle\psi_{2}|H| \psi_{1}\right\rangle=\left\langle\psi_{1}|H| \psi_{2}\right\rangle=\epsilon, \end{aligned}$$

  and that $\epsilon<s \mathcal{E}$, obtain an upper bound on $E_{0}$ in terms of $\mathcal{E}, \epsilon$ and $s$.

  The normalized ground-state wavefunction of the Hamiltonian

  $$H_{1}=\frac{p^{2}}{2 m}-K \delta(x), \quad K>0,$$

  $$\psi_{1}(x)=\sqrt{\lambda} e^{-\lambda|x|}, \quad \lambda=\frac{m K}{\hbar^{2}} .$$

  Verify that the ground state energy of $H_{1}$ is

  $$E_{B} \equiv\left\langle\psi_{1}|H| \psi_{1}\right\rangle=-\frac{1}{2} K \lambda .$$

  Now consider the Hamiltonian

  $$H=\frac{p^{2}}{2 m}-K \delta(x)-K \delta(x-R)$$

  and let $E_{0}(R)$ be its ground-state energy as a function of $R$. Assuming that

  $$\psi_{2}(x)=\sqrt{\lambda} e^{-\lambda|x-R|},$$

  use $(*)$ to compute $s, \mathcal{E}$ and $\epsilon$ for $\psi_{1}$ and $\psi_{2}$ as given. Hence show that

  $$E_{0}(R) \leqslant E_{B}\left[1+2 \frac{e^{-\lambda R}\left(1+e^{-\lambda R}\right)}{1+(1+\lambda R) e^{-\lambda R}}\right]$$

  Why should you expect this inequality to become an approximate equality for sufficiently large $R$ ? Describe briefly how this is relevant to molecular binding.

  comment

• 3.II.33B

  Applications of Quantum Mechanics | Part II, 2005

  Let $\{\mathbf{l}\}$ be the set of lattice vectors of some lattice. Define the reciprocal lattice. What is meant by a Bravais lattice?

  Let $\mathbf{i}, \mathbf{j}, \mathbf{k}$ be mutually orthogonal unit vectors. A crystal has identical atoms at positions given by the vectors

  $$\begin{array}{ll} a\left[n_{1} \mathbf{i}+n_{2} \mathbf{j}+n_{3} \mathbf{k}\right], & a\left[\left(n_{1}+\frac{1}{2}\right) \mathbf{i}+\left(n_{2}+\frac{1}{2}\right) \mathbf{j}+n_{3} \mathbf{k}\right] \\ a\left[\left(n_{1}+\frac{1}{2}\right) \mathbf{i}+\mathbf{j}+\left(n_{3}+\frac{1}{2}\right) \mathbf{k}\right], & a\left[n_{1} \mathbf{i}+\left(n_{2}+\frac{1}{2}\right) \mathbf{j}+\left(n_{3}+\frac{1}{2}\right) \mathbf{k}\right] \end{array}$$

  where $\left(n_{1}, n_{2}, n_{3}\right)$ are arbitrary integers and $a$ is a constant. Show that these vectors define a Bravais lattice with basis vectors

  $$\mathbf{a}_{1}=a \frac{1}{2}(\mathbf{j}+\mathbf{k}), \quad \mathbf{a}_{2}=a \frac{1}{2}(\mathbf{i}+\mathbf{k}), \quad \mathbf{a}_{3}=a \frac{1}{2}(\mathbf{i}+\mathbf{j})$$

  Verify that a basis for the reciprocal lattice is

  $$\mathbf{b}_{1}=\frac{2 \pi}{a}(\mathbf{j}+\mathbf{k}-\mathbf{i}), \quad \mathbf{b}_{2}=\frac{2 \pi}{a}(\mathbf{i}+\mathbf{k}-\mathbf{j}), \quad \mathbf{b}_{3}=\frac{2 \pi}{a}(\mathbf{i}+\mathbf{j}-\mathbf{k})$$

  In Bragg scattering, an incoming plane wave of wave-vector $\mathbf{k}$ is scattered to an outgoing wave of wave-vector $\mathbf{k}^{\prime}$. Explain why $\mathbf{k}^{\prime}=\mathbf{k}+\mathbf{g}$ for some reciprocal lattice vector g. Given that $\theta$ is the scattering angle, show that

  $$\sin \frac{1}{2} \theta=\frac{|\mathbf{g}|}{2|\mathbf{k}|} .$$

  For the above lattice, explain why you would expect scattering through angles $\theta_{1}$ and $\theta_{2}$ such that

  $$\frac{\sin \frac{1}{2} \theta_{1}}{\sin \frac{1}{2} \theta_{2}}=\frac{\sqrt{3}}{2}$$

  comment

• 4.II.33B

  Applications of Quantum Mechanics | Part II, 2005

  A semiconductor has a valence energy band with energies $E \leqslant 0$ and density of states $g_{v}(E)$, and a conduction energy band with energies $E \geqslant E_{g}$ and density of states $g_{c}(E)$. Assume that $g_{v}(E) \sim A_{v}(-E)^{\frac{1}{2}}$ as $E \rightarrow 0$, and that $g_{c}(E) \sim A_{c}\left(E-E_{g}\right)^{\frac{1}{2}}$ as $E \rightarrow E_{g}$. At zero temperature all states in the valence band are occupied and the conduction band is empty. Let $p$ be the number of holes in the valence band and $n$ the number of electrons in the conduction band at temperature $T$. Under suitable approximations derive the result

  $$p n=N_{v} N_{c} e^{-E_{g} / k T}$$

  where

  $$N_{v}=\frac{1}{2} \sqrt{\pi} A_{v}(k T)^{\frac{3}{2}}, \quad N_{c}=\frac{1}{2} \sqrt{\pi} A_{c}(k T)^{\frac{3}{2}} .$$

  Briefly describe how a semiconductor may conduct electricity but with a conductivity that is strongly temperature dependent.

  Describe how doping of the semiconductor leads to $p \neq n$. A $p n$ junction is formed between an $n$-type semiconductor, with $N_{d}$ donor atoms, and a $p$-type semiconductor, with $N_{a}$ acceptor atoms. Show that there is a potential difference $V_{n p}=\Delta E /|e|$ across the junction, where $e$ is the electron charge, and

  $$\Delta E=E_{g}-k T \ln \frac{N_{v} N_{c}}{N_{d} N_{a}} .$$

  Two semiconductors, one $p$-type and one $n$-type, are joined to make a closed circuit with two $p n$ junctions. Explain why a current will flow around the circuit if the junctions are at different temperatures.

  [The Fermi-Dirac distribution function at temperature $T$ and chemical potential $\mu$ is $\frac{g(E)}{e^{(E-\mu) / k T}+1}$, where $g(E)$ is the number of states with energy $E$.

  Note that $\int_{0}^{\infty} x^{\frac{1}{2}} e^{-x} d x=\frac{1}{2} \sqrt{\pi}$.]

  comment

2004

• B1.23

  Applications of Quantum Mechanics | Part II, 2004

  The operator corresponding to a rotation through an angle $\theta$ about an axis $\mathbf{n}$, where $\mathbf{n}$ is a unit vector, is

  $$U(\mathbf{n}, \theta)=e^{i \theta \mathbf{n} \cdot \mathbf{J} / \hbar}$$

  If $U$ is unitary show that $\mathbf{J}$ must be hermitian. Let $\mathbf{V}=\left(V_{1}, V_{2}, V_{3}\right)$ be a vector operator such that

  $$U(\mathbf{n}, \delta \theta) \mathbf{V} U(\mathbf{n}, \delta \theta)^{-1}=\mathbf{V}+\delta \theta \mathbf{n} \times \mathbf{V} .$$

  Work out the commutators $\left[J_{i}, V_{j}\right]$. Calculate

  $$U(\hat{\mathbf{z}}, \theta) \mathbf{V U}(\hat{\mathbf{z}}, \theta)^{-1}$$

  for each component of $\mathbf{V}$.

  If $|j m\rangle$ are standard angular momentum states determine $\left\langle j m^{\prime}|U(\hat{\mathbf{z}}, \theta)| j m\right\rangle$ for any $j, m, m^{\prime}$ and also determine $\left\langle\frac{1}{2} m^{\prime}|U(\hat{\mathbf{y}}, \theta)| \frac{1}{2} m\right\rangle$.

  $\left[\right.$ Hint $\left.: J_{3}|j m\rangle=m \hbar|j m\rangle, J_{+}\left|\frac{1}{2}-\frac{1}{2}\right\rangle=\hbar\left|\frac{1}{2} \frac{1}{2}\right\rangle, J_{-}\left|\frac{1}{2} \frac{1}{2}\right\rangle=\hbar\left|\frac{1}{2}-\frac{1}{2}\right\rangle \cdot\right]$

  comment

• B2.23

  Applications of Quantum Mechanics | Part II, 2004

  The wave function for a single particle with a potential $V(r)$ has the asymptotic form for large $r$

  $$\psi(r, \theta) \sim e^{i k r \cos \theta}+f(\theta) \frac{e^{i k r}}{r} .$$

  How is $f(\theta)$ related to observable quantities? Show how $f(\theta)$ can be expressed in terms of phase shifts $\delta_{\ell}(k)$ for $\ell=0,1,2, \ldots$..

  Assume that $V(r)=0$ for $r \geq a$, and let $R_{\ell}(r)$ denote the solution of the radial Schrödinger equation, regular at $r=0$, with energy $\hbar^{2} k^{2} / 2 m$ and angular momentum $\ell$. Let $N_{\ell}(k)=a R_{\ell}^{\prime}(a) / R_{\ell}(a)$. Show that

  $$\tan \delta_{\ell}(k)=\frac{N_{\ell}(k) j_{\ell}(k a)-k a j_{\ell}^{\prime}(k a)}{N_{\ell}(k) n_{\ell}(k a)-k a n_{\ell}^{\prime}(k a)} .$$

  Assuming that $N_{\ell}(k)$ is a smooth function for $k \approx 0$, determine the expected behaviour of $\delta_{\ell}(k)$ as $k \rightarrow 0$. Show that for $k \rightarrow 0$ then $f(\theta) \rightarrow c$, with $c$ a constant, and determine $c$ in terms of $N_{0}(0)$.

  [For $V=0$ the two independent solutions of the radial Schrödinger equation are $j_{\ell}(k r)$ and $n_{\ell}(k r)$ with

  $$\begin{aligned} j_{\ell}(\rho) & \sim \frac{1}{\rho} \sin \left(\rho-\frac{1}{2} \ell \pi\right), n_{\ell}(\rho) \sim-\frac{1}{\rho} \cos \left(\rho-\frac{1}{2} \ell \pi\right) \text { as } \rho \rightarrow \\ j_{\ell}(\rho) & \propto \rho^{\ell}, n_{\ell}(\rho) \propto \rho^{-\ell-1} \text { as } \rho \rightarrow 0 \\ e^{i \rho \cos \theta} &=\sum_{\ell=0}^{\infty}(2 \ell+1) i^{\ell} j_{\ell}(\rho) P_{\ell}(\cos \theta), \\ j_{0}(\rho) &=\frac{\sin \rho}{\rho}, \quad n_{0}(\rho)=-\frac{\cos \rho}{\rho} \end{aligned}$$

  comment

• B3.23

  Applications of Quantum Mechanics | Part II, 2004

  For a periodic potential $V(\mathbf{r})=V(\mathbf{r}+\ell)$, where $\ell$ is a lattice vector, show that we may write

  $$V(\mathbf{r})=\sum_{\mathbf{g}} a_{\mathbf{g}} e^{i \mathbf{g} \cdot \mathbf{r}}, \quad a_{\mathbf{g}}^{*}=a_{-\mathbf{g}}$$

  where the set of $g$ should be defined.

  Show how to construct general wave functions satisfying $\psi(\mathbf{r}+\boldsymbol{\ell})=e^{i \mathbf{k} \cdot \boldsymbol{\ell}} \psi(\mathbf{r})$ in terms of free plane-wave wave-functions.

  Show that the nearly free electron model gives an energy gap $2\left|a_{\mathbf{g}}\right|$ when $\mathbf{k}=\frac{1}{2} \mathbf{g}$.

  Explain why, for a periodic potential, the allowed energies form bands $E_{n}(\mathbf{k})$ where $\mathbf{k}$ may be restricted to a single Brillouin zone. Show that $E_{n}(\mathbf{k})=E_{n}(\mathbf{k}+\mathbf{g})$ if $\mathbf{k}$ and $\mathbf{k}+\mathbf{g}$ belong to the Brillouin zone.

  How are bands related to whether a material is a conductor or an insulator?

  comment

• B4.24

  Applications of Quantum Mechanics | Part II, 2004

  Describe briefly the variational approach to determining approximate energy eigenvalues for a Hamiltonian $H$.

  Consider a Hamiltonian $H$ and two states $\left|\psi_{1}\right\rangle,\left|\psi_{2}\right\rangle$ such that

  $$\begin{array}{cl} \left\langle\psi_{1}|H| \psi_{1}\right\rangle=\left\langle\psi_{2}|H| \psi_{2}\right\rangle=\mathcal{E}, & \left\langle\psi_{2}|H| \psi_{1}\right\rangle=\left\langle\psi_{1}|H| \psi_{2}\right\rangle=\varepsilon \\ \left\langle\psi_{1} \mid \psi_{1}\right\rangle=\left\langle\psi_{2} \mid \psi_{2}\right\rangle=1, & \left\langle\psi_{2} \mid \psi_{1}\right\rangle=\left\langle\psi_{1} \mid \psi_{2}\right\rangle=s \end{array}$$

  Show that, by considering a linear combination $\alpha\left|\psi_{1}\right\rangle+\beta\left|\psi_{2}\right\rangle$, the variational method gives

  $$\frac{\mathcal{E}-\varepsilon}{1-s}, \quad \frac{\mathcal{E}+\varepsilon}{1+s}$$

  as approximate energy eigenvalues.

  Consider the Hamiltonian for an electron in the presence of two protons at $\mathbf{0}$ and $\mathbf{R}$,

  $$H=\frac{\mathbf{p}^{2}}{2 m}+\frac{e^{2}}{4 \pi \epsilon_{0}}\left(\frac{1}{R}-\frac{1}{|\mathbf{r}|}-\frac{1}{|\mathbf{r}-\mathbf{R}|}\right), \quad R=|\mathbf{R}|$$

  Let $\psi_{0}(\mathbf{r})=e^{-r / a} /\left(\pi a^{3}\right)^{\frac{1}{2}}$ be the ground state hydrogen atom wave function which satisfies

  $$\left(\frac{\mathbf{p}^{2}}{2 m}-\frac{e^{2}}{4 \pi \epsilon_{0}|\mathbf{r}|}\right) \psi_{0}(\mathbf{r})=E_{0} \psi_{0}(\mathbf{r}) .$$

  It is given that

  $$\begin{aligned} &S=\int \mathrm{d}^{3} r \psi_{0}(\mathbf{r}) \psi_{0}(\mathbf{r}-\mathbf{R})=\left(1+\frac{R}{a}+\frac{R^{2}}{3 a^{2}}\right) e^{-R / a} \\ &U=\int \mathrm{d}^{3} r \frac{1}{|\mathbf{r}|} \psi_{0}(\mathbf{r}) \psi_{0}(\mathbf{r}-\mathbf{R})=\frac{1}{a}\left(1+\frac{R}{a}\right) e^{-R / a} \end{aligned}$$

  and, for large $R$, that

  $$\int \mathrm{d}^{3} r \frac{1}{|\mathbf{r}-\mathbf{R}|} \psi_{0}(\mathbf{r})^{2}-\frac{1}{R}=\mathrm{O}\left(e^{-2 R / a}\right)$$

  Consider the trial wave function $\alpha \psi_{0}(\mathbf{r})+\beta \psi_{0}(\mathbf{r}-\mathbf{R})$. Show that the variational estimate for the ground state energy for large $R$ is

  $$E(R)=E_{0}+\frac{e^{2}}{4 \pi \epsilon_{0} R}(S-R U)+\mathrm{O}\left(e^{-2 R / a}\right) .$$

  Explain why there is an attractive force between the two protons for large $R$.

  comment

2003

• B1.23

  Applications of Quantum Mechanics | Part II, 2003

  Define the differential cross section $\frac{d \sigma}{d \Omega}$. Show how it may be related to a scattering amplitude $f$ defined in terms of the behaviour of a wave function $\psi$ satisfying suitable boundary conditions as $r=|\mathbf{r}| \rightarrow \infty$.

  For a particle scattering off a potential $V(r)$ show how $f(\theta)$, where $\theta$ is the scattering angle, may be expanded, for energy $E=\hbar^{2} k^{2} / 2 m$, as

  $$f(\theta)=\sum_{\ell=0}^{\infty} f_{\ell}(k) P_{\ell}(\cos \theta),$$

  and find $f_{\ell}(k)$ in terms of the phase shift $\delta_{\ell}(k)$. Obtain the optical theorem relating $\sigma_{\text {total }}$ and $f(0)$.

  Suppose that

  $$e^{2 i \delta_{1}}=\frac{E-E_{0}-\frac{1}{2} i \Gamma}{E-E_{0}+\frac{1}{2} i \Gamma}$$

  Why for $E \approx E_{0}$ may $f_{1}(k)$ be dominant, and what is the expected behaviour of $\frac{d \sigma}{d \Omega}$ for $E \approx E_{0}$ ?

  [For large $r$

  $$e^{i k r \cos \theta} \sim \frac{1}{2 i k r} \sum_{\ell=0}^{\infty}(2 \ell+1)\left((-1)^{\ell+1} e^{-i k r}+e^{i k r}\right) P_{\ell}(\cos \theta)$$

  Legendre polynomials satisfy

  $$\left.\int_{-1}^{1} P_{\ell}(t) P_{\ell^{\prime}}(t) d t=\frac{2}{2 \ell+1} \delta_{\ell \ell^{\prime}} \cdot\right]$$

  comment

• B2.22

  Applications of Quantum Mechanics | Part II, 2003

  The Hamiltonian $H_{0}$ for a single electron atom has energy eigenstates $\left|\psi_{n}\right\rangle$ with energy eigenvalues $E_{n}$. There is an interaction with an electromagnetic wave of the form

  $$H_{1}=-e \mathbf{r} \cdot \boldsymbol{\epsilon} \cos (\mathbf{k} \cdot \mathbf{r}-\omega t), \quad \omega=|\mathbf{k}| c,$$

  where $\boldsymbol{\epsilon}$ is the polarisation vector. At $t=0$ the atom is in the state $\left|\psi_{0}\right\rangle$. Find a formula for the probability amplitude, to first order in $e$, to find the atom in the state $\left|\psi_{1}\right\rangle$ at time $t$. If the atom has a size $a$ and $|\mathbf{k}| a \ll 1$ what are the selection rules which are relevant? For $t$ large, under what circumstances will the transition rate be approximately constant?

  [You may use the result

  $$\left.\int_{-\infty}^{\infty} \frac{\sin ^{2} \lambda t}{\lambda^{2}} d \lambda=\pi|t| . \quad\right]$$

  comment

• B3.23

  Applications of Quantum Mechanics | Part II, 2003

  Consider the two Hamiltonians

  $$\begin{aligned} &H_{1}=\frac{\mathbf{p}^{2}}{2 m}+V(|\mathbf{r}|), \\ &H_{2}=\frac{\mathbf{p}^{2}}{2 m}+\sum_{n_{i} \in \mathbb{Z}} V\left(\left|\mathbf{r}-n_{1} \mathbf{a}_{1}-n_{2} \mathbf{a}_{2}-n_{3} \mathbf{a}_{3}\right|\right), \end{aligned}$$

  where $\mathbf{a}_{i}$ are three linearly independent vectors. For each of the Hamiltonians $H=H_{1}$ and $H=H_{2}$, what are the symmetries of $H$ and what unitary operators $U$ are there such that $U H U^{-1}=H$ ?

  For $\mathrm{H}_{2}$ derive Bloch's theorem. Suppose that $H_{1}$ has energy eigenfunction $\psi_{0}(\mathbf{r})$ with energy $E_{0}$ where $\psi_{0}(\mathbf{r}) \sim N e^{-K r}$ for large $r=|\mathbf{r}|$. Assume that $K\left|\mathbf{a}_{i}\right| \gg 1$ for each $i$. In a suitable approximation derive the energy eigenvalues for $H_{2}$ when $E \approx E_{0}$. Verify that the energy eigenfunctions and energy eigenvalues satisfy Bloch's theorem. What happens if $K\left|\mathbf{a}_{i}\right| \rightarrow \infty$ ?

  comment

• B4.24

  Applications of Quantum Mechanics | Part II, 2003

  Atoms of mass $m$ in an infinite one-dimensional periodic array, with interatomic spacing $a$, have perturbed positions $x_{n}=n a+y_{n}$, for integer $n$. The potential between neighbouring atoms is

  $$\frac{1}{2} \lambda\left(x_{n+1}-x_{n}-a\right)^{2}$$

  for positive constant $\lambda$. Write down the Lagrangian for the variables $y_{n}$. Find the frequency $\omega(k)$ of a normal mode of wavenumber $k$. Define the Brillouin zone and explain why $k$ may be restricted to lie within it.

  Assume now that the array is periodically-identified, so that there are effectively only $N$ atoms in the array and the atomic displacements $y_{n}$ satisfy the periodic boundary conditions $y_{n+N}=y_{n}$. Determine the allowed values of $k$ within the Brillouin zone. Show, for allowed wavenumbers $k$ and $k^{\prime}$, that

  $$\sum_{n=0}^{N-1} e^{i n\left(k-k^{\prime}\right) a}=N \delta_{k, k^{\prime}}$$

  By writing $y_{n}$ as

  $$y_{n}=\frac{1}{\sqrt{N}} \sum_{k} q_{k} e^{i n k a}$$

  where the sum is over allowed values of $k$, find the Lagrangian for the variables $q_{k}$, and hence the Hamiltonian $H$ as a function of $q_{k}$ and the conjugate momenta $p_{k}$. Show that the Hamiltonian operator $\hat{H}$ of the quantum theory can be written in the form

  $$\hat{H}=E_{0}+\sum_{k} \hbar \omega(k) a_{k}^{\dagger} a_{k}$$

  where $E_{0}$ is a constant and $a_{k}, a_{k}^{\dagger}$ are harmonic oscillator annihilation and creation operators. What is the physical interpretation of $a_{k}$ and $a_{k}^{\dagger}$ ? How does this show that phonons have quantized energies?

  comment

2002

• B1.23

  Applications of Quantum Mechanics | Part II, 2002

  A quantum system, with Hamiltonian $H_{0}$, has continuous energy eigenstates $|E\rangle$ for all $E \geq 0$, and also a discrete eigenstate $|0\rangle$, with $H_{0}|0\rangle=E_{0}|0\rangle,\langle 0 \mid 0\rangle=1, E_{0}>0$. A time-independent perturbation $H_{1}$, such that $\left\langle E\left|H_{1}\right| 0\right\rangle \neq 0$, is added to $H_{0}$. If the system is initially in the state $|0\rangle$ obtain the formula for the decay rate

  $$w=\frac{2 \pi}{\hbar} \rho\left(E_{0}\right)\left|\left\langle E_{0}\left|H_{1}\right| 0\right\rangle\right|^{2},$$

  where $\rho$ is the density of states.

  [You may assume that $\frac{1}{t}\left(\frac{\sin \frac{1}{2} \omega t}{\frac{1}{2} \omega}\right)^{2}$ behaves like $2 \pi \delta(\omega)$ for large $t$.]

  Assume that, for a particle moving in one dimension,

  $$H_{0}=E_{0}|0\rangle\left\langle 0\left|+\int_{-\infty}^{\infty} p^{2}\right| p\right\rangle\langle p| d p, \quad H_{1}=f \int_{-\infty}^{\infty}(|p\rangle\langle 0|+| 0\rangle\langle p|) d p$$

  where $\left\langle p^{\prime} \mid p\right\rangle=\delta\left(p^{\prime}-p\right)$, and $f$ is constant. Obtain $w$ in this case.

  comment

• B2.22

  Applications of Quantum Mechanics | Part II, 2002

  Define the reciprocal lattice for a lattice $L$ with lattice vectors $\ell$.

  A beam of electrons, with wave vector $\mathbf{k}$, is incident on a Bravais lattice $L$ with a large number of atoms, $N$. If the scattering amplitude for scattering on an individual atom in the direction $\hat{\mathbf{k}}^{\prime}$ is $f\left(\hat{\mathbf{k}}^{\prime}\right)$, show that the scattering amplitude for the whole lattice

  $$\sum_{\ell \in L} e^{i \mathbf{q} \cdot \ell} f\left(\hat{\mathbf{k}}^{\prime}\right), \quad \mathbf{q}=\mathbf{k}-|\mathbf{k}| \hat{\mathbf{k}}^{\prime}$$

  Derive the formula for the differential cross section

  $$\frac{\mathrm{d} \sigma}{\mathrm{d} \Omega}=N\left|f\left(\hat{\mathbf{k}}^{\prime}\right)\right|^{2} \Delta(\mathbf{q}),$$

  obtaining an explicit form for $\Delta(\mathbf{q})$. Show that $\Delta(\mathbf{q})$ is strongly peaked when $\mathbf{q}=\mathbf{g}$, a reciprocal lattice vector. Show that this leads to the Bragg formula $2 d \sin \frac{\theta}{2}=\lambda$, where $\theta$ is the scattering angle, $\lambda$ the electron wavelength and $d$ the separation between planes of atoms in the lattice.

  comment

• B3.23

  Applications of Quantum Mechanics | Part II, 2002

  A periodic potential is expressed as $V(\mathbf{x})=\sum_{\mathbf{g}} a_{\mathbf{g}} e^{i \mathbf{g} \cdot \mathbf{x}}$, where $\{\mathbf{g}\}$ are reciprocal lattice vectors and $a_{\mathbf{g}}{ }^{*}=a_{-\mathbf{g}}, a_{\mathbf{0}}=0$. In the nearly free electron model explain why it is appropriate, near the boundaries of energy bands, to consider a Bloch wave state

  $$\left|\psi_{\mathbf{k}}\right\rangle=\sum_{r} \alpha_{r}\left|\mathbf{k}_{r}\right\rangle, \quad \mathbf{k}_{r}=\mathbf{k}+\mathbf{g}_{r},$$

  where $|\mathbf{k}\rangle$ is a free electron state for wave vector $\mathbf{k},\left\langle\mathbf{k}^{\prime} \mid \mathbf{k}\right\rangle=\delta_{\mathbf{k}^{\prime} \mathbf{k}}$, and the sum is restricted to reciprocal lattice vectors $\mathbf{g}_{r}$ such that $\left|\mathbf{k}_{r}\right| \approx|\mathbf{k}|$. Obtain a determinantal formula for the possible energies $E(\mathbf{k})$ corresponding to Bloch wave states of this form.

  [You may take $\mathbf{g}_{1}=\mathbf{0}$ and assume $e^{i \mathbf{b} \cdot \mathbf{x}}|\mathbf{k}\rangle=|\mathbf{k}+\mathbf{b}\rangle$ for any $\mathbf{b}$.]

  Suppose the sum is restricted to just $\mathbf{k}$ and $\mathbf{k}+\mathbf{g}$. Show that there is a gap $2\left|a_{\mathbf{g}}\right|$ between energy bands. Setting $\mathbf{k}=-\frac{1}{2} \mathbf{g}+\mathbf{q}$, show that there are two Bloch wave states with energies near the boundaries of the energy bands

  $$E_{\pm}(\mathbf{k}) \approx \frac{\hbar^{2}|\mathbf{g}|^{2}}{8 m} \pm\left|a_{\mathbf{g}}\right|+\frac{\hbar^{2}|\mathbf{q}|^{2}}{2 m} \pm \frac{\hbar^{4}}{8 m^{2}\left|a_{\mathbf{g}}\right|}(\mathbf{q} \cdot \mathbf{g})^{2}$$

  What is meant by effective mass? Determine the value of the effective mass at the top and the bottom of the adjacent energy bands if $\mathbf{q}$ is parallel to $\mathrm{g}$.

  comment

• B4.24

  Applications of Quantum Mechanics | Part II, 2002

  Explain the variational method for computing the ground state energy for a quantum Hamiltonian.

  For the one-dimensional Hamiltonian

  $$H=\frac{1}{2} p^{2}+\lambda x^{4},$$

  obtain an approximate form for the ground state energy by considering as a trial state the state $|w\rangle$ defined by $a|w\rangle=0$, where $\langle w \mid w\rangle=1$ and $a=(w / 2 \hbar)^{\frac{1}{2}}(x+i p / w)$.

  [It is useful to note that $\left\langle w\left|\left(a+a^{\dagger}\right)^{4}\right| w\right\rangle=\left\langle w\left|\left(a^{2} a^{\dagger 2}+a a^{\dagger} a a^{\dagger}\right)\right| w\right\rangle$.]

  Explain why the states $a^{\dagger}|w\rangle$ may be used as trial states for calculating the first excited energy level.

  comment

2001

• B1.23

  Applications of Quantum Mechanics | Part II, 2001

  A steady beam of particles, having wavenumber $k$ and moving in the $z$ direction, scatters on a spherically-symmetric potential. Write down the asymptotic form of the wave function at large $r$.

  The incoming wave is written as a partial-wave series

  $$\sum_{\ell=0}^{\infty} \chi_{\ell}(k r) P_{\ell}(\cos \theta)$$

  Show that for large $r$

  $$\chi_{\ell}(k r) \sim \frac{\ell+\frac{1}{2}}{i k r}\left(e^{i k r}-(-1)^{\ell} e^{-i k r}\right)$$

  and calculate $\chi_{0}(k r)$ and $\chi_{1}(k r)$ for all $r$.

  Write down the second-order differential equation satisfied by the $\chi_{\ell}(k r)$. Construct a second linearly-independent solution for each $\ell$ that is singular at $r=0$ and, when it is suitably normalised, has large- $r$ behaviour

  $$\frac{\ell+\frac{1}{2}}{i k r}\left(e^{i k r}+(-1)^{\ell} e^{-i k r}\right)$$

  comment

• B2.22

  Applications of Quantum Mechanics | Part II, 2001

  A particle of charge $e$ moves freely within a cubical box of side $a$. Its initial wavefunction is

  $$(2 / a)^{-\frac{3}{2}} \sin (\pi x / a) \sin (\pi y / a) \sin (\pi z / a) .$$

  A uniform electric field $\mathcal{E}$ in the $x$ direction is switched on for a time $T$. Derive from first principles the probability, correct to order $\mathcal{E}^{2}$, that after the field has been switched off the wave function will be found to be

  $$(2 / a)^{-\frac{3}{2}} \sin (2 \pi x / a) \sin (\pi y / a) \sin (\pi z / a) .$$

  comment

• B3.23

  Applications of Quantum Mechanics | Part II, 2001

  Write down the commutation relations satisfied by the cartesian components of the total angular momentum operator $\mathbf{J}$.

  In quantum mechanics an operator $\mathbf{V}$ is said to be a vector operator if, under rotations, its components transform in the same way as those of the coordinate operator r. Show from first principles that this implies that its cartesian components satisfy the commutation relations

  $$\left[J_{j}, V_{k}\right]=i \epsilon_{j k l} V_{l}$$

  Hence calculate the total angular momentum of the nonvanishing states $V_{j}|0\rangle$, where $|0\rangle$ is the vacuum state.

  comment

• B4.24

  Applications of Quantum Mechanics | Part II, 2001

  Derive the Bloch form of the wave function $\psi(x)$ of an electron moving in a onedimensional crystal lattice.

  The potential in such an $N$-atom lattice is modelled by

  $$V(x)=\sum_{n}\left(-\frac{\hbar^{2} U}{2 m} \delta(x-n L)\right)$$

  Assuming that $\psi(x)$ is continuous across each lattice site, and applying periodic boundary conditions, derive an equation for the allowed electron energy levels. Show that for suitable values of $U L$ they have a band structure, and calculate the number of levels in each band when $U L>2$. Verify that when $U L \gg 1$ the levels are very close to those corresponding to a solitary atom.

  Describe briefly how the band structure in a real 3-dimensional crystal differs from that of this simple model.

  comment