Part II, 2007, Paper 4

Algebraic Topology

• 4.II $. 21 \mathrm{H}$

  Algebraic Topology | Part II, 2007

  Compute the homology of the space obtained from the torus $S^{1} \times S^{1}$ by identifying $S^{1} \times\{p\}$ to a point and $S^{1} \times\{q\}$ to a point, for two distinct points $p$ and $q$ in $S^{1} .$

  comment

Applications of Quantum Mechanics

• 4.II.33A

  Applications of Quantum Mechanics | Part II, 2007

  Consider a 1-dimensional chain of $2 N$ atoms of mass $m$ (with $N$ large and with periodic boundary conditions). The interactions between neighbouring atoms are modelled by springs with alternating spring constants $K$ and $G$, with $K>G$.

  

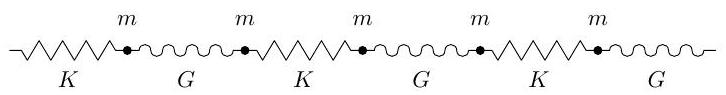
  In equilibrium, the separation of the atoms is $a$, the natural length of the springs.

  Find the frequencies of the longitudinal modes of vibration for this system, and show that they are labelled by a wavenumber $q$ that is restricted to a Brillouin zone. Identify the acoustic and optical bands of the vibration spectrum, and determine approximations for the frequencies near the centre of the Brillouin zone. What is the frequency gap between the acoustic and optical bands at the zone boundary?

  Describe briefly the properties of the phonons in this system.

  comment

Applied Probability

• 4.II.26J

  Applied Probability | Part II, 2007

  A population of rare Monarch butterflies functions as follows. At the times of a Poisson process of rate $\lambda$ a caterpillar is produced from an egg. After an exponential time, the caterpillar is transformed into a pupa which, after an exponential time, becomes a butterfly. The butterfly lives for another exponential time and then dies. (The Poissonian assumption reflects the fact that butterflies lay a huge number of eggs most of which do not develop.) Suppose that all lifetimes are independent (of the arrival process and of each other) and let their rate be $\mu$. Assume that the population is in an equilibrium and let $C$ be the number of caterpillars, $R$ the number of pupae and $B$ the number of butterflies (so that the total number of insects, in any metamorphic form, equals $N=C+R+B)$. Let $\pi_{(c, r, b)}$ be the equilibrium probability $\mathbb{P}(C=c, R=r, B=b)$ where $c, r, b=0,1, \ldots$

  (a) Specify the rates of transitions $(c, r, b) \rightarrow\left(c^{\prime}, r^{\prime}, b^{\prime}\right)$ for the resulting continuous-time Markov chain $\left(X_{t}\right)$ with states $(c, r, b)$. (The rates are non-zero only when $c^{\prime}=c$ or $c^{\prime}=c \pm 1$ and similarly for other co-ordinates.) Check that the holding rate for state $(c, r, b)$ is $\lambda+\mu n$ where $n=c+r+b$.

  (b) Let $Q$ be the Q-matrix from (a). Consider the invariance equation $\pi Q=0$. Verify that the only solution is

  $$\pi_{(c, r, b)}=\frac{(3 \lambda / \mu)^{n}}{3^{n} c ! r ! b !} \exp \left(-\frac{3 \lambda}{\mu}\right), \quad n=c+r+b$$

  (c) Derive the marginal equilibrium probabilities $\mathbb{P}(N=n)$ and the conditional equilibrium probabilities $\mathbb{P}(C=c, R=r, B=b \mid N=n)$.

  (d) Determine whether the chain $\left(X_{t}\right)$ is positive recurrent, null-recurrent or transient.

  (e) Verify that the equilibrium probabilities $\mathbb{P}(N=n)$ are the same as in the corresponding $M / G I / \infty$ system (with the correct specification of the arrival rate and the service-time distribution).

  comment

Asymptotic Methods

• 4.II.31B

  Asymptotic Methods | Part II, 2007

  Consider the time-independent Schrödinger equation

  $$\frac{d^{2} \psi}{d x^{2}}+\lambda^{2} q(x) \psi(x)=0$$

  where $\lambda \gg 1$ denotes $\hbar^{-1}$ and $q(x)$ denotes $2 m[E-V(x)]$. Suppose that

  and consider a bound state $\psi(x)$. Write down the possible Liouville-Green approximate solutions for $\psi(x)$ in each region, given that $\psi \rightarrow 0$ as $|x| \rightarrow \infty$.

  Assume that $q(x)$ may be approximated by $q^{\prime}(a)(x-a)$ near $x=a$, where $q^{\prime}(a)>0$, and by $q^{\prime}(b)(x-b)$ near $x=b$, where $q^{\prime}(b)<0$. The Airy function $\operatorname{Ai}(z)$ satisfies

  $$\frac{d^{2}(\mathrm{Ai})}{d z^{2}}-z(\mathrm{Ai})=0$$

  and has the asymptotic expansions

  $$\operatorname{Ai}(z) \sim \frac{1}{2} \pi^{-1 / 2} z^{-1 / 4} \exp \left(-\frac{2}{3} z^{3 / 2}\right) \quad \text { as } \quad z \rightarrow+\infty$$

  and

  $$\operatorname{Ai}(z) \sim \pi^{-1 / 2}|z|^{-1 / 4} \cos \left[\left(\frac{2}{3}|z|^{3 / 2}\right)-\frac{\pi}{4}\right] \quad \text { as } \quad z \rightarrow-\infty .$$

  Deduce that the energies $E$ of bound states are given approximately by the WKB condition:

  $$\lambda \int_{a}^{b} q^{1 / 2}(x) d x=\left(n+\frac{1}{2}\right) \pi \quad(n=0,1,2, \ldots)$$

  $$\begin{aligned} & q(x)>0 \quad \text { for } \quad a<x<b \\ & \text { and } q(x)<0 \text { for }-\infty<x<a \text { and } b<x<\infty \end{aligned}$$

  comment

Classical Dynamics

• 4.I $9 \mathrm{C} \quad$

  Classical Dynamics | Part II, 2007

  (a) Show that the principal moments of inertia for the oblate spheroid of mass $M$ defined by

  $$\frac{\left(x_{1}^{2}+x_{2}^{2}\right)}{a^{2}}+\frac{x_{3}^{2}}{a^{2}\left(1-e^{2}\right)} \leqslant 1$$

  are given by $\left(I_{1}, I_{2}, I_{3}\right)=\frac{2}{5} M a^{2}\left(1-\frac{1}{2} e^{2}, 1-\frac{1}{2} e^{2}, 1\right)$. Here $a$ is the semi-major axis and $e$ is the eccentricity.

  [You may assume that a sphere of radius a has principal moments of inertia $\frac{2}{5} M a^{2}$.]

  (b) The spheroid in part (a) rotates about an axis that is not a principal axis. Euler's equations governing the angular velocity $\left(\omega_{1}, \omega_{2}, \omega_{3}\right)$ as viewed in the body frame are

  $$\begin{aligned} &I_{1} \frac{d \omega_{1}}{d t}=\left(I_{2}-I_{3}\right) \omega_{2} \omega_{3} \\ &I_{2} \frac{d \omega_{2}}{d t}=\left(I_{3}-I_{1}\right) \omega_{3} \omega_{1} \end{aligned}$$

  and

  $$I_{3} \frac{d \omega_{3}}{d t}=\left(I_{1}-I_{2}\right) \omega_{1} \omega_{2}$$

  Show that $\omega_{3}$ is constant. Show further that the angular momentum vector precesses around the $x_{3}$ axis with period

  $$P=\frac{2 \pi\left(2-e^{2}\right)}{e^{2} \omega_{3}}$$

  comment

• 4.II.15C

  Classical Dynamics | Part II, 2007

  The Hamiltonian for an oscillating particle with one degree of freedom is

  $$H=\frac{p^{2}}{2 m}+V(q, \lambda)$$

  The mass $m$ is a constant, and $\lambda$ is a function of time $t$ alone. Write down Hamilton's equations and use them to show that

  $$\frac{d H}{d t}=\frac{\partial H}{\partial \lambda} \frac{d \lambda}{d t}$$

  Now consider a case in which $\lambda$ is constant and the oscillation is exactly periodic. Denote the constant value of $H$ in that case by $E$. Consider the quantity $I=$ $(2 \pi)^{-1} \oint p d q$, where the integral is taken over a single oscillation cycle. For any given function $V(q, \lambda)$ show that $I$ can be expressed as a function of $E$ and $\lambda$ alone, namely

  $$I=I(E, \lambda)=\frac{(2 m)^{1 / 2}}{2 \pi} \oint(E-V(q, \lambda))^{1 / 2} d q$$

  where the sign of the integrand alternates between the two halves of the oscillation cycle. Let $\tau$ be the period of oscillation. Show that the function $I(E, \lambda)$ has partial derivatives

  $$\frac{\partial I}{\partial E}=\frac{\tau}{2 \pi} \quad \text { and } \quad \frac{\partial I}{\partial \lambda}=-\frac{1}{2 \pi} \oint \frac{\partial V}{\partial \lambda} d t$$

  You may assume without proof that $\partial / \partial E$ and $\partial / \partial \lambda$ may be taken inside the integral.

  Now let $\lambda$ change very slowly with time $t$, by a negligible amount during an oscillation cycle. Assuming that, to sufficient approximation,

  $$\frac{d\langle H\rangle}{d t}=\frac{\partial\langle H\rangle}{\partial \lambda} \frac{d \lambda}{d t}$$

  where $\langle H\rangle$ is the average value of $H$ over an oscillation cycle, and that

  $$\frac{d I}{d t}=\frac{\partial I}{\partial E} \frac{d\langle H\rangle}{d t}+\frac{\partial I}{\partial \lambda} \frac{d \lambda}{d t}$$

  deduce that $d I / d t=0$, carefully explaining your reasoning.

  When

  $$V(q, \lambda)=\lambda q^{2 n}$$

  with $n$ a positive integer and $\lambda$ positive, deduce that

  $$\langle H\rangle=C \lambda^{1 /(n+1)}$$

  for slowly-varying $\lambda$, where $C$ is a constant.

  [Do not try to solve Hamilton's equations. Rather, consider the form taken by $I$. ]

  comment

Coding and Cryptography

• 4.I.4G

  Coding and Cryptography | Part II, 2007

  What is a linear feedback shift register? Explain the Berlekamp-Massey method for recovering the feedback polynomial of a linear feedback shift register from its output. Illustrate in the case when we observe output

  $101011001000 \ldots \ldots$

  comment

Cosmology

• 4.I.10A

  Cosmology | Part II, 2007

  The equation governing density perturbation modes $\delta_{\mathbf{k}}(t)$ in a matter-dominated universe (with $a(t)=\left(t / t_{0}\right)^{2 / 3}$ ) is

  $$\ddot{\delta}_{\mathbf{k}}+2 \frac{\dot{a}}{a} \dot{\delta}_{\mathbf{k}}-\frac{3}{2}\left(\frac{\dot{a}}{a}\right)^{2} \delta_{\mathbf{k}}=0$$

  where $\mathbf{k}$ is the comoving wavevector. Find the general solution for the perturbation, showing that there is a growing mode such that

  $$\delta_{\mathbf{k}}(t) \approx \frac{a(t)}{a\left(t_{i}\right)} \delta_{\mathbf{k}}\left(t_{i}\right) \quad\left(t \gg t_{i}\right)$$

  Show that the physical wavelength corresponding to the comoving wavenumber $k=|\mathbf{k}|$ crosses the Hubble radius $c H^{-1}$ at a time $t_{k}$ given by

  $$\frac{t_{k}}{t_{0}}=\left(\frac{k_{0}}{k}\right)^{3} \quad, \quad \text { where } \quad k_{0}=\frac{2 \pi}{c H_{0}^{-1}}$$

  According to inflationary theory, the amplitude of the variance at horizon-crossing is constant, that is, $\left\langle\left|\delta_{\mathbf{k}}\left(t_{k}\right)\right|^{2}\right\rangle=A V^{-1} / k^{3}$ where $A$ and $V$ (the volume) are constants. Given this amplitude and the results obtained above, deduce that the power spectrum today takes the form

  $$P(k) \equiv V\left\langle\left|\delta_{\mathbf{k}}\left(t_{0}\right)\right|^{2}\right\rangle=\frac{A}{k_{0}^{4}} k$$

  comment

Differential Geometry

• 4.II.24H

  Differential Geometry | Part II, 2007

  (i) What is a geodesic? Show that geodesics are critical points of the energy functional.

  (ii) Let $S$ be a surface which admits a parametrization $\phi(u, v)$ defined on an open subset $W$ of $\mathbb{R}^{2}$ such that $E=G=U+V$ and $F=0$, where $U=U(u)$ is a function of $u$ alone and $V=V(v)$ is a function of $v$ alone. Let $\gamma: I \rightarrow \phi(W)$ be a geodesic and write $\gamma(t)=\phi(u(t), v(t))$. Show that

  $$[U(u(t))+V(v(t))]\left[V(v(t)) \dot{u}^{2}-U(u(t)) \dot{v}^{2}\right]$$

  is independent of $t$.

  comment

Dynamical Systems

• 4.I.7E

  Dynamical Systems | Part II, 2007

  By considering the binary representation of the sawtooth $\operatorname{map}, F(x)=2 x[\bmod 1]$ for $x \in[0,1)$, show that:

  (i) $F$ has sensitive dependence on initial conditions on $[0,1)$.

  (ii) $F$ has topological transitivity on $[0,1)$.

  (iii) Periodic points are dense in $[0,1)$.

  Find all the 4-cycles of $F$ and express them as fractions.

  comment

• 4.II.14E

  Dynamical Systems | Part II, 2007

  Consider the one-dimensional map $F: \mathbb{R} \rightarrow \mathbb{R}$ defined by

  $$x_{i+1}=F\left(x_{i}\right)=x_{i}\left(a x_{i}^{2}+b x_{i}+\mu\right)$$

  where $a$ and $b$ are constants, $\mu$ is a parameter and $a \neq 0$.

  (i) Find the fixed points of $F$ and determine the linear stability of $x=0$. Hence show that there are bifurcations at $\mu=1$, at $\mu=-1$ and, if $b \neq 0$, at $\mu=1+b^{2} / 4 a$.

  Sketch the bifurcation diagram for each of the cases:

  $$\text { (1) } a>b=0, \quad \text { (2) } a, b>0 \text { and (3) } a, b<0$$

  In each case show the locus and stability of the fixed points in the $(\mu, x)$-plane, and state the type of each bifurcation. [Assume that there are no further bifurcations in the region sketched.]

  (ii) For the case $F(x)=x\left(\mu-x^{2}\right)$ (i.e. $\left.a=-1, b=0\right)$, you may assume that

  $$F^{2}(x)=x+x\left(\mu-1-x^{2}\right)\left(\mu+1-x^{2}\right)\left(1-\mu x^{2}+x^{4}\right)$$

  Show that there are at most three 2-cycles and determine when they exist. By considering $F^{\prime}\left(x_{i}\right) F^{\prime}\left(x_{i+1}\right)$, or otherwise, show further that one 2-cycle is always unstable when it exists and that the others are unstable when $\mu>\sqrt{5}$. Sketch the bifurcation diagram showing the locus and stability of the fixed points and 2 -cycles. State briefly what you would expect to occur in the region $\mu>\sqrt{5}$.

  comment

Electrodynamics

• 4.II.35E

  Electrodynamics | Part II, 2007

  An action

  $$S[\varphi]=\int d^{4} x L(\varphi, \varphi, a)$$

  is given, where $\varphi(x)$ is a scalar field. Explain heuristically how to compute the functional derivative $\delta S / \delta \varphi$.

  Consider the action for electromagnetism,

  $$S\left[A_{a}\right]=-\int d^{4} x\left\{\frac{1}{4 \mu_{0}} F^{a b} F_{a b}+J^{a} A_{a}\right\} .$$

  Here $J^{a}$ is the 4-current density, $A_{a}$ is the 4-potential and $F_{a b}=A_{b, a}-A_{a, b}$ is the Maxwell field tensor. Obtain Maxwell's equations in 4-vector form.

  Another action that is sometimes suggested is

  $$\widehat{S}\left[A_{a}\right]=-\int d^{4} x\left\{\frac{1}{2 \mu_{0}} A^{a, b} A_{a, b}+J^{a} A_{a}\right\} .$$

  Under which additional assumption can Maxwell's equations be obtained using this action?

  Using this additional assumption establish the relationship between the actions $S$ and $\widehat{S}$.

  comment

Fluid Dynamics II

• 4.II.37B

  Fluid Dynamics II | Part II, 2007

  (i) Assuming that axisymmetric incompressible flow $\mathbf{u}=\left(u_{R}, u_{\theta}, 0\right)$, with vorticity $(0,0, \omega)$ in spherical polar coordinates $(R, \theta, \phi)$ satisfies the equations

  $$\mathbf{u}=\nabla \times\left(0,0, \frac{\Psi}{R \sin \theta}\right), \quad \omega=-\frac{1}{R \sin \theta} D^{2} \Psi$$

  where

  $$D^{2} \equiv \frac{\partial^{2}}{\partial R^{2}}+\frac{\sin \theta}{R^{2}} \frac{\partial}{\partial \theta}\left(\frac{1}{\sin \theta} \frac{\partial}{\partial \theta}\right)$$

  show that for Stokes flow $\Psi$ satisfies the equation

  $$D^{4} \Psi=0$$

  (ii) A rigid sphere of radius $a$ moves at velocity $U \hat{\mathbf{z}}$ through viscous fluid of density $\rho$ and dynamic viscosity $\mu$ which is at rest at infinity. Assuming Stokes flow and by applying the boundary conditions at $R=a$ and as $R \rightarrow \infty$, verify that $\Psi=(A R+B / R) \sin ^{2} \theta$ is the appropriate solution to $(*)$ for this flow, where $A$ and $B$ are to be determined.

  (iii) Hence find the velocity field outside the sphere. Without direct calculation, explain why the drag is in the $z$ direction and has magnitude proportional to $U$.

  (iv) A second identical sphere is introduced into the flow, at a distance $b \gg a$ from the first, and moving at the same velocity. Justify the assertion that, when the two spheres are at the same height, or when one is vertically above the other, the drag on each sphere is the same. Calculate the leading correction to the drag in each case, to leading order in $a / b$.

  [You may quote without proof the fact that, for an axisymmetric function $F(R, \theta)$,

  $$\nabla \times(0,0, F)=\left(\frac{1}{R \sin \theta} \frac{\partial}{\partial \theta}(\sin \theta F),-\frac{1}{R} \frac{\partial}{\partial R}(R F), 0\right)$$

  in spherical polar coordinates $(R, \theta, \phi)$.]

  comment

Further Complex Methods

• 4.I.8B

  Further Complex Methods | Part II, 2007

  The hypergeometric function $F(a, b ; c ; z)$ is defined by

  $$F(a, b ; c ; z)=K \int_{0}^{1} t^{b-1}(1-t)^{c-b-1}(1-t z)^{-a} d t$$

  where $|\arg (1-t z)|<\pi$ and $K$ is a constant determined by the condition $F(a, b ; c ; 0)=1$.

  (i) Express $K$ in terms of Gamma functions.

  (ii) By considering the $n$th derivative $F^{(n)}(a, b ; c ; 0)$, show that $F(a, b ; c ; z)=F(b, a ; c ; z)$.

  comment

Galois Theory

• 4.II.18F

  Galois Theory | Part II, 2007

  Let $f(x) \in K[x]$ be a monic polynomial, $L$ a splitting field for $f, \alpha_{1}, \ldots, \alpha_{n}$ the roots of $f$ in $L$. Let $\triangle(f)=\prod_{i<j}\left(\alpha_{i}-\alpha_{j}\right)^{2}$ be the discriminant of $f$. Explain why $\triangle(f)$ is a polynomial function in the coefficients of $f$, and determine $\triangle(f)$ when $f(x)=x^{3}+p x+q$.

  Compute the Galois group of the polynomial $x^{3}-3 x+1 \in \mathbb{Q}[x]$.

  comment

General Relativity

• 4.II.36A

  General Relativity | Part II, 2007

  Consider a particle on a trajectory $x^{a}(\lambda)$. Show that the geodesic equations, with affine parameter $\lambda$, coincide with the variational equations obtained by varying the integral

  $$I=\int_{\lambda_{0}}^{\lambda_{1}} g_{a b}(x) \frac{\mathrm{d} x^{a}}{\mathrm{~d} \lambda} \frac{\mathrm{d} x^{b}}{\mathrm{~d} \lambda} \mathrm{d} \lambda$$

  the end-points being fixed.

  In the case that $f(r)=1-2 G M u$, show that the space-time metric is given in the form

  $$\mathrm{d} s^{2}=-f(r) \mathrm{d} t^{2}+\frac{1}{f(r)} \mathrm{d} r^{2}+r^{2}\left(\mathrm{~d} \theta^{2}+\sin ^{2} \theta \mathrm{d} \phi^{2}\right)$$

  for a certain function $f(r)$. Assuming the particle motion takes place in the plane $\theta=\frac{\pi}{2}$ show that

  $$\frac{\mathrm{d} \phi}{\mathrm{d} \lambda}=\frac{h}{r^{2}}, \quad \frac{\mathrm{d} t}{\mathrm{~d} \lambda}=\frac{E}{f(r)},$$

  for $h, E$ constants. Writing $u=1 / r$, obtain the equation

  $$\left(\frac{\mathrm{d} u}{\mathrm{~d} \phi}\right)^{2}+f(r) u^{2}=-\frac{k}{h^{2}} f(r)+\frac{E^{2}}{h^{2}}$$

  where $k$ can be chosen to be 1 or 0 , according to whether the particle is massive or massless. In the case that $f(r)=1-G M u$, show that

  $$\frac{\mathrm{d}^{2} u}{\mathrm{~d} \phi^{2}}+u=k \frac{G M}{h^{2}}+3 G M u^{2}$$

  In the massive case, show that there is an approximate solution of the form

  $$u=\frac{1}{\ell}(1+e \cos (\alpha \phi)),$$

  where

  $$1-\alpha=\frac{3 G M}{\ell} .$$

  What is the interpretation of this solution?

  comment

Geometry of Group Actions

• 4.I $. 3 \mathrm{G}$

  Geometry of Group Actions | Part II, 2007

  Let $\Gamma$ be a circle on the Riemann sphere. Explain what it means to say that two points of the sphere are inverse points for the circle $\Gamma$. Show that, for each point $z$ on the Riemann sphere, there is a unique point $z^{\prime}$ with $z, z^{\prime}$ inverse points. Define inversion in $\Gamma$.

  Prove that the composition of an even number of inversions is a Möbius transformation.

  comment

• 4.II.12G

  Geometry of Group Actions | Part II, 2007

  Explain what it means to say that a group $G$ is a Kleinian group. What is the definition of the limit set for the group $G$ ? Prove that a fixed point of a parabolic element in $G$ must lie in the limit set.

  Show that the matrix $\left(\begin{array}{cc}1+a w & -a w^{2} \\ a & 1-a w\end{array}\right)$ represents a parabolic transformation for any non-zero choice of the complex numbers $a$ and $w$. Find its fixed point.

  The Gaussian integers are $\mathbb{Z}[i]=\{m+i n: m, n \in \mathbb{Z}\}$. Let $G$ be the set of Möbius transformations $z \mapsto \frac{a z+b}{c z+d}$ with $a, b, c, d \in \mathbb{Z}[i]$ and $a d-b c=1$. Prove that $G$ is a Kleinian group. For each point $w=\frac{p+i q}{r}$ with $p, q, r$ non-zero integers, find a parabolic transformation $T \in G$ that fixes $w$. Deduce that the limit set for $G$ is all of the Riemann sphere.

  comment

Graph Theory

• 4.II.17H

  Graph Theory | Part II, 2007

  Let $G$ be a graph with $n$ vertices and $m$ edges. Show that if $G$ contains no $C_{4}$, then $m \leqslant \frac{n}{4}(1+\sqrt{4 n-3})$.

  Let $C_{4}(G)$ denote the number of subgraphs of $G$ isomorphic to $C_{4}$. Show that if $m \geqslant \frac{n(n-1)}{4}$, then $G$ contains at least $\frac{n(n-1)(n-3)}{8}$ paths of length 2 . By considering the numbers $r_{1}, r_{2}, \ldots, r_{\left(\begin{array}{c}n \\ 2\end{array}\right)}$ of vertices joined to each pair of vertices of $G$, deduce that

  $$C_{4}(G) \geqslant \frac{1}{2}\left(\begin{array}{l} n \\ 2 \end{array}\right)\left(\begin{array}{c} (n-3) / 4 \\ 2 \end{array}\right)$$

  Now let $G=G(n, 1 / 2)$ be the random graph on $\{1,2, \ldots, n\}$ in which each pair of vertices is joined independently with probability $1 / 2$. Find the expectation $\mathbb{E}\left(C_{4}(G)\right)$ of $C_{4}(G)$. Deduce that if $0<\epsilon<1 / 2$, then

  $$\operatorname{Pr}\left(C_{4}(G) \leqslant(1+2 \epsilon) \frac{3}{16}\left(\begin{array}{l} n \\ 4 \end{array}\right)\right) \geqslant \epsilon$$

  comment

Linear Analysis

• 4.II.22G

  Linear Analysis | Part II, 2007

  Let $X$ be a Banach space and $T: X \rightarrow X$ a bounded linear map. Define the spectrum $\sigma(T)$, point spectrum $\sigma_{p}(T)$, resolvent $R_{T}(\lambda)$, and resolvent set $\rho(T)$. Show that the spectrum is a closed and bounded subset of $\mathbb{C}$. Is the point spectrum always closed? Justify your answer.

  Now suppose $H$ is a Hilbert space, and $T: H \rightarrow H$ is self-adjoint. Show that the point spectrum $\sigma_{p}(T)$ is real.

  comment

Mathematical Biology

• 4.I.6B

  Mathematical Biology | Part II, 2007

  The non-dimensional equations for two competing populations are

  $$\begin{aligned} &\frac{d u}{d t}=u(1-v)-\epsilon_{1} u^{2} \\ &\frac{d v}{d t}=\alpha\left[v(1-u)-\epsilon_{2} v^{2}\right] \end{aligned}$$

  Explain the meaning of each term in these equations.

  Find all the fixed points of this system when $\alpha>0,0<\epsilon_{1}<1$ and $0<\epsilon_{2}<1$, and investigate their stability.

  comment

Number Fields

• 4.II.20H

  Number Fields | Part II, 2007

  Let $K$ be a finite extension of $\mathbb{Q}$ and let $\mathcal{O}=\mathcal{O}_{K}$ be its ring of integers. We will assume that $\mathcal{O}=\mathbb{Z}[\theta]$ for some $\theta \in \mathcal{O}$. The minimal polynomial of $\theta$ will be denoted by $g$. For a prime number $p$ let

  $$\bar{g}(X)=\bar{g}_{1}(X)^{e_{1}} \cdot \ldots \cdot \bar{g}_{r}(X)^{e_{r}}$$

  be the decomposition of $\bar{g}(X)=g(X)+p \mathbb{Z}[X] \in(\mathbb{Z} / p \mathbb{Z})[X]$ into distinct irreducible monic factors $\bar{g}_{i}(X) \in(\mathbb{Z} / p \mathbb{Z})[X]$. Let $g_{i}(X) \in \mathbb{Z}[X]$ be a polynomial whose reduction modulo $p$ is $\bar{g}_{i}(X)$. Show that

  $$\mathfrak{p}_{i}=\left[p, g_{i}(\theta)\right], \quad i=1, \ldots, r,$$

  are the prime ideals of $\mathcal{O}$ containing $p$, that these are pairwise different, and

  $$[p]=\mathfrak{p}_{1}^{e_{1}} \cdot \ldots \cdot \mathfrak{p}_{r}^{e_{r}}$$

  comment

Number Theory

• 4.I.1F

  Number Theory | Part II, 2007

  Prove Legendre's formula relating $\pi(x)$ and $\pi(\sqrt{x})$ for any positive real number $x$. Use this formula to compute $\pi(48)$.

  comment

• 4.II.11F

  Number Theory | Part II, 2007

  Let $p$ be a prime number, and let $f(x)$ be a polynomial with integer coefficients, whose leading coefficient is not divisible by $p$. Prove that the congruence

  $$f(x) \equiv 0 \quad(\bmod p)$$

  has at most $d$ solutions, where $d$ is the degree of $f(x)$.

  Deduce that all coefficients of the polynomial

  $$x^{p-1}-1-((x-1)(x-2) \cdots(x-p+1))$$

  must be divisible by $p$, and prove that:

  (i) $(p-1) !+1 \equiv 0 \quad(\bmod p)$;

  (ii) if $p$ is odd, the numerator of the fraction

  $$u_{p}=1+\frac{1}{2}+\cdots+\frac{1}{p-1}$$

  is divisible by $p$.

  Assume now that $p \geqslant 5$. Show by example that (i) cannot be strengthened to $(p-1) !+1 \equiv 0 \quad\left(\bmod p^{2}\right)$.

  comment

Numerical Analysis

• 4.II.39C

  Numerical Analysis | Part II, 2007

  (a) Suppose that $A$ is a real $n \times n$ matrix, and that $w \in \mathbb{R}^{n}$ and $\lambda_{1} \in \mathbb{R}$ are given so that $A w=\lambda_{1} w$. Further, let $S$ be a non-singular matrix such that $S w=c e^{(1)}$, where $e^{(1)}$ is the first coordinate vector and $c \neq 0$. Let $\widehat{A}=S A S^{-1}$. Prove that the eigenvalues of $A$ are $\lambda_{1}$ together with the eigenvalues of the bottom right $(n-1) \times(n-1)$ submatrix of $\widehat{A} .$

  (b) Suppose again that $A$ is a real $n \times n$ matrix, and that two linearly independent vectors $v, w \in \mathbb{R}^{n}$ are given such that the linear subspace $L\{v, w\}$ spanned by $v$ and $w$ is invariant under the action of $A$, i.e.,

  $$x \in L\{v, w\} \quad \Rightarrow \quad A x \in L\{v, w\}$$

  Denote by $V$ an $n \times 2$ matrix whose two columns are the vectors $v$ and $w$, and let $S$ be a non-singular matrix such that $R=S V$ is upper triangular, that is,

  $$R=S V=S \times\left[\begin{array}{cc} v_{1} & w_{1} \\ v_{2} & w_{2} \\ v_{3} & w_{3} \\ : & : \\ v_{n} & w_{n} \end{array}\right]=\left[\begin{array}{cc} r_{11} & r_{12} \\ 0 & r_{22} \\ 0 & 0 \\ : & : \\ 0 & 0 \end{array}\right]$$

  Again let $\widehat{A}=S A S^{-1}$. Prove that the eigenvalues of $A$ are the eigenvalues of the top left $2 \times 2$ submatrix of $\widehat{A}$together with the eigenvalues of the bottom right $(n-2) \times(n-2)$ submatrix of $\widehat{A}$

  comment

Optimization and Control

• 4.II.29I

  Optimization and Control | Part II, 2007

  Consider the scalar controllable linear system, whose state $X_{n}$ evolves by

  $$X_{n+1}=X_{n}+U_{n}+\varepsilon_{n+1}$$

  with observations $Y_{n}$ given by

  $$Y_{n+1}=X_{n}+\eta_{n+1}$$

  Here, $U_{n}$ is the control variable, which is to be determined on the basis of the observations up to time $n$, and $\varepsilon_{n}, \eta_{n}$ are independent $N(0,1)$ random variables. You wish to minimize the long-run average expected cost, where the instantaneous cost at time $n$ is $X_{n}^{2}+U_{n}^{2}$. You may assume that the optimal control in equilibrium has the form $U_{n}=-K \hat{X}_{n}$, where $\hat{X}_{n}$ is given by a recursion of the form

  $$\hat{X}_{n+1}=\hat{X}_{n}+U_{n}+H\left(Y_{n+1}-\hat{X}_{n}\right)$$

  and where $H$ is chosen so that $\Delta_{n}=X_{n}-\hat{X}_{n}$ is independent of the observations up to time $n$. Show that $K=H=(\sqrt{5}-1) / 2=2 /(\sqrt{5}+1)$, and determine the minimal long-run average expected cost. You are not expected to simplify the arithmetic form of your answer but should show clearly how you have obtained it.

  comment

Partial Differential Equations

• 4.II.30A

  Partial Differential Equations | Part II, 2007

  State and prove the mean value property for harmonic functions on $\mathbb{R}^{3}$.

  Obtain a generalization of the mean value property for sub-harmonic functions on $\mathbb{R}^{3}$, i.e. $C^{2}$ functions for which

  $$-\Delta u(x) \leqslant 0$$

  for all $x \in \mathbb{R}^{3}$.

  Let $\phi \in C^{2}\left(\mathbb{R}^{3} ; \mathbb{C}\right)$ solve the equation

  $$-\Delta \phi+i V(x) \phi=0$$

  where $V$ is a real-valued continuous function. By considering the function $w(x)=|\phi(x)|^{2}$ show that, on any ball $B(y, R)=\{x:\|x-y\|<R\} \subset \mathbb{R}^{3}$,

  $$\sup _{x \in B(y, R)}|\phi(x)| \leqslant \sup _{\|x-y\|=R}|\phi(x)| .$$

  comment

Principles of Quantum Mechanics

• 4.II.32D

  Principles of Quantum Mechanics | Part II, 2007

  The Hamiltonian for a particle of spin $\frac{1}{2}$ in a magnetic field $\mathbf{B}$ is

  $$H=-\frac{1}{2} \hbar \gamma \mathbf{B} \cdot \boldsymbol{\sigma} \quad \text { where } \quad \sigma_{x}=\left(\begin{array}{cc} 0 & 1 \\ 1 & 0 \end{array}\right), \quad \sigma_{y}=\left(\begin{array}{cc} 0 & -i \\ i & 0 \end{array}\right), \quad \sigma_{z}=\left(\begin{array}{cc} 1 & 0 \\ 0 & -1 \end{array}\right)$$

  and $\gamma$ is a constant (the motion of the particle in space can be ignored). Consider a magnetic field which is independent of time. Writing $\mathbf{B}=B \mathbf{n}$, where $\mathbf{n}$ is a unit vector, calculate the time evolution operator and show that if the particle is initially in a state $|\chi\rangle$ the probability of measuring it to be in an orthogonal state $\left|\chi^{\prime}\right\rangle$ after a time $t$ is

  $$\left|\left\langle\chi^{\prime}|\mathbf{n} \cdot \boldsymbol{\sigma}| \chi\right\rangle\right|^{2} \sin ^{2} \frac{\gamma B t}{2} .$$

  Evaluate this to find the probability for a transition from a state of spin up along the $z$ direction to one of spin down along the $z$ direction when $\mathbf{B}=\left(B_{x}, 0, B_{z}\right)$.

  Now consider a magnetic field whose $x$ and $y$ components are time-dependent but small:

  $$\mathbf{B}=\left(A \cos \alpha t, A \sin \alpha t, B_{z}\right) .$$

  Show that the probability for a transition from a spin-up state at time zero to a spin-down state at time $t$ (with spin measured along the $z$ direction, as before) is approximately

  $$\left(\frac{\gamma A}{\gamma B_{z}+\alpha}\right)^{2} \sin ^{2} \frac{\left(\gamma B_{z}+\alpha\right) t}{2}$$

  where you may assume $|A| \ll\left|B_{z}+\alpha \gamma^{-1}\right|$. Comment on how this compares, when $\alpha=0$, with the result for a time-independent field.

  [The first-order transition amplitude due to a perturbation $V(t)$ is

  $$-\frac{i}{\hbar} \int_{0}^{t} d t^{\prime} e^{i\left(E^{\prime}-E\right) t^{\prime} / \hbar}\left\langle\chi^{\prime}\left|V\left(t^{\prime}\right)\right| \chi\right\rangle$$

  where $|\chi\rangle$ and $\left|\chi^{\prime}\right\rangle$ are orthogonal eigenstates of the unperturbed Hamiltonian with eigenvalues $E$ and $E^{\prime}$ respectively.]

  comment

Principles of Statistics

• 4.II.27I

  Principles of Statistics | Part II, 2007

  Assuming sufficient regularity conditions on the likelihood $f(x \mid \theta)$ for a univariate parameter $\theta \in \Theta$, establish the Cramér-Rao lower bound for the variance of an unbiased estimator of $\theta$.

  If $\hat{\theta}(X)$ is an unbiased estimator of $\theta$ whose variance attains the Cramér-Rao lower bound for every value of $\theta \in \Theta$, show that the likelihood function is an exponential family.

  comment

Probability and Measure

• 4.II.25J

  Probability and Measure | Part II, 2007

  Let $(E, \mathcal{E}, \mu)$ be a measure space with $\mu(E)<\infty$ and let $\theta: E \rightarrow E$ be measurable.

  (a) Define an invariant set $A \in \mathcal{E}$ and an invariant function $f: E \rightarrow \mathbb{R}$.

  What is meant by saying that $\theta$ is measure-preserving?

  What is meant by saying that $\theta$ is ergodic?

  (b) Which of the following functions $\theta_{1}$ to $\theta_{4}$ is ergodic? Justify your answer.

  On the measure space $([0,1], \mathcal{B}([0,1]), \mu)$ with Lebesgue measure $\mu$ consider

  $$\theta_{1}(x)=1+x, \quad \theta_{2}(x)=x^{2}, \quad \theta_{3}(x)=1-x$$

  On the discrete measure space $\left(\{-1,1\}, \mathcal{P}(\{-1,1\}), \frac{1}{2} \delta_{-1}+\frac{1}{2} \delta_{1}\right)$ consider

  $$\theta_{4}(x)=-x$$

  (c) State Birkhoff's almost everywhere ergodic theorem.

  (d) Let $\theta$ be measure-preserving and let $f: E \rightarrow \mathbb{R}$ be bounded.

  Prove that $\frac{1}{n}\left(f+f \circ \theta+\ldots+f \circ \theta^{n-1}\right)$ converges in $L^{p}$ for all $p \in[1, \infty)$.

  comment

Representation Theory

• 4.II.19H

  Representation Theory | Part II, 2007

  Write an essay on the representation theory of $\mathrm{SU}_{2}$.

  Your answer should include a description of each irreducible representation and an explanation of how to decompose arbitrary representations into a direct sum of these.

  comment

Riemann Surfaces

• 4.II.23F

  Riemann Surfaces | Part II, 2007

  Let $R$ be a Riemann surface, $\widetilde{R}$a topological surface, and $p: \widetilde{R} \rightarrow R$ a continuous map. Suppose that every point $x \in \widetilde{R}$admits a neighbourhood $\widetilde{U}$such that $p$ maps $\widetilde{U}$homeomorphically onto its image. Prove that $\widetilde{R}$has a complex structure such that $p$ is a holomorphic map.

  A holomorphic map $\pi: Y \rightarrow X$ between Riemann surfaces is called a covering map if every $x \in X$ has a neighbourhood $V$ with $\pi^{-1}(V)$ a disjoint union of open sets $W_{k}$ in $Y$, so that $\pi: W_{k} \rightarrow V$ is biholomorphic for each $W_{k}$. Suppose that a Riemann surface $Y$ admits a holomorphic covering map from the unit $\operatorname{disc}\{z \in \mathbb{C}:|z|<1\}$. Prove that any holomorphic map $\mathbb{C} \rightarrow Y$ is constant.

  [You may assume any form of the monodromy theorem and basic results about the lifts of paths, provided that these are accurately stated.]

  comment

Set Theory and Logic

• 4.II.16G

  Set Theory and Logic | Part II, 2007

  Explain what is meant by a well-founded binary relation on a set.

  Given a set $a$, we say that a mapping $f: a \rightarrow \mathcal{P} a$ is recursive if, given any set $b$ equipped with a mapping $g: \mathcal{P} b \rightarrow b$, there exists a unique $h: a \rightarrow b$ such that $h=g \circ h_{*} \circ f$, where $h_{*}: \mathcal{P} a \rightarrow \mathcal{P} b$ denotes the mapping $a^{\prime} \mapsto\left\{h(x) \mid x \in a^{\prime}\right\}$. Show that $f$ is recursive if and only if the relation $\{\langle x, y\rangle \mid x \in f(y)\}$ is well-founded.

  [If you need to use any form of the recursion theorem, you should prove it.]

  comment

Statistical Modelling

• 4.I.5I

  Statistical Modelling | Part II, 2007

  Consider the normal linear model $Y=X \beta+\varepsilon$ in vector notation, where

  $Y=\left(\begin{array}{c}Y_{1} \\ \vdots \\ Y_{n}\end{array}\right), \quad X=\left(\begin{array}{c}x_{1}^{\mathrm{T}} \\ \vdots \\ x_{n}^{\mathrm{T}}\end{array}\right), \quad \beta=\left(\begin{array}{c}\beta_{1} \\ \vdots \\ \beta_{p}\end{array}\right), \quad \varepsilon=\left(\begin{array}{c}\varepsilon_{1} \\ \vdots \\ \varepsilon_{n}\end{array}\right), \quad \varepsilon_{i} \sim$ i.i.d. $N\left(0, \sigma^{2}\right)$,

  where $x_{i}^{\mathrm{T}}=\left(x_{i 1}, \ldots, x_{i p}\right)$ is known and $X$ is of full rank $(p<n)$. Give expressions for maximum likelihood estimators $\hat{\beta}$ and $\hat{\sigma}^{2}$ of $\beta$ and $\sigma^{2}$ respectively, and state their joint distribution.

  Suppose that there is a new pair $\left(x^{*}, y^{*}\right)$, independent of $\left(x_{1}, y_{1}\right), \ldots,\left(x_{n}, y_{n}\right)$, satisfying the relationship

  $$y^{*}=x^{* \mathrm{~T}} \beta+\varepsilon^{*}, \quad \text { where } \quad \varepsilon^{*} \sim N\left(0, \sigma^{2}\right) .$$

  We suppose that $x^{*}$ is known, and estimate $y^{*}$ by $\tilde{y}=x^{* \mathrm{~T}} \hat{\beta}$. State the distribution of

  $$\frac{\tilde{y}-y^{*}}{\tilde{\sigma} \tau}, \quad \text { where } \quad \tilde{\sigma}^{2}=\frac{n}{n-p} \hat{\sigma}^{2} \quad \text { and } \quad \tau^{2}=x^{* \mathrm{~T}}\left(X^{\mathrm{T}} X\right)^{-1} x^{*}+1$$

  Find the form of a $(1-\alpha)$-level prediction interval for $y^{*}$.

  comment

• 4.II.13I

  Statistical Modelling | Part II, 2007

  Let $Y$ have a Gamma distribution with density

  $$f(y ; \alpha, \lambda)=\frac{\lambda^{\alpha} y^{\alpha-1}}{\Gamma(\alpha)} e^{-\lambda y}$$

  Show that the Gamma distribution is of exponential dispersion family form. Deduce directly the corresponding expressions for $\mathbb{E}[Y]$ and $\operatorname{Var}[Y]$ in terms of $\alpha$ and $\lambda$. What is the canonical link function?

  Let $p<n$. Consider a generalised linear model (g.l.m.) for responses $y_{i}, i=1, \ldots, n$ with random component defined by the Gamma distribution with canonical link $g(\mu)$, so that $g\left(\mu_{i}\right)=\eta_{i}=x_{i}^{\mathrm{T}} \beta$, where $\beta=\left(\beta_{1}, \ldots, \beta_{p}\right)^{\mathrm{T}}$ is the vector of unknown regression coefficients and $x_{i}=\left(x_{i 1}, \ldots, x_{i p}\right)^{\mathrm{T}}$ is the vector of known values of the explanatory variables for the $i$ th observation, $i=1, \ldots, n$.

  Obtain expressions for the score function and Fisher information matrix and explain how these can be used in order to approximate $\hat{\beta}$, the maximum likelihood estimator (m.l.e.) of $\beta$.

  [Use the canonical link function and assume that the dispersion parameter is known.]

  Finally, obtain an expression for the deviance for a comparison of the full (saturated) model to the g.l.m. with canonical link using the m.l.e. $\hat{\beta}$ (or estimated mean $\hat{\mu}=X \hat{\beta})$.

  comment

Statistical Physics

• 4.II.34D

  Statistical Physics | Part II, 2007

  Consider a classical gas of diatomic molecules whose orientation is fixed by a strong magnetic field. The molecules are not free to rotate, but they are free to vibrate. Assuming that the vibrations are approximately harmonic, calculate the contribution to the partition function due to vibrations.

  Evaluate the free energy $F=-k T \ln Z$, where $Z$ is the total partition function for the gas, and hence calculate the entropy.

  $\left[\right.$ Note that $\int_{-\infty}^{\infty} \exp \left(-a u^{2}\right) d u=\sqrt{\pi / a}$ and $\int_{0}^{\infty} u^{2} \exp \left(-a u^{2}\right) d u=\sqrt{\pi} / 4 a^{3 / 2} . \quad$ You may approximate $\ln N$ ! by $N \ln N-N$.]

  comment

Stochastic Financial Models

• 4.II.28J

  Stochastic Financial Models | Part II, 2007

  Briefly describe the Black-Scholes model. Consider a "cash-or-nothing" option with strike price $K$, i.e. an option whose payoff at maturity is

  $$f\left(S_{T}\right)= \begin{cases}1 & \text { if } \quad S_{T}>K \\ 0 & \text { if } \quad S_{T} \leqslant K\end{cases}$$

  It can be interpreted as a bet that the stock will be worth at least $K$ at time $T$. Find a formula for its value at time $t$, in terms of the spot price $S_{t}$. Find a formula for its Delta (i.e. its hedge ratio). How does the Delta behave as $t \rightarrow T$ ? Why is it difficult, in practice, to hedge such an instrument?

  comment

Topics in Analysis

• 4.I.2F

  Topics in Analysis | Part II, 2007

  State Brouwer's fixed point theorem for a triangle in two dimensions.

  Let $A=\left(a_{i j}\right)$ be a $3 \times 3$ matrix with real positive entries and such that all its columns are non-zero vectors. Show that $A$ has an eigenvector with positive entries.

  comment

Waves

• 4.II $. 38 \mathrm{C} \quad$

  Waves | Part II, 2007

  Show that, for a plane acoustic wave, the acoustic intensity $\tilde{p} \mathbf{u}$ may be written as $\rho_{0} c_{0}|\mathbf{u}|^{2} \hat{\mathbf{k}}$ in the standard notation.

  Derive the general spherically-symmetric solution of the wave equation. Use it to find the velocity potential $\phi(r, t)$ for waves radiated into an unbounded fluid by a pulsating sphere of radius

  $$a\left(1+\varepsilon e^{i \omega t}\right) \quad(\varepsilon \ll 1)$$

  By considering the far field, or otherwise, find the time-average rate at which energy is radiated by the sphere.

  $\left[\right.$ You may assume that $\nabla^{2} \phi=\frac{1}{r^{2}} \frac{\partial}{\partial r}\left(r^{2} \frac{\partial \phi}{\partial r}\right)$.]

  comment