Part II, 2012

Algebraic Geometry

• Paper 1, Section II, I

  Algebraic Geometry | Part II, 2012

  (a) Let $X$ be an affine variety, $k[X]$ its ring of functions, and let $p \in X$. Assume $k$ is algebraically closed. Define the tangent space $T_{p} X$ at $p$. Prove the following assertions.

  (i) A morphism of affine varieties $f: X \rightarrow Y$ induces a linear map

  $$d f: T_{p} X \rightarrow T_{f(p)} Y$$

  (ii) If $g \in k[X]$ and $U:=\{x \in X \mid g(x) \neq 0\}$, then $U$ has the natural structure of an affine variety, and the natural morphism of $U$ into $X$ induces an isomorphism $T_{p} U \rightarrow T_{p} X$ for all $p \in U$.

  (iii) For all $s \geqslant 0$, the subset $\left\{x \in X \mid \operatorname{dim} T_{x} X \geqslant s\right\}$ is a Zariski-closed subvariety of $X$.

  (b) Show that the set of nilpotent $2 \times 2$ matrices

  $$X=\left\{x \in \operatorname{Mat}_{2}(k) \mid x^{2}=0\right\}$$

  may be realised as an affine surface in $\mathbf{A}^{3}$, and determine its tangent space at all points $x \in X$.

  Define what it means for two varieties $Y_{1}$ and $Y_{2}$ to be birationally equivalent, and show that the variety $X$ of nilpotent $2 \times 2$ matrices is birationally equivalent to $\mathbf{A}^{2}$.

  comment

• Paper 2, Section II, I

  Algebraic Geometry | Part II, 2012

  Let $k$ be a field, $J$ an ideal of $k\left[x_{1}, \ldots, x_{n}\right]$, and let $R=k\left[x_{1}, \ldots, x_{n}\right] / J$. Define the radical $\sqrt{J}$ of $J$ and show that it is also an ideal.

  The Nullstellensatz says that if $J$ is a maximal ideal, then the inclusion $k \subseteq R$ is an algebraic extension of fields. Suppose from now on that $k$ is algebraically closed. Assuming the above statement of the Nullstellensatz, prove the following.

  (i) If $J$ is a maximal ideal, then $J=\left(x_{1}-a_{1}, \ldots, x_{n}-a_{n}\right)$, for some $\left(a_{1}, \ldots, a_{n}\right) \in k^{n}$.

  (ii) If $J \neq k\left[x_{1}, \ldots, x_{n}\right]$, then $Z(J) \neq \emptyset$, where

  $$Z(J)=\left\{a \in k^{n} \mid f(a)=0 \text { for all } f \in J\right\}$$

  (iii) For $V$ an affine subvariety of $k^{n}$, we set

  $$I(V)=\left\{f \in k\left[x_{1}, \ldots, x_{n}\right] \mid f(a)=0 \text { for all } a \in V\right\}$$

  Prove that $J=I(V)$ for some affine subvariety $V \subseteq k^{n}$, if and only if $J=\sqrt{J}$.

  [Hint. Given $f \in J$, you may wish to consider the ideal in $k\left[x_{1}, \ldots, x_{n}, y\right]$ generated $b y J$ and $y f-1$.]

  (iv) If $A$ is a finitely generated algebra over $k$, and $A$ does not contain nilpotent elements, then there is an affine variety $V \subseteq k^{n}$, for some $n$, with $A=k\left[x_{1}, \ldots, x_{n}\right] / I(V)$.

  Assuming $\operatorname{char}(k) \neq 2$, find $\sqrt{J}$ when $J$ is the ideal $\left(x(x-y)^{2}, y(x+y)^{2}\right)$ in $k[x, y]$.

  comment

• Paper 3, Section II, I

  Algebraic Geometry | Part II, 2012

  Let $X \subset \mathbf{P}^{2}(\mathbf{C})$ be the projective closure of the affine curve $y^{3}=x^{4}+1$. Let $\omega$ denote the differential $d x / y^{2}$. Show that $X$ is smooth, and compute $v_{p}(\omega)$ for all $p \in X$.

  Calculate the genus of $X$.

  comment

• Paper 4, Section II, I

  Algebraic Geometry | Part II, 2012

  Let $X$ be a smooth projective curve of genus 2, defined over the complex numbers. Show that there is a morphism $f: X \rightarrow \mathbf{P}^{1}$ which is a double cover, ramified at six points.

  Explain briefly why $X$ cannot be embedded into $\mathbf{P}^{2}$.

  For any positive integer $n$, show that there is a smooth affine plane curve which is a double cover of $\mathbf{A}^{1}$ ramified at $n$ points.

  [State clearly any theorems that you use.]

  comment

Algebraic Topology

• Paper 1, Section II, G

  Algebraic Topology | Part II, 2012

  Define the notions of covering projection and of locally path-connected space. Show that a locally path-connected space is path-connected if it is connected.

  Suppose $f: Y \rightarrow X$ and $g: Z \rightarrow X$ are continuous maps, the space $Y$ is connected and locally path-connected and that $g$ is a covering projection. Suppose also that we are given base-points $x_{0}, y_{0}, z_{0}$ satisfying $f\left(y_{0}\right)=x_{0}=g\left(z_{0}\right)$. Show that there is a continuous $\tilde{f}: Y \rightarrow Z$ satisfying $\tilde{f}\left(y_{0}\right)=z_{0}$ and $g \tilde{f}=f$ if and only if the image of $f_{*}: \Pi_{1}\left(Y, y_{0}\right) \rightarrow \Pi_{1}\left(X, x_{0}\right)$ is contained in that of $g_{*}: \Pi_{1}\left(Z, z_{0}\right) \rightarrow \Pi_{1}\left(X, x_{0}\right)$. [You may assume the path-lifting and homotopy-lifting properties of covering projections.]

  Now suppose $X$ is locally path-connected, and both $f: Y \rightarrow X$ and $g: Z \rightarrow X$ are covering projections with connected domains. Show that $Y$ and $Z$ are homeomorphic as spaces over $X$ if and only if the images of their fundamental groups under $f_{*}$ and $g_{*}$ are conjugate subgroups of $\Pi_{1}\left(X, x_{0}\right)$.

  comment

• Paper 2, Section II, G

  Algebraic Topology | Part II, 2012

  State the Seifert-Van Kampen Theorem. Deduce that if $f: S^{1} \rightarrow X$ is a continuous map, where $X$ is path-connected, and $Y=X \cup_{f} B^{2}$ is the space obtained by adjoining a disc to $X$ via $f$, then $\Pi_{1}(Y)$ is isomorphic to the quotient of $\Pi_{1}(X)$ by the smallest normal subgroup containing the image of $f_{*}: \Pi_{1}\left(S^{1}\right) \rightarrow \Pi_{1}(X)$.

  State the classification theorem for connected triangulable 2-manifolds. Use the result of the previous paragraph to obtain a presentation of $\Pi_{1}\left(M_{g}\right)$, where $M_{g}$ denotes the compact orientable 2 -manifold of genus $g>0$.

  comment

• Paper 3, Section II, G

  Algebraic Topology | Part II, 2012

  State the Mayer-Vietoris Theorem for a simplicial complex $K$ expressed as the union of two subcomplexes $L$ and $M$. Explain briefly how the connecting homomorphism $\delta_{*}: H_{n}(K) \rightarrow H_{n-1}(L \cap M)$, which appears in the theorem, is defined. [You should include a proof that $\delta_{*}$ is well-defined, but need not verify that it is a homomorphism.]

  Now suppose that $|K| \cong S^{3}$, that $|L|$ is a solid torus $S^{1} \times B^{2}$, and that $|L \cap M|$ is the boundary torus of $|L|$. Show that $\delta_{*}: H_{3}(K) \rightarrow H_{2}(L \cap M)$ is an isomorphism, and hence calculate the homology groups of $M$. [You may assume that a generator of $H_{3}(K)$ may be represented by a 3 -cycle which is the sum of all the 3 -simplices of $K$, with 'matching' orientations.]

  comment

• Paper 4, Section II, G

  Algebraic Topology | Part II, 2012

  State and prove the Lefschetz fixed-point theorem. Hence show that the $n$-sphere $S^{n}$ does not admit a topological group structure for any even $n>0$. [The existence and basic properties of simplicial homology with rational coefficients may be assumed.]

  comment

Applications of Quantum Mechanics

• Paper 1, Section II, E

  Applications of Quantum Mechanics | Part II, 2012

  Give an account of the variational principle for establishing an upper bound on the ground-state energy $E_{0}$ of a particle moving in a potential $V(x)$ in one dimension.

  A particle of unit mass moves in the potential

  $$V(x)= \begin{cases}\infty & x \leqslant 0 \\ \lambda x & x>0\end{cases}$$

  with $\lambda$ a positive constant. Explain why it is important that any trial wavefunction used to derive an upper bound on $E_{0}$ should be chosen to vanish for $x \leqslant 0$.

  Use the trial wavefunction

  $$\psi(x)= \begin{cases}0 & x \leqslant 0 \\ x e^{-a x} & x>0\end{cases}$$

  where $a$ is a positive real parameter, to establish an upper bound $E_{0} \leqslant E(a, \lambda)$ for the energy of the ground state, and hence derive the lowest upper bound on $E_{0}$ as a function of $\lambda$.

  Explain why the variational method cannot be used in this case to derive an upper bound for the energy of the first excited state.

  comment

• Paper 2, Section II, E

  Applications of Quantum Mechanics | Part II, 2012

  A solution of the $S$-wave Schrödinger equation at large distances for a particle of mass $m$ with momentum $\hbar k$ and energy $E=\hbar^{2} k^{2} / 2 m$, has the form

  $$\psi_{0}(\boldsymbol{r}) \sim \frac{A}{r}[\sin k r+g(k) \cos k r] .$$

  Define the phase shift $\delta_{0}$ and verify that $\tan \delta_{0}(k)=g(k)$.

  Write down a formula for the cross-section $\sigma$, for a particle of momentum $\hbar k$ scattering on a radially symmetric potential of finite range, as a function of the phase shifts $\delta_{l}$ for the partial waves with quantum number $l$.

  (i) Suppose that $g(k)=-k / K$ for $K>0$. Show that there is a bound state of energy $E_{B}=-\hbar^{2} K^{2} / 2 m$. Neglecting the contribution from partial waves with $l>0$ show that the cross section is

  $$\sigma=\frac{4 \pi}{K^{2}+k^{2}} .$$

  (ii) Suppose now that $g(k)=\gamma /\left(K_{0}-k\right)$ with $K_{0}>0, \gamma>0$ and $\gamma \ll K_{0}$. Neglecting the contribution from partial waves with $l>0$, derive an expression for the cross section $\sigma$, and show that it has a local maximum when $E \approx \hbar^{2} K_{0}^{2} / 2 \mathrm{~m}$. Discuss the interpretation of this phenomenon in terms of resonant behaviour and derive an expression for the decay width of the resonant state.

  comment

• Paper 3, Section II, E

  Applications of Quantum Mechanics | Part II, 2012

  A simple model of a crystal consists of a 1D linear array of sites at positions $x=n a$, for all integer $n$ and separation $a$, each occupied by a similar atom. The potential due to the atom at the origin is $U(x)$, which is symmetric: $U(-x)=U(x)$. The Hamiltonian, $H_{0}$, for the atom at the $n$-th site in isolation has electron eigenfunction $\psi_{n}(x)$ with energy $E_{0}$. Write down $H_{0}$ and state the relationship between $\psi_{n}(x)$ and $\psi_{0}(x)$.

  The Hamiltonian $H$ for an electron moving in the crystal is $H=H_{0}+V(x)$. Give an expression for $V(x)$.

  In the tight-binding approximation for this model the $\psi_{n}$ are assumed to be orthonormal, $\left(\psi_{n}, \psi_{m}\right)=\delta_{n m}$, and the only non-zero matrix elements of $H_{0}$ and $V$ are

  $$\left(\psi_{n}, H_{0} \psi_{n}\right)=E_{0}, \quad\left(\psi_{n}, V \psi_{n}\right)=\alpha, \quad\left(\psi_{n}, V \psi_{n \pm 1}\right)=-A$$

  where $A>0$. By considering the trial wavefunction $\Psi(x, t)=\sum_{n} c_{n}(t) \psi_{n}(x)$, show that the time-dependent Schrödinger equation governing the amplitudes $c_{n}(t)$ is

  $$i \hbar \dot{c}_{n}=\left(E_{0}+\alpha\right) c_{n}-A\left(c_{n+1}+c_{n-1}\right)$$

  By examining a solution of the form

  $$c_{n}=e^{i(k n a-E t / \hbar)}$$

  show that $E$, the energy of the electron in the crystal, lies in a band given by

  $$E=E_{0}+\alpha-2 A \cos k a$$

  Using the fact that $\psi_{0}(x)$ is a parity eigenstate show that

  $$\left(\psi_{n}, x \psi_{n}\right)=n a .$$

  The electron in this model is now subject to an electric field $\mathcal{E}$ in the direction of increasing $x$, so that $V(x)$ is replaced by $V(x)-e \mathcal{E} x$, where $-e$ is the charge on the electron. Assuming that $\left(\psi_{n}, x \psi_{m}\right)=0, n \neq m$, write down the new form of the time-dependent Schrödinger equation for the probability amplitudes $c_{n}$. Verify that it has solutions of the form

  $$c_{n}=\exp \left[-\frac{i}{\hbar} \int_{0}^{t} \epsilon\left(t^{\prime}\right) d t^{\prime}+i\left(k+\frac{e \mathcal{E} t}{\hbar}\right) n a\right]$$

  where

  $$\epsilon(t)=E_{0}+\alpha-2 A \cos \left[\left(k+\frac{e \mathcal{E} t}{\hbar}\right) a\right]$$

  Use this result to show that the dynamical behaviour of an electron near the bottom of an energy band is the same as that for a free particle in the presence of an electric field with an effective mass $m^{*}=\hbar^{2} /\left(2 A a^{2}\right)$.

  comment

• Paper 4, Section II, $33 E$

  Applications of Quantum Mechanics | Part II, 2012

  Consider a one-dimensional crystal lattice of lattice spacing $a$ with the $n$-th atom having position $r_{n}=n a+x_{n}$ and momentum $p_{n}$, for $n=0,1, \ldots, N-1$. The atoms interact with their nearest neighbours with a harmonic force and the classical Hamiltonian is

  $$H=\sum_{n} \frac{p_{n}^{2}}{2 m}+\frac{1}{2} \lambda\left(x_{n}-x_{n-1}\right)^{2}$$

  where we impose periodic boundary conditions: $x_{N}=x_{0}$. Show that the normal mode frequencies for the classical harmonic vibrations of the system are given by

  $$\omega_{l}=2 \sqrt{\frac{\lambda}{m}}\left|\sin \left(\frac{k_{l} a}{2}\right)\right|,$$

  where $k_{l}=2 \pi l / N a$, with $l$ integer and (for $N$ even, which you may assume) $-N / 2<l \leqslant$ $N / 2$. What is the velocity of sound in this crystal?

  Show how the system may be quantized to give the quantum operator

  $$x_{n}(t)=X_{0}(t)+\sum_{l \neq 0} \sqrt{\frac{\hbar}{2 N m \omega_{l}}}\left[a_{l} e^{-i\left(\omega_{l} t-k_{l} n a\right)}+a_{l}^{\dagger} e^{i\left(\omega_{l} t-k_{l} n a\right)}\right],$$

  where $a_{l}^{\dagger}$ and $a_{l}$ are creation and annihilation operators, respectively, whose commutation relations should be stated. Briefly describe the spectrum of energy eigenstates for this system, stating the definition of the ground state $|0\rangle$ and giving the expression for the energy eigenvalue of any eigenstate.

  The Debye-Waller factor $e^{-W(Q)}$ associated with Bragg scattering from this crystal is defined by the matrix element

  $$e^{-W(Q)}=\left\langle 0\left|e^{i Q x_{0}(0)}\right| 0\right\rangle$$

  In the case where $\left\langle 0\left|X_{0}\right| 0\right\rangle=0$, calculate $W(Q)$.

  comment

Applied Probability

• Paper 1, Section II, $27 \mathrm{~K}$

  Applied Probability | Part II, 2012

  (a) Give the definition of a Poisson process $\left(N_{t}, t \geqslant 0\right)$ with rate $\lambda$, using its transition rates. Show that for each $t \geqslant 0$, the distribution of $N_{t}$ is Poisson with a parameter to be specified.

  Let $J_{0}=0$ and let $J_{1}, J_{2}, \ldots$ denote the jump times of $\left(N_{t}, t \geqslant 0\right)$. What is the distribution of $\left(J_{n+1}-J_{n}, n \geqslant 0\right) ?$ (You do not need to justify your answer.)

  (b) Let $n \geqslant 1$. Compute the joint probability density function of $\left(J_{1}, J_{2}, \ldots, J_{n}\right)$ given $\left\{N_{t}=n\right\}$. Deduce that, given $\left\{N_{t}=n\right\},\left(J_{1}, \ldots, J_{n}\right)$ has the same distribution as the nondecreasing rearrangement of $n$ independent uniform random variables on $[0, t]$.

  (c) Starting from time 0, passengers arrive on platform $9 \mathrm{~B}$ at King's Cross station, with constant rate $\lambda>0$, in order to catch a train due to depart at time $t>0$. Using the above results, or otherwise, find the expected total time waited by all passengers (the sum of all passengers' waiting times).

  comment

• Paper 2, Section II, K

  Applied Probability | Part II, 2012

  (a) A colony of bacteria evolves as follows. Let $X$ be a random variable with values in the positive integers. Each bacterium splits into $X$ copies of itself after an exponentially distributed time of parameter $\lambda>0$. Each of the $X$ daughters then splits in the same way but independently of everything else. This process keeps going forever. Let $Z_{t}$ denote the number of bacteria at time $t$. Specify the $Q$-matrix of the Markov chain $Z=\left(Z_{t}, t \geqslant 0\right)$. [It will be helpful to introduce $p_{n}=\mathbb{P}(X=n)$, and you may assume for simplicity that $\left.p_{0}=p_{1}=0 .\right]$

  (b) Using the Kolmogorov forward equation, or otherwise, show that if $u(t)=$ $\mathbb{E}\left(Z_{t} \mid Z_{0}=1\right)$, then $u^{\prime}(t)=\alpha u(t)$ for some $\alpha$ to be explicitly determined in terms of $X$. Assuming that $\mathbb{E}(X)<\infty$, deduce the value of $u(t)$ for all $t \geqslant 0$, and show that $Z$ does not explode. [You may differentiate series term by term and exchange the order of summation without justification.]

  (c) We now assume that $X=2$ with probability 1 . Fix $0<q<1$ and let $\phi(t)=\mathbb{E}\left(q^{Z_{t}} \mid Z_{0}=1\right)$. Show that $\phi$ satisfies

  $$\phi(t)=q e^{-\lambda t}+\int_{0}^{t} \lambda e^{-\lambda s} \phi(t-s)^{2} d s$$

  By making the change of variables $u=t-s$, show that $d \phi / d t=\lambda \phi(\phi-1)$. Deduce that for all $n \geqslant 1, \mathbb{P}\left(Z_{t}=n \mid Z_{0}=1\right)=\beta^{n-1}(1-\beta)$ where $\beta=1-e^{-\lambda t}$.

  comment

• Paper 3, Section II, K

  Applied Probability | Part II, 2012

  We consider a system of two queues in tandem, as follows. Customers arrive in the first queue at rate $\lambda$. Each arriving customer is immediately served by one of infinitely many servers at rate $\mu_{1}$. Immediately after service, customers join a single-server second queue which operates on a first-come, first-served basis, and has a service rate $\mu_{2}$. After service in this second queue, each customer returns to the first queue with probability $0<1-p<1$, and otherwise leaves the system forever. A schematic representation is given below:

  

  (a) Let $M_{t}$ and $N_{t}$ denote the number of customers at time $t$ in queues number 1 and 2 respectively, including those currently in service at time $t$. Give the transition rates of the Markov chain $\left(M_{t}, N_{t}\right)_{t \geqslant 0}$.

  (b) Write down an equation satisfied by any invariant measure $\pi$ for this Markov chain. Let $\alpha>0$ and $\beta \in(0,1)$. Define a measure $\pi$ by

  $$\pi(m, n):=e^{-\alpha} \frac{\alpha^{m}}{m !} \beta^{n}(1-\beta), \quad m, n \in\{0,1, \ldots\}$$

  Show that it is possible to find $\alpha>0, \beta \in(0,1)$ so that $\pi$ is an invariant measure of $\left(M_{t}, N_{t}\right)_{t \geqslant 0}$, if and only if $\lambda<\mu_{2} p$. Give the values of $\alpha$ and $\beta$ in this case.

  (c) Assume now that $\lambda p>\mu_{2}$. Show that the number of customers is not positive recurrent.

  [Hint. One way to solve the problem is as follows. Assume it is positive recurrent. Observe that $M_{t}$ is greater than a $M / M / \infty$ queue with arrival rate $\lambda$. Deduce that $N_{t}$ is greater than a $M / M / 1$ queue with arrival rate $\lambda p$ and service rate $\mu_{2}$. You may use without proof the fact that the departure process from the first queue is then, at equilibrium, a Poisson process with rate $\lambda$, and you may use without proof properties of thinned Poisson processes.]

  comment

• Paper 4, Section II, $26 K$

  Applied Probability | Part II, 2012

  (a) Define the Moran model and Kingman's $n$-coalescent. State and prove a theorem which describes the relationship between them. [You may use without proof a construction of the Moran model for all $-\infty<t<\infty$.]

  (b) Let $\theta>0$. Suppose that a population of $N \geqslant 2$ individuals evolves according to the rules of the Moran model. Assume also that each individual in the population undergoes a mutation at constant rate $u=\theta /(N-1)$. Each time a mutation occurs, we assume that the allelic type of the corresponding individual changes to an entirely new type, never seen before in the population. Let $p(\theta)$ be the homozygosity probability, i.e., the probability that two individuals sampled without replacement from the population have the same genetic type. Give an expression for $p(\theta)$.

  (c) Let $q(\theta)$ denote the probability that a sample of size $n$ consists of one allelic type (monomorphic population). Show that $q(\theta)=\mathbb{E}\left(\exp \left\{-(\theta / 2) L_{n}\right\}\right)$, where $L_{n}$ denotes the sum of all the branch lengths in the genealogical tree of the sample - that is, $L_{n}=\sum_{i=2}^{n} i\left(\tau_{i}-\tau_{i-1}\right)$, where $\tau_{i}$ is the first time that the genealogical tree of the sample has $i$ lineages. Deduce that

  $$q(\theta)=\frac{(n-1) !}{\prod_{i=1}^{n-1}(\theta+i)}$$

  comment

Asymptotic Methods

• Paper 1, Section II, B

  Asymptotic Methods | Part II, 2012

  What precisely is meant by the statement that

  $$f(x) \sim \sum_{n=0}^{\infty} d_{n} x^{n}$$

  as $x \rightarrow 0 ?$

  Consider the Stieltjes integral

  $$I(x)=\int_{1}^{\infty} \frac{\rho(t)}{1+x t} d t$$

  where $\rho(t)$ is bounded and decays rapidly as $t \rightarrow \infty$, and $x>0$. Find an asymptotic series for $I(x)$ of the form $(*)$, as $x \rightarrow 0$, and prove that it has the asymptotic property.

  In the case that $\rho(t)=e^{-t}$, show that the coefficients $d_{n}$ satisfy the recurrence relation

  $$d_{n}=(-1)^{n} \frac{1}{e}-n d_{n-1} \quad(n \geqslant 1)$$

  and that $d_{0}=\frac{1}{e}$. Hence find the first three terms in the asymptotic series.

  comment

• Paper 3, Section II, B

  Asymptotic Methods | Part II, 2012

  Find the two leading terms in the asymptotic expansion of the Laplace integral

  $$I(x)=\int_{0}^{1} f(t) e^{x t^{4}} d t$$

  as $x \rightarrow \infty$, where $f(t)$ is smooth and positive on $[0,1]$.

  comment

• Paper 4, Section II, B

  Asymptotic Methods | Part II, 2012

  The stationary Schrödinger equation in one dimension has the form

  $$\epsilon^{2} \frac{d^{2} \psi}{d x^{2}}=-(E-V(x)) \psi$$

  where $\epsilon$ can be assumed to be small. Using the Liouville-Green method, show that two approximate solutions in a region where $V(x)<E$ are

  $$\psi(x) \sim \frac{1}{(E-V(x))^{1 / 4}} \exp \left\{\pm \frac{i}{\epsilon} \int_{c}^{x}\left(E-V\left(x^{\prime}\right)\right)^{1 / 2} d x^{\prime}\right\}$$

  where $c$ is suitably chosen.

  Without deriving connection formulae in detail, describe how one obtains the condition

  $$\frac{1}{\epsilon} \int_{a}^{b}\left(E-V\left(x^{\prime}\right)\right)^{1 / 2} d x^{\prime}=\left(n+\frac{1}{2}\right) \pi$$

  for the approximate energies $E$ of bound states in a smooth potential well. State the appropriate values of $a, b$ and $n$.

  Estimate the range of $n$ for which $(*)$ gives a good approximation to the true bound state energies in the cases

  (i) $V(x)=|x|$,

  (ii) $V(x)=x^{2}+\lambda x^{6}$ with $\lambda$ small and positive,

  (iii) $V(x)=x^{2}-\lambda x^{6}$ with $\lambda$ small and positive.

  comment

Classical Dynamics

• Paper 1, Section I, A

  Classical Dynamics | Part II, 2012

  Consider a heavy symmetric top of mass $M$, pinned at point $P$, which is a distance $l$ from the centre of mass.

  (a) Working in the body frame $\left(\mathbf{e}_{1}, \mathbf{e}_{2}, \mathbf{e}_{3}\right)$ (where $\mathbf{e}_{3}$ is the symmetry axis of the top) define the Euler angles $(\psi, \theta, \phi)$ and show that the components of the angular velocity can be expressed in terms of the Euler angles as

  $$\boldsymbol{\omega}=(\dot{\phi} \sin \theta \sin \psi+\dot{\theta} \cos \psi, \dot{\phi} \sin \theta \cos \psi-\dot{\theta} \sin \psi, \dot{\psi}+\dot{\phi} \cos \theta)$$

  (b) Write down the Lagrangian of the top in terms of the Euler angles and the principal moments of inertia $I_{1}, I_{3}$.

  (c) Find the three constants of motion.

  comment

• Paper 2, Section I, A

  Classical Dynamics | Part II, 2012

  (a) The action for a system with a generalized coordinate $q$ is given by

  $$S=\int_{t_{1}}^{t_{2}} L(q, \dot{q}, t) d t$$

  State the Principle of Least Action and state the Euler-Lagrange equation.

  (b) Consider a light rigid circular wire of radius $a$ and centre $O$. The wire lies in a vertical plane, which rotates about the vertical axis through $O$. At time $t$ the plane containing the wire makes an angle $\phi(t)$ with a fixed vertical plane. A bead of mass $m$ is threaded onto the wire. The bead slides without friction along the wire, and its location is denoted by $A$. The angle between the line $O A$ and the downward vertical is $\theta(t)$.

  Show that the Lagrangian of this system is

  $$\frac{m a^{2}}{2} \dot{\theta}^{2}+\frac{m a^{2}}{2} \dot{\phi}^{2} \sin ^{2} \theta+m g a \cos \theta .$$

  Calculate two independent constants of the motion, and explain their physical significance.

  comment

• Paper 2, Section II, A

  Classical Dynamics | Part II, 2012

  Consider a rigid body with principal moments of inertia $I_{1}, I_{2}, I_{3}$.

  (a) Derive Euler's equations of torque-free motion

  $$\begin{aligned} &I_{1} \dot{\omega}_{1}=\left(I_{2}-I_{3}\right) \omega_{2} \omega_{3}, \\ &I_{2} \dot{\omega}_{2}=\left(I_{3}-I_{1}\right) \omega_{3} \omega_{1} \\ &I_{3} \dot{\omega}_{3}=\left(I_{1}-I_{2}\right) \omega_{1} \omega_{2} \end{aligned}$$

  with components of the angular velocity $\boldsymbol{\omega}=\left(\omega_{1}, \omega_{2}, \omega_{3}\right)$ given in the body frame.

  (b) Show that rotation about the second principal axis is unstable if $\left(I_{2}-I_{3}\right)\left(I_{1}-I_{2}\right)>0$.

  (c) The principal moments of inertia of a uniform cylinder of radius $R$, height $h$ and mass $M$ about its centre of mass are

  $$I_{1}=I_{2}=\frac{M R^{2}}{4}+\frac{M h^{2}}{12} \quad ; \quad I_{3}=\frac{M R^{2}}{2} .$$

  The cylinder has two identical cylindrical holes of radius $r$ drilled along its length. The axes of symmetry of the holes are at a distance $a$ from the axis of symmetry of the cylinder such that $r<R / 2$ and $r<a<R-r$. All three axes lie in a single plane. Compute the principal moments of inertia of the body.

  comment

• Paper 3, Section I, A

  Classical Dynamics | Part II, 2012

  The motion of a particle of charge $q$ and mass $m$ in an electromagnetic field with scalar potential $\phi(\mathbf{r}, t)$ and vector potential $\mathbf{A}(\mathbf{r}, t)$ is characterized by the Lagrangian

  $$L=\frac{m \dot{\mathbf{r}}^{2}}{2}-q(\phi-\dot{\mathbf{r}} \cdot \mathbf{A})$$

  (a) Show that the Euler-Lagrange equation is invariant under the gauge transformation

  $$\phi \rightarrow \phi-\frac{\partial \Lambda}{\partial t}, \quad \mathbf{A} \rightarrow \mathbf{A}+\nabla \Lambda$$

  for an arbitrary function $\Lambda(\mathbf{r}, t)$.

  (b) Derive the equations of motion in terms of the electric and magnetic fields $\mathbf{E}(\mathbf{r}, t)$ and $\mathbf{B}(\mathbf{r}, t)$.

  [Recall that $\mathbf{B}=\nabla \times \mathbf{A}$ and $\mathbf{E}=-\nabla \phi-\frac{\partial \mathbf{A}}{\partial t}$.]

  comment

• Paper 4, Section I, A

  Classical Dynamics | Part II, 2012

  Consider a one-dimensional dynamical system with generalized coordinate and momentum $(q, p)$.

  (a) Define the Poisson bracket $\{f, g\}$ of two functions $f(q, p, t)$ and $g(q, p, t)$.

  (b) Find the Poisson brackets $\{q, q\},\{p, p\}$ and $\{q, p\}$.

  (c) Assuming Hamilton's equations of motion prove that

  $$\frac{d f}{d t}=\{f, H\}+\frac{\partial f}{\partial t}$$

  (d) State the condition for a transformation $(q, p) \rightarrow(Q, P)$ to be canonical in terms of the Poisson brackets found in (b). Use this to determine whether or not the following transformations are canonical:

  (i) $Q=\sin q, P=\frac{p-a}{\cos q}$,

  (ii) $Q=\cos q, P=\frac{p-a}{\sin q}$,

  where $a$ is constant.

  comment

• Paper 4, Section II, A

  Classical Dynamics | Part II, 2012

  A homogenous thin rod of mass $M$ and length $l$ is constrained to rotate in a horizontal plane about its centre $O$. A bead of mass $m$ is set to slide along the rod without friction. The bead is attracted to $O$ by a force resulting in a potential $k x^{2} / 2$, where $x$ is the distance from $O$.

  (a) Identify suitable generalized coordinates and write down the Lagrangian of the system.

  (b) Identify all conserved quantities.

  (c) Derive the equations of motion and show that one of them can be written as

  $$m \ddot{x}=-\frac{\partial V_{\mathrm{eff}}(x)}{\partial x}$$

  where the form of the effective potential $V_{\text {eff }}(x)$ should be found explicitly.

  (d) Sketch the effective potential. Find and characterize all points of equilibrium.

  (e) Find the frequencies of small oscillations around the stable equilibria.

  comment

Coding and Cryptography

• Paper 1, Section I, G

  Coding and Cryptography | Part II, 2012

  Let $\mathcal{A}$ and $\mathcal{B}$ be alphabets of sizes $m$ and a respectively. What does it mean to say that $c: \mathcal{A} \rightarrow \mathcal{B}^{*}$ is a decodable code? State Kraft's inequality.

  Suppose that a source emits letters from the alphabet $\mathcal{A}=\{1,2, \ldots, m\}$, each letter $j$ occurring with (known) probability $p_{j}>0$. Let $S$ be the codeword-length random variable for a decodable code $c: \mathcal{A} \rightarrow \mathcal{B}^{*}$, where $|\mathcal{B}|=a$. It is desired to find a decodable code that minimizes the expected value of $a^{S}$. Establish the lower bound $\mathbb{E}\left(a^{S}\right) \geqslant\left(\sum_{j=1}^{m} \sqrt{p_{j}}\right)^{2}$, and characterise when equality occurs. [Hint. You may use without proof the Cauchy-Schwarz inequality, that (for positive $x_{i}, y_{i}$ )

  $$\sum_{i=1}^{m} x_{i} y_{i} \leqslant\left(\sum_{i=1}^{m} x_{i}^{2}\right)^{1 / 2}\left(\sum_{i=1}^{m} y_{i}^{2}\right)^{1 / 2}$$

  with equality if and only if $x_{i}=\lambda y_{i}$ for all i.]

  comment

• Paper 1, Section II, 12G

  Coding and Cryptography | Part II, 2012

  Define a cyclic binary code of length $n$.

  Show how codewords can be identified with polynomials in such a way that cyclic binary codes correspond to ideals in the polynomial ring with a suitably chosen multiplication rule.

  Prove that any cyclic binary code $C$ has a unique generator, that is, a polynomial $c(X)$ of minimum degree, such that the code consists of the multiples of this polynomial. Prove that the rank of the code equals $n-\operatorname{deg} c(X)$, and show that $c(X)$ divides $X^{n}-1$.

  Show that the repetition and parity check codes are cyclic, and determine their generators.

  comment

• Paper 2, Section I, G

  Coding and Cryptography | Part II, 2012

  What is a (binary) linear code? What does it mean to say that a linear code has length $n$ and minimum weight $d$ ? When is a linear code perfect? Show that, if $n=2^{r}-1$, there exists a perfect linear code of length $n$ and minimum weight 3 .

  comment

• Paper 2, Section II, G

  Coding and Cryptography | Part II, 2012

  What does it mean to say that $f: \mathbb{F}_{2}^{d} \rightarrow \mathbb{F}_{2}^{d}$ is a linear feedback shift register? Let $\left(x_{n}\right)_{n \geqslant 0}$ be a stream produced by such a register. Show that there exist $N, M$ with $N+M \leqslant 2^{d}-1$ such that $x_{r+N}=x_{r}$ for all $r \geqslant M$.

  Describe and justify the Berlekamp-Massey method for 'breaking' a cipher stream arising from a linear feedback register of unknown length.

  Let $x_{n}, y_{n}, z_{n}$ be three streams produced by linear feedback registers. Set

  $$\begin{aligned} &k_{n}=x_{n} \quad \text { if } y_{n}=z_{n} \\ &k_{n}=y_{n} \quad \text { if } y_{n} \neq z_{n} \end{aligned}$$

  Show that $k_{n}$ is also a stream produced by a linear feedback register. Sketch proofs of any theorems you use.

  comment

• Paper 3, Section $I$, G

  Coding and Cryptography | Part II, 2012

  Describe the RSA system with public key $(N, e)$ and private key $d$. Give a simple example of how the system is vulnerable to a homomorphism attack. Explain how a signature system prevents such an attack.

  comment

• Paper 4, Section I, G

  Coding and Cryptography | Part II, 2012

  Describe the BB84 protocol for quantum key exchange.

  Suppose we attempt to implement the BB84 protocol but cannot send single photons. Instead we send $K$ photons at a time all with the same polarization. An enemy can separate one of these photons from the other $K-1$. Explain briefly how the enemy can intercept the key exchange without our knowledge.

  Show that an enemy can find our common key if $K=3$. Can she do so when $K=2$ (with suitable equipment)?

  comment

Cosmology

• Paper 1, Section I, E

  Cosmology | Part II, 2012

  The number density of photons in equilibrium at temperature $T$ is given by

  $$n=\frac{8 \pi}{(h c)^{3}} \int_{0}^{\infty} \frac{\nu^{2} d \nu}{e^{\beta h \nu}-1}$$

  where $\beta=1 /\left(k_{B} T\right)\left(k_{B}\right.$ is Boltzmann's constant). Show that $n \propto T^{3}$. Show further that $\epsilon \propto T^{4}$, where $\epsilon$ is the photon energy density.

  Write down the Friedmann equation for the scale factor $a(t)$ of a flat homogeneous and isotropic universe. State the relation between $a$ and the mass density $\rho$ for a radiation-dominated universe and hence deduce the time-dependence of $a$. How does the temperature $T$ depend on time?

  comment

• Paper 1, Section II, E

  Cosmology | Part II, 2012

  The Friedmann equation for the scale factor $a(t)$ of a homogeneous and isotropic universe of mass density $\rho$ is

  $$\left(\frac{\dot{a}}{a}\right)^{2}=\frac{8 \pi}{3} G \rho-\frac{k c^{2}}{a^{2}}$$

  where $\dot{a}=d a / d t$. Explain how the value of the constant $k$ affects the late-time $(t \rightarrow \infty)$ behaviour of $a$.

  Explain briefly why $\rho \propto 1 / a^{3}$ in a matter-dominated (zero-pressure) universe. By considering the scale factor $a$ of a closed universe as a function of conformal time $\tau$, defined by $d \tau=a^{-1} d t$, show that

  $$a(\tau)=\frac{\Omega_{0}}{2\left(\Omega_{0}-1\right)}[1-\cos (\sqrt{k} c \tau)]$$

  where $\Omega_{0}$ is the present $\left(\tau=\tau_{0}\right)$ density parameter, with $a\left(\tau_{0}\right)=1$. Use this result to show that

  $$t(\tau)=\frac{\Omega_{0}}{2 H_{0}\left(\Omega_{0}-1\right)^{3 / 2}}[\sqrt{k} c \tau-\sin (\sqrt{k} c \tau)],$$

  where $H_{0}$ is the present Hubble parameter. Find the time $t_{B C}$ at which this model universe ends in a "big crunch".

  Given that $\sqrt{k} c \tau_{0} \ll 1$, obtain an expression for the present age of the universe in terms of $H_{0}$ and $\Omega_{0}$, according to this model. How does it compare with the age of a flat universe?

  comment

• Paper 2, Section I, E

  Cosmology | Part II, 2012

  The Friedmann equation for the scale factor $a(t)$ of a homogeneous and isotropic universe of mass density $\rho$ is

  $$H^{2}=\frac{8 \pi G \rho}{3}-\frac{k c^{2}}{a^{2}}, \quad\left(H=\frac{\dot{a}}{a}\right)$$

  where $\dot{a}=d a / d t$ and $k$ is a constant. The mass conservation equation for a fluid of mass density $\rho$ and pressure $P$ is

  $$\dot{\rho}=-3\left(\rho+P / c^{2}\right) H$$

  Conformal time $\tau$ is defined by $d \tau=a^{-1} d t$. Show that

  $$\mathcal{H}=a H, \quad\left(\mathcal{H}=\frac{a^{\prime}}{a}\right)$$

  where $a^{\prime}=d a / d \tau$. Hence show that the acceleration equation can be written as

  $$\mathcal{H}^{\prime}=-\frac{4 \pi}{3} G\left(\rho+3 P / c^{2}\right) a^{2}$$

  Define the density parameter $\Omega_{m}$ and show that in a matter-dominated era, in which $P=0$, it satisfies the equation

  $$\Omega_{m}^{\prime}=\mathcal{H} \Omega_{m}\left(\Omega_{m}-1\right)$$

  Use this result to briefly explain the "flatness problem" of cosmology.

  comment

• Paper 3, Section I, E

  Cosmology | Part II, 2012

  For an ideal Fermi gas in equilibrium at temperature $T$ and chemical potential $\mu$, the average occupation number of the $k$ th energy state, with energy $E_{k}$, is

  $$\bar{n}_{k}=\frac{1}{e^{\left(E_{k}-\mu\right) / k_{B} T}+1} .$$

  Discuss the limit $T \rightarrow 0$. What is the Fermi energy $\epsilon_{F} ?$ How is it related to the Fermi momentum $p_{F}$ ? Explain why the density of states with momentum between $p$ and $p+d p$ is proportional to $p^{2} d p$ and use this fact to deduce that the fermion number density at zero temperature takes the form

  $$n \propto p_{F}^{3} .$$

  Consider an ideal Fermi gas that, at zero temperature, is either (i) non-relativistic or (ii) ultra-relativistic. In each case show that the fermion energy density $\epsilon$ takes the form

  $$\epsilon \propto n^{\gamma}$$

  for some constant $\gamma$ which you should compute.

  comment

• Paper 3, Section II, E

  Cosmology | Part II, 2012

  In a flat expanding universe with scale factor $a(t)$, average mass density $\bar{\rho}$ and average pressure $\bar{P} \ll \bar{\rho} c^{2}$, the fractional density perturbations $\delta_{k}(t)$ at co-moving wavenumber $k$ satisfy the equation

  $$\ddot{\delta}_{k}=-2\left(\frac{\dot{a}}{a}\right) \dot{\delta}_{k}+4 \pi G \bar{\rho} \delta_{k}-\frac{c_{s}^{2} k^{2}}{a^{2}} \delta_{k}$$

  Discuss briefly the meaning of each term on the right hand side of this equation. What is the Jeans length $\lambda_{J}$, and what is its significance? How is it related to the Jeans mass?

  How does the equation $(*)$ simplify at $\lambda \gg \lambda_{J}$ in a flat universe? Use your result to show that density perturbations can grow. For a growing density perturbation, how does $\dot{\delta} / \delta$ compare to the inverse Hubble time?

  Explain qualitatively why structure only forms after decoupling, and why cold dark matter is needed for structure formation.

  comment

• Paper 4, Section I, E

  Cosmology | Part II, 2012

  The number density of a species $\star$ of non-relativistic particles of mass $m$, in equilibrium at temperature $T$ and chemical potential $\mu$, is

  $$n_{\star}=g_{\star}\left(\frac{2 \pi m k T}{h^{2}}\right)^{3 / 2} e^{\left(\mu-m c^{2}\right) / k T},$$

  where $g_{\star}$ is the spin degeneracy. During primordial nucleosynthesis, deuterium, $D$, forms through the nuclear reaction

  $$p+n \leftrightarrow D,$$

  where $p$ and $n$ are non-relativistic protons and neutrons. Write down the relationship between the chemical potentials in equilibrium.

  Using the fact that $g_{D}=4$, and explaining the approximations you make, show that

  $$\frac{n_{D}}{n_{n} n_{p}} \approx\left(\frac{h^{2}}{\pi m_{p} k T}\right)^{3 / 2} \exp \left(\frac{B_{D}}{k T}\right)$$

  where $B_{D}$ is the deuterium binding energy, i.e. $B_{D}=\left(m_{n}+m_{p}-m_{D}\right) c^{2}$.

  Let $X_{\star}=n_{\star} / n_{B}$ where $n_{B}$ is the baryon number density of the universe. Using the fact that $n_{\gamma} \propto T^{3}$, show that

  $$\frac{X_{D}}{X_{n} X_{p}} \propto T^{3 / 2} \eta \exp \left(\frac{B_{D}}{k T}\right)$$

  where $\eta$ is the baryon asymmetry parameter

  $$\eta=\frac{n_{B}}{n_{\gamma}}$$

  Briefly explain why primordial deuterium does not form until temperatures well below $k T \sim B_{D}$.

  comment

Differential Geometry

• Paper 1, Section II, I

  Differential Geometry | Part II, 2012

  Define the geodesic curvature $k_{g}$ of a regular curve in an oriented surface $S \subset \mathbb{R}^{3}$. When is $k_{g}=0$ along a curve?

  Explain briefly what is meant by the Euler characteristic $\chi$ of a compact surface $S \subset \mathbb{R}^{3}$. State the global Gauss-Bonnet theorem with boundary terms.

  Let $S$ be a surface with positive Gaussian curvature that is diffeomorphic to the sphere $S^{2}$ and let $\gamma_{1}, \gamma_{2}$ be two disjoint simple closed curves in $S$. Can both $\gamma_{1}$ and $\gamma_{2}$ be geodesics? Can both $\gamma_{1}$ and $\gamma_{2}$ have constant geodesic curvature? Justify your answers.

  [You may assume that the complement of a simple closed curve in $S^{2}$ consists of two open connected regions.]

  comment

• Paper 2, Section II, I

  Differential Geometry | Part II, 2012

  Define the Gauss map $N$ for an oriented surface $S \subset \mathbb{R}^{3}$. Show that at each $p \in S$ the derivative of the Gauss map

  $$d N_{p}: T_{p} S \rightarrow T_{N(p)} S^{2}=T_{p} S$$

  is self-adjoint. Define the principal curvatures $k_{1}, k_{2}$ of $S$.

  Now suppose that $S$ is compact (and without boundary). By considering the square of the distance to the origin, or otherwise, prove that $S$ has a point $p$ with $k_{1}(p) k_{2}(p)>0$.

  [You may assume that the intersection of $S$ with a plane through the normal direction at $p \in S$ contains a regular curve through $p$.]

  comment

• Paper 3, Section II, I

  Differential Geometry | Part II, 2012

  For a surface $S \subset \mathbb{R}^{3}$, define what is meant by the exponential mapping exp $p$ at $p \in S$, geodesic polar coordinates $(r, \theta)$ and geodesic circles.

  Let $E, F, G$ be the coefficients of the first fundamental form in geodesic polar coordinates $(r, \theta)$. Prove that $\lim _{r \rightarrow 0} \sqrt{G}(r, \theta)=0$ and $\lim _{r \rightarrow 0}(\sqrt{G})_{r}(r, \theta)=1$. Give an expression for the Gaussian curvature $K$ in terms of $G$.

  Prove that the Gaussian curvature at a point $p \in S$ satisfies

  $$K(p)=\lim _{r \rightarrow 0} \frac{12\left(\pi r^{2}-A_{p}(r)\right)}{\pi r^{4}}$$

  where $A_{p}(r)$ is the area of the region bounded by the geodesic circle of radius $r$ centred at $p$.

  [You may assume that $E=1, F=0$ and $d\left(\exp _{p}\right)_{0}$ is an isometry. Taylor's theorem with any form of the remainder may be assumed if accurately stated.]

  comment

• Paper 4, Section II, I

  Differential Geometry | Part II, 2012

  For manifolds $X, Y \subset \mathbb{R}^{n}$, define the terms tangent space to $X$ at a point $x \in X$ and derivative $d f_{x}$ of a smooth map $f: X \rightarrow Y$. State the Inverse Function Theorem for smooth maps between manifolds without boundary.

  Now let $X$ be a submanifold of $Y$ and $f: X \rightarrow Y$ the inclusion map. By considering the map $f^{-1}: f(X) \rightarrow X$, or otherwise, show that $d f_{x}$ is injective for each $x \in X$.

  Show further that there exist local coordinates around $x$ and around $y=f(x)$ such that $f$ is given in these coordinates by

  $$\left(x_{1}, \ldots, x_{l}\right) \in \mathbb{R}^{l} \mapsto\left(x_{1}, \ldots, x_{l}, 0, \ldots, 0\right) \in \mathbb{R}^{k},$$

  where $l=\operatorname{dim} X$ and $k=\operatorname{dim} Y$. [You may assume that any open ball in $\mathbb{R}^{l}$ is diffeomorphic to $\mathbb{R}^{l}$.]

  comment

Dynamical Systems

• Paper 1, Section I, $7 \mathrm{D}$

  Dynamical Systems | Part II, 2012

  State the Poincaré-Bendixson theorem.

  A model of a chemical process obeys the second-order system

  $$\dot{x}=1-x(1+a)+x^{2} y, \quad \dot{y}=a x-x^{2} y$$

  where $a>0$. Show that there is a unique fixed point at $(x, y)=(1, a)$ and that it is unstable if $a>2$. Show that trajectories enter the region bounded by the lines $x=1 / q$, $y=0, y=a q$ and $x+y=1+a q$, provided $q>(1+a)$. Deduce that there is a periodic orbit when $a>2$.

  comment

• Paper 2, Section I, D

  Dynamical Systems | Part II, 2012

  Consider the dynamical system

  $$\dot{x}=\mu x+x^{3}-a x y, \quad \dot{y}=\mu-x^{2}-y,$$

  where $a$ is a constant.

  (a) Show that there is a bifurcation from the fixed point $(0, \mu)$ at $\mu=0$.

  (b) Find the extended centre manifold at leading non-trivial order in $x$. Hence find the type of bifurcation, paying particular attention to the special values $a=1$ and $a=-1$. [Hint. At leading order, the extended centre manifold is of the form $y=\mu+\alpha x^{2}+\beta \mu x^{2}+\gamma x^{4}$, where $\alpha, \beta, \gamma$ are constants to be determined.]

  comment

• Paper 3, Section I, D

  Dynamical Systems | Part II, 2012

  State without proof Lyapunov's first theorem, carefully defining all the terms that you use.

  Consider the dynamical system

  $$\begin{aligned} &\dot{x}=-2 x+y-x y+3 y^{2}-x y^{2}+x^{3} \\ &\dot{y}=-2 y-x-y^{2}-3 x y+2 x^{2} y \end{aligned}$$

  By choosing a Lyapunov function $V(x, y)=x^{2}+y^{2}$, prove that the origin is asymptotically stable.

  By factorising the expression for $\dot{V}$, or otherwise, show that the basin of attraction of the origin includes the set $V<7 / 4$.

  comment

• Paper 3, Section II, D

  Dynamical Systems | Part II, 2012

  Consider the dynamical system

  $$\ddot{x}-(a-b x) \dot{x}+x-x^{2}=0, \quad a, b>0 .$$

  (a) Show that the fixed point at the origin is an unstable node or focus, and that the fixed point at $x=1$ is a saddle point.

  (b) By considering the phase plane $(x, \dot{x})$, or otherwise, show graphically that the maximum value of $x$ for any periodic orbit is less than one.

  (c) By writing the system in terms of the variables $x$ and $z=\dot{x}-\left(a x-b x^{2} / 2\right)$, or otherwise, show that for any periodic orbit $\mathcal{C}$

  $$\oint_{\mathcal{C}}\left(x-x^{2}\right)\left(2 a x-b x^{2}\right) d t=0$$

  Deduce that if $a / b>1 / 2$ there are no periodic orbits.

  (d) If $a=b=0$ the system (1) is Hamiltonian and has homoclinic orbit

  $$X(t)=\frac{1}{2}\left(3 \tanh ^{2}\left(\frac{t}{2}\right)-1\right)$$

  which approaches $X=1$ as $t \rightarrow \pm \infty$. Now suppose that $a, b$ are very small and that we seek the value of $a / b$ corresponding to a periodic orbit very close to $X(t)$. By using equation (3) in equation (2), find an approximation to the largest value of $a / b$ for a periodic orbit when $a, b$ are very small.

  [Hint. You may use the fact that $\left.(1-X)=\frac{3}{2} \operatorname{sech}^{2}\left(\frac{t}{2}\right)=3 \frac{d}{d t}\left(\tanh \left(\frac{t}{2}\right)\right)\right]$

  comment

• Paper 4, Section I, D

  Dynamical Systems | Part II, 2012

  Describe the different types of bifurcation from steady states of a one-dimensional map of the form $x_{n+1}=f\left(x_{n}\right)$, and give examples of simple equations exhibiting each type.

  Consider the map $x_{n+1}=\alpha x_{n}^{2}\left(1-x_{n}\right), 0<x_{n}<1$. What is the maximum value of $\alpha$ for which the interval is mapped into itself?

  Show that as $\alpha$ increases from zero to its maximum value there is a saddle-node bifurcation and a period-doubling bifurcation, and determine the values of $\alpha$ for which they occur.

  comment

• Paper 4, Section II, D

  Dynamical Systems | Part II, 2012

  What is meant by the statement that a continuous map of an interval $I$ into itself has a horseshoe? State without proof the properties of such a map.

  Define the property of chaos of such a map according to Glendinning.

  A continuous map $f: I \rightarrow I$ has a periodic orbit of period 5 , in which the elements $x_{j}, j=1, \ldots, 5$ satisfy $x_{j}<x_{j+1}, j=1, \ldots, 4$ and the points are visited in the order $x_{1} \rightarrow x_{3} \rightarrow x_{4} \rightarrow x_{2} \rightarrow x_{5} \rightarrow x_{1}$. Show that the map is chaotic. [The Intermediate Value theorem can be used without proof.]

  comment

Electrodynamics

• Paper 1, Section II, B

  Electrodynamics | Part II, 2012

  A particle of mass $m$ and charge $q$ moves relativistically under the influence of a constant electric field $E$ in the positive $z$-direction, and a constant magnetic field $B$ also in the positive $z$-direction.

  In some inertial observer's coordinate system, the particle starts at

  $$x=R, \quad y=0, \quad z=0, \quad t=0$$

  with velocity given by

  $$\dot{x}=0, \quad \dot{y}=u, \quad \dot{z}=0,$$

  where the dot indicates differentiation with respect to the proper time of the particle. Show that the subsequent motion of the particle, as seen by the inertial observer, is a helix.

  a) What is the radius of the helix as seen by the inertial observer?

  b) What are the $x$ and $y$ coordinates of the axis of the helix?

  c) What is the $z$ coordinate of the particle after a proper time $\tau$ has elapsed, as measured by the particle?

  comment

• Paper 3, Section II, B

  Electrodynamics | Part II, 2012

  The non-relativistic Larmor formula for the power, $P$, radiated by a particle of charge $q$ and mass $m$ that is being accelerated with an acceleration a is

  $$P=\frac{\mu_{0}}{6 \pi} q^{2}|\mathbf{a}|^{2} .$$

  Starting from the Liénard-Wiechert potentials, sketch a derivation of this result. Explain briefly why the relativistic generalization of this formula is

  $$P=\frac{\mu_{0}}{6 \pi} \frac{q^{2}}{m^{2}}\left(\frac{d p^{\mu}}{d \tau} \frac{d p^{\nu}}{d \tau} \eta_{\mu \nu}\right)$$

  where $p^{\mu}$ is the relativistic momentum of the particle and $\tau$ is the proper time along the worldline of the particle.

  A particle of mass $m$ and charge $q$ moves in a plane perpendicular to a constant magnetic field $B$. At time $t=0$ as seen by an observer $\mathbf{O}$ at rest, the particle has energy $E=\gamma m$. At what rate is electromagnetic energy radiated by this particle?

  At time $t$ according to the observer $\mathbf{O}$, the particle has energy $E^{\prime}=\gamma^{\prime} m$. Find an expression for $\gamma^{\prime}$ in terms of $\gamma$ and $t$.

  comment

• Paper 4, Section II, B

  Electrodynamics | Part II, 2012

  The charge and current densities are given by $\rho(t, \mathbf{x}) \neq 0$ and $\mathbf{j}(t, \mathbf{x})$ respectively. The electromagnetic scalar and vector potentials are given by $\phi(t, \mathbf{x})$ and $\mathbf{A}(t, \mathbf{x})$ respectively. Explain how one can regard $j^{\mu}=(\rho, \mathbf{j})$ as a four-vector that obeys the current conservation rule $\partial_{\mu} j^{\mu}=0$.

  In the Lorenz gauge $\partial_{\mu} A^{\mu}=0$, derive the wave equation that relates $A^{\mu}=(\phi, \mathbf{A})$ to $j^{\mu}$ and hence show that it is consistent to treat $A^{\mu}$ as a four-vector.

  In the Lorenz gauge, with $j^{\mu}=0$, a plane wave solution for $A^{\mu}$ is given by

  $$A^{\mu}=\epsilon^{\mu} \exp \left(i k_{\nu} x^{\nu}\right)$$

  where $\epsilon^{\mu}, k^{\mu}$ and $x^{\mu}$ are four-vectors with

  $$\epsilon^{\mu}=\left(\epsilon^{0}, \boldsymbol{\epsilon}\right), \quad k^{\mu}=\left(k^{0}, \mathbf{k}\right), \quad x^{\mu}=(t, \mathbf{x}) .$$

  Show that $k_{\mu} k^{\mu}=k_{\mu} \epsilon^{\mu}=0$.

  Interpret the components of $k^{\mu}$ in terms of the frequency and wavelength of the wave.

  Find what residual gauge freedom there is and use it to show that it is possible to set $\epsilon^{0}=0$. What then is the physical meaning of the components of $\boldsymbol{\epsilon}$ ?

  An observer at rest in a frame $S$ measures the angular frequency of a plane wave travelling parallel to the $z$-axis to be $\omega$. A second observer travelling at velocity $v$ in $S$ parallel to the $z$-axis measures the radiation to have frequency $\omega^{\prime}$. Express $\omega^{\prime}$ in terms of $\omega$.

  comment

Fluid Dynamics II

• Paper 1, Section II, C

  Fluid Dynamics II | Part II, 2012

  Define the strain-rate tensor $e_{i j}$ in terms of the velocity components $u_{i}$. Write down the relation between $e_{i j}$, the pressure $p$ and the stress $\sigma_{i j}$ in an incompressible Newtonian fluid of viscosity $\mu$. Show that the local rate of stress-working $\sigma_{i j} \partial u_{i} / \partial x_{j}$ is equal to the local rate of dissipation $2 \mu e_{i j} e_{i j}$.

  An incompressible fluid of density $\rho$ and viscosity $\mu$ occupies the semi-infinite region $y>0$ above a rigid plane boundary $y=0$ which oscillates with velocity $(V \cos \omega t, 0,0)$. The fluid is at rest at infinity. Determine the velocity field produced by the boundary motion after any transients have decayed.

  Show that the time-averaged rate of dissipation is

  $$\frac{1}{4} \sqrt{2} V^{2}(\mu \rho \omega)^{1 / 2}$$

  per unit area of the boundary. Verify that this is equal to the time average of the rate of working by the boundary on the fluid per unit area.

  comment

• Paper 2, Section II, C

  Fluid Dynamics II | Part II, 2012

  An incompressible viscous liquid occupies the long thin region $0 \leqslant y \leqslant h(x)$ for $0 \leqslant x \leqslant \ell$, where $h(x)=d_{1}+\alpha x$ with $h(0)=d_{1}, h(\ell)=d_{2}<d_{1}$ and $d_{1} \ll \ell$. The top boundary at $y=h(x)$ is rigid and stationary. The bottom boundary at $y=0$ is rigid and moving at velocity $(U, 0,0)$. Fluid can move in and out of the ends $x=0$ and $x=\ell$, where the pressure is the same, namely $p_{0}$.

  Explaining the approximations of lubrication theory as you use them, find the velocity profile in the long thin region, and show that the volume flux $Q$ (per unit width in the $z$-direction) is

  $$Q=\frac{U d_{1} d_{2}}{d_{1}+d_{2}}$$

  Find also the value of $h(x)$ (i) where the pressure is maximum, (ii) where the tangential viscous stress on the bottom $y=0$ vanishes, and (iii) where the tangential viscous stress on the top $y=h(x)$ vanishes.

  comment

• Paper 3, Section II, C

  Fluid Dynamics II | Part II, 2012

  For two Stokes flows $\mathbf{u}^{(1)}(\mathbf{x})$ and $\mathbf{u}^{(2)}(\mathbf{x})$ inside the same volume $V$ with different boundary conditions on its boundary $S$, prove the reciprocal theorem

  $$\int_{S} \sigma_{i j}^{(1)} n_{j} u_{i}^{(2)} d S=\int_{S} \sigma_{i j}^{(2)} n_{j} u_{i}^{(1)} d S$$

  where $\sigma^{(1)}$ and $\sigma^{(2)}$ are the stress fields associated with the flows.

  When a rigid sphere of radius $a$ translates with velocity $\mathbf{U}$ through unbounded fluid at rest at infinity, it may be shown that the traction per unit area, $\boldsymbol{\sigma} \cdot \mathbf{n}$, exerted by the sphere on the fluid has the uniform value $3 \mu \mathbf{U} / 2 a$ over the sphere surface. Find the drag on the sphere.

  Suppose that the same sphere is now free of external forces and is placed with its centre at the origin in an unbounded Stokes flow given in the absence of the sphere as $\mathbf{u}^{*}(\mathbf{x})$. By applying the reciprocal theorem to the perturbation to the flow generated by the presence of the sphere, and assuming this tends to zero sufficiently rapidly at infinity, show that the instantaneous velocity of the centre of the sphere is

  $$\frac{1}{4 \pi a^{2}} \int \mathbf{u}^{*}(\mathbf{x}) d S$$

  where the integral is taken over the sphere of radius $a$.

  comment

• Paper 4, Section II, C

  Fluid Dynamics II | Part II, 2012

  A steady, two-dimensional flow in the region $y>0$ takes the form $(u, v)=$ $(E x,-E y)$ at large $y$, where $E$ is a positive constant. The boundary at $y=0$ is rigid and no-slip. Consider the velocity field $u=\partial \psi / \partial y, v=-\partial \psi / \partial x$ with stream function $\psi=\operatorname{Ex} \delta f(\eta)$, where $\eta=y / \delta$ and $\delta=(\nu / E)^{1 / 2}$ and $\nu$ is the kinematic viscosity. Show that this velocity field satisfies the Navier-Stokes equations provided that $f(\eta)$ satisfies

  $$f^{\prime \prime \prime}+f f^{\prime \prime}-\left(f^{\prime}\right)^{2}=-1$$

  What are the conditions on $f$ at $\eta=0$ and as $\eta \rightarrow \infty$ ?

  comment

Further Complex Methods

• Paper 1, Section I, E

  Further Complex Methods | Part II, 2012

  Recall that if $f(z)$ is analytic in a neighbourhood of $z_{0} \neq 0$, then

  $$f(z)+\overline{f\left(z_{0}\right)}=2 u\left(\frac{z+\overline{z_{0}}}{2}, \frac{z-\overline{z_{0}}}{2 i}\right)$$

  where $u(x, y)$ is the real part of $f(z)$. Use this fact to construct the imaginary part of an analytic function whose real part is given by

  $$u(x, y)=y \int_{-\infty}^{\infty} \frac{g(t) d t}{(t-x)^{2}+y^{2}}, \quad x, y \in \mathbb{R}, y \neq 0$$

  where $g(t)$ is real and has sufficient smoothness and decay.

  comment

• Paper 1, Section II, E

  Further Complex Methods | Part II, 2012

  (a) Suppose that $F(z), z=x+i y, x, y \in \mathbb{R}$, is analytic in the upper-half complex $z$-plane and $O(1 / z)$ as $z \rightarrow \infty, y \geqslant 0$. Show that the real and imaginary parts of $F(x)$, denoted by $U(x)$ and $V(x)$ respectively, satisfy the so-called Kramers-Kronig formulae:

  $$U(x)=H V(x), \quad V(x)=-H U(x), \quad x \in \mathbb{R} .$$

  Here, $H$ denotes the Hilbert transform, i.e.,

  $$(H f)(x)=\frac{1}{\pi} \mathrm{PV} \int_{-\infty}^{\infty} \frac{f(\xi)}{\xi-x} d \xi$$

  where $\mathrm{PV}$ denotes the principal value integral.

  (b) Let the real function $u(x, y)$ satisfy the Laplace equation in the upper-half complex z-plane, i.e.,

  $$\frac{\partial^{2} u(x, y)}{\partial x^{2}}+\frac{\partial^{2} u(x, y)}{\partial y^{2}}=0, \quad-\infty<x<\infty, \quad y>0$$

  Assuming that $u(x, y)$ decays for large $|x|$ and for large $y$, show that $F=u_{z}$ is an analytic function for $\operatorname{Im} z>0, z=x+i y$. Then, find an expression for $u_{y}(x, 0)$ in terms of $u_{x}(x, 0)$.

  comment

• Paper 2, Section I, E

  Further Complex Methods | Part II, 2012

  The hypergeometric function $F(a, b ; c ; z)$ is defined as the particular solution of the second order linear ODE characterised by the Papperitz symbol

  $$\mathrm{P}\left\{\begin{array}{cccc} 0 & 1 & \infty \\ 0 & 0 & a & z \\ 1-c & c-a-b & b \end{array}\right\}$$

  that is analytic at $z=0$ and satisfies $F(a, b ; c ; 0)=1$.

  Using the fact that a second solution $w(z)$ of the above ODE is of the form

  $$w(z)=z^{1-c} u(z)$$

  where $u(z)$ is analytic in the neighbourhood of the origin, express $w(z)$ in terms of $F$.

  comment

• Paper 2, Section II, $14 \mathrm{E}$

  Further Complex Methods | Part II, 2012

  Let the complex function $q(x, t)$ satisfy

  $$i \frac{\partial q(x, t)}{\partial t}+\frac{\partial^{2} q(x, t)}{\partial x^{2}}=0, \quad 0<x<\infty, 0<t<T$$

  where $T$ is a positive constant. The unified transform method implies that the solution of any well-posed problem for the above equation is given by

  $$\begin{aligned} q(x, t) &=\frac{1}{2 \pi} \int_{-\infty}^{\infty} e^{i k x-i k^{2} t} \hat{q}_{0}(k) d k \\ &-\frac{1}{2 \pi} \int_{L} e^{i k x-i k^{2} t}\left[k \tilde{g}_{0}\left(i k^{2}, t\right)-i \tilde{g}_{1}\left(i k^{2}, t\right)\right] d k \end{aligned}$$

  where $L$ is the union of the rays $(i \infty, 0)$ and $(0, \infty), \hat{q}_{0}(k)$ denotes the Fourier transform of the initial condition $q_{0}(x)$, and $\tilde{g}_{0}, \tilde{g}_{1}$ denote the $t$-transforms of the boundary values $q(0, t), q_{x}(0, t)$ :

  $$\begin{gathered} \hat{q}_{0}(k)=\int_{0}^{\infty} e^{-i k x} q_{0}(x) d x, \quad \operatorname{Im} k \leqslant 0, \\ =\int_{0}^{t} e^{k s} q(0, s) d s, \quad \tilde{g}_{1}(k, t)=\int_{0}^{t} e^{k s} q_{x}(0, s) d s, \quad k \in \mathbb{C}, \quad 0<t<T . \end{gathered}$$

  Furthermore, $q_{0}(x), q(0, t)$ and $q_{x}(0, t)$ are related via the so-called global relation

  $$e^{i k^{2} t} \hat{q}(k, t)=\hat{q}_{0}(k)+k \tilde{g}_{0}\left(i k^{2}, t\right)-i \tilde{g}_{1}\left(i k^{2}, t\right), \quad \operatorname{Im} k \leqslant 0$$

  where $\hat{q}(k, t)$ denotes the Fourier transform of $q(x, t)$.

  (a) Assuming the validity of (1) and (2), use the global relation to eliminate $\tilde{g}_{1}$ from equation (1).

  (b) For the particular case that

  $$q_{0}(x)=e^{-a^{2} x}, \quad 0<x<\infty ; \quad q(0, t)=\cos b t, \quad 0<t<T,$$

  where $a$ and $b$ are real numbers, use the representation obtained in (a) to express the solution in terms of an integral along the real axis and an integral along $L$ (you should not attempt to evaluate these integrals). Show that it is possible to deform these two integrals to a single integral along a new contour $\tilde{L}$, which you should sketch.

  [You may assume the validity of Jordan's lemma.]

  comment

• Paper 3, Section I, E

  Further Complex Methods | Part II, 2012

  The Beta function, denoted by $B\left(z_{1}, z_{2}\right)$, is defined by

  $$B\left(z_{1}, z_{2}\right)=\frac{\Gamma\left(z_{1}\right) \Gamma\left(z_{2}\right)}{\Gamma\left(z_{1}+z_{2}\right)}, \quad z_{1}, z_{2} \in \mathbb{C}$$

  where $\Gamma(z)$ denotes the Gamma function. It can be shown that

  $$B\left(z_{1}, z_{2}\right)=\int_{0}^{\infty} \frac{v^{z_{2}-1} d v}{(1+v)^{z_{1}+z_{2}}}, \quad \operatorname{Re} z_{1}>0, \operatorname{Re} z_{2}>0$$

  By computing this integral for the particular case of $z_{1}+z_{2}=1$, and by employing analytic continuation, deduce that $\Gamma(z)$ satisfies the functional equation

  $$\Gamma(z) \Gamma(1-z)=\frac{\pi}{\sin \pi z}, \quad z \in \mathbb{C} .$$

  comment

• Paper 4, Section I, E

  Further Complex Methods | Part II, 2012

  Use the Laplace kernel method to write integral representations in the complex $t$-plane for two linearly independent solutions of the confluent hypergeometric equation

  $$z \frac{d^{2} w(z)}{d z^{2}}+(c-z) \frac{d w(z)}{d z}-a w(z)=0$$

  in the case that $\operatorname{Re}(z)>0, \operatorname{Re}(c)>\operatorname{Re}(a)>0, a$ and $c-a$ are not integers.

  comment

Galois Theory

• Paper 1, Section II, 18H

  Galois Theory | Part II, 2012

  List all subfields of the cyclotomic field $\mathbb{Q}\left(\boldsymbol{\mu}_{20}\right)$ obtained by adjoining all 20 th roots of unity to $\mathbb{Q}$, and draw the lattice diagram of inclusions among them. Write all the subfields in the form $\mathbb{Q}(\alpha)$ or $\mathbb{Q}(\alpha, \beta)$. Briefly justify your answer.

  [The description of the Galois group of cyclotomic fields and the fundamental theorem of Galois theory can be used freely without proof.]

  comment

• Paper 2, Section II, H

  Galois Theory | Part II, 2012

  Let $K, L$ be subfields of $\mathbb{C}$ with $K \subset L$.

  Suppose that $K$ is contained in $\mathbb{R}$ and $L / K$ is a finite Galois extension of odd degree. Prove that $L$ is also contained in $\mathbb{R}$.

  Give one concrete example of $K, L$ as above with $K \neq L$. Also give an example in which $K$ is contained in $\mathbb{R}$ and $L / K$ has odd degree, but is not Galois and $L$ is not contained in $\mathbb{R}$.

  [Standard facts on fields and their extensions can be quoted without proof, as long as they are clearly stated.]

  comment

• Paper 3, Section II, H

  Galois Theory | Part II, 2012

  Let $q=p^{f}(f \geqslant 1)$ be a power of the prime $p$, and $\mathbb{F}_{q}$ be a finite field consisting of $q$ elements.

  Let $N$ be a positive integer prime to $p$, and $\mathbb{F}_{q}\left(\boldsymbol{\mu}_{N}\right)$ be the cyclotomic extension obtained by adjoining all $N$ th roots of unity to $\mathbb{F}_{q}$. Prove that $\mathbb{F}_{q}\left(\boldsymbol{\mu}_{N}\right)$ is a finite field with $q^{n}$ elements, where $n$ is the order of the element $q \bmod N$ in the multiplicative group $(\mathbb{Z} / N \mathbb{Z})^{\times}$of the $\operatorname{ring} \mathbb{Z} / N \mathbb{Z}$.

  Explain why what is proven above specialises to the following fact: the finite field $\mathbb{F}_{p}$ for an odd prime $p$ contains a square root of $-1$ if and only if $p \equiv 1(\bmod 4)$.

  [Standard facts on finite fields and their extensions can be quoted without proof, as long as they are clearly stated.]

  comment

• Paper 4, Section II, H

  Galois Theory | Part II, 2012

  Let $F=\mathbb{C}\left(X_{1}, \ldots, X_{n}\right)$ be a field of rational functions in $n$ variables over $\mathbb{C}$, and let $s_{1}, \ldots, s_{n}$ be the elementary symmetric polynomials:

  $$s_{j}:=\sum_{\left\{i_{1}, \ldots, i_{j}\right\} \subset\{1, \ldots, n\}} X_{i_{1}} \cdots X_{i_{j}} \in F \quad(1 \leqslant j \leqslant n),$$

  and let $K=\mathbb{C}\left(s_{1}, \ldots, s_{n}\right)$ be the subfield of $F$ generated by $s_{1}, \ldots, s_{n}$. Let $1 \leqslant m \leqslant n$, and $Y:=X_{1}+\cdots+X_{m} \in F$. Let $K(Y)$ be the subfield of $F$ generated by $Y$ over $K$. Find the degree $[K(Y): K]$.

  [Standard facts about the fields $F, K$ and Galois extensions can be quoted without proof, as long as they are clearly stated.]

  comment

General Relativity

• Paper 1, Section II, B

  General Relativity | Part II, 2012

  (i) Using the condition that the metric tensor $g_{a b}$ is covariantly constant, derive an expression for the Christoffel symbol $\Gamma_{b c}^{a}=\Gamma_{c b}^{a}$.

  (ii) Show that

  $$\Gamma_{b a}^{a}=\frac{1}{2} g^{a c} g_{a c, b}$$

  Hence establish the covariant divergence formula

  $$V_{; a}^{a}=\frac{1}{\sqrt{-g}} \frac{\partial}{\partial x^{a}}\left(\sqrt{-g} V^{a}\right)$$

  where $g$ is the determinant of the metric tensor.

  [It may be assumed that $\partial_{a}(\log \operatorname{det} M)=\operatorname{trace}\left(M^{-1} \partial_{a} M\right)$ for any invertible matrix $M$ ].

  (iii) The Kerr-Newman metric, describing the spacetime outside a rotating black hole of mass $M$, charge $Q$ and angular momentum per unit mass $a$, is given in appropriate units by

  $$\begin{aligned} d s^{2}=&-\left(d t-a \sin ^{2} \theta d \phi\right)^{2} \frac{\Delta}{\rho^{2}} \\ &+\left(\left(r^{2}+a^{2}\right) d \phi-a d t\right)^{2} \frac{\sin ^{2} \theta}{\rho^{2}}+\left(\frac{d r^{2}}{\Delta}+d \theta^{2}\right) \rho^{2} \end{aligned}$$

  where $\rho^{2}=r^{2}+a^{2} \cos ^{2} \theta$ and $\Delta=r^{2}-2 M r+a^{2}+Q^{2}$. Explain why this metric is stationary, and make a choice of one of the parameters which reduces it to a static metric.

  Show that, in the static metric obtained, the equation

  $$\left(g^{a b} \Phi_{, b}\right)_{; a}=0$$

  for a function $\Phi=\Phi(t, r)$ admits solutions of the form

  $$\Phi=\sin (\omega t) R(r)$$

  where $\omega$ is constant and $R(r)$ satisfies an ordinary differential equation which should be found.

  comment

• Paper 2, Section II, B

  General Relativity | Part II, 2012

  The metric of any two-dimensional rotationally-symmetric curved space can be written in terms of polar coordinates, $(r, \theta)$, with $0 \leqslant \theta<2 \pi, r \geqslant 0$, as

  $$d s^{2}=e^{2 \phi}\left(d r^{2}+r^{2} d \theta^{2}\right)$$

  where $\phi=\phi(r)$. Show that the Christoffel symbols $\Gamma_{r \theta}^{r}, \Gamma_{r r}^{\theta}$ and $\Gamma_{\theta \theta}^{\theta}$ are each zero, and compute $\Gamma_{r r}^{r}, \Gamma_{\theta \theta}^{r}$ and $\Gamma_{r \theta}^{\theta}=\Gamma_{\theta r}^{\theta}$.

  The Ricci tensor is defined by

  $$R_{a b}=\Gamma_{a b, c}^{c}-\Gamma_{a c, b}^{c}+\Gamma_{c d}^{c} \Gamma_{a b}^{d}-\Gamma_{a c}^{d} \Gamma_{b d}^{c}$$

  where a comma here denotes partial derivative. Prove that $R_{r \theta}=0$ and that

  $$R_{r r}=-\phi^{\prime \prime}-\frac{\phi^{\prime}}{r}, \quad R_{\theta \theta}=r^{2} R_{r r}$$

  Suppose now that, in this space, the Ricci scalar takes the constant value $-2$. Find a differential equation for $\phi(r)$.

  By a suitable coordinate transformation $r \rightarrow \chi(r), \theta$ unchanged, this space of constant Ricci scalar can be described by the metric

  $$d s^{2}=d \chi^{2}+\sinh ^{2} \chi d \theta^{2}$$

  From this coordinate transformation, find $\cosh \chi$ and $\sinh \chi$ in terms of $r$. Deduce that

  $$e^{\phi(r)}=\frac{2 A}{1-A^{2} r^{2}},$$

  where $0 \leqslant A r<1$, and $A$ is a positive constant.

  [You may use

  $$\left.\int \frac{d \chi}{\sinh \chi}=\frac{1}{2} \log (\cosh \chi-1)-\frac{1}{2} \log (\cosh \chi+1)+\text { constant } .\right]$$

  comment

• Paper 3, Section II, 37B

  General Relativity | Part II, 2012

  (i) The Schwarzschild metric is given by

  $$d s^{2}=-\left(1-\frac{2 M}{r}\right) d t^{2}+\left(1-\frac{2 M}{r}\right)^{-1} d r^{2}+r^{2}\left(d \theta^{2}+\sin ^{2} \theta d \phi^{2}\right)$$

  Consider a time-like geodesic $x^{a}(\tau)$, where $\tau$ is the proper time, lying in the plane $\theta=\pi / 2$. Use the Lagrangian $L=g_{a b} \dot{x}^{a} \dot{x}^{b}$ to derive the equations governing the geodesic, showing that

  $$r^{2} \dot{\phi}=h$$

  with $h$ constant, and hence demonstrate that

  $$\frac{d^{2} u}{d \phi^{2}}+u=\frac{M}{h^{2}}+3 M u^{2}$$

  where $u=1 / r$. State which term in this equation makes it different from an analogous equation in Newtonian theory.

  (ii) Now consider Kruskal coordinates, in which the Schwarzschild $t$ and $r$ are replaced by $U$ and $V$, defined for $r>2 M$ by

  $$\begin{aligned} &U \equiv\left(\frac{r}{2 M}-1\right)^{1 / 2} e^{r /(4 M)} \cosh \left(\frac{t}{4 M}\right) \\ &V \equiv\left(\frac{r}{2 M}-1\right)^{1 / 2} e^{r /(4 M)} \sinh \left(\frac{t}{4 M}\right) \end{aligned}$$

  and for $r<2 M$ by

  $$\begin{aligned} &U \equiv\left(1-\frac{r}{2 M}\right)^{1 / 2} e^{r /(4 M)} \sinh \left(\frac{t}{4 M}\right) \\ &V \equiv\left(1-\frac{r}{2 M}\right)^{1 / 2} e^{r /(4 M)} \cosh \left(\frac{t}{4 M}\right) \end{aligned}$$

  Given that the metric in these coordinates is

  $$d s^{2}=\frac{32 M^{3}}{r} e^{-r /(2 M)}\left(-d V^{2}+d U^{2}\right)+r^{2}\left(d \theta^{2}+\sin ^{2} \theta d \phi^{2}\right),$$

  where $r=r(U, V)$ is defined implicitly by

  $$\left(\frac{r}{2 M}-1\right) e^{r /(2 M)}=U^{2}-V^{2}$$

  sketch the Kruskal diagram, indicating the positions of the singularity at $r=0$, the event horizon at $r=2 M$, and general lines of constant $r$ and of constant $t$.

  comment

• Paper 4, Section II, B

  General Relativity | Part II, 2012

  The metric for a homogenous isotropic universe, in comoving coordinates, can be written as

  $$d s^{2}=-d t^{2}+a^{2}\left\{d r^{2}+f^{2}\left[d \theta^{2}+\sin ^{2} \theta d \phi^{2}\right]\right\}$$

  where $a=a(t)$ and $f=f(r)$ are some functions.

  Write down expressions for the Hubble parameter $H$ and the deceleration parameter $q$ in terms of $a(\eta)$ and $h \equiv d \log a / d \eta$, where $\eta$ is conformal time, defined by $d \eta=a^{-1} d t$.

  The universe is composed of a perfect fluid of density $\rho$ and pressure $p=(\gamma-1) \rho$, where $\gamma$ is a constant. Defining $\Omega=\rho / \rho_{c}$, where $\rho_{c}=3 H^{2} / 8 \pi G$, show that

  $$\frac{k}{h^{2}}=\Omega-1, \quad q=\alpha \Omega, \quad \frac{d \Omega}{d \eta}=2 q h(\Omega-1)$$

  where $k$ is the curvature parameter $(k=+1,0$ or $-1)$ and $\alpha \equiv \frac{1}{2}(3 \gamma-2)$. Hence deduce that

  $$\frac{d \Omega}{d a}=\frac{2 \alpha}{a} \Omega(\Omega-1)$$

  and

  $$\Omega=\frac{1}{1-A a^{2 \alpha}}$$

  where $A$ is a constant. Given that $A=\frac{k}{2 G M}$, sketch curves of $\Omega$ against $a$ in the case when $\gamma>2 / 3$.

  [You may assume an Einstein equation, for the given metric, in the form

  $$\frac{h^{2}}{a^{2}}+\frac{k}{a^{2}}=\frac{8}{3} \pi G \rho$$

  and the energy conservation equation

  $$\left.\frac{d \rho}{d t}+3 H(\rho+p)=0 .\right]$$

  comment

Geometry and Groups

• Paper 1, Section I, G

  Geometry and Groups | Part II, 2012

  Let $G$ be a crystallographic group of the Euclidean plane. Define the lattice and the point group of $G$. Suppose that the lattice for $G$ is $\{(k, 0): k \in \mathbb{Z}\}$. Show that there are five different possibilities for the point group. Show that at least one of these point groups can arise from two groups $G$ that are not conjugate in the group of all isometries of the Euclidean plane.

  comment

• Paper 1, Section II, G

  Geometry and Groups | Part II, 2012

  Define the axis of a loxodromic Möbius transformation acting on hyperbolic 3-space.

  When do two loxodromic transformations commute? Justify your answer.

  Let $G$ be a Kleinian group that contains a loxodromic transformation. Show that the fixed point of any loxodromic transformation in $G$ lies in the limit set of $G$. Prove that the set of such fixed points is dense in the limit set. Give examples to show that the set of such fixed points can be equal to the limit set or a proper subset.

  comment

• Paper 2, Section I, G

  Geometry and Groups | Part II, 2012

  Define the modular group acting on the upper half-plane. Explain briefly why it acts discontinuously and describe a fundamental domain. You should prove that the region which you describe is a fundamental domain.

  comment

• Paper 3, Section I, G

  Geometry and Groups | Part II, 2012

  Let $A$ be a Möbius transformation acting on the Riemann sphere. Show that, if $A$ is not loxodromic, then there is a disc $\Delta$ in the Riemann sphere with $A(\Delta)=\Delta$. Describe all such discs for each Möbius transformation $A$.

  Hence, or otherwise, show that the group $G$ of Möbius transformations generated by

  $$A: z \mapsto i z \quad \text { and } \quad B: z \mapsto 2 z$$

  does not map any disc onto itself.

  Describe the set of points of the Riemann sphere at which $G$ acts discontinuously. What is the quotient of this set by the action of $G$ ?

  comment

• Paper 4, Section I, G

  Geometry and Groups | Part II, 2012

  Explain briefly how to extend a Möbius transformation

  $$T: z \mapsto \frac{a z+b}{c z+d} \quad \text { with } a d-b c=1$$

  from the boundary of the upper half-space $\mathbb{R}_{+}^{3}$ to give a hyperbolic isometry $\widetilde{T}$of the upper half-space. Write down explicitly the extension of the transformation $z \mapsto \lambda^{2} z$ for any constant $\lambda \in \mathbb{C} \backslash\{0\}$.

  Show that, if $\tilde{T}$ has an axis, which is a hyperbolic line that is mapped onto itself by $\tilde{T}$ with the orientation preserved, then $\widetilde{T}$moves each point of this axis by the same hyperbolic distance, $\ell$ say. Prove that

  $$\ell=2|\log | \frac{1}{2}\left(a+d+\sqrt{(a+d)^{2}-4}\right)||$$

  comment

• Paper 4, Section II, $12 \mathrm{G}$

  Geometry and Groups | Part II, 2012

  Define the Hausdorff dimension of a subset of the Euclidean plane.

  Let $\Delta$ be a closed disc of radius $r_{0}$ in the Euclidean plane. Define a sequence of sets $K_{n} \subseteq \Delta, n=1,2, \ldots$, as follows: $K_{1}=\Delta$ and for each $n \geqslant 1$ a subset $K_{n+1} \subset K_{n}$ is produced by replacing each component disc $\Gamma$ of $K_{n}$ by three disjoint, closed discs inside $\Gamma$ with radius at most $c_{n}$ times the radius of $\Gamma$. Let $K$ be the intersection of the sets $K_{n}$. Show that if the factors $c_{n}$ converge to a limit $c$ with $0<c<1$, then the Hausdorff dimension of $K$ is at most $\log \frac{1}{3} / \log c$.

  comment

Graph Theory

• Paper 1, Section II, F

  Graph Theory | Part II, 2012

  State Markov's inequality and Chebyshev's inequality.

  Let $\mathcal{G}^{(2)}(n, p)$ denote the probability space of bipartite graphs with vertex classes $U=\{1,2, \ldots, n\}$ and $V=\{-1,-2, \ldots,-n\}$, with each possible edge $u v(u \in U,$, $v \in V)$ present, independently, with probability $p$. Let $X$ be the number of subgraphs of $G \in \mathcal{G}^{(2)}(n, p)$ that are isomorphic to the complete bipartite graph $K_{2,2}$. Write down $\mathbb{E} X$ and $\operatorname{Var}(X)$. Hence show that $p=1 / n$ is a threshold for $G \in \mathcal{G}^{(2)}(n, p)$ to contain $K_{2,2}$, in the sense that if $n p \rightarrow \infty$ then a. e. $G \in \mathcal{G}^{(2)}(n, p)$ contains a $K_{2,2}$, whereas if $n p \rightarrow 0$ then a. e. $G \in \mathcal{G}^{(2)}(n, p)$ does not contain a $K_{2,2}$.

  By modifying a random $G \in \mathcal{G}^{(2)}(n, p)$ for suitably chosen $p$, show that, for each $n$, there exists a bipartite graph $H$ with $n$ vertices in each class such that $K_{2,2} \not \subset H$ but $e(H) \geqslant \frac{3}{4}\left(\frac{n}{\sqrt[3]{n-1}}\right)^{2} .$

  comment

• Paper 2, Section II, F

  Graph Theory | Part II, 2012

  Let $G$ be a $k$-connected graph $(k \geqslant 2)$. Let $v \in G$ and let $U \subset V(G) \backslash\{v\}$ with $|U| \geqslant k$. Show that $G$ contains $k$ paths from $v$ to $U$ with any two having only the vertex $v$ in common.

  [No form of Menger's theorem or of the Max-Flow-Min-Cut theorem may be assumed without proof.]

  Deduce that $G$ must contain a cycle of length at least $k$.

  Suppose further that $G$ has no independent set of vertices of size $>k$. Show that $G$ is Hamiltonian.

  [Hint. If not, let $C$ be a cycle of maximum length in $G$ and let $v \in V(G) \backslash V(C)$; consider the set of vertices on $C$ immediately preceding the endvertices of a collection of $k$ paths from $v$ to $C$ that have only the vertex $v$ in common.]

  comment

• Paper 3, Section II, $17 \mathrm{~F}$

  Graph Theory | Part II, 2012

  Let $H$ be a graph with at least one edge. Define ex $(n ; H)$, where $n$ is an integer with $n \geqslant|H|$. Without assuming the Erdős-Stone theorem, show that the sequence $\operatorname{ex}(n ; H) /\left(\begin{array}{l}n \\ 2\end{array}\right)$ converges as $n \rightarrow \infty$.

  State precisely the Erdős-Stone theorem. Hence determine, with justification, $\lim _{n \rightarrow \infty} \operatorname{ex}(n ; H) /\left(\begin{array}{c}n \\ 2\end{array}\right) .$

  Let $K$ be another graph with at least one edge. For each integer $n$ such that $n \geqslant \max \{|H|,|K|\}$, let

  $$f(n)=\max \{e(G):|G|=n ; H \not \subset G \text { and } K \not \subset G\}$$

  and let

  $$g(n)=\max \{e(G):|G|=n ; H \not \subset G \text { or } K \not \subset G\} .$$

  Find, with justification, $\lim _{n \rightarrow \infty} f(n) /\left(\begin{array}{l}n \\ 2\end{array}\right)$ and $\lim _{n \rightarrow \infty} g(n) /\left(\begin{array}{l}n \\ 2\end{array}\right)$.

  comment

• Paper 4, Section II, F

  Graph Theory | Part II, 2012

  (a) Show that every finite tree of order at least 2 has a leaf. Hence, or otherwise, show that a tree of order $n \geqslant 1$ must have precisely $n-1$ edges.

  (b) Let $G$ be a graph. Explain briefly why $|G| / \alpha(G) \leqslant \chi(G) \leqslant \Delta(G)+1$.

  Let $k=\chi(G)$, and assume $k \geqslant 2$. By induction on $|G|$, or otherwise, show that $G$ has a subgraph $H$ with $\delta(H) \geqslant k-1$. Hence, or otherwise, show that if $T$ is a tree of order $k$ then $T \subseteq G$.

  (c) Let $s, t \geqslant 2$ be integers, let $n=(s-1)(t-1)+1$ and let $T$ be a tree of order $t$. Show that whenever the edges of the complete graph $K_{n}$ are coloured blue and yellow then it must contain either a blue $K_{s}$ or a yellow $T$.

  Does this remain true if $K_{n}$ is replaced by $K_{n-1}$ ? Justify your answer.

  [The independence number $\alpha(G)$ of a graph $G$ is the size of the largest set $W \subseteq V(G)$ of vertices such that no edge of $G$ joins two points of $W$. Recall that $\chi(G)$ is the chromatic number and $\delta(G), \Delta(G)$ are respectively the minimal/maximal degrees of vertices in $G$.]

  comment

Integrable Systems

• Paper 1, Section II, D

  Integrable Systems | Part II, 2012

  State the Arnold-Liouville theorem.

  Consider an integrable system with six-dimensional phase space, and assume that $\nabla \wedge \mathbf{p}=0$ on any Liouville tori $p_{i}=p_{i}\left(q_{j}, c_{j}\right)$, where $\nabla=\left(\partial / \partial q_{1}, \partial / \partial q_{2}, \partial / \partial q_{3}\right)$.

  (a) Define the action variables and use Stokes' theorem to show that the actions are independent of the choice of the cycles.

  (b) Define the generating function, and show that the angle coordinates are periodic with period $2 \pi$.

  comment

• Paper 2, Section II, D

  Integrable Systems | Part II, 2012

  Consider the $\mathrm{KdV}$ equation for the function $u(x, t)$

  $$u_{t}=6 u u_{x}-u_{x x x}$$

  (a) Write equation (1) in the Hamiltonian form

  $$u_{t}=\frac{\partial}{\partial x} \frac{\delta H[u]}{\delta u}$$

  where the functional $H[u]$ should be given. Use equation (1), together with the boundary conditions $u \rightarrow 0$ and $u_{x} \rightarrow 0$ as $|x| \rightarrow \infty$, to show that $\int_{\mathbb{R}} u^{2} d x$ is independent of $t$.

  (b) Use the Gelfand-Levitan-Marchenko equation

  $$K(x, y)+F(x+y)+\int_{x}^{\infty} K(x, z) F(z+y) d z=0$$

  to find the one soliton solution of the KdV equation, i.e.

  $$u(x, t)=-\frac{4 \beta \chi \exp (-2 \chi x)}{\left[1+\frac{\beta}{2 \chi} \exp (-2 \chi x)\right]^{2}}$$

  [Hint. Consider $F(x)=\beta \exp (-\chi x)$, with $\beta=\beta_{0} \exp \left(8 \chi^{3} t\right)$, where $\beta_{0}, \chi$ are constants, and $t$ should be regarded as a parameter in equation (2). You may use any facts about the Inverse Scattering Transform without proof.]

  comment

• Paper 3, Section II, D

  Integrable Systems | Part II, 2012

  Consider a one-parameter group of transformations acting on $\mathbb{R}^{4}$

  $$(x, y, t, u) \longrightarrow(\exp (\epsilon \alpha) x, \exp (\epsilon \beta) y, \exp (\epsilon \gamma) t, \exp (\epsilon \delta) u)$$

  where $\epsilon$ is a group parameter and $(\alpha, \beta, \gamma, \delta)$ are constants.

  (a) Find a vector field $W$ which generates this group.

  (b) Find two independent Lie point symmetries $S_{1}$ and $S_{2}$ of the $\mathrm{PDE}$

  $$\left(u_{t}-u u_{x}\right)_{x}=u_{y y}, \quad u=u(x, y, t),$$

  which are of the form (1).

  (c) Find three functionally-independent invariants of $S_{1}$, and do the same for $S_{2}$. Find a non-constant function $G=G(x, y, t, u)$ which is invariant under both $S_{1}$ and $S_{2}$.

  (d) Explain why all the solutions of (2) that are invariant under a two-parameter group of transformations generated by vector fields

  $$W=u \frac{\partial}{\partial u}+x \frac{\partial}{\partial x}+\frac{1}{2} y \frac{\partial}{\partial y}, \quad V=\frac{\partial}{\partial y},$$

  are of the form $u=x F(t)$, where $F$ is a function of one variable. Find an ODE for $F$ characterising these group-invariant solutions.

  comment

Linear Analysis

• Paper 1, Section II, G

  Linear Analysis | Part II, 2012

  What is meant by the dual $X^{*}$ of a normed space $X$ ? Show that $X^{*}$ is a Banach space.

  Let $X=C^{1}(0,1)$, the space of functions $f:(0,1) \rightarrow \mathbb{R}$ possessing a bounded, continuous first derivative. Endow $X$ with the sup norm $\|f\|_{\infty}=\sup _{x \in(0,1)}|f(x)|$. Which of the following maps $T: X \rightarrow \mathbb{R}$ are elements of $X^{*}$ ? Give brief justifications or counterexamples as appropriate.

  1. $T f=f\left(\frac{1}{2}\right)$;
  2. $T f=\|f\|_{\infty}$
  3. $T f=\int_{0}^{1} f(x) d x$
  4. $T f=f^{\prime}\left(\frac{1}{2}\right)$.

  Now suppose that $X$ is a (real) Hilbert space. State and prove the Riesz representation theorem. Describe the natural $\operatorname{map} X \rightarrow X^{* *}$ and show that it is surjective.

  [All normed spaces are over $\mathbb{R}$. You may assume that if $Y$ is a closed subspace of a Hilbert space $X$ then $\left.X=Y \oplus Y^{\perp} .\right]$

  comment

• Paper 2, Section II, G

  Linear Analysis | Part II, 2012

  What is meant by a normal topological space? State and prove Urysohn's lemma.

  Let $X$ be a normal topological space and let $S \subseteq X$ be closed. Show that there is a continuous function $f: X \rightarrow[0,1]$ with $f^{-1}(0)=S$ if, and only if, $S$ is a countable intersection of open sets.

  [Hint. If $S=\bigcap_{n=1}^{\infty} U_{n}$ then consider $\sum_{n=1}^{\infty} 2^{-n} f_{n}$, where the functions $f_{n}: X \rightarrow[0,1]$ are supplied by an appropriate application of Urysohn's lemma.]

  comment

• Paper 3, Section II, G

  Linear Analysis | Part II, 2012

  State the closed graph theorem.

  (i) Let $X$ be a Banach space and $Y$ a vector space. Suppose that $Y$ is endowed with two norms $\|\cdot\|_{1}$ and $\|\cdot\|_{2}$ and that there is a constant $c>0$ such that $\|y\|_{2} \geqslant c\|y\|_{1}$ for all $y \in Y$. Suppose that $Y$ is a Banach space with respect to both norms. Suppose that $T: X \rightarrow Y$ is a linear operator, and that it is bounded when $Y$ is endowed with the $\|\cdot\|_{1}$ norm. Show that it is also bounded when $Y$ is endowed with the $\|\cdot\|_{2}$ norm.

  (ii) Suppose that $X$ is a normed space and that $\left(x_{n}\right)_{n=1}^{\infty} \subseteq X$ is a sequence with $\sum_{n=1}^{\infty}\left|f\left(x_{n}\right)\right|<\infty$ for all $f$ in the dual space $X^{*}$. Show that there is an $M$ such that

  $$\sum_{n=1}^{\infty}\left|f\left(x_{n}\right)\right| \leqslant M\|f\|$$

  for all $f \in X^{*}$.

  (iii) Suppose that $X$ is the space of bounded continuous functions $f: \mathbb{R} \rightarrow \mathbb{R}$ with the sup norm, and that $Y \subseteq X$ is the subspace of continuously differentiable functions with bounded derivative. Let $T: Y \rightarrow X$ be defined by $T f=f^{\prime}$. Show that the graph of $T$ is closed, but that $T$ is not bounded.

  comment

• Paper 4, Section II, G

  Linear Analysis | Part II, 2012

  Let $X$ be a Banach space and suppose that $T: X \rightarrow X$ is a bounded linear operator. What is an eigenvalue of $T ?$ What is the spectrum $\sigma(T)$ of $T ?$

  Let $X=C[0,1]$ be the space of continuous real-valued functions $f:[0,1] \rightarrow \mathbb{R}$ endowed with the sup norm. Define an operator $T: X \rightarrow X$ by

  $$T f(x)=\int_{0}^{1} G(x, y) f(y) d y$$

  where

  $$G(x, y)= \begin{cases}y(x-1) & \text { if } y \leqslant x \\ x(y-1) & \text { if } x \leqslant y\end{cases}$$

  Prove the following facts about $T$ :

  (i) $T f(0)=T f(1)=0$ and the second derivative $(T f)^{\prime \prime}(x)$ is equal to $f(x)$ for $x \in(0,1)$;

  (ii) $T$ is compact;

  (iii) $T$ has infinitely many eigenvalues;

  (iv) 0 is not an eigenvalue of $T$;

  (v) $0 \in \sigma(T)$.

  [The Arzelà-Ascoli theorem may be assumed without proof.]

  comment

Logic and Set Theory

• Paper 1, Section II, H

  Logic and Set Theory | Part II, 2012

  State Zorn's lemma, and show how it may be deduced from the Axiom of Choice using the Bourbaki-Witt theorem (which should be clearly stated but not proved).

  Show that, if $a$ and $b$ are distinct elements of a distributive lattice $L$, there is a lattice homomorphism $f: L \rightarrow\{0,1\}$ with $f(a) \neq f(b)$. Indicate briefly how this result may be used to prove the completeness theorem for propositional logic.

  comment

• Paper 2, Section II, H

  Logic and Set Theory | Part II, 2012

  Explain what is meant by a substructure of a $\Sigma$-structure $A$, where $\Sigma$ is a first-order signature (possibly including both predicate symbols and function symbols). Show that if $B$ is a substructure of $A$, and $\phi$ is a first-order formula over $\Sigma$ with $n$ free variables, then $[\phi]_{B}=[\phi]_{A} \cap B^{n}$ if $\phi$ is quantifier-free. Show also that $[\phi]_{B} \subseteq[\phi]_{A} \cap B^{n}$ if $\phi$ is an existential formula (that is, one of the form $\left(\exists x_{1}, \ldots, x_{m}\right) \psi$ where $\psi$ is quantifier-free), and $[\phi]_{B} \supseteq[\phi]_{A} \cap B^{n}$ if $\phi$ is a universal formula. Give examples to show that the two latter inclusions can be strict.

  Show also that

  (a) if $T$ is a first-order theory whose axioms are all universal sentences, then any substructure of a $T$-model is a $T$-model;

  (b) if $T$ is a first-order theory such that every first-order formula $\phi$ is $T$-provably equivalent to a universal formula (that is, $T \vdash(\phi \Leftrightarrow \psi)$ for some universal $\psi$ ), and $B$ is a sub-T-model of a $T$-model $A$, then $[\phi]_{B}=[\phi]_{A} \cap B^{n}$ for every first-order formula $\phi$ with $n$ free variables.

  comment

• Paper 3, Section II, H

  Logic and Set Theory | Part II, 2012

  Write down either the synthetic or the recursive definitions of ordinal addition and multiplication. Using your definitions, give proofs or counterexamples for the following statements:

  (i) For all $\alpha, \beta$ and $\gamma$, we have $\alpha \cdot(\beta+\gamma)=\alpha \cdot \beta+\alpha \cdot \gamma$.

  (ii) For all $\alpha, \beta$ and $\gamma$, we have $(\alpha+\beta) \cdot \gamma=\alpha \cdot \gamma+\beta \cdot \gamma$.

  (iii) For all $\alpha$ and $\beta$ with $\beta>0$, there exist $\gamma$ and $\delta$ with $\delta<\beta$ and $\alpha=\beta \cdot \gamma+\delta$.

  (iv) For all $\alpha$ and $\beta$ with $\beta>0$, there exist $\gamma$ and $\delta$ with $\delta<\beta$ and $\alpha=\gamma \cdot \beta+\delta$.

  (v) For every $\alpha$, either there exists a cofinal map $f: \omega \rightarrow \alpha$ (that is, one such that $\left.\alpha=\bigcup\left\{f(n)^{+} \mid n \in \omega\right\}\right)$, or there exists $\beta$ such that $\alpha=\omega_{1} \cdot \beta .$

  comment

• Paper 4, Section II, H

  Logic and Set Theory | Part II, 2012

  State and prove Hartogs' lemma. [You may assume the result that any well-ordered set is isomorphic to a unique ordinal.]

  Let $a$ and $b$ be sets such that there is a bijection $a \sqcup b \rightarrow a \times b$. Show, without assuming the Axiom of Choice, that there is either a surjection $b \rightarrow a$ or an injection $b \rightarrow a$. By setting $b=\gamma(a)$ (the Hartogs ordinal of $a$ ) and considering $(a \sqcup b) \times(a \sqcup b)$, show that the assertion 'For all infinite cardinals $m$, we have $m^{2}=m^{\prime}$ implies the Axiom of Choice. [You may assume the Cantor-Bernstein theorem.]

  comment

Mathematical Biology

• Paper 1, Section I, $\mathbf{6 C}$

  Mathematical Biology | Part II, 2012

  Krill is the main food source for baleen whales. The following model has been proposed for the coupled evolution of populations of krill and whales, with $x(t)$ being the number of krill and $y(t)$ being the number of whales:

  $$\begin{array}{r} \frac{d x}{d t}=r x\left(1-\frac{x}{K}\right)-a x y \\ \frac{d y}{d t}=s y\left(1-\frac{y}{b x}\right) \end{array}$$

  where $r, s, a, b$ and $K$ are positive constants.

  Give a biological interpretation for the form of the two differential equations.

  Show that a steady state is possible with $x>0$ and $y>0$ and write down expressions for the steady-state values of $x$ and $y$.

  Determine whether this steady state is stable.

  comment

• Paper 2, Section I, $\mathbf{6 C}$

  Mathematical Biology | Part II, 2012

  Consider a birth-death process in which the birth rate per individual is $\lambda$ and the death rate per individual in a population of size $n$ is $\beta n$.

  Let $P(n, t)$ be the probability that the population has size $n$ at time $t$. Write down the master equation for the system, giving an expression for $\partial P(n, t) / \partial t$.

  Show that

  $$\frac{d}{d t}\langle n\rangle=\lambda\langle n\rangle-\beta\left\langle n^{2}\right\rangle$$

  where $\langle.\rangle$ denotes the mean.

  Deduce that in a steady state $\langle n\rangle \leqslant \lambda / \beta$.

  comment

• Paper 2, Section II, C

  Mathematical Biology | Part II, 2012

  A population of blowflies is modelled by the equation

  $$\frac{d x}{d t}=R(x(t-T))-k x(t)$$

  where $k$ is a constant death rate and $R$ is a function of one variable such that $R(z)>0$ for $z>0$, with $R(z) \sim \beta z$ as $z \rightarrow 0$ and $R(z) \rightarrow 0$ as $z \rightarrow \infty$. The constants $T, k$ and $\beta$ are all positive, with $\beta>k$. Give a brief biological motivation for the term $R(x(t-T))$, in which you explain both the form of the function $R$ and the appearance of a delay time $T$.

  A suitable model for $R(z)$ is $\beta z \exp (-z / d)$, where $d$ is a positive constant. Show that in this case there is a single steady state of the system with non-zero population, i.e. with $x(t)=x_{s}>0$, with $x_{s}$ constant.

  Now consider the stability of this steady state. Show that if $x(t)=x_{s}+y(t)$, with $y(t)$ small, then $y(t)$ satisfies a delay differential equation of the form

  $$\frac{d y}{d t}=-k y(t)+B y(t-T),$$

  where $B$ is a constant to be determined. Show that $y(t)=e^{s t}$ is a solution of (2) if $s=-k+B e^{-s T}$. If $s=\sigma+i \omega$, where $\sigma$ and $\omega$ are both real, write down two equations relating $\sigma$ and $\omega$.

  Deduce that the steady state is stable if $|B|<k$. Show that, for this particular model for $R,|B|>k$ is possible only if $B<0$.

  By considering $B$ decreasing from small negative values, show that an instability will appear when $|B|>\left[k^{2}+\frac{g(k T)^{2}}{T^{2}}\right]^{1 / 2}$, where $\pi / 2<g(k T)<\pi$.

  Deduce that the steady state $x_{s}$ of (1) is unstable if

  $$\beta>k \exp \left[\left(1+\frac{\pi^{2}}{k^{2} T^{2}}\right)^{1 / 2}+1\right]$$

  comment

• Paper 3, Section I, C

  Mathematical Biology | Part II, 2012

  Consider a model of insect dispersal in two dimensions given by

  $$\frac{\partial C}{\partial t}=\frac{1}{r} \frac{\partial}{\partial r}\left(r D C \frac{\partial C}{\partial r}\right)$$

  where $r$ is a radial coordinate, $t$ is time, $C(r, t)$ is the density of insects and $D$ is a constant coefficient such that $D C$ is a diffusivity.

  Show that under suitable assumptions

  $$2 \pi \int_{0}^{\infty} r C d r=N$$

  where $N$ is constant, and interpret this condition.

  Suppose that after a long time the form of $C$ depends only on $r, t, D$ and $N$ (and is thus independent of any detailed form of the initial condition). Show that there is a solution of the form

  $$C(r, t)=\left(\frac{N}{D t}\right)^{1 / 2} g\left(\frac{r}{(N D t)^{1 / 4}}\right) \text {, }$$

  and deduce that the function $g(\xi)$ satisfies

  $$\frac{d}{d \xi}\left(\xi g \frac{d g}{d \xi}+\frac{1}{4} \xi^{2} g\right)=0$$

  Show that this equation has a continuous solution with $g>0$ for $\xi<\xi_{0}$ and $g=0$ for $\xi \geqslant \xi_{0}$, and determine $\xi_{0}$. Hence determine the area within which $C(r, t)>0$ as a function of $t$.

  comment

• Paper 3, Section II, C

  Mathematical Biology | Part II, 2012

  Consider the two-variable reaction-diffusion system

  $$\begin{gathered} \frac{\partial u}{\partial t}=a-u+u^{2} v+\nabla^{2} u \\ \frac{\partial v}{\partial t}=b-u^{2} v+d \nabla^{2} v \end{gathered}$$

  where $a, b$ and $d$ are positive constants.

  Show that there is one possible spatially homogeneous steady state with $u>0$ and $v>0$ and show that it is stable to small-amplitude spatially homogeneous disturbances provided that $\gamma<\beta$, where

  $$\gamma=\frac{b-a}{b+a} \quad \text { and } \quad \beta=(a+b)^{2}$$

  Now assuming that the condition $\gamma<\beta$ is satisfied, investigate the stability of the homogeneous steady state to spatially varying perturbations by considering the timedependence of disturbances whose spatial form is such that $\nabla^{2} u=-k^{2} u$ and $\nabla^{2} v=-k^{2} v$, with $k$ constant. Show that such disturbances vary as $e^{p t}$, where $p$ is one of the roots of

  $$p^{2}+\left(\beta-\gamma+d k^{2}+k^{2}\right) p+d k^{4}+(\beta-d \gamma) k^{2}+\beta$$

  By comparison with the stability condition for the homogeneous case above, give a simple argument as to why the system must be stable if $d=1$.

  Show that the boundary between stability and instability (as some combination of $\beta, \gamma$ and $d$ is varied) must correspond to $p=0$.

  Deduce that $d \gamma>\beta$ is a necessary condition for instability and, furthermore, that instability will occur for some $k$ if

  $$d>\frac{\beta}{\gamma}\left\{1+\frac{2}{\gamma}+2 \sqrt{\frac{1}{\gamma}+\frac{1}{\gamma^{2}}}\right\} .$$

  Deduce that the value of $k^{2}$ at which instability occurs as the stability boundary is crossed is given by

  $$k^{2}=\sqrt{\frac{\beta}{d}}$$

  comment

• Paper 4, Section I, $\mathbf{6 C}$

  Mathematical Biology | Part II, 2012

  The master equation describing the evolution of the probability $P(n, t)$ that a population has $n$ members at time $t$ takes the form

  $$\frac{\partial P(n, t)}{\partial t}=b(n-1) P(n-1, t)-[b(n)+d(n)] P(n, t)+d(n+1) P(n+1, t)$$

  where the functions $b(n)$ and $d(n)$ are both positive for all $n$.

  From (1) derive the corresponding Fokker-Planck equation in the form

  $$\frac{\partial P(x, t)}{\partial t}=-\frac{\partial}{\partial x}\left\{a_{1}(x) P(x, t)\right\}+\frac{1}{2} \frac{\partial^{2}}{\partial x^{2}}\left\{a_{2}(x) P(x, t)\right\}$$

  making clear any assumptions that you make and giving explicit forms for $a_{1}(x)$ and $a_{2}(x)$.

  Assume that (2) has a steady state solution $P_{s}(x)$ and that $a_{1}(x)$ is a decreasing function of $x$ with a single zero at $x_{0}$. Under what circumstances may $P_{s}(x)$ be approximated by a Gaussian centred at $x_{0}$ and what is the corresponding estimate of the variance?

  comment

Number Fields

• Paper 1, Section II, F

  Number Fields | Part II, 2012

  Let $K$ be a number field, and $\mathcal{O}_{K}$ its ring of integers. Write down a characterisation of the units in $\mathcal{O}_{K}$ in terms of the norm. Without assuming Dirichlet's units theorem, prove that for $K$ a quadratic field the quotient of the unit group by $\{\pm 1\}$ is cyclic (i.e. generated by one element). Find a generator in the cases $K=\mathbb{Q}(\sqrt{-3})$ and $K=\mathbb{Q}(\sqrt{11})$.

  Determine all integer solutions of the equation $x^{2}-11 y^{2}=n$ for $n=-1,5,14$.

  comment

• Paper 2, Section II, F

  Number Fields | Part II, 2012

  Let $K=\mathbb{Q}(\alpha)$ where $\alpha$ is a root of $X^{2}-X+12=0$. Factor the elements 2,3 , $\alpha$ and $\alpha+2$ as products of prime ideals in $\mathcal{O}_{K}$. Hence compute the class group of $K$.

  Show that the equation $y^{2}+y=3\left(x^{5}-4\right)$ has no integer solutions.

  comment

• Paper 4, Section II, F

  Number Fields | Part II, 2012

  Let $K=\mathbb{Q}(\sqrt{p}, \sqrt{q})$ where $p$ and $q$ are distinct primes with $p \equiv q \equiv 3(\bmod 4)$. By computing the relative traces $\operatorname{Tr}_{K / k}(\theta)$ where $k$ runs through the three quadratic subfields of $K$, show that the algebraic integers $\theta$ in $K$ have the form

  $$\theta=\frac{1}{2}(a+b \sqrt{p})+\frac{1}{2}(c+d \sqrt{p}) \sqrt{q}$$

  where $a, b, c, d$ are rational integers. Show further that if $c$ and $d$ are both even then $a$ and $b$ are both even. Hence prove that an integral basis for $K$ is

  $$1, \sqrt{p}, \frac{1+\sqrt{p q}}{2}, \frac{\sqrt{p}+\sqrt{q}}{2} .$$

  Calculate the discriminant of $K$.

  comment

Number Theory

• Paper 1, Section I, I

  Number Theory | Part II, 2012

  Show that the continued fraction for $\sqrt{13}$ is $[3 ; \overline{1,1,1,1,6}]$.

  Hence, or otherwise, find a solution to the equation $x^{2}-13 y^{2}=1$ in positive integers $x$ and $y$. Write down an expression for another solution.

  comment

• Paper 2, Section I, I

  Number Theory | Part II, 2012

  Define the Legendre symbol and the Jacobi symbol.

  State the law of quadratic reciprocity for the Jacobi symbol.

  Compute the value of the Jacobi symbol $\left(\frac{247}{321}\right)$, stating clearly any results you use.

  comment

• Paper 3 , Section II, I

  Number Theory | Part II, 2012

  Let $p$ be an odd prime. Prove that the multiplicative groups $\left(\mathbb{Z} / p^{n} \mathbb{Z}\right)^{\times}$are cyclic for $n \geqslant 2$. [You may assume that the multiplicative group $(\mathbb{Z} / p \mathbb{Z})^{\times}$is cyclic.]

  Find an integer which generates $\left(\mathbb{Z} / 7^{n} \mathbb{Z}\right)^{\times}$for all $n \geqslant 1$, justifying your answer.

  comment

• Paper 3, Section I, I

  Number Theory | Part II, 2012

  Define the discriminant of the binary quadratic form $f(x, y)=a x^{2}+b x y+c y^{2}$.

  Assuming that this form is positive definite, define what it means for $f$ to be reduced.

  Show that there are precisely two reduced positive definite binary quadratic forms of discriminant $-35$.

  comment

• Paper 4, Section I, I

  Number Theory | Part II, 2012

  Define what it means for the composite natural number $N$ to be a pseudoprime to the base $b$.

  Find the number of bases (less than 21) to which 21 is a pseudoprime. [You may, if you wish, assume the Chinese Remainder Theorem.]

  comment

• Paper 4, Section II, I

  Number Theory | Part II, 2012

  Let $f: \mathbb{N} \rightarrow \mathbb{R}$ be a function, where $\mathbb{N}$ denotes the (positive) natural numbers.

  Define what it means for $f$ to be a multiplicative function.

  Prove that if $f$ is a multiplicative function, then the function $g: \mathbb{N} \rightarrow \mathbb{R}$ defined by

  $$g(n)=\sum_{d \mid n} f(d)$$

  is also multiplicative.

  Define the Möbius function $\mu$. Is $\mu$ multiplicative? Briefly justify your answer.

  Compute

  $$\sum_{d \mid n} \mu(d)$$

  for all positive integers $n$.

  Define the Riemann zeta function $\zeta$ for complex numbers $s$ with $\Re(s)>1$.

  Prove that if $s$ is a complex number with $\Re(s)>1$, then

  $$\frac{1}{\zeta(s)}=\sum_{n=1}^{\infty} \frac{\mu(n)}{n^{s}}$$

  comment

Numerical Analysis

• Paper 1, Section II, D

  Numerical Analysis | Part II, 2012

  The Poisson equation $u_{x x}=f$ in the unit interval $\Omega=[0,1], u=0$ on $\partial \Omega$ is discretised with the formula

  $$u_{i-1}+u_{i+1}-2 u_{i}=h^{2} f_{i}$$

  where $1 \leqslant i \leqslant n, u_{i} \approx u(i h)$ and $i h$ are the grid points.

  (i) Define the above system of equations in vector form $A \mathbf{u}=\mathbf{b}$ and describe the relaxed Jacobi method with relaxation parameter $\omega$ for solving this linear system. For $\mathbf{x}^{*}$ and $\mathbf{x}^{(\nu)}$ being the exact solution and the iterated solution respectively, let $\mathbf{e}^{(\nu)}=\mathbf{x}^{(\nu)}-\mathbf{x}^{*}$ be the error and $H_{\omega}$ the iteration matrix, so that

  $$\mathbf{e}^{(\nu+1)}=H_{\omega} \mathbf{e}^{(\nu)}$$

  Express $H_{\omega}$ in terms of the matrix $A$, the diagonal part $D$ of $A$ and $\omega$, and find the eigenvectors $\mathbf{v}_{k}$ and the eigenvalues $\lambda_{k}(\omega)$ of $H_{\omega}$.

  (ii) For $A$ as above, let

  $$\mathbf{e}^{(\nu)}=\sum_{k=1}^{n} a_{k}^{(\nu)} \mathbf{v}_{k}$$

  be the expansion of the error with respect to the eigenvectors of $H_{\omega}$. Derive conditions on $\omega$ such that the method converges for any $n$, and prove that, for any such $\omega$, the rate of convergence of $\mathbf{e}^{(\nu)} \rightarrow 0$ is not faster than $\left(1-c / n^{2}\right)^{\nu}$.

  (iii) Show that, for some $\omega$, the high frequency components $\left(\frac{n+1}{2} \leqslant k \leqslant n\right)$ of the error $\mathbf{e}^{(\nu)}$ tend to zero much faster than $\left(1-c / n^{2}\right)^{\nu}$. Determine the optimal parameter $\omega_{*}$ which provides the largest suppression of the high frequency components per iteration, and find the corresponding attenuation factor $\mu_{*}$ (i.e., the least $\mu_{\omega}$ such that $\left|a_{k}^{(\nu+1)}\right| \leqslant \mu_{\omega}\left|a_{k}^{(\nu)}\right|$ for $\left.\frac{n+1}{2} \leqslant k \leqslant n\right)$.

  comment

• Paper 2, Section II, D

  Numerical Analysis | Part II, 2012

  (i) The diffusion equation

  $$\frac{\partial u}{\partial t}=\frac{\partial^{2} u}{\partial x^{2}}, \quad 0 \leqslant x \leqslant 1, t \geqslant 0$$

  with the initial condition $u(x, 0)=\phi(x), 0 \leqslant x \leqslant 1$, and with zero boundary conditions at $x=0$ and $x=1$, can be solved numerically by the method

  $$u_{m}^{n+1}=u_{m}^{n}+\mu\left(u_{m-1}^{n}-2 u_{m}^{n}+u_{m+1}^{n}\right), \quad m=1,2, \ldots, M, n \geqslant 0$$

  where $\Delta x=1 /(M+1), \mu=\Delta t /(\Delta x)^{2}$, and $u_{m}^{n} \approx u(m \Delta x, n \Delta t)$. Prove that $\mu \leqslant 1 / 2$ implies convergence.

  (ii) By discretising the diffusion equation and employing the same notation as in (i) above, determine [without using Fourier analysis] conditions on $\mu$ and the constant $\alpha$ such that the method

  $$u_{m}^{n+1}-\frac{1}{2}(\mu-\alpha)\left(u_{m-1}^{n+1}-2 u_{m}^{n+1}+u_{m+1}^{n+1}\right)=u_{m}^{n}+\frac{1}{2}(\mu+\alpha)\left(u_{m-1}^{n}-2 u_{m}^{n}+u_{m+1}^{n}\right)$$

  is stable.

  comment

• Paper 3, Section II, D

  Numerical Analysis | Part II, 2012

  The inverse discrete Fourier transform $\mathcal{F}_{n}^{-1}: \mathbb{R}^{n} \rightarrow \mathbb{R}^{n}$ is given by the formula

  $$\mathbf{x}=\mathcal{F}_{n}^{-1} \mathbf{y}, \quad \text { where } \quad x_{l}=\sum_{j=0}^{n-1} \omega_{n}^{j l} y_{j}, \quad l=0, \ldots, n-1$$

  Here, $\omega_{n}=\exp (2 \pi i / n)$ is the primitive root of unity of degree $n$ and $n=2^{p}, p=1,2, \ldots$

  (i) Show how to assemble $\mathbf{x}=\mathcal{F}_{2 m}^{-1} \mathbf{y}$ in a small number of operations if the Fourier transforms of the even and odd parts of $\mathbf{y}$,

  $$\mathbf{x}^{(E)}=\mathcal{F}_{m}^{-1} \mathbf{y}^{(E)}, \quad \mathbf{x}^{(O)}=\mathcal{F}_{m}^{-1} \mathbf{y}^{(O)}$$

  are already known.

  (ii) Describe the Fast Fourier Transform (FFT) method for evaluating $\mathbf{x}$, and draw a relevant diagram for $n=8$.

  (iii) Find the costs of the FFT method for $n=2^{p}$ (only multiplications count).

  (iv) For $n=4$ use the FFT method to find $\mathbf{x}=\mathcal{F}_{4}^{-1} \mathbf{y}$ when:

  (a) $\mathbf{y}=(1,1,-1,-1)$,

  (b) $\mathbf{y}=(1,-1,1,-1)$.

  comment

• Paper 4, Section II, $39 \mathrm{D}$

  Numerical Analysis | Part II, 2012

  (i) Formulate the conjugate gradient method for the solution of a system $A \mathbf{x}=\mathbf{b}$ with $A \in \mathbb{R}^{n \times n}$ and $\mathbf{b} \in \mathbb{R}^{n}, n>0$.

  (ii) Prove that if the conjugate gradient method is applied in exact arithmetic then, for any $\mathbf{x}^{(0)} \in \mathbb{R}^{n}$, termination occurs after at most $n$ iterations.

  (iii) The polynomial $p(x)=x^{m}+\sum_{i=0}^{m-1} c_{i} x^{i}$ is the minimal polynomial of the $n \times n$ matrix $A$ if it is the polynomial of lowest degree that satisfies $p(A)=0 . \quad[$ Note that $m \leqslant n$.] Give an example of a $3 \times 3$ symmetric positive definite matrix with a quadratic minimal polynomial.

  Prove that (in exact arithmetic) the conjugate gradient method requires at most $m$ iterations to calculate the exact solution of $A \mathbf{x}=\mathbf{b}$, where $m$ is the degree of the minimal polynomial of $A$.

  comment

Optimization and Control

• Paper 2, Section II, J

  Optimization and Control | Part II, 2012

  Describe the elements of a generic stochastic dynamic programming equation for the problem of maximizing the expected sum of discounted rewards accrued at times $0,1, \ldots$ What is meant by the positive case? What is specially true in this case that is not true in general?

  An investor owns a single asset which he may sell once, on any of the days $t=0,1, \ldots$. On day $t$ he will be offered a price $X_{t}$. This value is unknown until day $t$, is independent of all other offers, and a priori it is uniformly distributed on $[0,1]$. Offers remain open, so that on day $t$ he may sell the asset for the best of the offers made on days $0, \ldots, t$. If he sells for $x$ on day $t$ then the reward is $x \beta^{t}$. Show from first principles that if $0<\beta<1$ then there exists $\bar{x}$ such that the expected reward is maximized by selling the first day the offer is at least $\bar{x}$.

  For $\beta=4 / 5$, find both $\bar{x}$ and the expected reward under the optimal policy.

  Explain what is special about the case $\beta=1$.

  comment

• Paper 3, Section II, J

  Optimization and Control | Part II, 2012

  A state variable $x=\left(x_{1}, x_{2}\right) \in \mathbb{R}^{2}$ is subject to dynamics

  $$\begin{aligned} &\dot{x}_{1}(t)=x_{2}(t) \\ &\dot{x}_{2}(t)=u(t) \end{aligned}$$

  where $u=u(t)$ is a scalar control variable constrained to the interval $[-1,1]$. Given an initial value $x(0)=\left(x_{1}, x_{2}\right)$, let $F\left(x_{1}, x_{2}\right)$ denote the minimal time required to bring the state to $(0,0)$. Prove that

  $$\max _{u \in[-1,1]}\left\{-x_{2} \frac{\partial F}{\partial x_{1}}-u \frac{\partial F}{\partial x_{2}}-1\right\}=0$$

  Explain how this equation figures in Pontryagin's maximum principle.

  Use Pontryagin's maximum principle to show that, on an optimal trajectory, $u(t)$ only takes the values 1 and $-1$, and that it makes at most one switch between them.

  Show that $u(t)=1,0 \leqslant t \leqslant 2$ is optimal when $x(0)=(2,-2)$.

  Find the optimal control when $x(0)=(7,-2)$.

  comment

• Paper 4, Section II, J

  Optimization and Control | Part II, 2012

  A factory has a tank of capacity $3 \mathrm{~m}^{3}$ in which it stores chemical waste. Each week the factory produces, independently of other weeks, an amount of waste that is equally likely to be 0,1 , or $2 \mathrm{~m}^{3}$. If the amount of waste exceeds the remaining space in the tank then the excess must be specially handled at a cost of $£ C$ per $\mathrm{m}^{3}$. The tank may be emptied or not at the end of each week. Emptying costs $£ D$, plus a variable cost of $£ \alpha$ for each $\mathrm{m}^{3}$ of its content. It is always emptied when it ends the week full.

  It is desired to minimize the average cost per week. Write down equations from which one can determine when it is optimal to empty the tank.

  Find the average cost per week of a policy $\pi$, which empties the tank if and only if its content at the end of the week is 2 or $3 \mathrm{~m}^{3}$.

  Describe the policy improvement algorithm. Explain why, starting from $\pi$, this algorithm will find an optimal policy in at most three iterations.

  Prove that $\pi$ is optimal if and only if $C \geqslant \alpha+(4 / 3) D$.

  comment

Partial Differential Equations

• Paper 1, Section II, B

  Partial Differential Equations | Part II, 2012

  Let $u_{0}: \mathbb{R} \rightarrow \mathbb{R}, u_{0} \in C^{1}(\mathbb{R}), u_{0}(x) \geqslant 0$ for all $x \in \mathbb{R}$. Consider the partial differential equation for $u=u(x, y)$,

  $$4 y u_{x}+3 u_{y}=u^{2}, \quad(x, y) \in \mathbb{R}^{2}$$

  subject to the Cauchy condition $u(x, 0)=u_{0}(x)$.

  i) Compute the solution of the Cauchy problem by the method of characteristics.

  ii) Prove that the domain of definition of the solution contains

  $$(x, y) \in \mathbb{R} \times\left(-\infty, \frac{3}{\sup _{x \in \mathbb{R}}\left(u_{0}(x)\right)}\right)$$

  comment

• Paper 2, Section II, B

  Partial Differential Equations | Part II, 2012

  Consider the elliptic Dirichlet problem on $\Omega \subset \mathbb{R}^{n}, \Omega$ bounded with a smooth boundary:

  $$\Delta u-e^{u}=f \text { in } \Omega, \quad u=u_{D} \text { on } \partial \Omega .$$

  Assume that $u_{D} \in L^{\infty}(\partial \Omega)$ and $f \in L^{\infty}(\Omega)$.

  (i) State the strong Minimum-Maximum Principle for uniformly elliptic operators.

  (ii) Prove that there exists at most one classical solution of the boundary value problem.

  (iii) Assuming further that $f \geqslant 0$ in $\Omega$, use the maximum principle to obtain an upper bound on the solution (assuming that it exists).

  comment

• Paper 3, Section II, 30B

  Partial Differential Equations | Part II, 2012

  Consider the nonlinear partial differential equation for a function $u(x, t), x \in \mathbb{R}^{n}, t>0$,

  $$\begin{array}{r} u_{t}=\Delta u-\alpha|\nabla u|^{2} \\ \text { subject to } \quad u(x, 0)=u_{0}(x) \end{array}$$

  where $u_{0} \in L^{\infty}\left(\mathbb{R}^{n}\right)$.

  (i) Find a transformation $w:=F(u)$ such that $w$ satisfies the heat equation

  $$w_{t}=\Delta w, \quad x \in \mathbb{R}^{n}$$

  if (1) holds for $u$.

  (ii) Use the transformation obtained in (i) (and its inverse) to find a solution to the initial value problem (1), (2).

  [Hint. Use the fundamental solution of the heat equation.]

  (iii) The equation (1) is posed on a bounded domain $\Omega \subseteq \mathbb{R}^{n}$ with smooth boundary, subject to the initial condition (2) on $\Omega$ and inhomogeneous Dirichlet boundary conditions

  $$u=u_{D} \text { on } \partial \Omega$$

  where $u_{D}$ is a bounded function. Use the maximum-minimum principle to prove that there exists at most one classical solution of this boundary value problem.

  comment

• Paper 4, Section II, 30B

  Partial Differential Equations | Part II, 2012

  i) State the Lax-Milgram lemma.

  ii) Consider the boundary value problem

  $$\begin{aligned} \Delta^{2} u-\Delta u+u=f & \text { in } \Omega \\ u=\nabla u \cdot \gamma=0 & \text { on } \partial \Omega \end{aligned}$$

  where $\Omega$ is a bounded domain in $\mathbb{R}^{n}$ with a smooth boundary, $\gamma$ is the exterior unit normal vector to $\partial \Omega$, and $f \in L^{2}(\Omega)$. Show (using the Lax-Milgram lemma) that the boundary value problem has a unique weak solution in the space

  $$H_{0}^{2}(\Omega):=\{u: \Omega \rightarrow \mathbb{R} ; u=\nabla u \cdot \gamma=0 \text { on } \partial \Omega\}$$

  [Hint. Show that

  $$\|\Delta u\|_{L^{2}(\Omega)}^{2}=\sum_{i, j=1}^{n}\left\|\frac{\partial^{2} u}{\partial x_{i} \partial x_{j}}\right\|_{L^{2}(\Omega)}^{2} \quad \text { for all } u \in C_{0}^{\infty}(\Omega)$$

  and then use the fact that $C_{0}^{\infty}(\Omega)$ is dense in $\left.H_{0}^{2}(\Omega) .\right]$

  comment

Principles of Quantum Mechanics

• Paper 1, Section II, A

  Principles of Quantum Mechanics | Part II, 2012

  Let $a$ and $a^{\dagger}$ be the simple harmonic oscillator annihilation and creation operators, respectively. Write down the commutator $\left[a, a^{\dagger}\right]$.

  Consider a new operator $b=c a+s a^{\dagger}$, where $c \equiv \cosh \theta, s \equiv \sinh \theta$ with $\theta$ a real constant. Show that

  $$\left[b, b^{\dagger}\right]=1$$

  Consider the Hamiltonian

  $$H=\epsilon a^{\dagger} a+\frac{1}{2} \lambda\left(a^{\dagger^{2}}+a^{2}\right),$$

  where $\epsilon$ and $\lambda$ are real and such that $\epsilon>\lambda>0$. Assuming that $\epsilon c-\lambda s=E c$ and $\lambda c-\epsilon s=E s$, with $E$ a real constant, show that

  $$[b, H]=E b$$

  Thus, calculate the energy of $b\left|E_{a}\right\rangle$ in terms of $E$ and $E_{a}$, where $E_{a}$ is an eigenvalue of $H$.

  Assuming that $b\left|E_{\min }\right\rangle=0$, calculate $E_{\min }$ in terms of $\lambda, s$ and $c$. Find the possible values of $x=s / c$. Finally, show that the energy eigenvalues of the system are

  $$E_{n}=-\frac{\epsilon}{2}+\left(n+\frac{1}{2}\right) \sqrt{\epsilon^{2}-\lambda^{2}}$$

  comment

• Paper 2, Section II, A

  Principles of Quantum Mechanics | Part II, 2012

  (a) Define the Heisenberg picture of quantum mechanics in relation to the Schrödinger picture. Explain how the two pictures provide equivalent descriptions of physical results.

  (b) Derive the equation of motion for an operator in the Heisenberg picture.

  For a particle of mass $m$ moving in one dimension, the Hamiltonian is

  $$\hat{H}=\frac{\hat{p}^{2}}{2 m}+V(\hat{x})$$

  where $\hat{x}$ and $\hat{p}$ are the position and momentum operators, and the state vector is $|\Psi\rangle$. The eigenstates of $\hat{x}$ and $\hat{p}$ satisfy

  $$\langle x \mid p\rangle=\frac{1}{\sqrt{2 \pi \hbar}} e^{i p x / \hbar}, \quad\left\langle x \mid x^{\prime}\right\rangle=\delta\left(x-x^{\prime}\right), \quad\left\langle p \mid p^{\prime}\right\rangle=\delta\left(p-p^{\prime}\right) .$$

  Use standard methods in the Dirac formalism to show that

  $$\begin{gathered} \left\langle x|\hat{p}| x^{\prime}\right\rangle=-i \hbar \frac{\partial}{\partial x} \delta\left(x-x^{\prime}\right) \\ \left\langle p|\hat{x}| p^{\prime}\right\rangle=i \hbar \frac{\partial}{\partial p} \delta\left(p-p^{\prime}\right) \end{gathered}$$

  Calculate $\left\langle x|\hat{H}| x^{\prime}\right\rangle$ and express $\langle x|\hat{p}| \Psi\rangle,\langle x|\hat{H}| \Psi\rangle$ in terms of the position space wavefunction $\Psi(x)$.

  Write down the momentum space Hamiltonian for the potential

  $$V(\hat{x})=m \omega^{2} \hat{x}^{4} / 2$$

  comment

• Paper 3, Section II, A

  Principles of Quantum Mechanics | Part II, 2012

  Discuss the consequences of indistinguishability for a quantum mechanical state consisting of two identical, non-interacting particles when the particles have (a) spin zero, (b) spin 1/2.

  The stationary Schrödinger equation for one particle in the potential

  $$\frac{-2 e^{2}}{4 \pi \epsilon_{0} r}$$

  has normalised, spherically-symmetric real wavefunctions $\psi_{n}(\mathbf{r})$ and energy eigenvalues $E_{n}$ with $E_{0}<E_{1}<E_{2}<\cdots$. The helium atom can be modelled by considering two non-interacting spin 1/2 particles in the above potential. What are the consequences of the Pauli exclusion principle for the ground state? Write down the two-electron state for this model in the form of a spatial wavefunction times a spin state. Assuming that wavefunctions are spherically-symmetric, find the states of the first excited energy level of the helium atom. What combined angular momentum quantum numbers $J, M$ does each state have?

  Assuming standard perturbation theory results, arrive at a multi-dimensional integral in terms of the one-particle wavefunctions for the first-order correction to the helium ground state energy, arising from the electron-electron interaction.

  comment

• Paper 4, Section II, A

  Principles of Quantum Mechanics | Part II, 2012

  Setting $\hbar=1$, the raising and lowering operators $J_{\pm}=J_{1} \pm i J_{2}$ for angular momentum satisfy

  $$\left[J_{3}, J_{\pm}\right]=\pm J_{\pm}, \quad J_{\pm}|j m\rangle=\sqrt{(j \mp m)(j \pm m+1)}|j m \pm 1\rangle,$$

  where $J_{3}|j m\rangle=m|j m\rangle$. Find the matrix representation $S_{\pm}$for $J_{\pm}$in the basis

  

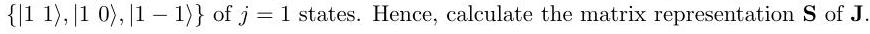
  Suppose that the angular momentum of the state $\mathbf{v}=|1 m\rangle$ is measured in the direction $\mathbf{n}=(0, \sin \theta, \cos \theta)$ to be $+1$. Find the components of $\mathbf{v}$, expressing each component by a single term consisting of products of powers of $\sin (\theta / 2)$ and $\cos (\theta / 2)$ multiplied by constants.

  Suppose that two measurements of a total angular momentum 1 system are made. The first is made in the third direction with value $+1$, and the second measurement is subsequently immediately made in direction $\mathbf{n}$. What is the probability that the second measurement is also $+1$ ?

  comment

Principles of Statistics

• Paper 1, Section II, $28 \mathrm{~K}$

  Principles of Statistics | Part II, 2012

  Prove that, if $T$ is complete sufficient for $\Theta$, and $S$ is a function of $T$, then $S$ is the minimum variance unbiased estimator of $\mathbb{E}(S \mid \Theta)$.

  When the parameter $\Theta$ takes a value $\theta>0$, observables $\left(X_{1}, \ldots, X_{n}\right)$ arise independently from the exponential distribution $\mathcal{E}(\theta)$, having probability density function

  $$p(x \mid \theta)=\theta e^{-\theta x} \quad(x>0) .$$

  Show that the family of distributions

  $$\Theta \sim \operatorname{Gamma}(\alpha, \beta) \quad(\alpha>0, \beta>0),$$

  with probability density function

  $$\pi(\theta)=\frac{\beta^{\alpha}}{\Gamma(\alpha)} \theta^{\alpha-1} e^{-\beta \theta} \quad(\theta>0),$$

  is a conjugate family for Bayesian inference about $\Theta$ (where $\Gamma(\alpha)$ is the Gamma function).

  Show that the expectation of $\Lambda:=\log \Theta$, under prior distribution (1), is $\psi(\alpha)-\log \beta$, where $\psi(\alpha):=(\mathrm{d} / \mathrm{d} \alpha) \log \Gamma(\alpha)$. What is the prior variance of $\Lambda$ ? Deduce the posterior expectation and variance of $\Lambda$, given $\left(X_{1}, \ldots, X_{n}\right)$.

  Let $\tilde{\Lambda}$ denote the limiting form of the posterior expectation of $\Lambda$ as $\alpha, \beta \downarrow 0$. Show that $\tilde{\Lambda}$ is the minimum variance unbiased estimator of $\Lambda$. What is its variance?

  comment

• Paper 2, Section II, $28 \mathrm{~K}$

  Principles of Statistics | Part II, 2012

  Carefully defining all italicised terms, show that, if a sufficiently general method of inference respects both the Weak Sufficiency Principle and the Conditionality Principle, then it respects the Likelihood Principle.

  The position $X_{t}$ of a particle at time $t>0$ has the Normal distribution $\mathcal{N}(0, \phi t)$, where $\phi$ is the value of an unknown parameter $\Phi$; and the time, $T_{x}$, at which the particle first reaches position $x \neq 0$ has probability density function

  $$p_{x}(t)=\frac{|x|}{\sqrt{2 \pi \phi t^{3}}} \exp \left(-\frac{x^{2}}{2 \phi t}\right) \quad(t>0) .$$

  Experimenter $E_{1}$ observes $X_{\tau}$, and experimenter $E_{2}$ observes $T_{\xi}$, where $\tau>0, \xi \neq 0$ are fixed in advance. It turns out that $T_{\xi}=\tau$. What does the Likelihood Principle say about the inferences about $\Phi$ to be made by the two experimenters?

  $E_{1}$ bases his inference about $\Phi$ on the distribution and observed value of $X_{\tau}^{2} / \tau$, while $E_{2}$ bases her inference on the distribution and observed value of $\xi^{2} / T_{\xi}$. Show that these choices respect the Likelihood Principle.

  comment

• Paper 3, Section II, $27 \mathrm{~K}$

  Principles of Statistics | Part II, 2012

  The parameter vector is $\Theta \equiv\left(\Theta_{1}, \Theta_{2}, \Theta_{3}\right)$, with $\Theta_{i}>0, \Theta_{1}+\Theta_{2}+\Theta_{3}=1$. Given $\boldsymbol{\Theta}=\boldsymbol{\theta} \equiv\left(\theta_{1}, \theta_{2}, \theta_{3}\right)$, the integer random vector $\boldsymbol{X}=\left(X_{1}, X_{2}, X_{3}\right)$ has a trinomial distribution, with probability mass function

  $$p(\boldsymbol{x} \mid \boldsymbol{\theta})=\frac{n !}{x_{1} ! x_{2} ! x_{3} !} \theta_{1}^{x_{1}} \theta_{2}^{x_{2}} \theta_{3}^{x_{3}}, \quad\left(x_{i} \geqslant 0, \sum_{i=1}^{3} x_{i}=n\right)$$

  Compute the score vector for the parameter $\Theta^{*}:=\left(\Theta_{1}, \Theta_{2}\right)$, and, quoting any relevant general result, use this to determine $\mathbb{E}\left(X_{i}\right)(i=1,2,3)$.

  Considering (1) as an exponential family with mean-value parameter $\Theta^{*}$, what is the corresponding natural parameter $\boldsymbol{\Phi} \equiv\left(\Phi_{1}, \Phi_{2}\right)$ ?

  Compute the information matrix $I$ for $\Theta^{*}$, which has $(i, j)$-entry

  $$I_{i j}=-\mathbb{E}\left(\frac{\partial^{2} l}{\partial \theta_{i} \partial \theta_{j}}\right) \quad(i, j=1,2)$$

  where $l$ denotes the log-likelihood function, based on $\boldsymbol{X}$, expressed in terms of $\left(\theta_{1}, \theta_{2}\right)$.

  Show that the variance of $\log \left(X_{1} / X_{3}\right)$ is asymptotic to $n^{-1}\left(\theta_{1}^{-1}+\theta_{3}^{-1}\right)$ as $n \rightarrow \infty$. [Hint. The information matrix $I_{\Phi}$ for $\boldsymbol{\Phi}$ is $I^{-1}$ and the dispersion matrix of the maximum likelihood estimator $\widehat{\boldsymbol{\Phi}}$behaves, asymptotically (for $n \rightarrow \infty$ ) as $I_{\Phi}^{-1}$.]

  comment

• Paper 4, Section II, K

  Principles of Statistics | Part II, 2012

  For $i=1, \ldots, n$, the pairs $\left(X_{i}, Y_{i}\right)$ have independent bivariate normal distributions, with $\mathbb{E}\left(X_{i}\right)=\mu_{X}, \mathbb{E}\left(Y_{i}\right)=\mu_{Y}, \operatorname{var}\left(X_{i}\right)=\operatorname{var}\left(Y_{i}\right)=\phi$, and $\operatorname{corr}\left(X_{i}, Y_{i}\right)=\rho$. The means $\mu_{X}, \mu_{Y}$ are known; the parameters $\phi>0$ and $\rho \in(-1,1)$ are unknown.

  Show that the joint distribution of all the variables belongs to an exponential family, and identify the natural sufficient statistic, natural parameter, and mean-value parameter. Hence or otherwise, find the maximum likelihood estimator $\hat{\rho}$ of $\rho$.

  Let $U_{i}:=X_{i}+Y_{i}, V_{i}:=X_{i}-Y_{i}$. What is the joint distribution of $\left(U_{i}, V_{i}\right) ?$

  Show that the distribution of

  $$\frac{(1+\hat{\rho}) /(1-\hat{\rho})}{(1+\rho) /(1-\rho)}$$

  is $F_{n}^{n}$. Hence describe a $(1-\alpha)$-level confidence interval for $\rho$. Briefly explain what would change if $\mu_{X}$ and $\mu_{Y}$ were also unknown.

  [Recall that the distribution $F_{\nu_{2}}^{\nu_{1}}$ is that of $\left(W_{1} / \nu_{1}\right) /\left(W_{2} / \nu_{2}\right)$, where, independently for $j=1$ and $j=2, W_{j}$ has the chi-squared distribution with $\nu_{j}$ degrees of freedom.]

  comment

Probability and Measure

• Paper 1, Section II, J

  Probability and Measure | Part II, 2012

  Carefully state and prove Jensen's inequality for a convex function $c: I \rightarrow \mathbb{R}$, where $I \subseteq \mathbb{R}$ is an interval. Assuming that $c$ is strictly convex, give necessary and sufficient conditions for the inequality to be strict.

  Let $\mu$ be a Borel probability measure on $\mathbb{R}$, and suppose $\mu$ has a strictly positive probability density function $f_{0}$ with respect to Lebesgue measure. Let $\mathcal{P}$ be the family of all strictly positive probability density functions $f$ on $\mathbb{R}$ with respect to Lebesgue measure such that $\log \left(f / f_{0}\right) \in L^{1}(\mu)$. Let $X$ be a random variable with distribution $\mu$. Prove that the mapping

  $$f \mapsto \mathbb{E}\left[\log \frac{f}{f_{0}}(X)\right]$$

  has a unique maximiser over $\mathcal{P}$, attained when $f=f_{0}$ almost everywhere.

  comment

• Paper 2, Section II, J

  Probability and Measure | Part II, 2012

  The Fourier transform of a Lebesgue integrable function $f \in L^{1}(\mathbb{R})$ is given by

  $$\hat{f}(u)=\int_{\mathbb{R}} f(x) e^{i x u} d \mu(x)$$

  where $\mu$ is Lebesgue measure on the real line. For $f(x)=e^{-a x^{2}}, x \in \mathbb{R}, a>0$, prove that

  $$\hat{f}(u)=\sqrt{\frac{\pi}{a}} e^{-\frac{u^{2}}{4 a}}$$

  [You may use properties of derivatives of Fourier transforms without proof provided they are clearly stated, as well as the fact that $\phi(x)=(2 \pi)^{-1 / 2} e^{-x^{2} / 2}$ is a probability density function.]

  State and prove the almost everywhere Fourier inversion theorem for Lebesgue integrable functions on the real line. [You may use standard results from the course, such as the dominated convergence and Fubini's theorem. You may also use that $g_{t} * f(x):=\int_{\mathbb{R}} g_{t}(x-y) f(y) d y$ where $g_{t}(z)=t^{-1} \phi(z / t), t>0$, converges to $f$ in $L^{1}(\mathbb{R})$ as $t \rightarrow 0$ whenever $\left.f \in L^{1}(\mathbb{R}) .\right]$

  The probability density function of a Gamma distribution with scalar parameters $\lambda>0, \alpha>0$ is given by

  $$f_{\alpha, \lambda}(x)=\lambda e^{-\lambda x}(\lambda x)^{\alpha-1} 1_{[0, \infty)}(x)$$

  Let $0<\alpha<1, \lambda>0$. Is $\widehat{f_{\alpha, \lambda}}$ integrable?

  comment

• Paper 3, Section II, J

  Probability and Measure | Part II, 2012

  Carefully state and prove the first and second Borel-Cantelli lemmas.

  Now let $\left(A_{n}: n \in \mathbb{N}\right)$ be a sequence of events that are pairwise independent; that is, $\mathbb{P}\left(A_{n} \cap A_{m}\right)=\mathbb{P}\left(A_{n}\right) \mathbb{P}\left(A_{m}\right)$ whenever $m \neq n$. For $N \geqslant 1$, let $S_{N}=\sum_{n=1}^{N} 1_{A_{n}}$. Show that $\operatorname{Var}\left(S_{N}\right) \leqslant \mathbb{E}\left(S_{N}\right)$.

  Using Chebyshev's inequality or otherwise, deduce that if $\sum_{n=1}^{\infty} \mathbb{P}\left(A_{n}\right)=\infty$, then $\lim _{N \rightarrow \infty} S_{N}=\infty$ almost surely. Conclude that $\mathbb{P}\left(A_{n}\right.$ infinitely often $)=1 .$

  comment

• Paper 4, Section II, J

  Probability and Measure | Part II, 2012

  State and prove Fatou's lemma. [You may use the monotone convergence theorem.]

  For $(E, \mathcal{E}, \mu)$ a measure space, define $L^{1}:=L^{1}(E, \mathcal{E}, \mu)$ to be the vector space of $\mu$ integrable functions on $E$, where functions equal almost everywhere are identified. Prove that $L^{1}$ is complete for the norm $\|\cdot\|_{1}$,

  $$\|f\|_{1}:=\int_{E}|f| d \mu, \quad f \in L^{1} .$$

  [You may assume that $\|\cdot\|_{1}$ indeed defines a norm on $L^{1}$.] Give an example of a measure space $(E, \mathcal{E}, \mu)$ and of a sequence $f_{n} \in L^{1}$ that converges to $f$ almost everywhere such that $f \notin L^{1}$.

  Now let

  $$\mathcal{D}:=\left\{f \in L^{1}: f \geqslant 0 \text { almost everywhere }, \int_{E} f d \mu=1\right\} \text {. }$$

  If a sequence $f_{n} \in \mathcal{D}$ converges to $f$ in $L^{1}$, does it follow that $f \in \mathcal{D} ?$ If $f_{n} \in \mathcal{D}$ converges to $f$ almost everywhere, does it follow that $f \in \mathcal{D}$ ? Justify your answers.

  comment

Representation Theory

• Paper 1, Section II, 19H

  Representation Theory | Part II, 2012

  Write down the character table of $D_{10}$.

  Suppose that $G$ is a group of order 60 containing 24 elements of order 5,20 elements of order 3 and 15 elements of order 2 . Calculate the character table of $G$, justifying your answer.

  [You may assume the formula for induction of characters, provided you state it clearly.]

  comment

• Paper 2, Section II, H

  Representation Theory | Part II, 2012

  Suppose that $G$ is a finite group. Define the inner product of two complex-valued class functions on $G$. Prove that the characters of the irreducible representations of $G$ form an orthonormal basis for the space of complex-valued class functions.

  Suppose that $p$ is a prime and $\mathbb{F}_{p}$ is the field of $p$ elements. Let $G=\mathrm{GL}_{2}\left(\mathbb{F}_{p}\right)$. List the conjugacy classes of $G$.

  Let $G$ act naturally on the set of lines in the space $\mathbb{F}_{p}^{2}$. Compute the corresponding permutation character and show that it is reducible. Decompose this character as a sum of two irreducible characters.

  comment

• Paper 3, Section II, H

  Representation Theory | Part II, 2012

  Show that every complex representation of a finite group $G$ is equivalent to a unitary representation. Let $\chi$ be a character of some finite group $G$ and let $g \in G$. Explain why there are roots of unity $\omega_{1}, \ldots, \omega_{d}$ such that

  $$\chi\left(g^{i}\right)=\omega_{1}^{i}+\cdots+\omega_{d}^{i}$$

  for all integers $i$.

  For the rest of the question let $G$ be the symmetric group on some finite set. Explain why $\chi(g)=\chi\left(g^{i}\right)$ whenever $i$ is coprime to the order of $g$.

  Prove that $\chi(g) \in \mathbb{Z}$.

  State without proof a formula for $\sum_{g \in G} \chi(g)^{2}$ when $\chi$ is irreducible. Is there an irreducible character $\chi$ of degree at least 2 with $\chi(g) \neq 0$ for all $g \in G$ ? Explain your answer.

  [You may assume basic facts about the symmetric group, and about algebraic integers, without proof. You may also use without proof the fact that $\sum_{\substack{1 \leqslant i \leqslant n \\ \operatorname{gcd}(i, n)=1}} \omega^{i} \in \mathbb{Z}$ for any $n$th root of unity $\omega .]$

  comment

• Paper 4, Section II, H

  Representation Theory | Part II, 2012

  Write an essay on the finite-dimensional representations of $\mathrm{SU}(2)$, including a proof of their complete reducibility, and a description of the irreducible representations and the decomposition of their tensor products.

  comment

Riemann Surfaces

• Paper 1, Section II, I

  Riemann Surfaces | Part II, 2012

  (i) Let $f(z)=\sum_{n=1}^{\infty} z^{2^{n}}$. Show that the unit circle is the natural boundary of the function element $(D(0,1), f)$.

  (ii) Let $U=\{z \in \mathbf{C}: \operatorname{Re}(z)>0\} \subset \mathbf{C}$; explain carefully how a holomorphic function $f$ may be defined on $U$ satisfying the equation

  $$\left(f(z)^{2}-1\right)^{2}=z$$

  Let $\mathcal{F}$ denote the connected component of the space of germs $\mathcal{G}$ (of holomorphic functions on $\mathbf{C} \backslash\{0\})$ corresponding to the function element $(U, f)$, with associated holomorphic $\operatorname{map} \pi: \mathcal{F} \rightarrow \mathbf{C} \backslash\{0\}$. Determine the number of points of $\mathcal{F}$ in $\pi^{-1}(w)$ when (a) $w=\frac{1}{2}$, and (b) $w=1$.

  [You may assume any standard facts about analytic continuations that you may need.]

  comment

• Paper 2, Section II, I

  Riemann Surfaces | Part II, 2012

  Let $X$ be the algebraic curve in $\mathbb{C}^{2}$ defined by the polynomial $p(z, w)=z^{d}+w^{d}+1$ where $d$ is a natural number. Using the implicit function theorem, or otherwise, show that there is a natural complex structure on $X$. Let $f: X \rightarrow \mathbb{C}$ be the function defined by $f(a, b)=b$. Show that $f$ is holomorphic. Find the ramification points and the corresponding branching orders of $f$.

  Assume that $f$ extends to a holomorphic map $g: Y \rightarrow \mathbb{C} \cup\{\infty\}$ from a compact Riemann surface $Y$ to the Riemann sphere so that $g^{-1}(\infty)=Y \backslash X$ and that $g$ has no ramification points in $g^{-1}(\infty)$. State the Riemann-Hurwitz formula and apply it to $g$ to calculate the Euler characteristic and the genus of $Y$.

  comment

• Paper 3, Section II, I

  Riemann Surfaces | Part II, 2012

  Let $\Lambda$ be the lattice $\mathbb{Z}+\mathbb{Z} i, X$ the torus $\mathbb{C} / \Lambda$, and $\wp$ the Weierstrass elliptic function with respect to $\Lambda$.

  (i) Let $x \in X$ be the point given by $0 \in \Lambda$. Determine the group

  $$G=\{f \in \operatorname{Aut}(X) \mid f(x)=x\}$$

  (ii) Show that $\wp^{2}$ defines a degree 4 holomorphic map $h: X \rightarrow \mathbb{C} \cup\{\infty\}$, which is invariant under the action of $G$, that is, $h(f(y))=h(y)$ for any $y \in X$ and any $f \in G$. Identify a ramification point of $h$ distinct from $x$ which is fixed by every element of $G$.

  [If you use the Monodromy theorem, then you should state it correctly. You may use the fact that $\operatorname{Aut}(\mathbb{C})=\{a z+b \mid a \in \mathbb{C} \backslash\{0\}, b \in \mathbb{C}\}$, and may assume without proof standard facts about $\wp$.]

  comment

Statistical Modelling

• Paper 1, Section I, K

  Statistical Modelling | Part II, 2012

  Let $Y_{1}, \ldots, Y_{n}$ be independent with $Y_{i} \sim \frac{1}{n_{i}} \operatorname{Bin}\left(n_{i}, \mu_{i}\right), i=1, \ldots, n$, and

  $$\log \left(\frac{\mu_{i}}{1-\mu_{i}}\right)=x_{i}^{\top} \beta$$

  where $x_{i}$ is a $p \times 1$ vector of regressors and $\beta$ is a $p \times 1$ vector of parameters. Write down the likelihood of the data $Y_{1}, \ldots, Y_{n}$ as a function of $\mu=\left(\mu_{1}, \ldots, \mu_{n}\right)$. Find the unrestricted maximum likelihood estimator of $\mu$, and the form of the maximum likelihood estimator $\hat{\mu}=\left(\hat{\mu}_{1}, \ldots, \hat{\mu}_{n}\right)$ under the logistic model (1).

  Show that the deviance for a comparison of the full (saturated) model to the generalised linear model with canonical link (1) using the maximum likelihood estimator $\hat{\beta}$ can be simplified to

  $$D(y ; \hat{\mu})=-2 \sum_{i=1}^{n}\left[n_{i} y_{i} x_{i}^{\top} \hat{\beta}-n_{i} \log \left(1-\hat{\mu}_{i}\right)\right]$$

  Finally, obtain an expression for the deviance residual in this generalised linear model.

  comment

• Paper 1, Section II, K

  Statistical Modelling | Part II, 2012

  The treatment for a patient diagnosed with cancer of the prostate depends on whether the cancer has spread to the surrounding lymph nodes. It is common to operate on the patient to obtain samples from the nodes which can then be analysed under a microscope. However it would be preferable if an accurate assessment of nodal involvement could be made without surgery. For a sample of 53 prostate cancer patients, a number of possible predictor variables were measured before surgery. The patients then had surgery to determine nodal involvement. We want to see if nodal involvement can be accurately predicted from the available variables and determine which ones are most important. The variables take the values 0 or 1 .

  $r$ An indicator $0=$ no $/ 1=$ yes of nodal involvement.

  aged The patient's age, split into less than $60(=0)$ and 60 or over $(=1)$.

  stage A measurement of the size and position of the tumour observed by palpation with the fingers. A serious case is coded as 1 and a less serious case as 0 .

  grade Another indicator of the seriousness of the cancer which is determined by a pathology reading of a biopsy taken by needle before surgery. A value of 1 indicates a more serious case of cancer.

  xray Another measure of the seriousness of the cancer taken from an X-ray reading. A value of 1 indicates a more serious case of cancer.

  acid The level of acid phosphatase in the blood serum where $1=$ high and $0=$ low.

  A binomial generalised linear model with a logit link was fitted to the data to predict nodal involvement and the following output obtained:

  

  Part II, 2012 List of Questions

  [TURN OVER (a) Give an interpretation of the coefficient of xray.

  (b) Give the numerical value of the sum of the squared deviance residuals.

  (c) Suppose that the predictors, stage, grade and xray are positively correlated. Describe the effect that this correlation is likely to have on our ability to determine the strength of these predictors in explaining the response.

  (d) The probability of observing a value of $70.252$ under a Chi-squared distribution with 52 degrees of freedom is $0.047$. What does this information tell us about the null model for this data? Justify your answer.

  (e) What is the lowest predicted probability of the nodal involvement for any future patient?

  (f) The first plot in Figure 1 shows the (Pearson) residuals and the fitted values. Explain why the points lie on two curves.

  (g) The second plot in Figure 1 shows the value of $\hat{\beta}-\hat{\beta}_{(i)}$ where $(i)$ indicates that patient $i$ was dropped in computing the fit. The values for each predictor, including the intercept, are shown. Could a single case change our opinion of which predictors are important in predicting the response?

  Figure 1: The plot on the left shows the Pearson residuals and the fitted values. The plot on the right shows the changes in the regression coefficients when a single point is omitted for each predictor.

  comment

• Paper 2, Section I, K

  Statistical Modelling | Part II, 2012

  The purpose of the following study is to investigate differences among certain treatments on the lifespan of male fruit flies, after allowing for the effect of the variable 'thorax length' (thorax) which is known to be positively correlated with lifespan. Data was collected on the following variables:

  longevity lifespan in days

  thorax (body) length in $\mathrm{mm}$

  treat a five level factor representing the treatment groups. The levels were labelled as follows: "00", "10", "80", "11", "81".

  No interactions were found between thorax length and the treatment factor. A linear model with thorax as the covariate, treat as a factor (having the above 5 levels) and longevity as the response was fitted and the following output was obtained. There were 25 males in each of the five groups, which were treated identically in the provision of fresh food.

  Coefficients :

  $\begin{array}{lrrrr} & \text{Estimate} & \text{Std. Error} & \text{t value} & \operatorname{Pr}(>|t|) \\ \text { (Intercept) } & -49.98 & 10.61 & -4.71 & 6.7 e-06 \\ \text { treat10 } & 2.65 & 2.98 & 0.89 & 0.37 \\ \text { treat11 } & -7.02 & 2.97 & -2.36 & 0.02 \\ \text { treat80 } & 3.93 & 3.00 & 1.31 & 0.19 \\ \text { treat81 } & -19.95 & 3.01 & -6.64 & 1.0 e-09 \\ \text { thorax } & 135.82 & 12.44 & 10.92 & <2 \mathrm{e}-16\end{array}$

  Residual standard error: $10.5$ on 119 degrees of freedom

  Multiple R-Squared: $0.656$, Adjusted R-squared: $0.642$

  F-statistics: $45.5$ on 5 and 119 degrees of freedom, p-value: 0

  (a) Assuming the same treatment, how much longer would you expect a fly with a thorax length $0.1 \mathrm{~mm}$ greater than another to live?

  (b) What is the predicted difference in longevity between a male fly receiving treatment treat 10 and treat81 assuming they have the same thorax length?

  (c) Because the flies were randomly assigned to the five groups, the distribution of thorax lengths in the five groups are essentially equal. What disadvantage would the investigators have incurred by ignoring the thorax length in their analysis (i.e., had they done a one-way ANOVA instead)?

  (d) The residual-fitted plot is shown in the left panel of Figure 1 overleaf. Is it possible to determine if the regular residuals or the studentized residuals have been used to construct this plot? Explain.

  (e) The Box-Cox procedure was used to determine a good transformation for this data. The plot of the log-likelihood for $\lambda$ is shown in the right panel of Figure 1 . What transformation should be used to improve the fit and yet retain some interpretability?

  

  Figure 1: Residual-Fitted plot on the left and Box-Cox plot on the right

  comment

• Paper 3, Section I, 5K

  Statistical Modelling | Part II, 2012

  Consider the linear model

  $$Y_{i}=\beta_{0}+\beta_{1} x_{i 1}+\beta_{2} x_{i 2}+\varepsilon_{i}$$

  for $i=1,2, \ldots, n$, where the $\varepsilon_{i}$ are independent and identically distributed with $N\left(0, \sigma^{2}\right)$ distribution. What does it mean for the pair $\beta_{1}$ and $\beta_{2}$ to be orthogonal? What does it mean for all the three parameters $\beta_{0}, \beta_{1}$ and $\beta_{2}$ to be mutually orthogonal? Give necessary and sufficient conditions on $\left(x_{i 1}\right)_{i=1}^{n},\left(x_{i 2}\right)_{i=1}^{n}$ so that $\beta_{0}, \beta_{1}$ and $\beta_{2}$ are mutually orthogonal. If $\beta_{0}, \beta_{1}, \beta_{2}$ are mutually orthogonal, find the joint distribution of the corresponding maximum likelihood estimators $\hat{\beta}_{0}, \hat{\beta}_{1}$ and $\hat{\beta}_{2}$.

  comment

• Paper 4, Section I, K

  Statistical Modelling | Part II, 2012

  Define the concepts of an exponential dispersion family and the corresponding variance function. Show that the family of Poisson distributions with parameter $\lambda>0$ is an exponential dispersion family. Find the corresponding variance function and deduce from it expressions for $E(Y)$ and $\operatorname{Var}(Y)$ when $Y \sim \operatorname{Pois}(\lambda)$. What is the canonical link function in this case?

  comment

• Paper 4, Section II, K

  Statistical Modelling | Part II, 2012

  Let $\left(X_{1}, Y_{1}\right), \ldots,\left(X_{n}, Y_{n}\right)$ be jointly independent and identically distributed with $X_{i} \sim N(0,1)$ and conditional on $X_{i}=x, Y_{i} \sim N(x \theta, 1), i=1,2, \ldots, n$.

  (a) Write down the likelihood of the data $\left(X_{1}, Y_{1}\right), \ldots,\left(X_{n}, Y_{n}\right)$, and find the maximum likelihood estimate $\hat{\theta}$ of $\theta$. [You may use properties of conditional probability/expectation without providing a proof.]

  (b) Find the Fisher information $I(\theta)$ for a single observation, $\left(X_{1}, Y_{1}\right)$.

  (c) Determine the limiting distribution of $\sqrt{n}(\hat{\theta}-\theta)$. [You may use the result on the asymptotic distribution of maximum likelihood estimators, without providing a proof.]

  (d) Give an asymptotic confidence interval for $\theta$ with coverage $(1-\alpha)$ using your answers to (b) and (c).

  (e) Define the observed Fisher information. Compare the confidence interval in part (d) with an asymptotic confidence interval with coverage $(1-\alpha)$ based on the observed Fisher information.

  (f) Determine the exact distribution of $\left(\sum_{i=1}^{n} X_{i}^{2}\right)^{1 / 2}(\hat{\theta}-\theta)$ and find the true coverage probability for the interval in part (e). [Hint. Condition on $X_{1}, X_{2}, \ldots, X_{n}$ and use the following property of conditional expectation: for $U, V$ random vectors, any suitable function $g$, and $x \in \mathbb{R}$,

  $$P\{g(U, V) \leqslant x\}=E[P\{g(U, V) \leqslant x \mid V\}] .]$$

  comment

Statistical Physics

• Paper 1, Section II, C

  Statistical Physics | Part II, 2012

  A meson consists of two quarks, attracted by a linear potential energy

  $$V=\alpha x$$

  where $x$ is the separation between the quarks and $\alpha$ is a constant. Treating the quarks classically, compute the vibrational partition function that arises from the separation of quarks. What is the average separation of the quarks at temperature $T$ ?

  Consider an ideal gas of these mesons that have the orientation of the quarks fixed so the mesons do not rotate. Compute the total partition function of the gas. What is its heat capacity $C_{V}$ ?

  [Note: $\int_{-\infty}^{+\infty} d x e^{-a x^{2}}=\sqrt{\pi / a} .$ ]

  comment

• Paper 2, Section II, C

  Statistical Physics | Part II, 2012

  Explain what is meant by an isothermal expansion and an adiabatic expansion of a gas.

  By first establishing a suitable Maxwell relation, show that

  $$\left.\frac{\partial E}{\partial V}\right|_{T}=\left.T \frac{\partial p}{\partial T}\right|_{V}-p$$

  and

  $$\left.\frac{\partial C_{V}}{\partial V}\right|_{T}=\left.T \frac{\partial^{2} p}{\partial T^{2}}\right|_{V}$$

  The energy in a gas of blackbody radiation is given by $E=a V T^{4}$, where $a$ is a constant. Derive an expression for the pressure $p(V, T)$.

  Show that if the radiation expands adiabatically, $V T^{3}$ is constant.

  comment

• Paper 3, Section II, C

  Statistical Physics | Part II, 2012

  A ferromagnet has magnetization order parameter $m$ and is at temperature $T$. The free energy is given by

  $$F(T ; m)=F_{0}(T)+\frac{a}{2}\left(T-T_{c}\right) m^{2}+\frac{b}{4} m^{4}$$

  where $a, b$ and $T_{c}$ are positive constants. Find the equilibrium value of the magnetization at both high and low temperatures.

  Evaluate the free energy of the ground state as a function of temperature. Hence compute the entropy and heat capacity. Determine the jump in the heat capacity and identify the order of the phase transition.

  After imposing a background magnetic field $B$, the free energy becomes

  $$F(T ; m)=F_{0}(T)+B m+\frac{a}{2}\left(T-T_{c}\right) m^{2}+\frac{b}{4} m^{4}$$

  Explain graphically why the system undergoes a first-order phase transition at low temperatures as $B$ changes sign.

  The spinodal point occurs when the meta-stable vacuum ceases to exist. Determine the temperature $T$ of the spinodal point as a function of $T_{c}, a, b$ and $B$.

  comment

• Paper 4, Section II, C

  Statistical Physics | Part II, 2012

  Non-relativistic electrons of mass $m$ are confined to move in a two-dimensional plane of area $A$. Each electron has two spin states. Compute the density of states $g(E)$ and show that it is constant.

  Write down expressions for the number of particles $N$ and the average energy $\langle E\rangle$ of a gas of fermions in terms of the temperature $T$ and chemical potential $\mu$. Find an expression for the Fermi Energy $E_{F}$ in terms of $N$.

  For $k_{B} T \ll E_{F}$, you may assume that the chemical potential does not change with temperature. Compute the low temperature heat capacity of a gas of fermions. [You may use the approximation that, for large $z$,

  $$\left.\int_{0}^{\infty} \frac{x^{n} d x}{z^{-1} e^{x}+1} \approx \frac{1}{n+1}(\log z)^{n+1}+\frac{\pi^{2} n}{6}(\log z)^{n-1} .\right]$$

  comment

Stochastic Financial Models

• Paper 1, Section II, J

  Stochastic Financial Models | Part II, 2012

  Consider a multi-period binomial model with a risky asset $\left(S_{0}, \ldots, S_{T}\right)$ and a riskless asset $\left(B_{0}, \ldots, B_{T}\right)$. In each period, the value of the risky asset $S$ is multiplied by $u$ if the period was good, and by $d$ otherwise. The riskless asset is worth $B_{t}=(1+r)^{t}$ at time $0 \leqslant t \leqslant T$, where $r \geqslant 0$.

  (i) Assuming that $T=1$ and that

  $$d<1+r<u$$

  show how any contingent claim to be paid at time 1 can be priced and exactly replicated. Briefly explain the significance of the condition (1), and indicate how the analysis of the single-period model extends to many periods.

  (ii) Now suppose that $T=2$. We assume that $u=2, d=1 / 3, r=1 / 2$, and that the risky asset is worth $S_{0}=27$ at time zero. Find the time- 0 value of an American put option with strike price $K=28$ and expiry at time $T=2$, and find the optimal exercise policy. (Assume that the option cannot be exercised immediately at time zero.)

  comment

• Paper 2, Section II, J

  Stochastic Financial Models | Part II, 2012

  (i) Give the definition of Brownian motion.

  (ii) The price $S_{t}$ of an asset evolving in continuous time is represented as

  $$S_{t}=S_{0} \exp \left(\sigma W_{t}+\mu t\right)$$

  where $\left(W_{t}\right)_{t \geqslant 0}$ is a standard Brownian motion and $\sigma$ and $\mu$ are constants. If riskless investment in a bank account returns a continuously compounded rate of interest $r$, derive the Black-Scholes formula for the time-0 price of a European call option on asset $S$ with strike price $K$ and expiry $T$. [Standard results from the course may be used without proof but must be stated clearly.]

  (iii) In the same financial market, a certain contingent claim $C$ pays $\left(S_{T}\right)^{n}$ at time $T$, where $n \geqslant 1$. Find the closed-form expression for the time- 0 value of this contingent claim.

  Show that for every $s>0$ and $n \geqslant 1$,

  $$s^{n}=n(n-1) \int_{0}^{s} k^{n-2}(s-k) d k .$$

  Using this identity, how would you replicate (at least approximately) the contingent claim $C$ with a portfolio consisting only of European calls?

  comment

• Paper 3, Section II, J

  Stochastic Financial Models | Part II, 2012

  (i) Let $\mathcal{F}=\left\{\mathcal{F}_{n}\right\}_{n=0}^{\infty}$ be a filtration. Give the definition of a martingale and a stopping time with respect to the filtration $\mathcal{F}$.

  (ii) State Doob's optional stopping theorem. Give an example of a martingale $M$ and a stopping time $T$ such that $\mathbb{E}\left(M_{T}\right) \neq \mathbb{E}\left(M_{0}\right)$.

  (iii) Let $S_{n}$ be a standard random walk on $\mathbb{Z}$, that is, $S_{0}=0, S_{n}=X_{1}+\ldots+X_{n}$, where $X_{i}$ are i.i.d. and $X_{i}=1$ or $-1$ with probability $1 / 2$.

  Let $T_{a}=\inf \left\{n \geqslant 0: S_{n}=a\right\}$ where $a$ is a positive integer. Show that for all $\theta>0$,

  $$\mathbb{E}\left(e^{-\theta T_{a}}\right)=\left(e^{\theta}-\sqrt{e^{2 \theta}-1}\right)^{a} .$$

  Carefully justify all steps in your derivation.

  [Hint. For all $\lambda>0$ find $\theta$ such that $M_{n}=\exp \left(-\theta n+\lambda S_{n}\right)$ is a martingale. You may assume that $T_{a}$ is almost surely finite.]

  Let $T=T_{a} \wedge T_{-a}=\inf \left\{n \geqslant 0:\left|S_{n}\right|=a\right\}$. By introducing a suitable martingale, compute $\mathbb{E}\left(e^{-\theta T}\right)$.

  comment

• Paper 4, Section II, J

  Stochastic Financial Models | Part II, 2012

  In a one-period market, there are $n$ risky assets whose returns at time 1 are given by a column vector $R=\left(R^{1}, \ldots, R^{n}\right)^{\prime}$. The return vector $R$ has a multivariate Gaussian distribution with expectation $\mu$ and non-singular covariance matrix $V$. In addition, there is a bank account giving interest $r>0$, so that one unit of cash invested at time 0 in the bank account will be worth $R_{f}=1+r$ units of cash at time 1 .

  An agent with the initial wealth $w$ invests $x=\left(x_{1}, \ldots, x_{n}\right)^{\prime}$ in risky assets and keeps the remainder $x_{0}=w-x \cdot \mathbf{1}$ in the bank account. The return on the agent's portfolio is

  $$Z:=x \cdot R+(w-x \cdot \mathbf{1}) R_{f}$$

  The agent's utility function is $u(Z)=-\exp (-\gamma Z)$, where $\gamma>0$ is a parameter. His objective is to maximize $\mathbb{E}(u(Z))$.

  (i) Find the agent's optimal portfolio and its expected return.

  [Hint. Relate $\mathbb{E}(u(Z))$ to $\mathbb{E}(Z)$ and $\operatorname{Var}(Z) .]$

  (ii) Under which conditions does the optimal portfolio that you found in (i) require borrowing from the bank account?

  (iii) Find the optimal portfolio if it is required that all of the agent's wealth be invested in risky assets.

  comment

Topics in Analysis

• Paper 1, Section I, F

  Topics in Analysis | Part II, 2012

  State a version of the Baire category theorem for a complete metric space. Let $T$ be the set of real numbers $x$ with the property that, for each positive integer $n$, there exist integers $p$ and $q$ with $q \geqslant 2$ such that

  $$0<\left|x-\frac{p}{q}\right|<\frac{1}{q^{n}} .$$

  Is $T$ an open subset of $\mathbb{R}$ ? Is $T$ a dense subset of $\mathbb{R}$ ? Justify your answers.

  comment

• Paper 2, Section I, $2 F$

  Topics in Analysis | Part II, 2012

  (a) Let $\gamma:[0,1] \rightarrow \mathbb{C} \backslash\{0\}$ be a continuous map such that $\gamma(0)=\gamma(1)$. Define the winding number $w(\gamma ; 0)$ of $\gamma$ about the origin. State precisely a theorem about homotopy invariance of the winding number.

  (b) Let $f: \mathbb{C} \rightarrow \mathbb{C}$ be a continuous map such that $z^{-10} f(z)$ is bounded as $|z| \rightarrow \infty$. Prove that there exists a complex number $z_{0}$ such that

  $$f\left(z_{0}\right)=z_{0}^{11}$$

  comment

• Paper 2, Section II, F

  Topics in Analysis | Part II, 2012

  (a) State Runge's theorem about uniform approximability of analytic functions by complex polynomials.

  (b) Let $K$ be a compact subset of the complex plane.

  (i) Let $\Sigma$ be an unbounded, connected subset of $\mathbb{C} \backslash K$. Prove that for each $\zeta \in \Sigma$, the function $f(z)=(z-\zeta)^{-1}$ is uniformly approximable on $K$ by a sequence of complex polynomials.

  [You may not use Runge's theorem without proof.]

  (ii) Let $\Gamma$ be a bounded, connected component of $\mathbb{C} \backslash K$. Prove that there is no point $\zeta \in \Gamma$ such that the function $f(z)=(z-\zeta)^{-1}$ is uniformly approximable on $K$ by a sequence of complex polynomials.

  comment

• Paper 3, Section I, $2 F$

  Topics in Analysis | Part II, 2012

  State and prove Liouville's theorem concerning approximation of algebraic numbers by rationals.

  comment

• Paper 3, Section II, F

  Topics in Analysis | Part II, 2012

  State Brouwer's fixed point theorem on the plane, and also an equivalent version of it concerning continuous retractions. Prove the equivalence of the two statements.

  Let $f: \mathbb{R}^{2} \rightarrow \mathbb{R}^{2}$ be a continuous map with the property that $|f(x)| \leqslant 1$ whenever $|x|=1$. Show that $f$ has a fixed point. [Hint. Compose $f$ with the map that sends $x$ to the nearest point to $x$ inside the closed unit disc.]

  comment

• Paper 4, Section I, $2 F$

  Topics in Analysis | Part II, 2012

  Let $A_{1}, A_{2}, \ldots, A_{n}$ be real numbers and suppose that $x_{1}, x_{2}, \ldots, x_{n} \in[-1,1]$ are distinct. Suppose that the formula

  $$\int_{-1}^{1} p(x) d x=\sum_{j=1}^{n} A_{j} p\left(x_{j}\right)$$

  is valid for every polynomial $p$ of degree $\leqslant 2 n-1$. Prove the following:

  (i) $A_{j}>0$ for each $j=1,2, \ldots, n$.

  (ii) $\sum_{j=1}^{n} A_{j}=2$.

  (iii) $x_{1}, x_{2}, \ldots, x_{n}$ are the roots of the Legendre polynomial of degree $n$.

  [You may assume standard orthogonality properties of the Legendre polynomials.]

  comment

Waves

• Paper 1, Section II, 39D

  Waves | Part II, 2012

  Write down the linearized equations governing motion in an inviscid compressible fluid and, assuming an adiabatic relationship $p=p(\rho)$, derive the wave equation for the velocity potential $\phi(\mathbf{x}, t)$. Obtain from these linearized equations the energy equation

  $$\frac{\partial E}{\partial t}+\nabla \cdot \mathbf{I}=0$$

  and give expressions for the acoustic energy density $E$ and the acoustic intensity, or energyflux vector, I.

  An inviscid compressible fluid occupies the half-space $y>0$, and is bounded by a very thin flexible membrane of negligible mass at an undisturbed position $y=0$. Small acoustic disturbances with velocity potential $\phi(x, y, t)$ in the fluid cause the membrane to be deflected to $y=\eta(x, t)$. The membrane is supported by springs that, in the deflected state, exert a restoring force $K \eta \delta x$ on an element $\delta x$ of the membrane. Show that the dispersion relation for waves proportional to $\exp (i k x-i \omega t)$ propagating freely along the membrane is

  $$\left(k^{2}-\frac{\omega^{2}}{c_{0}^{2}}\right)^{1 / 2}-\frac{\rho_{0} \omega^{2}}{K}=0$$

  where $\rho_{0}$ is the density of the fluid and $c_{0}$ is the sound speed. Show that in such a wave the component $\left\langle I_{y}\right\rangle$ of mean acoustic intensity perpendicular to the membrane is zero.

  comment

• Paper 2, Section II, 38D

  Waves | Part II, 2012

  Derive the ray-tracing equations

  $$\frac{d x_{i}}{d t}=\frac{\partial \Omega}{\partial k_{i}}, \quad \frac{d k_{i}}{d t}=-\frac{\partial \Omega}{\partial x_{i}}, \quad \frac{d \omega}{d t}=\frac{\partial \Omega}{\partial t}$$

  for wave propagation through a slowly-varying medium with local dispersion relation $\omega=\Omega(\mathbf{k}, \mathbf{x}, t)$. The meaning of the notation $d / d t$ should be carefully explained.

  A non-dispersive slowly varying medium has a local wave speed $c$ that depends only on the $z$ coordinate. State and prove Snell's Law relating the angle $\psi$ between a ray and the $z$-axis to $c$.

  Consider the case of a medium with wavespeed $c=A \cosh \beta z$, where $A$ and $\beta$ are positive constants. Find the equation of the ray that passes through the origin with wavevector $\left(k_{0}, 0, m_{0}\right)$, and show that it remains in the region $\beta|z| \leqslant \sinh ^{-1}\left(m_{0} / k_{0}\right)$. Sketch several rays passing through the origin.

  comment

• Paper 3, Section II, 39D

  Waves | Part II, 2012

  The function $\phi(x, t)$ satisfies the equation

  $$\frac{\partial \phi}{\partial t}+U \frac{\partial \phi}{\partial x}+\frac{1}{5} \frac{\partial^{5} \phi}{\partial x^{5}}=0$$

  where $U>0$ is a constant. Find the dispersion relation for waves of frequency $\omega$ and wavenumber $k$. Sketch a graph showing both the phase velocity $c(k)$ and the group velocity $c_{g}(k)$, and state whether wave crests move faster or slower than a wave packet.

  Suppose that $\phi(x, 0)$ is real and given by a Fourier transform as

  $$\phi(x, 0)=\int_{-\infty}^{\infty} A(k) e^{i k x} d k$$

  Use the method of stationary phase to obtain an approximation for $\phi(V t, t)$ for fixed $V>U$ and large $t$. If, in addition, $\phi(x, 0)=\phi(-x, 0)$, deduce an approximation for the sequence of times at which $\phi(V t, t)=0$.

  What can be said about $\phi(V t, t)$ if $V<U$ ? [Detailed calculation is not required in this case.]

  [You may assume that $\int_{-\infty}^{\infty} e^{-a u^{2}} d u=\sqrt{\frac{\pi}{a}}$ for $\operatorname{Re}(a) \geqslant 0, a \neq 0 .$ ]

  comment

• Paper 4, Section II, 38D

  Waves | Part II, 2012

  The shallow-water equations

  $$\frac{\partial h}{\partial t}+u \frac{\partial h}{\partial x}+h \frac{\partial u}{\partial x}=0, \quad \frac{\partial u}{\partial t}+u \frac{\partial u}{\partial x}+g \frac{\partial h}{\partial x}=0$$

  describe one-dimensional flow in a channel with depth $h(x, t)$ and velocity $u(x, t)$, where $g$ is the acceleration due to gravity.

  (i) Find the speed $c(h)$ of linearized waves on fluid at rest and of uniform depth.

  (ii) Show that the Riemann invariants $u \pm 2 c$ are constant on characteristic curves $C_{\pm}$of slope $u \pm c$ in the $(x, t)$-plane.

  (iii) Use the shallow-water equations to derive the equation of momentum conservation

  $$\frac{\partial(h u)}{\partial t}+\frac{\partial I}{\partial x}=0$$

  and identify the horizontal momentum flux $I$.

  (iv) A hydraulic jump propagates at constant speed along a straight constant-width channel. Ahead of the jump the fluid is at rest with uniform depth $h_{0}$. Behind the jump the fluid has uniform depth $h_{1}=h_{0}(1+\beta)$, with $\beta>0$. Determine both the speed $V$ of the jump and the fluid velocity $u_{1}$ behind the jump.

  Express $V / c\left(h_{0}\right)$ and $\left(V-u_{1}\right) / c\left(h_{1}\right)$ as functions of $\beta$. Hence sketch the pattern of characteristics in the frame of reference of the jump.

  comment