Part II, 2014, Paper 1

Algebraic Geometry

• Paper 1, Section II, H

  Algebraic Geometry | Part II, 2014

  Let $k$ be an algebraically closed field and $n \geqslant 1$. We say that $f \in k\left[x_{1}, \ldots, x_{n}\right]$ is singular at $p \in \mathbf{A}^{n}$ if either $p$ is a singularity of the hypersurface $\{f=0\}$ or $f$ has an irreducible factor $h$ of multiplicity strictly greater than one with $h(p)=0$. Given $d \geqslant 1$, let $X=\left\{f \in k\left[x_{1}, \ldots, x_{n}\right] \mid \operatorname{deg} f \leqslant d\right\}$ and let

  $$Y=\left\{(f, p) \in X \times \mathbf{A}^{n} \mid f \text { is singular at } p\right\}$$

  (i) Show that $X \simeq \mathbf{A}^{N}$ for some $N$ (you need not determine $N$ ) and that $Y$ is a Zariski closed subvariety of $X \times \mathbf{A}^{n}$.

  (ii) Show that the fibres of the projection map $Y \rightarrow \mathbf{A}^{n}$ are linear subspaces of $\operatorname{dim} e n s i o n N-(n+1)$. Conclude that $\operatorname{dim} Y<\operatorname{dim} X$.

  (iii) Hence show that $\{f \in X \mid \operatorname{deg} f=d, Z(f)$ smooth $\}$ is dense in $X$.

  [You may use standard results from lectures if they are accurately quoted.]

  comment

Algebraic Topology

• Paper 1, Section II, F

  Algebraic Topology | Part II, 2014

  Define what it means for a map $p: \widetilde{X} \rightarrow X$ to be a covering space. State the homotopy lifting lemma.

  Let $p:\left(\tilde{X}, \tilde{x}_{0}\right) \rightarrow\left(X, x_{0}\right)$ be a based covering space and let $f:\left(Y, y_{0}\right) \rightarrow\left(X, x_{0}\right)$ be a based map from a path-connected and locally path-connected space. Show that there is a based lift $\tilde{f}:\left(Y, y_{0}\right) \rightarrow\left(\tilde{X}, \tilde{x}_{0}\right)$ of $f$ if and only if $f_{*}\left(\pi_{1}\left(Y, y_{0}\right)\right) \subseteq p_{*}\left(\pi_{1}\left(\widetilde{X}, \tilde{x}_{0}\right)\right)$.

  comment

Applications of Quantum Mechanics

• Paper 1, Section II, A

  Applications of Quantum Mechanics | Part II, 2014

  A particle of mass $m$ scatters on a localised potential well $V(x)$ in one dimension. With reference to the asymptotic behaviour of the wavefunction as $x \rightarrow \pm \infty$, define the reflection and transmission amplitudes, $r$ and $t$, for a right-moving incident particle of wave number $k$. Define also the corresponding amplitudes, $r^{\prime}$ and $t^{\prime}$, for a left-moving incident particle of wave number $k$. Derive expressions for $r^{\prime}$ and $t^{\prime}$ in terms of $r$ and $t$.

  (a) Define the $S$-matrix, giving its elements in terms of $r$ and $t$. Using the relation

  $$|r|^{2}+|t|^{2}=1$$

  (which you need not derive), show that the S-matrix is unitary. How does the S-matrix simplify if the potential well satisfies $V(-x)=V(x)$ ?

  (b) Consider the potential well

  $$V(x)=-\frac{3 \hbar^{2}}{m} \frac{1}{\cosh ^{2}(x)}$$

  The corresponding Schrödinger equation has an exact solution

  $$\psi_{k}(x)=\exp (i k x)\left[3 \tanh ^{2}(x)-3 i k \tanh (x)-\left(1+k^{2}\right)\right]$$

  with energy $E=\hbar^{2} k^{2} / 2 m$, for every real value of $k$. [You do not need to verify this.] Find the S-matrix for scattering on this potential. What special feature does the scattering have in this case?

  (c) Explain the connection between singularities of the S-matrix and bound states of the potential well. By analytic continuation of the solution $\psi_{k}(x)$ to appropriate complex values of $k$, find the wavefunctions and energies of the bound states of the well. [You do not need to normalise the wavefunctions.]

  comment

Applied Probability

• Paper 1, Section II, J

  Applied Probability | Part II, 2014

  (i) Explain what a $Q$-matrix is. Let $Q$ be a $Q$-matrix. Define the notion of a Markov chain $\left(X_{t}, t \geqslant 0\right)$ in continuous time with $Q$-matrix given by $Q$, and give a construction of $\left(X_{t}, t \geqslant 0\right)$. [You are not required to justify this construction.]

  (ii) A population consists of $N_{t}$ individuals at time $t \geqslant 0$. We assume that each individual gives birth to a new individual at constant rate $\lambda>0$. As the population is competing for resources, we assume that for each $n \geqslant 1$, if $N_{t}=n$, then any individual in the population at time $t$ dies in the time interval $[t, t+h)$ with probability $\delta_{n} h+o(h)$, where $\left(\delta_{n}\right)_{n=1}^{\infty}$ is a given sequence satisfying $\delta_{1}=0, \delta_{n}>0$ for $n \geqslant 2$. Formulate a Markov chain model for $\left(N_{t}, t \geqslant 0\right)$ and write down the $Q$-matrix explicitly. Then find a necessary and sufficient condition on $\left(\delta_{n}\right)_{n=1}^{\infty}$ so that the Markov chain has an invariant distribution. Compute the invariant distribution in the case where $\delta_{n}=\mu(n-1)$ and $\mu>0$.

  comment

Asymptotic Methods

• Paper 1, Section II, C

  Asymptotic Methods | Part II, 2014

  (a) Consider the integral

  $$I(k)=\int_{0}^{\infty} f(t) e^{-k t} d t, \quad k>0$$

  Suppose that $f(t)$ possesses an asymptotic expansion for $t \rightarrow 0^{+}$of the form

  $$f(t) \sim t^{\alpha} \sum_{n=0}^{\infty} a_{n} t^{\beta n}, \quad \alpha>-1, \quad \beta>0$$

  where $a_{n}$ are constants. Derive an asymptotic expansion for $I(k)$ as $k \rightarrow \infty$ in the form

  $$I(k) \sim \sum_{n=0}^{\infty} \frac{A_{n}}{k^{\gamma+\beta n}}$$

  giving expressions for $A_{n}$ and $\gamma$ in terms of $\alpha, \beta, n$ and the gamma function. Hence establish the asymptotic approximation as $k \rightarrow \infty$

  $$I_{1}(k)=\int_{0}^{1} e^{k t} t^{-a}\left(1-t^{2}\right)^{-b} d t \sim 2^{-b} \Gamma(1-b) e^{k} k^{b-1}\left(1+\frac{(a+b / 2)(1-b)}{k}\right)$$

  where $a<1, b<1$.

  (b) Using Laplace's method, or otherwise, find the leading-order asymptotic approximation as $k \rightarrow \infty$ for

  $$I_{2}(k)=\int_{0}^{\infty} e^{-\left(2 k^{2} / t+t^{2} / k\right)} d t$$

  [You may assume that $\Gamma(z)=\int_{0}^{\infty} t^{z-1} e^{-t} d t$ for $\operatorname{Re} z>0$,

  $$\text { and that } \left.\int_{-\infty}^{\infty} e^{-q t^{2}} d t=\sqrt{\pi / q} \text { for } q>0 .\right]$$

  comment

Classical Dynamics

• Paper 1, Section I, A

  Classical Dynamics | Part II, 2014

  Consider a one-dimensional dynamical system with generalized coordinate and momentum $(q, p)$.

  (a) Define the Poisson bracket $\{f, g\}$ of two functions $f(q, p, t)$ and $g(q, p, t)$.

  (b) Verify the Leibniz rule

  $$\{f g, h\}=f\{g, h\}+g\{f, h\}$$

  (c) Explain what is meant by a canonical transformation $(q, p) \rightarrow(Q, P)$.

  (d) State the condition for a transformation $(q, p) \rightarrow(Q, P)$ to be canonical in terms of the Poisson bracket $\{Q, P\}$. Use this to determine whether or not the following transformations are canonical:

  (i) $Q=\frac{q^{2}}{2}, P=\frac{p}{q}$,

  (ii) $Q=\tan q, P=p \cos q$,

  (iii) $Q=\sqrt{2 q} e^{t} \cos p, P=\sqrt{2 q} e^{-t} \sin p$.

  comment

Coding and Cryptography

• Paper 1, Section I, $4 \mathrm{I}$

  Coding and Cryptography | Part II, 2014

  State and prove Gibbs' inequality.

  Show that, for a pair of discrete random variables $X$ and $Y$, each taking finitely many values, the joint entropy $H(X, Y)$ satisfies

  $$H(X, Y) \leqslant H(X)+H(Y)$$

  with equality precisely when $X$ and $Y$ are independent.

  comment

• Paper 1, Section II, I

  Coding and Cryptography | Part II, 2014

  Describe, briefly, either the RSA or the Elgamal public key cipher. You should explain, without proof, why it is believed to be difficult to break the cipher you describe.

  How can such a cipher be used to sign messages? You should explain how the intended recipient of the message can (a) know from whom it came; (b) know that the message has not been changed; and (c) demonstrate that the sender must have signed it.

  Let $I_{0}, I_{1}, \ldots, I_{N}$ be friendly individuals each of whom has a public key cipher. $I_{0}$ wishes to send a message to $I_{N}$ by passing it first to $I_{1}$, then $I_{1}$ passes it to $I_{2}, I_{2}$ to $I_{3}$, until finally it is received by $I_{N}$. At each stage the message can be modified to show from whom it was received and to whom it is sent. Devise a way in which these modifications can be made so that $I_{N}$ can be confident both of the content of the original message and that the message has been passed through the intermediaries $I_{1}, I_{2}, \ldots, I_{N-1}$ in that order and has not been modified by an enemy agent. Assume that it takes a negligible time to transmit a message from $I_{k}$ to $I_{k+1}$ for each $k$, but the time needed to modify a message is not negligible.

  comment

Cosmology

• Paper 1, Section I, E

  Cosmology | Part II, 2014

  Which particle states are expected to be relativistic and which interacting when the temperature $T$ of the early universe satisfies (i) $10^{10} \mathrm{~K}<T<5 \times 10^{10} \mathrm{~K}$, (ii) $5 \times 10^{9} \mathrm{~K}<T<10^{10} \mathrm{~K}$, (iii) $T<5 \times 10^{9} \mathrm{~K}$ ?

  Calculate the total spin weight factor, $g_{*}$, of the relativistic particles and the total spin weight factor, $g_{I}$, of the interacting particles, in each of the three temperature intervals.

  What happens when the temperature falls below $5 \times 10^{9} \mathrm{~K} ?$ Calculate the ratio of the temperatures of neutrinos and photons. Find the effective value of $g_{*}$ after the universe cools below this temperature. [Note that the equilibrium entropy density is given by $s=\left(\rho c^{2}+P\right) / T$, where $\rho$ is the density and $P$ is the pressure.]

  comment

• Paper 1, Section II, E

  Cosmology | Part II, 2014

  What are the cosmological flatness and horizon problems? Explain what form of time evolution of the cosmological expansion scale factor $a(t)$ must occur during a period of inflationary expansion in a Friedmann universe. How can inflation solve the horizon and flatness problems? [You may assume an equation of state where pressure $P$ is proportional to density $\rho$.]

  The universe has Hubble expansion rate $H=\dot{a} / a$ and contains only a scalar field $\phi$ with self-interaction potential $V(\phi)>0$. The density and pressure are given by

  $$\begin{aligned} \rho &=\frac{1}{2} \dot{\phi}^{2}+V(\phi) \\ P &=\frac{1}{2} \dot{\phi}^{2}-V(\phi) \end{aligned}$$

  in units where $c=\hbar=1$. Show that the conservation equation

  $$\dot{\rho}+3 H(\rho+P)=0$$

  requires

  $$\ddot{\phi}+3 H \dot{\phi}+d V / d \phi=0 .$$

  If the Friedmann equation has the form

  $$3 H^{2}=8 \pi G \rho$$

  and the scalar-field potential has the form

  $$V(\phi)=V_{0} e^{-\lambda \phi}$$

  where $V_{0}$ and $\lambda$ are positive constants, show that there is an exact cosmological solution with

  $$\begin{aligned} &a(t) \propto t^{16 \pi G / \lambda^{2}} \\ &\phi(t)=\phi_{0}+\frac{2}{\lambda} \ln (t), \end{aligned}$$

  where $\phi_{0}$ is a constant. Find the algebraic relation between $\lambda, V_{0}$ and $\phi_{0}$. Show that a solution only exists when $0<\lambda^{2}<48 \pi G$. For what range of values of $\lambda^{2}$ does inflation occur? Comment on what happens when $\lambda \rightarrow 0$.

  comment

Differential Geometry

• Paper 1, Section II, G

  Differential Geometry | Part II, 2014

  Define the concepts of (smooth) manifold and manifold with boundary for subsets of $\mathbf{R}^{N}$.

  Let $X \subset \mathbf{R}^{6}$ be the subset defined by the equations

  $$x_{1}^{2}+x_{2}^{2}+x_{3}^{2}-x_{4}^{2}=1, \quad x_{4}^{2}-x_{5}^{2}-x_{6}^{2}=-1 .$$

  Prove that $X$ is a manifold of dimension four.

  For $a>0$, let $B(a) \subset \mathbf{R}^{6}$ denote the spherical ball $x_{1}^{2}+\ldots+x_{6}^{2} \leqslant a$. Prove that $X \cap B(a)$ is empty if $a<2$, is a manifold diffeomorphic to $S^{2} \times S^{1}$ if $a=2$, and is a manifold with boundary if $a>2$, with each component of the boundary diffeomorphic to $S^{2} \times S^{1}$.

  [You may quote without proof any general results from lectures that you may need.]

  comment

Dynamical Systems

• Paper 1, Section I, D

  Dynamical Systems | Part II, 2014

  Consider the system

  $$\begin{aligned} &\dot{x}=y+x y \\ &\dot{y}=x-\frac{3}{2} y+x^{2} \end{aligned}$$

  Show that the origin is a hyperbolic fixed point and find the stable and unstable invariant subspaces of the linearised system.

  Calculate the stable and unstable manifolds correct to quadratic order, expressing $y$ as a function of $x$ for each.

  comment

Electrodynamics

• Paper 1, Section II, 36C

  Electrodynamics | Part II, 2014

  (i) Starting from the field-strength tensor $F_{\mu \nu}=\partial_{\mu} A_{\nu}-\partial_{\nu} A_{\mu}$, where $A^{\mu}=(\phi / c, \mathbf{A})$ is the 4-vector potential with components such that

  $$\mathbf{E}=-\frac{\partial \mathbf{A}}{\partial t}-\boldsymbol{\nabla} \phi \quad \text { and } \quad \mathbf{B}=\boldsymbol{\nabla} \times \mathbf{A}$$

  derive the transformation laws for the components of the electric field $\mathbf{E}$ and the magnetic field $\mathbf{B}$ under the standard Lorentz boost $x^{\prime \mu}=\Lambda_{\nu}^{\mu} x^{\nu}$ with

  $$\Lambda_{\nu}^{\mu}=\left(\begin{array}{cccc} \gamma & -\gamma \beta & 0 & 0 \\ -\gamma \beta & \gamma & 0 & 0 \\ 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 1 \end{array}\right)$$

  (ii) Two point charges, each with electric charge $q$, are at rest and separated by a distance $d$ in some inertial frame $S$. By transforming the fields from the rest frame $S$, calculate the magnitude and direction of the force between the two charges in an inertial frame in which the charges are moving with speed $\beta c$ in a direction perpendicular to their separation.

  (iii) The 4-force for a particle with 4-momentum $p^{\mu}$ is $F^{\mu}=d p^{\mu} / d \tau$, where $\tau$ is proper time. Show that the components of $F^{\mu}$ in an inertial frame in which the particle has 3 -velocity $\mathbf{v}$ are

  $$F^{\mu}=\gamma(\mathbf{F} \cdot \mathbf{v} / c, \mathbf{F})$$

  where $\gamma=\left(1-\mathbf{v} \cdot \mathbf{v} / c^{2}\right)^{-1 / 2}$ and $\mathbf{F}$ is the 3-force acting on the particle. Hence verify that your result in (ii) above is consistent with Lorentz transforming the electromagnetic 3 -force from the rest frame $S$.

  comment

Fluid Dynamics II

• Paper 1, Section II, B

  Fluid Dynamics II | Part II, 2014

  A particle of arbitrary shape and volume $4 \pi a^{3} / 3$ moves at velocity $\mathbf{U}(t)$ through an unbounded incompressible fluid of density $\rho$ and viscosity $\mu$. The Reynolds number of the flow is very small so that the inertia of the fluid can be neglected. Show that the particle experiences a force $\mathbf{F}(t)$ due to the surface stresses given by

  $$F_{i}(t)=-\mu a A_{i j} U_{j}(t)$$

  where $A_{i j}$ is a dimensionless second-rank tensor determined solely by the shape and orientation of the particle. State the reason why $A_{i j}$ must be positive definite.

  Show further that, if the particle has the same reflectional symmetries as a cube, then

  $$A_{i j}=\lambda \delta_{i j}$$

  Let $b$ be the radius of the smallest sphere that contains the particle (still assuming cubic symmetry). By considering the Stokes flow associated with this sphere, suitably extended, and using the minimum dissipation theorem (which should be stated carefully), show that

  $$\lambda \leqslant 6 \pi b / a .$$

  [You may assume the expression for the Stokes drag on a sphere.]

  comment

Further Complex Methods

• Paper 1, Section $I$, B

  Further Complex Methods | Part II, 2014

  Show that the Cauchy-Riemann equations for $f: \mathbb{C} \rightarrow \mathbb{C}$ are equivalent to

  $$\frac{\partial f}{\partial \bar{z}}=0 \text {, }$$

  where $z=x+i y$, and $\partial / \partial \bar{z}$ should be defined in terms of $\partial / \partial x$ and $\partial / \partial y$. Use Green's theorem, together with the formula $d z d \bar{z}=-2 i d x d y$, to establish the generalised Cauchy formula

  $$\oint_{\gamma} f(z, \bar{z}) d z=-\iint_{D} \frac{\partial f}{\partial \bar{z}} d z d \bar{z}$$

  where $\gamma$ is a contour in the complex plane enclosing the region $D$ and $f$ is sufficiently differentiable.

  comment

• Paper 1, Section II, 14B

  Further Complex Methods | Part II, 2014

  Obtain solutions of the second-order ordinary differential equation

  $$z w^{\prime \prime}-w=0$$

  in the form

  $$w(z)=\int_{\gamma} f(t) e^{-z t} d t$$

  where the function $f$ and the choice of contour $\gamma$ should be determined from the differential equation.

  Show that a non-trivial solution can be obtained by choosing $\gamma$ to be a suitable closed contour, and find the resulting solution in this case, expressing your answer in the form of a power series.

  Describe a contour $\gamma$ that would provide a second linearly independent solution for the case $\operatorname{Re}(z)>0$.

  comment

Galois Theory

• Paper 1, Section II, 18H

  Galois Theory | Part II, 2014

  What is meant by the statement that $L$ is a splitting field for $f \in K[X] ?$

  Show that if $f \in K[X]$, then there exists a splitting field for $f$ over $K$. Explain the sense in which a splitting field for $f$ over $K$ is unique.

  Determine the degree $[L: K]$ of a splitting field $L$ of the polynomial $f=X^{4}-4 X^{2}+2$ over $K$ in the cases (i) $K=\mathbb{Q}$, (ii) $K=\mathbb{F}_{5}$, and (iii) $K=\mathbb{F}_{7}$.

  comment

General Relativity

• Paper 1, Section II, 37E

  General Relativity | Part II, 2014

  For a timelike geodesic in the equatorial plane $\left(\theta=\frac{1}{2} \pi\right)$ of the Schwarzschild spacetime with line element

  $$d s^{2}=-\left(1-r_{s} / r\right) c^{2} d t^{2}+\left(1-r_{s} / r\right)^{-1} d r^{2}+r^{2}\left(d \theta^{2}+\sin ^{2} \theta d \phi^{2}\right)$$

  derive the equation

  $$\frac{1}{2} \dot{r}^{2}+V(r)=\frac{1}{2}(E / c)^{2}$$

  where

  $$\frac{2 V(r)}{c^{2}}=1-\frac{r_{s}}{r}+\frac{h^{2}}{c^{2} r^{2}}-\frac{h^{2} r_{s}}{c^{2} r^{3}}$$

  and $h$ and $E$ are constants. The dot denotes the derivative with respect to an affine parameter $\tau$ satisfying $c^{2} d \tau^{2}=-d s^{2}$.

  Given that there is a stable circular orbit at $r=R$, show that

  $$\frac{h^{2}}{c^{2}}=\frac{R^{2} \epsilon}{2-3 \epsilon}$$

  where $\epsilon=r_{s} / R$.

  Compute $\Omega$, the orbital angular frequency (with respect to $\tau$ ).

  Show that the angular frequency $\omega$ of small radial perturbations is given by

  $$\frac{\omega^{2} R^{2}}{c^{2}}=\frac{\epsilon(1-3 \epsilon)}{2-3 \epsilon}$$

  Deduce that the rate of precession of the perihelion of the Earth's orbit, $\Omega-\omega$, is approximately $3 \Omega^{3} T^{2}$, where $T$ is the time taken for light to travel from the Sun to the Earth. [You should assume that the Earth's orbit is approximately circular, with $r_{s} / R \ll 1$ and $\left.E \simeq c^{2} .\right]$

  comment

Geometry and Groups

• Paper 1, Section I, F

  Geometry and Groups | Part II, 2014

  Let $G \leqslant S O(3)$ be a finite group. Suppose $G$ does not preserve any plane in $\mathbb{R}^{3}$. Show that for any point $p$ in the unit sphere $S^{2} \subset \mathbb{R}^{3}$, the stabiliser $\operatorname{Stab}_{G}(p)$ contains at most 5 elements.

  comment

• Paper 1, Section II, F

  Geometry and Groups | Part II, 2014

  Prove that an orientation-preserving isometry of the ball-model of hyperbolic space $\mathbb{H}^{3}$ which fixes the origin is an element of $S O(3)$. Hence, or otherwise, prove that a finite subgroup of the group of orientation-preserving isometries of hyperbolic space $\mathbb{H}^{3}$ has a common fixed point.

  Can an infinite non-cyclic subgroup of the isometry group of $\mathbb{H}^{3}$ have a common fixed point? Can any such group be a Kleinian group? Justify your answers.

  comment

Graph Theory

• Paper 1, Section II, I

  Graph Theory | Part II, 2014

  Show that a graph is bipartite if and only if all of its cycles are of even length.

  Show that a bridgeless plane graph is bipartite if and only if all of its faces are of even length.

  Let $G$ be an Eulerian plane graph. Show that the faces of $G$ can be coloured with two colours so that no two contiguous faces have the same colour. Deduce that it is possible to assign a direction to each edge of $G$ in such a way that the edges around each face form a directed cycle.

  comment

Integrable Systems

• Paper 1, Section II, D

  Integrable Systems | Part II, 2014

  Consider the coordinate transformation

  $$g^{\epsilon}:(x, u) \mapsto(\tilde{x}, \tilde{u})=(x \cos \epsilon-u \sin \epsilon, x \sin \epsilon+u \cos \epsilon)$$

  Show that $g^{\epsilon}$ defines a one-parameter group of transformations. Define what is meant by the generator $V$ of a one-parameter group of transformations and compute it for the above case.

  Now suppose $u=u(x)$. Explain what is meant by the first prolongation $\mathrm{pr}^{(1)} g^{\epsilon}$ of $g^{\epsilon}$. Compute $\mathrm{pr}^{(1)} g^{\epsilon}$ in this case and deduce that

  $$\mathrm{pr}^{(1)} V=V+\left(1+u_{x}^{2}\right) \frac{\partial}{\partial u_{x}}$$

  Similarly find $\mathrm{pr}^{(2)} V$.

  Define what is meant by a Lie point symmetry of the first-order differential equation $\Delta\left[x, u, u_{x}\right]=0$. Describe this condition in terms of the vector field that generates the Lie point symmetry. Consider the case

  $$\Delta\left[x, u, u_{x}\right] \equiv u_{x}-\frac{u+x f\left(x^{2}+u^{2}\right)}{x-u f\left(x^{2}+u^{2}\right)}$$

  where $f$ is an arbitrary smooth function of one variable. Using $(\star)$, show that $g^{\epsilon}$ generates a Lie point symmetry of the corresponding differential equation.

  comment

Linear Analysis

• Paper 1, Section II, G

  Linear Analysis | Part II, 2014

  Let $X$ and $Y$ be normed spaces. What is an isomorphism between $X$ and $Y$ ? Show that a bounded linear map $T: X \rightarrow Y$ is an isomorphism if and only if $T$ is surjective and there is a constant $c>0$ such that $\|T x\| \geqslant c\|x\|$ for all $x \in X$. Show that if there is an isomorphism $T: X \rightarrow Y$ and $X$ is complete, then $Y$ is complete.

  Show that two normed spaces of the same finite dimension are isomorphic. [You may assume without proof that any two norms on a finite-dimensional space are equivalent.] Briefly explain why this implies that every finite-dimensional space is complete, and every closed and bounded subset of a finite-dimensional space is compact.

  Let $Z$ and $F$ be subspaces of a normed space $X$ with $Z \cap F=\{0\}$. Assume that $Z$ is closed in $X$ and $F$ is finite-dimensional. Prove that $Z+F$ is closed in $X$. [Hint: First show that the function $x \mapsto d(x, Z)=\inf \{\|x-z\|: z \in Z\}$ restricted to the unit sphere of F achieves its minimum.]

  comment

Logic and Set Theory

• Paper 1, Section II, I

  Logic and Set Theory | Part II, 2014

  Explain what is meant by saying that a binary relation $r \subseteq a \times a$ is well-founded. Show that $r$ is well-founded if and only if, for any set $b$ and any function $f: \mathcal{P} b \rightarrow b$, there exists a unique function $g: a \rightarrow b$ satisfying

  $$g(x)=f(\{g(y) \mid\langle y, x\rangle \in r\})$$

  for all $x \in a$. [Hint: For 'if', it suffices to take $b=\{0,1\}$, with $f: \mathcal{P} b \rightarrow b$ defined by $\left.f\left(b^{\prime}\right)=1 \Leftrightarrow 1 \in b^{\prime} .\right]$

  comment

Mathematical Biology

• Paper 1, Section I, B

  Mathematical Biology | Part II, 2014

  A population model for two species is given by

  $$\begin{aligned} &\frac{d N}{d t}=a N-b N P-k N^{2} \\ &\frac{d P}{d t}=-d P+c N P \end{aligned}$$

  where $a, b, c, d$ and $k$ are positive parameters. Show that this may be rescaled to

  $$\begin{aligned} &\frac{d u}{d \tau}=u(1-v-\beta u) \\ &\frac{d v}{d \tau}=-\alpha v(1-u) \end{aligned}$$

  and give $\alpha$ and $\beta$ in terms of the original parameters.

  For $\beta<1$ find all fixed points in $u \geqslant 0, v \geqslant 0$, and analyse their stability. Assuming that both populations are present initially, what does this suggest will be the long-term outcome?

  comment

Number Fields

• Paper 1, Section II, F

  Number Fields | Part II, 2014

  State a result involving the discriminant of a number field that implies that the class group is finite.

  Use Dedekind's theorem to factor $2,3,5$ and 7 into prime ideals in $K=\mathbb{Q}(\sqrt{-34})$. By factoring $1+\sqrt{-34}$ and $4+\sqrt{-34}$, or otherwise, prove that the class group of $K$ is cyclic, and determine its order.

  comment

Number Theory

• Paper 1, Section I, $1 F$

  Number Theory | Part II, 2014

  Define what it means for a number $N$ to be a pseudoprime to the base $b$.

  Show that if there is a base $b$ to which $N$ is not a pseudoprime, then $N$ is a pseudoprime to at most half of all possible bases.

  Let $n$ be an integer greater than 1 such that $F_{n}=2^{2^{n}}+1$ is composite. Show that $F_{n}$ is a pseudoprime to the base 2 .

  comment

Numerical Analysis

• Paper 1, Section II, 40D

  Numerical Analysis | Part II, 2014

  (i) Consider the numerical approximation of the boundary-value problem

  $$\begin{gathered} u^{\prime \prime}=f, \quad u:[0,1] \rightarrow \mathbb{R}, \\ u(0)=\varphi_{0}, \quad u(1)=\varphi_{1}, \end{gathered}$$

  where $\varphi_{0}, \varphi_{1}$ are given constants and $f$ is a given smooth function on $[0,1]$. A grid $\left\{x_{1}, x_{2}, \ldots, x_{N}\right\}, N \geqslant 3$, on $[0,1]$ is given by

  $$x_{1}=\alpha_{1} h, \quad x_{i}=x_{i-1}+h \text { for } i=2, \ldots, N-1, \quad x_{N}=1-\alpha_{2} h$$

  where $0<\alpha_{1}, \alpha_{2}<1, \alpha_{1}+\alpha_{2}=1$ and $h=1 / N$. Derive finite-difference approximations for $u^{\prime \prime}\left(x_{i}\right)$, for $i=1, \ldots, N$, using at most one neighbouring grid point of $x_{i}$ on each side. Hence write down a numerical scheme to solve the problem, displaying explicitly the entries of the system matrix $A$ in the resulting system of linear equations $A u=b$, $A \in \mathbb{R}^{N \times N}, u, b \in \mathbb{R}^{N}$. What is the overall order of this numerical scheme? Explain briefly one strategy by which the order could be improved with the same grid.

  (ii) Consider the numerical approximation of the boundary-value problem

  $$\begin{aligned} &\nabla^{2} u=f, \quad u: \Omega \rightarrow \mathbb{R}, \\ &u(x)=0 \text { for all } x \in \partial \Omega \end{aligned}$$

  where $\Omega \subset \mathbb{R}^{2}$ is an arbitrary, simply connected bounded domain with smooth boundary $\partial \Omega$, and $f$ is a given smooth function. Define the 9-point formula used to approximate the Laplacian. Using this formula and an equidistant grid inside $\Omega$, define a numerical scheme for which the system matrix is symmetric and negative definite. Prove that the system matrix of your scheme has these properties for all choices of ordering of the grid points.

  Part II, $2014 \quad$ List of Questions

  [TURN OVER

  comment

Partial Differential Equations

• Paper 1, Section II, D

  Partial Differential Equations | Part II, 2014

  State the Cauchy-Kovalevskaya theorem, including a definition of the term noncharacteristic.

  For which values of the real number $a$, and for which functions $f$, does the CauchyKovalevskaya theorem ensure that the Cauchy problem

  $$u_{t t}=u_{x x}+a u_{x x x x}, \quad u(x, 0)=0, u_{t}(x, 0)=f(x)$$

  has a local solution?

  Now consider the Cauchy problem (1) in the case that $f(x)=\sum_{m \in \mathbb{Z}} \hat{f}(m) e^{i m x}$ is a smooth $2 \pi$-periodic function.

  (i) Show that if $a \leqslant 0$ there exists a unique smooth solution $u$ for all times, and show that for all $T \geqslant 0$ there exists a number $C=C(T)>0$, independent of $f$, such that

  $$\int_{-\pi}^{+\pi}|u(x, t)|^{2} d x \leqslant C \int_{-\pi}^{+\pi}|f(x)|^{2} d x$$

  for all $t:|t| \leqslant T$.

  (ii) If $a=1$ does there exist a choice of $C=C(T)$ for which (2) holds? Give a full justification for your answer.

  comment

Principles of Quantum Mechanics

• Paper 1, Section II, A

  Principles of Quantum Mechanics | Part II, 2014

  Let $\hat{x}, \hat{p}$ and $H(\hat{x}, \hat{p})=\hat{p}^{2} / 2 m+V(\hat{x})$ be the position operator, momentum operator and Hamiltonian for a particle moving in one dimension. Let $|\psi\rangle$ be the state vector for the particle. The position and momentum eigenstates have inner products

  $$\langle x \mid p\rangle=\frac{1}{\sqrt{2 \pi \hbar}} \exp (i p x / \hbar), \quad\left\langle x \mid x^{\prime}\right\rangle=\delta\left(x-x^{\prime}\right) \quad \text { and } \quad\left\langle p \mid p^{\prime}\right\rangle=\delta\left(p-p^{\prime}\right) .$$

  Show that

  $$\langle x|\hat{p}| \psi\rangle=-i \hbar \frac{\partial}{\partial x} \psi(x) \quad \text { and } \quad\langle p|\hat{x}| \psi\rangle=i \hbar \frac{\partial}{\partial p} \tilde{\psi}(p)$$

  where $\psi(x)$ and $\tilde{\psi}(p)$ are the wavefunctions in the position representation and momentum representation, respectively. Show how $\psi(x)$ and $\tilde{\psi}(p)$ may be expressed in terms of each other.

  For general $V(\hat{x})$, express $\langle p|V(\hat{x})| \psi\rangle$ in terms of $\tilde{\psi}(p)$, and hence write down the time-independent Schrödinger equation in the momentum representation satisfied by $\tilde{\psi}(p)$.

  Consider now the case $V(x)=-\left(\hbar^{2} \lambda / m\right) \delta(x), \lambda>0$. Show that there is a bound state with energy $E=-\varepsilon, \varepsilon>0$, with wavefunction $\tilde{\psi}(p)$ satisfying

  $$\tilde{\psi}(p)=\frac{\hbar \lambda}{\pi} \frac{1}{2 m \varepsilon+p^{2}} \int_{-\infty}^{\infty} \tilde{\psi}\left(p^{\prime}\right) d p^{\prime}$$

  Hence show that there is a unique value for $\varepsilon$ and determine this value.

  comment

Principles of Statistics

• Paper 1, Section II, J

  Principles of Statistics | Part II, 2014

  State without proof the inequality known as the Cramér-Rao lower bound in a parametric model $\{f(\cdot, \theta): \theta \in \Theta\}, \Theta \subseteq \mathbb{R}$. Give an example of a maximum likelihood estimator that attains this lower bound, and justify your answer.

  Give an example of a parametric model where the maximum likelihood estimator based on observations $X_{1}, \ldots, X_{n}$ is biased. State without proof an analogue of the Cramér-Rao inequality for biased estimators.

  Define the concept of a minimax decision rule, and show that the maximum likelihood estimator $\hat{\theta}_{M L E}$ based on $X_{1}, \ldots, X_{n}$ in a $N(\theta, 1)$ model is minimax for estimating $\theta \in \Theta=\mathbb{R}$ in quadratic risk.

  comment

Probability and Measure

• Paper 1, Section II, $26 \mathrm{~K}$

  Probability and Measure | Part II, 2014

  What is meant by the Borel $\sigma$-algebra on the real line $\mathbb{R}$ ?

  Define the Lebesgue measure of a Borel subset of $\mathbb{R}$ using the concept of outer measure.

  Let $\mu$ be the Lebesgue measure on $\mathbb{R}$. Show that, for any Borel set $B$ which is contained in the interval $[0,1]$, and for any $\varepsilon>0$, there exist $n \in \mathbb{N}$ and disjoint intervals $I_{1}, \ldots, I_{n}$ contained in $[0,1]$ such that, for $A=I_{1} \cup \cdots \cup I_{n}$, we have

  $$\mu(A \triangle B) \leqslant \varepsilon$$

  where $A \triangle B$ denotes the symmetric difference $(A \backslash B) \cup(B \backslash A)$.

  Show that there does not exist a Borel set $B$ contained in $[0,1]$ such that, for all intervals $I$ contained in $[0,1]$,

  $$\mu(B \cap I)=\mu(I) / 2$$

  comment

Representation Theory

• Paper 1, Section II, H

  Representation Theory | Part II, 2014

  (i) Let $K$ be any field and let $\lambda \in K$. Let $J_{\lambda, n}$ be the $n \times n$ Jordan block

  $$J_{\lambda, n}=\left(\begin{array}{ccccc} \lambda & 1 & 0 & \cdots & 0 \\ 0 & \ddots & \ddots & & \vdots \\ \vdots & & \ddots & \ddots & 0 \\ \vdots & & & \ddots & 1 \\ 0 & \cdots & \cdots & 0 & \lambda \end{array}\right)$$

  Compute $J_{\lambda, n}^{r}$ for each $r \geqslant 0$.

  (ii) Let $G$ be a cyclic group of order $N$, and let $K$ be an algebraically closed field of characteristic $p \geqslant 0$. Determine all the representations of $G$ on vector spaces over $K$, up to equivalence. Which are irreducible? Which do not split as a direct sum $W \oplus W^{\prime}$, with $W \neq 0$ and $W^{\prime} \neq 0 ?$

  comment

Riemann Surfaces

• Paper 1, Section II, H

  Riemann Surfaces | Part II, 2014

  If $X$ is a Riemann surface and $p: Y \rightarrow X$ is a covering map of topological spaces, show that there is a conformal structure on $Y$ such that $p: Y \rightarrow X$ is analytic.

  Let $f(z)$ be the complex polynomial $z^{5}-1$. Consider the subspace $R$ of $\mathbb{C}^{2}=\mathbb{C} \times \mathbb{C}$ given by the equation $w^{2}=f(z)$, where $(z, w)$ denotes coordinates in $\mathbb{C}^{2}$, and let $\pi: R \rightarrow \mathbb{C}$ be the restriction of the projection map onto the first factor. Show that $R$ has the structure of a Riemann surface which makes $\pi$ an analytic map. If $\tau$ denotes projection onto the second factor, show that $\tau$ is also analytic. [You may assume that $R$ is connected.]

  Find the ramification points and the branch points of both $\pi$ and $\tau$. Compute also the ramification indices at the ramification points.

  Assuming that it is possible to add a point $P$ to $R$ so that $X=R \cup\{P\}$ is a compact Riemann surface and $\tau$ extends to a holomorphic map $\tau: X \rightarrow \mathbb{C}_{\infty}$ such that $\tau^{-1}(\infty)=\{P\}$, compute the genus of $X .$

  comment

Statistical Modelling

• Paper 1, Section $I$, K

  Statistical Modelling | Part II, 2014

  Write down the model being fitted by the following $\mathrm{R}$ command, where $y \in\{0,1,2, \ldots\}^{n}$ and $X$ is an $n \times p$ matrix with real-valued entries.

  fit $<-\operatorname{glm}(\mathrm{y} \sim \mathrm{X}, \mathrm{family}=$ poisson)

  Write down the log-likelihood for the model. Explain why the command

  $\operatorname{sum}(y)-\operatorname{sum}($ predict (fit, type $=$ "response" $))$

  gives the answer 0, by arguing based on the log-likelihood you have written down. [Hint: Recall that if $Z \sim \operatorname{Pois}(\mu)$ then

  $$\mathbb{P}(Z=k)=\frac{\mu^{k} e^{-\mu}}{k !}$$

  for $k \in\{0,1,2, \ldots\}$.]

  comment

• Paper 1, Section II, $13 K$

  Statistical Modelling | Part II, 2014

  Consider the normal linear model where the $n$-vector of responses $Y$ satisfies $Y=X \beta+\varepsilon$ with $\varepsilon \sim N_{n}\left(0, \sigma^{2} I\right)$. Here $X$ is an $n \times p$ matrix of predictors with full column rank where $n \geqslant p+3$, and $\beta \in \mathbb{R}^{p}$ is an unknown vector of regression coefficients. Let $X_{0}$ be the matrix formed from the first $p_{0}<p$ columns of $X$, and partition $\beta$ as $\beta=\left(\beta_{0}^{T}, \beta_{1}^{T}\right)^{T}$ where $\beta_{0} \in \mathbb{R}^{p_{0}}$ and $\beta_{1} \in \mathbb{R}^{p-p_{0}}$. Denote the orthogonal projections onto the column spaces of $X$ and $X_{0}$ by $P$ and $P_{0}$ respectively.

  It is desired to test the null hypothesis $H_{0}: \beta_{1}=0$ against the alternative hypothesis $H_{1}: \beta_{1} \neq 0$. Recall that the $F$-test for testing $H_{0}$ against $H_{1}$ rejects $H_{0}$ for large values of

  $$F=\frac{\left\|\left(P-P_{0}\right) Y\right\|^{2} /\left(p-p_{0}\right)}{\|(I-P) Y\|^{2} /(n-p)} .$$

  Show that $(I-P)\left(P-P_{0}\right)=0$, and hence prove that the numerator and denominator of $F$ are independent under either hypothesis.

  Show that

  $$\mathbb{E}_{\beta, \sigma^{2}}(F)=\frac{(n-p)\left(\tau^{2}+1\right)}{n-p-2}$$

  where $\tau^{2}=\frac{\left\|\left(P-P_{0}\right) X \beta\right\|^{2}}{\left(p-p_{0}\right) \sigma^{2}}$.

  [In this question you may use the following facts without proof: $P-P_{0}$ is an orthogonal projection with rank $p-p_{0}$; any $n \times n$ orthogonal projection matrix $\Pi$ satisfies $\|\Pi \varepsilon\|^{2} \sim \sigma^{2} \chi_{\nu}^{2}$, where $\nu=\operatorname{rank}(\Pi) ;$ and if $Z \sim \chi_{\nu}^{2}$ then $\mathbb{E}\left(Z^{-1}\right)=(\nu-2)^{-1}$ when $\left.\nu>2 .\right]$

  comment

Statistical Physics

• Paper 1, Section II, E

  Statistical Physics | Part II, 2014

  Write down the equation of state and the internal energy of a monatomic ideal gas.

  Describe the meaning of an adiabatic process. Derive the equation for an adiabatic process in the pressure-volume $(P, V)$ plane for a monatomic ideal gas.

  Briefly describe the Carnot cycle. Sketch the Carnot cycle in the $(P, V)$ plane and in the temperature entropy $(T, S)$ plane.

  The Diesel cycle is an idealised version of the process realised in the Diesel engine. It consists of the following four reversible steps:

  

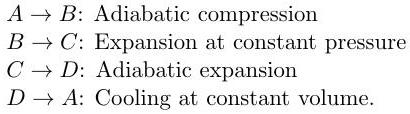
  Sketch the Diesel cycle for a monatomic gas in the $(P, V)$ plane and the $(T, S)$ plane. Determine the equations for the curves $B \rightarrow C$ and $D \rightarrow A$ in the $(T, S)$ plane.

  The efficiency $\eta$ of the cycle is defined as

  $$\eta=1-\frac{Q_{\text {out }}}{Q_{\text {in }}}$$

  where $Q_{\text {in }}$ is the heat entering the gas in step $B \rightarrow C$ and $Q_{\text {out }}$ is the heat leaving the gas in step $D \rightarrow A$. Calculate $\eta$ as a function of the temperatures at points $A, B, C$ and $D$.

  comment

Stochastic Financial Models

• Paper 1, Section II, K

  Stochastic Financial Models | Part II, 2014

  Suppose that $\bar{S}_{t} \equiv\left(S_{t}^{0}, \ldots, S_{t}^{d}\right)^{T}$ denotes the vector of prices of $d+1$ assets at times $t=0,1, \ldots$, and that $\bar{\theta}_{t} \equiv\left(\theta_{t}^{0}, \ldots, \theta_{t}^{d}\right)^{T}$ denotes the vector of the numbers of the $d+1$ different assets held by an investor from time $t-1$ to time $t$. Assuming that asset 0 is a bank account paying zero interest, that is, $S_{t}^{0}=1$ for all $t \geqslant 0$, explain what is meant by the statement that the portfolio process $\left(\bar{\theta}_{t}\right)_{t \geqslant 0}$ is self-financing. If the portfolio process is self-financing, prove that for any $t>0$

  $$\bar{\theta}_{t} \cdot \bar{S}_{t}-\bar{\theta}_{0} \cdot \bar{S}_{0}=\sum_{j=1}^{t} \theta_{j} \cdot \Delta S_{j}$$

  where $S_{j} \equiv\left(S_{j}^{1}, \ldots, S_{j}^{d}\right)^{T}, \Delta S_{j}=S_{j}-S_{j-1}$, and $\theta_{j} \equiv\left(\theta_{j}^{1}, \ldots, \theta_{j}^{d}\right)^{T}$.

  Suppose now that the $\Delta S_{t}$ are independent with common $N(0, V)$ distribution. Let

  $$F(z)=\inf E\left[\sum_{t \geqslant 1}(1-\beta) \beta^{t}\left\{\left(\bar{\theta}_{t} \cdot \bar{S}_{t}-\bar{\theta}_{0} \cdot \bar{S}_{0}\right)^{2}+\sum_{j=1}^{t}\left|\Delta \theta_{j}\right|^{2}\right\} \mid \theta_{0}=z\right]$$

  where $\beta \in(0,1)$ and the infimum is taken over all self-financing portfolio processes $\left(\bar{\theta}_{t}\right)_{t \geqslant 0}$ with $\theta_{0}^{0}=0$. Explain why $F$ should satisfy the equation

  $$F(z)=\beta \inf _{y}\left[y \cdot V y+|y-z|^{2}+F(y)\right]$$

  If $Q$ is a positive-definite symmetric matrix satisfying the equation

  $$Q=\beta(V+I+Q)^{-1}(V+Q)$$

  show that $(*)$ has a solution of the form $F(z)=z \cdot Q z$.

  comment

Topics in Analysis

• Paper 1, Section I, $2 \mathrm{G}$

  Topics in Analysis | Part II, 2014

  (i) State Brouwer's fixed point theorem in the plane and an equivalent theorem concerning mapping a triangle $T$ to its boundary $\partial T$.

  (ii) Let $A$ be a $3 \times 3$ matrix with positive real entries. Use the theorems you stated in (i) to prove that $A$ has an eigenvector with positive entries.

  comment

Waves

• Paper 1, Section II, C

  Waves | Part II, 2014

  State the equations that relate strain to displacement and stress to strain in a uniform, linear, isotropic elastic solid with Lamé moduli $\lambda$ and $\mu$. In the absence of body forces, the Cauchy momentum equation for the infinitesimal displacements $\mathbf{u}(\mathbf{x}, t)$ is

  $$\rho \frac{\partial^{2} \mathbf{u}}{\partial t^{2}}=\boldsymbol{\nabla} \cdot \boldsymbol{\sigma}$$

  where $\rho$ is the density and $\boldsymbol{\sigma}$ the stress tensor. Show that both the dilatation $\boldsymbol{\nabla} \cdot \mathbf{u}$ and the rotation $\nabla \wedge \mathbf{u}$ satisfy wave equations, and find the wave-speeds $c_{P}$ and $c_{S}$.

  A plane harmonic $\mathrm{P}$-wave with wavevector $\mathbf{k}$ lying in the $(x, z)$ plane is incident from $z<0$ at an oblique angle on the planar interface $z=0$ between two elastic solids with different densities and elastic moduli. Show in a diagram the directions of all the reflected and transmitted waves, labelled with their polarisations, assuming that none of these waves are evanescent. State the boundary conditions on components of $\mathbf{u}$ and $\boldsymbol{\sigma}$ that would, in principle, determine the amplitudes.

  Now consider a plane harmonic P-wave of unit amplitude incident with $\mathbf{k}=$ $k(\sin \theta, 0, \cos \theta)$ on the interface $z=0$ between two elastic (and inviscid) liquids with wave-speed $c_{P}$ and modulus $\lambda$ in $z<0$ and wave-speed $c_{P}^{\prime}$ and modulus $\lambda^{\prime}$ in $z>0$. Obtain solutions for the reflected and transmitted waves. Show that the amplitude of the reflected wave is zero if

  $$\sin ^{2} \theta=\frac{Z^{\prime 2}-Z^{2}}{Z^{\prime 2}-\left(c_{P}^{\prime} Z / c_{P}\right)^{2}}$$

  where $Z=\lambda / c_{P}$ and $Z^{\prime}=\lambda^{\prime} / c_{P}^{\prime}$

  comment