Write down the equation of state and the internal energy of a monatomic ideal gas.

Describe the meaning of an adiabatic process. Derive the equation for an adiabatic process in the pressure-volume $(P, V)$ plane for a monatomic ideal gas.

Briefly describe the Carnot cycle. Sketch the Carnot cycle in the $(P, V)$ plane and in the temperature entropy $(T, S)$ plane.

The Diesel cycle is an idealised version of the process realised in the Diesel engine. It consists of the following four reversible steps:

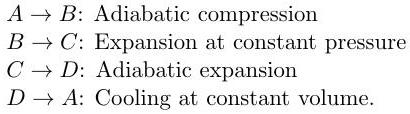
Sketch the Diesel cycle for a monatomic gas in the $(P, V)$ plane and the $(T, S)$ plane. Determine the equations for the curves $B \rightarrow C$ and $D \rightarrow A$ in the $(T, S)$ plane.

The efficiency $\eta$ of the cycle is defined as

$$\eta=1-\frac{Q_{\text {out }}}{Q_{\text {in }}}$$

where $Q_{\text {in }}$ is the heat entering the gas in step $B \rightarrow C$ and $Q_{\text {out }}$ is the heat leaving the gas in step $D \rightarrow A$. Calculate $\eta$ as a function of the temperatures at points $A, B, C$ and $D$.