Consider the following sum related to Riemann's zeta function:

$$S:=\sum_{m=1}^{\left[\frac{a}{2 \pi}\right]} m^{s-1}, \quad s=\sigma+i t, \sigma, t \in \mathbb{R}, \quad a>2 \pi, a \neq 2 \pi N, N \in \mathbb{Z}^{+},$$

where $[a / 2 \pi]$ denotes the integer part of $a / 2 \pi$.

(i) By using an appropriate branch cut, show that

$$S=\frac{e^{-\frac{i \pi s}{2}}}{(2 \pi)^{s}} \int_{L} f(z, s) d z, \quad f(z, s)=\frac{e^{-z}}{1-e^{-z}} z^{s-1}$$

where $L$ is the circle in the complex $z$-plane centred at $i(a+b) / 2$ with radius $(a-b) / 2$, $0<b<2 \pi$.

(ii) Use the above representation to show that, for $a>2 \pi$ and $0<b<2 \pi$,

$$\sum_{m=1}^{\left[\frac{a}{2 \pi}\right]} m^{s-1}=\frac{1}{(2 \pi)^{s}}\left[e^{-\frac{i \pi s}{2}} \int_{C_{b}^{a}} f(z, s) d z-e^{\frac{i \pi s}{2}} \int_{C_{-a}^{-b}} f(z, s) d z+\frac{a^{s}}{s}-\frac{b^{s}}{s}\right]$$

where $f(z, s)$ is defined in (i) and the curves $C_{b}^{a}, C_{-a}^{-b}$ are the following semi-circles in the right half complex $z$-plane:

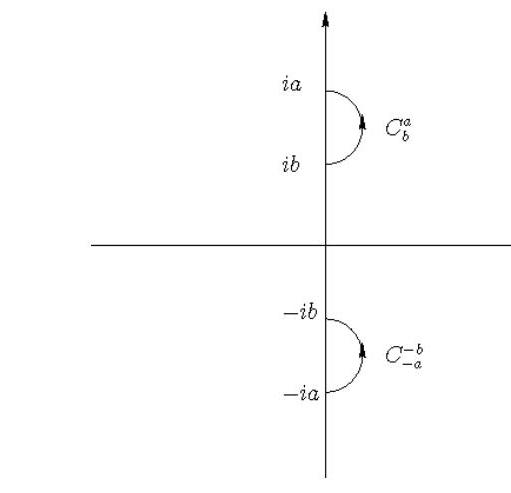
The curves $C_{b}^{a}$ and $C_{-a}^{-b}$.

$$\begin{aligned} C_{b}^{a} &=\left\{\frac{i(a+b)}{2}+\frac{(a-b)}{2} e^{i \theta}, \quad-\frac{\pi}{2}<\theta<\frac{\pi}{2}\right\} \\ C_{-a}^{-b} &=\left\{\frac{-i(a+b)}{2}+\frac{(a-b)}{2} e^{i \theta}, \quad-\frac{\pi}{2}<\theta<\frac{\pi}{2}\right\} \end{aligned}$$

Part II, 2011 List of Questions

[TURN OVER