Let $\left(X_{t}\right)$ be an irreducible continuous-time Markov chain with countably many states. What does it mean to say the chain is (i) positive recurrent, (ii) null recurrent? Consider the chain $\left(X_{t}\right)$ with the arrow diagram below.

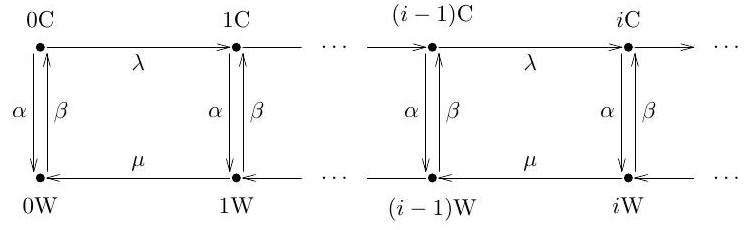
In this question we analyse the existence of equilibrium probabilities $\pi_{i \mathrm{C}}$ and $\pi_{i \mathrm{~W}}$ of the chain $\left(X_{t}\right)$ being in state $i \mathrm{C}$ or $i \mathrm{~W}, i=0,1, \ldots$, and the impact of this fact on positive and null recurrence of the chain.

(a) Write down the invariance equations $\pi Q=0$ and check that they have the form

$$\begin{aligned} \pi_{0 C} &=\frac{\beta}{\lambda+\alpha} \pi_{0 \mathrm{~W}}, \\ \left(\pi_{1 \mathrm{C}}, \pi_{1 \mathrm{~W}}\right) &=\frac{\beta \pi_{0 \mathrm{~W}}}{\lambda+\alpha}\left(\frac{\lambda(\mu+\beta)}{\mu(\lambda+\alpha)}, \frac{\lambda}{\mu}\right) \\ \left(\pi_{(i+1) \mathrm{C}}, \pi_{(i+1) \mathrm{W}}\right) &=\left(\pi_{i \mathrm{C}}, \pi_{i \mathrm{~W}}\right) B, \quad i=1,2, \ldots, \end{aligned}$$

where $B$ is a $2 \times 2$ recursion matrix:

$$B=\left(\begin{array}{cc} \frac{\lambda \mu-\beta \alpha}{\mu(\lambda+\alpha)} & -\frac{\alpha}{\mu} \\ \frac{\beta(\beta+\mu)}{\mu(\lambda+\alpha)} & \frac{\beta+\mu}{\mu} \end{array}\right)$$

(b) Verify that the row vector $\left(\pi_{1 \mathrm{C}}, \pi_{1 \mathrm{~W}}\right)$ is an eigenvector of $B$ with the eigenvalue $\theta$ where

$$\theta=\frac{\lambda(\mu+\beta)}{\mu(\lambda+\alpha)}$$

Hence, specify the form of equilibrium probabilities $\pi_{i \mathrm{C}}$ and $\pi_{i \mathrm{~W}}$ and conclude that the chain $\left(X_{t}\right)$ is positive recurrent if and only if $\mu \alpha>\lambda \beta$.