Part II, 2004, Paper 4

Algebraic Curves

• B4.9

  Algebraic Curves | Part II, 2004

  Let $F(X, Y, Z)$ be an irreducible homogeneous polynomial of degree $n$, and write $C=\left\{p \in \mathbb{P}^{2} \mid F(p)=0\right\}$ for the curve it defines in $\mathbb{P}^{2}$. Suppose $C$ is smooth. Show that the degree of its canonical class is $n(n-3)$.

  Hence, or otherwise, show that a smooth curve of genus 2 does not embed in $\mathbb{P}^{2}$.

  comment

Algebraic Topology

• B4.5

  Algebraic Topology | Part II, 2004

  Write down the definition of a covering space and a covering map. State and prove the path lifting property for covering spaces and state, without proof, the homotopy lifting property.

  Suppose that a group $G$ is a group of homeomorphisms of a space $X$. Prove that, under conditions to be stated, the quotient map $X \rightarrow X / G$ is a covering map and that $\pi_{1}(X / G)$ is isomorphic to $G$. Give two examples in which this last result can be used to determine the fundamental group of a space.

  comment

Algorithms and Networks

• A4.11

  Algorithms and Networks | Part II, 2004

  (i) Consider an unrestricted geometric programming problem

  $$\min g(t), \quad t=\left(t_{1}, \ldots, t_{m}\right)>0,$$

  where $g(t)$ is given by

  $$g(t)=\sum_{i=1}^{n} c_{i} t_{1}^{a_{i 1}} \ldots t_{m}^{a_{i m}}$$

  with $n \geq m$ and positive coefficients $c_{1} \ldots, c_{n}$. State the dual problem of $(*)$ and show that if $\lambda^{*}=\left(\lambda_{1}^{*}, \ldots, \lambda_{n}^{*}\right)$ is a dual optimum then any positive solution to the system

  $$t_{1}^{a_{i 1}} \ldots t_{m}^{a_{i m}}=\frac{1}{c_{i}} \lambda_{i}^{*} v\left(\lambda^{*}\right), \quad i=1, \ldots, n,$$

  gives an optimum for primal problem $(*)$. Here $v(\lambda)$ is the dual objective function.

  (ii) An amount of ore has to be moved from a pit in an open rectangular skip which is to be ordered from a supplier.

  The skip cost is $£ 36$ per $1 \mathrm{~m}^{2}$ for the bottom and two side walls and $£ 18$ per $1 \mathrm{~m}^{2}$ for the front and the back walls. The cost of loading ore into the skip is $£ 3$ per $1 \mathrm{~m}^{3}$, the cost of lifting is $£ 2$ per $1 \mathrm{~m}^{3}$, and the cost of unloading is $£ 1$ per $1 \mathrm{~m}^{3}$. The cost of moving an empty skip is negligible.

  Write down an unconstrained geometric programming problem for the optimal size (length, width, height) of skip minimizing the cost of moving $48 \mathrm{~m}^{3}$ of ore. By considering the dual problem, or otherwise, find the optimal cost and the optimal size of the skip.

  comment

Applications of Quantum Mechanics

• B4.24

  Applications of Quantum Mechanics | Part II, 2004

  Describe briefly the variational approach to determining approximate energy eigenvalues for a Hamiltonian $H$.

  Consider a Hamiltonian $H$ and two states $\left|\psi_{1}\right\rangle,\left|\psi_{2}\right\rangle$ such that

  $$\begin{array}{cl} \left\langle\psi_{1}|H| \psi_{1}\right\rangle=\left\langle\psi_{2}|H| \psi_{2}\right\rangle=\mathcal{E}, & \left\langle\psi_{2}|H| \psi_{1}\right\rangle=\left\langle\psi_{1}|H| \psi_{2}\right\rangle=\varepsilon \\ \left\langle\psi_{1} \mid \psi_{1}\right\rangle=\left\langle\psi_{2} \mid \psi_{2}\right\rangle=1, & \left\langle\psi_{2} \mid \psi_{1}\right\rangle=\left\langle\psi_{1} \mid \psi_{2}\right\rangle=s \end{array}$$

  Show that, by considering a linear combination $\alpha\left|\psi_{1}\right\rangle+\beta\left|\psi_{2}\right\rangle$, the variational method gives

  $$\frac{\mathcal{E}-\varepsilon}{1-s}, \quad \frac{\mathcal{E}+\varepsilon}{1+s}$$

  as approximate energy eigenvalues.

  Consider the Hamiltonian for an electron in the presence of two protons at $\mathbf{0}$ and $\mathbf{R}$,

  $$H=\frac{\mathbf{p}^{2}}{2 m}+\frac{e^{2}}{4 \pi \epsilon_{0}}\left(\frac{1}{R}-\frac{1}{|\mathbf{r}|}-\frac{1}{|\mathbf{r}-\mathbf{R}|}\right), \quad R=|\mathbf{R}|$$

  Let $\psi_{0}(\mathbf{r})=e^{-r / a} /\left(\pi a^{3}\right)^{\frac{1}{2}}$ be the ground state hydrogen atom wave function which satisfies

  $$\left(\frac{\mathbf{p}^{2}}{2 m}-\frac{e^{2}}{4 \pi \epsilon_{0}|\mathbf{r}|}\right) \psi_{0}(\mathbf{r})=E_{0} \psi_{0}(\mathbf{r}) .$$

  It is given that

  $$\begin{aligned} &S=\int \mathrm{d}^{3} r \psi_{0}(\mathbf{r}) \psi_{0}(\mathbf{r}-\mathbf{R})=\left(1+\frac{R}{a}+\frac{R^{2}}{3 a^{2}}\right) e^{-R / a} \\ &U=\int \mathrm{d}^{3} r \frac{1}{|\mathbf{r}|} \psi_{0}(\mathbf{r}) \psi_{0}(\mathbf{r}-\mathbf{R})=\frac{1}{a}\left(1+\frac{R}{a}\right) e^{-R / a} \end{aligned}$$

  and, for large $R$, that

  $$\int \mathrm{d}^{3} r \frac{1}{|\mathbf{r}-\mathbf{R}|} \psi_{0}(\mathbf{r})^{2}-\frac{1}{R}=\mathrm{O}\left(e^{-2 R / a}\right)$$

  Consider the trial wave function $\alpha \psi_{0}(\mathbf{r})+\beta \psi_{0}(\mathbf{r}-\mathbf{R})$. Show that the variational estimate for the ground state energy for large $R$ is

  $$E(R)=E_{0}+\frac{e^{2}}{4 \pi \epsilon_{0} R}(S-R U)+\mathrm{O}\left(e^{-2 R / a}\right) .$$

  Explain why there is an attractive force between the two protons for large $R$.

  comment

Applied Probability

• B4.12

  Applied Probability | Part II, 2004

  Consider an $M / G / 1$ queue with $\rho=\lambda \mathbb{E} S<1$. Here $\lambda$ is the arrival rate and $\mathbb{E} S$ is the mean service time. Prove that in equilibrium, the customer's waiting time $W$ has the moment-generating function $M_{W}(t)=\mathbb{E} e^{t W}$ given by

  $$M_{W}(t)=\frac{(1-\rho) t}{t+\lambda\left(1-M_{S}(t)\right)}$$

  where $M_{S}(t)=\mathbb{E} e^{t S}$ is the moment-generating function of service time $S$.

  [You may assume that in equilibrium, the $M / G / 1$ queue size $X$ at the time immediately after the customer's departure has the probability generating function

  $$\left.\mathbb{E} z^{X}=\frac{(1-\rho)(1-z) M_{S}(\lambda(z-1))}{M_{S}(\lambda(z-1))-z}, \quad 0 \leqslant z<1 .\right]$$

  Deduce that when the service times are exponential of rate $\mu$ then

  $$M_{W}(t)=1-\rho+\frac{\lambda(1-\rho)}{\mu-\lambda-t}, \quad-\infty<t<\mu-\lambda .$$

  Further, deduce that $W$ takes value 0 with probability $1-\rho$ and that

  $$\mathbb{P}(W>x \mid W>0)=e^{-(\mu-\lambda) x}, \quad x>0 .$$

  Sketch the graph of $\mathbb{P}(W>x)$ as a function of $x$.

  Now consider the $M / G / 1$ queue in the heavy traffic approximation, when the service-time distribution is kept fixed and the arrival rate $\lambda \rightarrow 1 / \mathbb{E} S$, so that $\rho \rightarrow 1$. Assuming that the second moment $\mathbb{E} S^{2}<\infty$, check that the limiting distribution of the re-scaled waiting time $\tilde{W}_{\lambda}=(1-\lambda \mathbb{E} S) W$ is exponential, with rate $2 \mathbb{E} S / \mathbb{E} S^{2}$.

  comment

Combinatorics

• B4.1

  Combinatorics | Part II, 2004

  Write an essay on Ramsey's theorem. You should include the finite and infinite versions, together with some discussion of bounds in the finite case, and give at least one application.

  comment

Computational Statistics and Statistical Modelling

• A4.14

  Computational Statistics and Statistical Modelling | Part II, 2004

  Suppose that $Y_{1}, \ldots, Y_{n}$ are independent observations, with $Y_{i}$ having probability density function of the following form

  $$f\left(y_{i} \mid \theta_{i}, \phi\right)=\exp \left[\frac{y_{i} \theta_{i}-b\left(\theta_{i}\right)}{\phi}+c\left(y_{i}, \phi\right)\right]$$

  where $\mathbb{E}\left(Y_{i}\right)=\mu_{i}$ and $g\left(\mu_{i}\right)=\beta^{T} x_{i}$. You should assume that $g()$ is a known function, and $\beta, \phi$ are unknown parameters, with $\phi>0$, and also $x_{1}, \ldots, x_{n}$ are given linearly independent covariate vectors. Show that

  $$\frac{\partial \ell}{\partial \beta}=\sum \frac{\left(y_{i}-\beta_{i}\right)}{g^{\prime}\left(\mu_{i}\right) V_{i}} x_{i}$$

  where $\ell$ is the log-likelihood and $V_{i}=\operatorname{var}\left(Y_{i}\right)=\phi b^{\prime \prime}\left(\theta_{i}\right)$.

  Discuss carefully the (slightly edited) $\mathrm{R}$ output given below, and briefly suggest another possible method of analysis using the function $\mathrm{glm}$ ( ).

  $>s<-\operatorname{scan}()$

  1: $\begin{array}{llllll}33 & 63 & 157 & 38 & 108 & 159\end{array}$

  7:

  Read 6 items

  $>r<-\operatorname{scan}()$

  1: 327172565065248688773520

  $7:$

  Read 6 items

  $>$ gender <- $\operatorname{scan}(, " \|)$

  1: b b b g g g

  $7:$

  Read 6 items

  $>$ age <- $\operatorname{scan}(, " \prime)$

  1: 13&under 14-18 19&over

  4: 13&under 14-18 19&over

  7 :

  Read 6 items

  $>$ gender <- factor (gender) ; age <- factor (age)

  $>\operatorname{summary}(\mathrm{glm}(\mathrm{s} / \mathrm{r} \sim$ gender $+$ age, binomial, weights $=\mathrm{r}))$

  Coefficients:

  

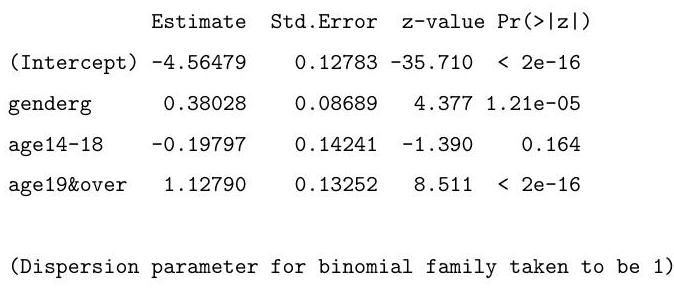
  Null deviance: $221.797542$ on 5 degrees of freedom

  Residual deviance: $0.098749$ on 2 degrees of freedom

  Number of Fisher Scoring iterations: 3

  comment

Differentiable Manifolds

• B4.4

  Differentiable Manifolds | Part II, 2004

  Define what it means for a manifold to be oriented, and define a volume form on an oriented manifold.

  Prove carefully that, for a closed connected oriented manifold of dimension $n$, $H^{n}(M)=\mathbb{R}$.

  [You may assume the existence of volume forms on an oriented manifold.]

  If $M$ and $N$ are closed, connected, oriented manifolds of the same dimension, define the degree of a map $f: M \rightarrow N$.

  If $f$ has degree $d>1$ and $y \in N$, can $f^{-1}(y)$ be

  (i) infinite? (ii) a single point? (iii) empty?

  Briefly justify your answers.

  comment

Electrodynamics

• B4.21

  Electrodynamics | Part II, 2004

  Using Lorentz gauge, $A_{, a}^{a}=0$, Maxwell's equations for a current distribution $J^{a}$ can be reduced to $\square A^{a}(x)=\mu_{0} J^{a}(x)$. The retarded solution is

  $$A^{a}(x)=\frac{\mu_{0}}{2 \pi} \int d^{4} y \theta\left(z^{0}\right) \delta\left(z_{c} z^{c}\right) J^{a}(y)$$

  where $z^{a}=x^{a}-y^{a}$. Explain, heuristically, the rôle of the $\delta$-function and Heaviside step function $\theta$ in this formula.

  The current distribution is produced by a point particle of charge $q$ moving on a world line $r^{a}(s)$, where $s$ is the particle's proper time, so that

  $$J^{a}(y)=q \int d s V^{a}(s) \delta^{(4)}(y-r(s))$$

  where $V^{a}=\dot{r}^{a}(s)=d r^{a} / d s$. Show that

  $$A^{a}(x)=\frac{\mu_{0} q}{2 \pi} \int d s \theta\left(X^{0}\right) \delta\left(X_{c} X^{c}\right) V^{a}(s),$$

  where $X^{a}=x^{a}-r^{a}(s)$, and further that, setting $\alpha=X_{c} V^{c}$,

  $$A^{a}(x)=\frac{\mu_{0} q}{4 \pi}\left[\frac{V^{a}}{\alpha}\right]_{s=s^{*}}$$

  where $s^{*}$ should be defined. Verify that

  $$s^{*}, a=\left[\frac{X_{a}}{\alpha}\right]_{s=s^{*}} .$$

  Evaluating quantities at $s=s^{*}$ show that

  $$\left[\frac{V^{a}}{\alpha}\right]_{, b}=\frac{1}{\alpha^{2}}\left[-V^{a} V_{b}+S^{a} X_{b}\right]$$

  where $S^{a}=\dot{V}^{a}+V^{a}\left(1-X_{c} \dot{V}^{c}\right) / \alpha$. Hence verify that $A^{a}{ }_{, a}(x)=0$ and

  $$F_{a b}=\frac{\mu_{0} q}{4 \pi \alpha^{2}}\left(S_{a} X_{b}-S_{b} X_{a}\right) .$$

  Verify this formula for a stationary point charge at the origin.

  [Hint: If $f(s)$ has simple zeros at $s_{i}, i=1,2, \ldots$ then

  $$\delta(f(s))=\sum_{i} \frac{\delta\left(s_{i}\right)}{\left|f^{\prime}\left(s_{i}\right)\right|}$$

  comment

Electromagnetism

• A4.5

  Electromagnetism | Part II, 2004

  Consider a frame $S^{\prime}$ moving with velocity v relative to the laboratory frame $S$ where $|\mathbf{v}|^{2} \ll c^{2}$. The electric and magnetic fields in $S$ are $\mathbf{E}$ and $\mathbf{B}$, while those measured in $S^{\prime}$ are $\mathbf{E}^{\prime}$ and $\mathbf{B}^{\prime}$. Given that $\mathbf{B}^{\prime}=\mathbf{B}$, show that

  $$\oint_{\Gamma} \mathbf{E}^{\prime} \cdot d \mathbf{l}=\oint_{\Gamma}(\mathbf{E}+\mathbf{v} \wedge \mathbf{B}) \cdot d \mathbf{l},$$

  for any closed circuit $\Gamma$ and hence that $\mathbf{E}^{\prime}=\mathbf{E}+\mathbf{v} \wedge \mathbf{B}$.

  Now consider a fluid with electrical conductivity $\sigma$ and moving with velocity $\mathbf{v}(\mathbf{r})$. Use Ohm's law in the moving frame to relate the current density $\mathbf{j}$ to the electric field $\mathbf{E}$ in the laboratory frame, and show that if $\mathbf{j}$ remains finite in the limit $\sigma \rightarrow \infty$ then

  $$\frac{\partial \mathbf{B}}{\partial t}=\nabla \wedge(\mathbf{v} \wedge \mathbf{B})$$

  The magnetic helicity $H$ in a volume $V$ is given by $\int_{V} \mathbf{A} \cdot \mathbf{B} d \tau$ where $\mathbf{A}$ is the vector potential. Show that if the normal components of $\mathbf{v}$ and $\mathbf{B}$ both vanish on the surface bounding $V$ then $d H / d t=0$.

  comment

Fluid Dynamics II

• B4.26

  Fluid Dynamics II | Part II, 2004

  Write an essay on the Kelvin-Helmholtz instability of a vortex sheet. Your essay should include a detailed linearised analysis, a physical interpretation of the instability, and an informal discussion of nonlinear effects and of the effects of viscosity.

  comment

Foundations of Quantum Mechanics

• A4.15 B4.22

  Foundations of Quantum Mechanics | Part II, 2004

  The states of the hydrogen atom are denoted by $|n l m\rangle$ with $l<n,-l \leq m \leq l$ and associated energy eigenvalue $E_{n}$, where

  $$E_{n}=-\frac{e^{2}}{8 \pi \epsilon_{0} a_{0} n^{2}} .$$

  A hydrogen atom is placed in a weak electric field with interaction Hamiltonian

  $$H_{1}=-e \mathcal{E} z$$

  a) Derive the necessary perturbation theory to show that to $O\left(\mathcal{E}^{2}\right)$ the change in the energy associated with the state $|100\rangle$ is given by

  $$\Delta E_{1}=e^{2} \mathcal{E}^{2} \sum_{n=2}^{\infty} \sum_{l=0}^{n-1} \sum_{m=-l}^{l} \frac{|\langle 100|z| n l m\rangle|^{2}}{E_{1}-E_{n}}$$

  The wavefunction of the ground state $|100\rangle$ is

  $$\psi_{n=1}(\mathbf{r})=\frac{1}{\left(\pi a_{0}^{3}\right)^{1 / 2}} e^{-r / a_{0}}$$

  By replacing $E_{n}, \forall n>1$, in the denominator of $(*)$ by $E_{2}$ show that

  $$\left|\Delta E_{1}\right|<\frac{32 \pi}{3} \epsilon_{0} \mathcal{E}^{2} a_{0}^{3}$$

  b) Find a matrix whose eigenvalues are the perturbed energies to $O(\mathcal{E})$ for the states $|200\rangle$ and $|210\rangle$. Hence, determine these perturbed energies to $O(\mathcal{E})$ in terms of the matrix elements of $z$ between these states.

  [Hint:

  $$\begin{aligned} \langle n l m|z| n l m\rangle &=0 & & \forall n, l, m \\ \left\langle n l m|z| n l^{\prime} m^{\prime}\right\rangle &=0 & & \forall n, l, l^{\prime}, m, m^{\prime}, \quad m \neq m^{\prime} \end{aligned}$$

  comment

Functional Analysis

• A4.3

  Functional Analysis | Part II, 2004

  State and prove the Dominated Convergence Theorem. [You may assume the Monotone Convergence Theorem.]

  Let $a$ and $p$ be real numbers, with $a>0$. Prove carefully that

  $$\int_{0}^{\infty} e^{-a x} \sin p x d x=\frac{p}{a^{2}+p^{2}}$$

  [Any standard results that you use should be stated precisely.]

  comment

Galois Theory

• B4.3

  Galois Theory | Part II, 2004

  Let $M / K$ be a finite Galois extension of fields. Explain what is meant by the Galois correspondence between subfields of $M$ containing $K$ and subgroups of $\operatorname{Gal}(M / K)$. Show that if $K \subset L \subset M$ then $\operatorname{Gal}(M / L)$ is a normal subgroup of $\operatorname{Gal}(M / K)$ if and only if $L / K$ is normal. What is $\operatorname{Gal}(L / K)$ in this case?

  Let $M$ be the splitting field of $X^{4}-3$ over $\mathbb{Q}$. Prove that $\operatorname{Gal}(M / \mathbb{Q})$ is isomorphic to the dihedral group of order 8. Hence determine all subfields of $M$, expressing each in the form $\mathbb{Q}(x)$ for suitable $x \in M$.

  comment

General Relativity

• A4.17 B4.25

  General Relativity | Part II, 2004

  Starting from the Ricci identity

  $$V_{a ; b ; c}-V_{a ; c ; b}=V_{e} R_{a b c}^{e}$$

  give an expression for the curvature tensor $R_{a b c}^{e}$ of the Levi-Civita connection in terms of the Christoffel symbols and their partial derivatives. Using local inertial coordinates, or otherwise, establish that

  $$R_{a b c}^{e}+R_{b c a}^{e}+R_{c a b}^{e}=0 .$$

  A vector field with components $V^{a}$ satisfies

  $$V_{a ; b}+V_{b ; a}=0$$

  Show, using equation $(*)$ that

  $$V_{a ; b ; c}=V_{e} R_{c b a}^{e}$$

  and hence that

  $$V_{a ; b} ; b+R_{a}{ }^{c} V_{c}=0,$$

  where $R_{a b}$ is the Ricci tensor. Show that equation $(* *)$ may be written as

  $$\left(\partial_{c} g_{a b}\right) V^{c}+g_{c b} \partial_{a} V^{c}+g_{a c} \partial_{b} V^{c}=0$$

  If the metric is taken to be the Schwarzschild metric

  $$d s^{2}=-\left(1-\frac{2 M}{r}\right) d t^{2}+\left(1-\frac{2 M}{r}\right)^{-1} d r^{2}+r^{2}\left(d \theta^{2}+\sin ^{2} \theta d \phi^{2}\right)$$

  show that $V^{a}=\delta^{a}{ }_{0}$ is a solution of $(* * *)$. Calculate $V^{a} ; a$.

  Electromagnetism can be described by a vector potential $A_{a}$ and a Maxwell field tensor $F_{a b}$ satisfying

  $$F_{a b}=A_{b ; a}-A_{a ; b} \quad \text { and } \quad F_{a b} ; b=0 .$$

  The divergence of $A_{a}$ is arbitrary and we may choose $A_{a} ; a=0$. With this choice show that in a general spacetime

  $$A_{a ; b}{ }^{; b}-R_{a}{ }^{c} A_{c}=0 .$$

  Hence show that in the Schwarzschild spacetime a tensor field whose only non-trivial components are $F_{t r}=-F_{r t}=Q / r^{2}$, where $Q$ is a constant, satisfies the field equations $(* * * *)$.

  comment

Geometry of Surfaces

• A4.7

  Geometry of Surfaces | Part II, 2004

  Write an essay on the Gauss-Bonnet theorem and its proof.

  comment

Graph Theory

• A4.9

  Graph Theory | Part II, 2004

  Write an essay on trees. You should include a proof of Cayley's result on the number of labelled trees of order $n$.

  Let $G$ be a graph of order $n \geq 2$. Which of the following statements are equivalent to the statement that $G$ is a tree? Give a proof or counterexample in each case.

  (a) $G$ is acyclic and $e(G) \geq n-1$.

  (b) $G$ is connected and $e(G) \leq n-1$.

  (c) $G$ is connected, triangle-free and has at least two leaves.

  (d) $G$ has the same degree sequence as $T$, for some tree $T$.

  comment

Groups, Rings and Fields

• A4.4

  Groups, Rings and Fields | Part II, 2004

  (a) Let $t$ be the maximal power of the prime $p$ dividing the order of the finite group $G$, and let $N\left(p^{t}\right)$ denote the number of subgroups of $G$ of order $p^{t}$. State clearly the numerical restrictions on $N\left(p^{t}\right)$ given by the Sylow theorems.

  If $H$ and $K$ are subgroups of $G$ of orders $r$ and $s$ respectively, and their intersection $H \cap K$ has order $t$, show the set $H K=\{h k: h \in H, k \in K\}$ contains $r s / t$ elements.

  (b) The finite group $G$ has 48 elements. By computing the possible values of $N(16)$, show that $G$ cannot be simple.

  comment

Hilbert Spaces

• B4.7

  Hilbert Spaces | Part II, 2004

  Suppose that $T$ is a bounded linear operator on an infinite-dimensional Hilbert space $H$, and that $\langle T(x), x\rangle$ is real and non-negative for each $x \in H$.

  (a) Show that $T$ is Hermitian.

  (b) Let $w(T)=\sup \{\langle T(x), x\rangle:\|x\|=1\}$. Show that

  $$\|T(x)\|^{2} \leqslant w(T)\langle T(x), x\rangle \quad \text { for each } x \in H$$

  (c) Show that $\|T\|$ is an approximate eigenvalue for $T$.

  Suppose in addition that $T$ is compact and injective.

  (d) Show that $\|T\|$ is an eigenvalue for $T$, with finite-dimensional eigenspace.

  Explain how this result can be used to diagonalise $T$.

  comment

Information Theory

• B4.13

  Information Theory | Part II, 2004

  Define a cyclic code of length $N$.

  Show how codewords can be identified with polynomials in such a way that cyclic codes correspond to ideals in the polynomial ring with a suitably chosen multiplication rule.

  Prove that any cyclic code $\mathcal{X}$ has a unique generator, i.e. a polynomial $c(X)$ of minimum degree, such that the code consists of the multiples of this polynomial. Prove that the rank of the code equals $N-\operatorname{deg} c(X)$, and show that $c(X)$ divides $X^{N}+1$.

  Let $\mathcal{X}$ be a cyclic code. Set

  $$\mathcal{X}^{\perp}=\left\{y=y_{1} \ldots y_{N}: \sum_{i=1}^{N} x_{i} y_{i}=0 \text { for all } x=x_{1} \ldots x_{N} \in \mathcal{X}\right\}$$

  (the dual code). Prove that $\mathcal{X}^{\perp}$ is cyclic and establish how the generators of $\mathcal{X}$ and $\mathcal{X}^{\perp}$ are related to each other.

  Show that the repetition and parity codes are cyclic, and determine their generators.

  comment

Logic, Computation and Set Theory

• A4.8 B4.10

  Logic, Computation and Set Theory | Part II, 2004

  Write an essay on recursive functions. Your essay should include a sketch of why every computable function is recursive, and an explanation of the existence of a universal recursive function, as well as brief discussions of the Halting Problem and of the relationship between recursive sets and recursively enumerable sets.

  [You may assume that every recursive function is computable. You do not need to give proofs that particular functions to do with prime-power decompositions are recursive.]

  comment

Markov Chains

• A4.1

  Markov Chains | Part II, 2004

  (a) Give three definitions of a continuous-time Markov chain with a given $Q$-matrix on a finite state space: (i) in terms of holding times and jump probabilities, (ii) in terms of transition probabilities over small time intervals, and (iii) in terms of finite-dimensional distributions.

  (b) A flea jumps clockwise on the vertices of a triangle; the holding times are independent exponential random variables of rate one. Find the eigenvalues of the corresponding $Q$-matrix and express transition probabilities $p_{x y}(t), t \geq 0, x, y=A, B, C$, in terms of these roots. Deduce the formulas for the sums

  $$S_{0}(t)=\sum_{n=0}^{\infty} \frac{t^{3 n}}{(3 n) !}, \quad S_{1}(t)=\sum_{n=0}^{\infty} \frac{t^{3 n+1}}{(3 n+1) !}, \quad S_{2}(t)=\sum_{n=0}^{\infty} \frac{t^{3 n+2}}{(3 n+2) !}$$

  in terms of the functions $e^{t}, e^{-t / 2}, \cos (\sqrt{3} t / 2)$ and $\sin (\sqrt{3} t / 2)$.

  Find the limits

  $$\lim _{t \rightarrow \infty} e^{-t} S_{j}(t), \quad j=0,1,2 .$$

  What is the connection between the decompositions $e^{t}=S_{0}(t)+S_{1}(t)+S_{2}(t)$ and $e^{t}=\cosh t+\sinh t ?$

  comment

Mathematical Methods

• A4.21

  Mathematical Methods | Part II, 2004

  State Watson's lemma, describing the asymptotic behaviour of the integral

  $$I(\lambda)=\int_{0}^{A} e^{-\lambda t} f(t) d t, \quad A>0$$

  as $\lambda \rightarrow \infty$, given that $f(t)$ has the asymptotic expansion

  $$f(t) \sim \sum_{n=0}^{\infty} a_{n} t^{n \beta}$$

  as $t \rightarrow 0_{+}$, where $\beta>0$.

  Consider the integral

  $$J(\lambda)=\int_{a}^{b} e^{\lambda \phi(t)} F(t) d t,$$

  where $\lambda \gg 1$ and $\phi(t)$ has a unique maximum in the interval $[a, b]$ at $c$, with $a<c<b$, such that

  $$\phi^{\prime}(c)=0, \quad \phi^{\prime \prime}(c)<0 .$$

  By using the change of variable from $t$ to $\zeta$, defined by

  $$\phi(t)-\phi(c)=-\zeta^{2}$$

  deduce an asymptotic expansion for $J(\lambda)$ as $\lambda \rightarrow \infty$. Show that the leading-order term gives

  $$J(\lambda) \sim e^{\lambda \phi(c)} F(c)\left(\frac{2 \pi}{\lambda\left|\phi^{\prime \prime}(c)\right|}\right)^{\frac{1}{2}}$$

  The gamma function $\Gamma(x)$ is defined for $x>0$ by

  $$\Gamma(x)=\int_{0}^{\infty} e^{(x-1) \log t-t} d t$$

  By means of the substitution $t=(x-1) s$, or otherwise, deduce that

  $$\Gamma(x+1) \sim x^{\left(x+\frac{1}{2}\right)} e^{-x} \sqrt{2 \pi}\left(1+\frac{1}{12 x}+\ldots\right)$$

  as $x \rightarrow \infty$

  comment

Methods of Mathematical Physics

• B4.19

  Methods of Mathematical Physics | Part II, 2004

  Let $h(t)=i\left(t+t^{2}\right)$. Sketch the path of $\operatorname{Im}(h(t))=$ const. through the point $t=0$, and the path of $\operatorname{Im}(h(t))=$ const. through the point $t=1$.

  By integrating along these paths, show that as $\lambda \rightarrow \infty$

  $$\int_{0}^{1} t^{-1 / 2} e^{i \lambda\left(t+t^{2}\right)} d t \sim \frac{c_{1}}{\lambda^{1 / 2}}+\frac{c_{2} e^{2 i \lambda}}{\lambda},$$

  where the constants $c_{1}$ and $c_{2}$ are to be computed.

  comment

Nonlinear Dynamical Systems

• A4.6 B4.17

  Nonlinear Dynamical Systems | Part II, 2004

  (a) Consider the map $G_{1}(x)=f(x+a)$, defined on $0 \leqslant x<1$, where $f(x)=x[\bmod 1]$, $0 \leqslant f<1$, and the constant $a$ satisfies $0 \leqslant a<1$. Give, with reasons, the values of $a$ (if any) for which the map has (i) a fixed point, (ii) a cycle of least period $n$, (iii) an aperiodic orbit. Does the map exhibit sensitive dependence on initial conditions?

  Show (graphically if you wish) that if the map has an $n$-cycle then it has an infinite number of such cycles. Is this still true if $G_{1}$ is replaced by $f(c x+a), 0<c<1 ?$

  (b) Consider the map

  $$G_{2}(x)=f\left(x+a+\frac{b}{2 \pi} \sin 2 \pi x\right)$$

  where $f(x)$ and $a$ are defined as in Part (a), and $b>0$ is a parameter.

  Find the regions of the $(a, b)$ plane for which the map has (i) no fixed points, (ii) exactly two fixed points.

  Now consider the possible existence of a 2-cycle of the map $G_{2}$ when $b \ll 1$, and suppose the elements of the cycle are $X, Y$ with $X<\frac{1}{2}$. By expanding $X, Y, a$ in powers of $b$, so that $X=X_{0}+b X_{1}+b^{2} X_{2}+O\left(b^{3}\right)$, and similarly for $Y$ and $a$, show that

  $$a=\frac{1}{2}+\frac{b^{2}}{8 \pi} \sin 4 \pi X_{0}+O\left(b^{3}\right)$$

  Use this result to sketch the region of the $(a, b)$ plane in which 2-cycles exist. How many distinct cycles are there for each value of $a$ in this region?

  comment

Nonlinear Waves

• A4.23

  Nonlinear Waves | Part II, 2004

  Let $\psi(k ; x, t)$ satisfy the linear integral equation

  $$\psi(k ; x, t)+i e^{i\left(k x+k^{3} t\right)} \int_{L} \frac{\psi(l ; x, t)}{l+k} d \lambda(l)=e^{i\left(k x+k^{3} t\right)}$$

  where the measure $d \lambda(k)$ and the contour $L$ are such that $\psi(k ; x, t)$ exists and is unique.

  Let $q(x, t)$ be defined in terms of $\psi(k ; x, t)$ by

  $$q(x, t)=-\frac{\partial}{\partial x} \int_{L} \psi(k ; x, t) d \lambda(k)$$

  (a) Show that

  $$(M \psi)+i e^{i\left(k x+k^{3} t\right)} \int_{L} \frac{(M \psi)(l ; x, t)}{l+k} d \lambda(l)=0$$

  where

  $$M \psi \equiv \frac{\partial^{2} \psi}{\partial x^{2}}-i k \frac{\partial \psi}{\partial x}+q \psi$$

  (b) Show that

  $(N \psi)+i e^{i\left(k x+k^{3} t\right)} \int_{L} \frac{(N \psi)(l ; x, t)}{l+k} d \lambda(l)=3 k e^{i\left(k x+k^{3} t\right)} \int_{L} \frac{(M \psi)(l ; x, t)}{l+k} d \lambda(l)$,

  where

  $$N \psi \equiv \frac{\partial \psi}{\partial t}+\frac{\partial^{3} \psi}{\partial x^{3}}+3 q \frac{\partial \psi}{\partial x}$$

  (c) By recalling that the $\mathrm{KdV}$ equation

  $$\frac{\partial q}{\partial t}+\frac{\partial^{3} q}{\partial x^{3}}+6 q \frac{\partial q}{\partial x}=0$$

  admits the Lax pair

  $$M \psi=0, \quad N \psi=0,$$

  write down an expression for $d \lambda(l)$ which gives rise to the one-soliton solution of the $\mathrm{KdV}$ equation. Write down an expression for $\psi(k ; x, t)$ and for $q(x, t)$.

  comment

Number Fields

• B4.6

  Number Fields | Part II, 2004

  Let $K$ be a finite extension of $\mathbb{Q}$, and $\mathcal{O}$ the ring of integers of $K$. Write an essay outlining the proof that every non-zero ideal of $\mathcal{O}$ can be written as a product of non-zero prime ideals, and that this factorisation is unique up to the order of the factors.

  comment

Number Theory

• A4.10

  Number Theory | Part II, 2004

  Write an essay on pseudoprimes and their role in primality testing. You should discuss pseudoprimes, Carmichael numbers, and Euler and strong pseudoprimes. Where appropriate, your essay should include small examples to illustrate your statements.

  comment

Numerical Analysis

• A4.22 B4.20

  Numerical Analysis | Part II, 2004

  Write an essay on the method of conjugate gradients. You should define the method, list its main properties and sketch the relevant proof. You should also prove that (in exact arithmetic) the method terminates in a finite number of steps, briefly mention the connection with Krylov subspaces, and describe the approach of preconditioned conjugate gradients.

  comment

Optimization and Control

• B4.14

  Optimization and Control | Part II, 2004

  Consider the deterministic dynamical system

  $$\dot{x}_{t}=A x_{t}+B u_{t}$$

  where $A$ and $B$ are constant matrices, $x_{t} \in \mathbb{R}^{n}$, and $u_{t}$ is the control variable, $u_{t} \in \mathbb{R}^{m}$. What does it mean to say that the system is controllable?

  Let $y_{t}=e^{-t A} x_{t}-x_{0}$. Show that if $V_{t}$ is the set of possible values for $y_{t}$ as the control $\left\{u_{s}: 0 \leq x \leq t\right\}$ is allowed to vary, then $V_{t}$ is a vector space.

  Show that each of the following three conditions is equivalent to controllability of the system.

  (i) The set $\left\{v \in \mathbb{R}^{n}: v^{\top} y_{t}=0\right.$ for all $\left.y_{t} \in V_{t}\right\}=\{0\}$.

  (ii) The matrix $H(t)=\int_{0}^{t} e^{-s A} B B^{\top} e^{-s A^{\top}} d s$ is (strictly) positive definite.

  (iii) The matrix $M_{n}=\left[\begin{array}{lllll}B & A B & A^{2} B & \cdots & A^{n-1} B\end{array}\right]$ has rank $n$.

  Consider the scalar system

  $$\sum_{j=0}^{n} a_{j}\left(\frac{d}{d t}\right)^{n-j} \xi_{t}=u_{t}$$

  where $a_{0}=1$. Show that this system is controllable.

  comment

Partial Differential Equations

• B4.18

  Partial Differential Equations | Part II, 2004

  (a) State a theorem of local existence, uniqueness and $C^{1}$ dependence on the initial data for a solution for an ordinary differential equation. Assuming existence, prove that the solution depends continuously on the initial data.

  (b) State a theorem of local existence of a solution for a general quasilinear firstorder partial differential equation with data on a smooth non-characteristic hypersurface. Prove this theorem in the linear case assuming the validity of the theorem in part (a); explain in your proof the importance of the non-characteristic condition.

  comment

Principles of Dynamics

• A4.2

  Principles of Dynamics | Part II, 2004

  Consider a system of coordinates rotating with angular velocity $\boldsymbol{\omega}$ relative to an inertial coordinate system.

  Show that if a vector $\mathbf{v}$ is changing at a rate $d \mathbf{v} / d t$ in the inertial system, then it is changing at a rate

  $$\left.\frac{d \mathbf{v}}{d t}\right|_{\text {rot }}=\frac{d \mathbf{v}}{d t}-\boldsymbol{\omega} \wedge \mathbf{v}$$

  with respect to the rotating system.

  A solid body rotates with angular velocity $\omega$ in the absence of external torque. Consider the rotating coordinate system aligned with the principal axes of the body.

  (a) Show that in this system the motion is described by the Euler equations

  $\left.I_{1} \frac{d \omega_{1}}{d t}\right|_{\text {rot }}=\omega_{2} \omega_{3}\left(I_{2}-I_{3}\right) \quad,\left.\quad I_{2} \frac{d \omega_{2}}{d t}\right|_{\text {rot }}=\omega_{3} \omega_{1}\left(I_{3}-I_{1}\right) \quad,\left.\quad I_{3} \frac{d \omega_{3}}{d t}\right|_{\text {rot }}=\omega_{1} \omega_{2}\left(I_{1}-I_{2}\right)$, where $\left(\omega_{1}, \omega_{2}, \omega_{3}\right)$ are the components of the angular velocity in the rotating system and $I_{1,2,3}$ are the principal moments of inertia.

  (b) Consider a body with three unequal moments of inertia, $I_{3}<I_{2}<I_{1}$. Show that rotation about the 1 and 3 axes is stable to small perturbations, but rotation about the 2 axis is unstable.

  (c) Use the Euler equations to show that the kinetic energy, $T$, and the magnitude of the angular momentum, $L$, are constants of the motion. Show further that

  $$2 T I_{3} \leq L^{2} \leq 2 T I_{1} \text {. }$$

  comment

Principles of Statistics

• A4.13 B4.15

  Principles of Statistics | Part II, 2004

  Suppose that $\theta \in \mathbb{R}^{d}$ is the parameter of a non-degenerate exponential family. Derive the asymptotic distribution of the maximum-likelihood estimator $\hat{\theta}_{n}$ of $\theta$ based on a sample of size $n$. [You may assume that the density is infinitely differentiable with respect to the parameter, and that differentiation with respect to the parameter commutes with integration.]

  Part II 2004

  comment

Probability and Measure

• B4.11

  Probability and Measure | Part II, 2004

  Let $(\Omega, \mathcal{F}, \mathbb{P})$ be a probability space and let $X, X_{1}, X_{2}, \ldots$ be random variables. Write an essay in which you discuss the statement: if $X_{n} \rightarrow X$ almost everywhere, then $\mathbb{E}\left(X_{n}\right) \rightarrow \mathbb{E}(X)$. You should include accounts of monotone, dominated, and bounded convergence, and of Fatou's lemma.

  [You may assume without proof the following fact. Let $(\Omega, \mathcal{F}, \mu)$ be a measure space, and let $f: \Omega \rightarrow \mathbb{R}$ be non-negative with finite integral $\mu(f) .$ If $\left(f_{n}: n \geqslant 1\right)$ are non-negative measurable functions with $f_{n}(\omega) \uparrow f(\omega)$ for all $\omega \in \Omega$, then $\mu\left(f_{n}\right) \rightarrow \mu(f)$ as $n \rightarrow \infty$.]

  comment

Quantum Physics

• A4.16

  Quantum Physics | Part II, 2004

  Explain the operation of the $n p$ junction. Your account should include a discussion of the following topics:

  (a) the rôle of doping and the fermi-energy;

  (b) the rôle of majority and minority carriers;

  (c) the contact potential;

  (d) the relationship $I(V)$ between the current $I$ flowing through the junction and the external voltage $V$ applied across the junction;

  (e) the property of rectification.

  comment

Representation Theory

• B4.2

  Representation Theory | Part II, 2004

  Write an essay on the finite-dimensional representations of $S U_{2}$, including a proof of their complete reducibility, and a description of the irreducible representations and the decomposition of their tensor products.

  comment

Riemann Surfaces

• B4.8

  Riemann Surfaces | Part II, 2004

  Let $\Lambda$ be a lattice in $\mathbb{C}, \Lambda=\mathbb{Z} \omega_{1}+\mathbb{Z} \omega_{2}$, where $\omega_{1}, \omega_{2} \neq 0$ and $\omega_{1} / \omega_{2} \notin \mathbb{R}$. By constructing an appropriate family of charts, show that the torus $\mathbb{C} / \Lambda$ is a Riemann surface and that the natural projection $\pi: z \in \mathbb{C} \rightarrow z+\Lambda \in \mathbb{C} / \Lambda$ is a holomorphic map.

  [You may assume without proof any known topological properties of $\mathbb{C} / \Lambda$.]

  Let $\Lambda^{\prime}=\mathbb{Z} \omega_{1}^{\prime}+\mathbb{Z} \omega_{2}^{\prime}$ be another lattice in $\mathbb{C}$, with $\omega_{1}^{\prime}, \omega_{2}^{\prime} \neq 0$ and $\omega_{1}^{\prime} / \omega_{2}^{\prime} \notin \mathbb{R}$. By considering paths from 0 to an arbitrary $z \in \mathbb{C}$, show that if $f: \mathbb{C} / \Lambda \rightarrow \mathbb{C} / \Lambda^{\prime}$ is a conformal equivalence then

  $$f(z+\Lambda)=(a z+b)+\Lambda^{\prime} \quad \text { for some } a, b, \in \mathbb{C}, \text { with } a \neq 0$$

  [Any form of the Monodromy Theorem and basic results on the lifts of paths may be used without proof, provided that these are correctly stated. You may assume without proof that every injective holomorphic function $F: \mathbb{C} \rightarrow \mathbb{C}$ is of the form $F(z)=a z+b$, for some $a, b \in \mathbb{C}$.]

  Give an explicit example of a non-constant holomorphic map $\mathbb{C} / \Lambda \rightarrow \mathbb{C} / \Lambda$ that is not a conformal equivalence.

  Part II 2004

  comment

Statistical Physics

• B4.23

  Statistical Physics | Part II, 2004

  Derive the Bose-Einstein expression for the mean number of Bose particles $\bar{n}$ occupying a particular single-particle quantum state of energy $\varepsilon$, when the chemical potential is $\mu$ and the temperature is $T$ in energy units.

  Why is the chemical potential for a gas of photons given by $\mu=0$ ?

  Show that, for black-body radiation in a cavity of volume $V$ at temperature $T$, the mean number of photons in the angular frequency range $(\omega, \omega+d \omega)$ is

  $$\frac{V}{\pi^{2} c^{3}} \frac{\omega^{2} d \omega}{e^{\hbar \omega / T}-1}$$

  Hence, show that the total energy $E$ of the radiation in the cavity is

  $$E=K V T^{4}$$

  where $K$ is a constant that need not be evaluated.

  Use thermodynamic reasoning to find the entropy $S$ and pressure $P$ of the radiation and verify that

  $$E-T S+P V=0$$

  Why is this last result to be expected for a gas of photons?

  comment

Statistical Physics and Cosmology

• A4.18

  Statistical Physics and Cosmology | Part II, 2004

  (a) Consider an ideal gas of Fermi particles obeying the Pauli exclusion principle with a set of one-particle energy eigenstates $E_{i}$. Given the probability $p_{i}\left(n_{i}\right)$ at temperature $T$ that there are $n_{i}$ particles in the eigenstate $E_{i}$ :

  $$p_{i}\left(n_{i}\right)=\frac{e^{\left(\mu-E_{i}\right) n_{i} / k T}}{Z_{i}},$$

  determine the appropriate normalization factor $Z_{i}$. Use this to find the average number $\bar{n}_{i}$ of Fermi particles in the eigenstate $E_{i}$.

  Explain briefly why in generalizing these discrete eigenstates to a continuum in momentum space (in the range $p$ to $p+d p$ ) we must multiply by the density of states

  $$g(p) d p=\frac{4 \pi g_{s} V}{h^{3}} p^{2} d p$$

  where $g_{s}$ is the degeneracy of the eigenstates and $V$ is the volume.

  (b) With the energy expressed as a momentum integral

  $$E=\int_{0}^{\infty} E(p) \bar{n}(p) d p$$

  consider the effect of changing the volume $V$ so slowly that the occupation numbers do not change (i.e. particle number $N$ and entropy $S$ remain fixed). Show that the momentum varies as $d p / d V=-p / 3 V$ and so deduce from the first law expression

  $$\left(\frac{\partial E}{\partial V}\right)_{N, S}=-P$$

  that the pressure is given by

  $$P=\frac{1}{3 V} \int_{0}^{\infty} p E^{\prime}(p) \bar{n}(p) d p .$$

  Show that in the non-relativistic limit $P=\frac{2}{3} U / V$ where $U$ is the internal energy, while for ultrarelativistic particles $P=\frac{1}{3} E / V$.

  (c) Now consider a Fermi gas in the limit $T \rightarrow 0$ with all momentum eigenstates filled up to the Fermi momentum $p_{\mathrm{F}}$. Explain why the number density can be written as

  $$n=\frac{4 \pi g_{s}}{h^{3}} \int_{0}^{p_{\mathrm{F}}} p^{2} d p \propto p_{\mathrm{F}}^{3}$$

  From similar expressions for the energy, deduce in both the non-relativistic and ultrarelativistic limits that the pressure may be written as

  $$P \propto n^{\gamma}$$

  where $\gamma$ should be specified in each case.

  (d) Examine the stability of an object of radius $R$ consisting of such a Fermi degenerate gas by comparing the gravitational binding energy with the total kinetic energy. Briefly point out the relevance of these results to white dwarfs and neutron stars.

  comment

Stochastic Financial Models

• A4.12 B4.16

  Stochastic Financial Models | Part II, 2004

  What is Brownian motion $\left(B_{t}\right)_{t \geqslant 0}$ ? Briefly explain how Brownian motion can be considered as a limit of simple random walks. State the Reflection Principle for Brownian motion, and use it to derive the distribution of the first passage time $\tau_{a} \equiv \inf \left\{t: B_{t}=a\right\}$ to some level $a>0$.

  Suppose that $X_{t}=B_{t}+c t$, where $c>0$ is constant. Stating clearly any results to which you appeal, derive the distribution of the first-passage time $\tau_{a}^{(c)} \equiv \inf \left\{t: X_{t}=a\right\}$ to $a>0$.

  Now let $\sigma_{a} \equiv \sup \left\{t: X_{t}=a\right\}$, where $a>0$. Find the density of $\sigma_{a}$.

  comment

Theoretical Geophysics

• A4.20

  Theoretical Geophysics | Part II, 2004

  In a reference frame rotating about a vertical axis with angular velocity $f / 2$, the horizontal components of the momentum equation for a shallow layer of inviscid, incompressible, fluid of uniform density $\rho$ are

  $$\begin{aligned} &\frac{D u}{D t}-f v=-\frac{1}{\rho} \frac{\partial p}{\partial x} \\ &\frac{D v}{D t}+f u=-\frac{1}{\rho} \frac{\partial p}{\partial y} \end{aligned}$$

  where $u$ and $v$ are independent of the vertical coordinate $z$, and $p$ is given by hydrostatic balance. State the nonlinear equations for conservation of mass and of potential vorticity for such a flow in a layer occupying $0<z<h(x, y, t)$. Find the pressure $p$.

  By linearising the equations about a state of rest and uniform thickness $H$, show that small disturbances $\eta=h-H$, where $\eta \ll H$, to the height of the free surface obey

  $$\frac{\partial^{2} \eta}{\partial t^{2}}-g H\left(\frac{\partial^{2} \eta}{\partial x^{2}}+\frac{\partial^{2} \eta}{\partial y^{2}}\right)+f^{2} \eta=f^{2} \eta_{0}-f H \zeta_{0}$$

  where $\eta_{0}$ and $\zeta_{0}$ are the values of $\eta$ and the vorticity $\zeta$ at $t=0$.

  Obtain the dispersion relation for homogeneous solutions of the form $\eta \propto \exp [i(k x-$ $\omega t)$ ] and calculate the group velocity of these Poincaré waves. Comment on the form of these results when $a k \ll 1$ and $a k \gg 1$, where the lengthscale $a$ should be identified.

  Explain what is meant by geostrophic balance. Find the long-time geostrophically balanced solution, $\eta_{\infty}$ and $\left(u_{\infty}, v_{\infty}\right)$, that results from initial conditions $\eta_{0}=A \operatorname{sgn}(x)$ and $(u, v)=0$. Explain briefly, without detailed calculation, how the evolution from the initial conditions to geostrophic balance could be found.

  comment

Transport Processes

• A4.19

  Transport Processes | Part II, 2004

  (a) Solute diffuses and is advected in a moving fluid. Derive the transport equation and deduce that the solute concentration $C(\mathbf{x}, t)$ satisfies the advection-diffusion equation

  $$C_{t}+\nabla \cdot(\mathbf{u} C)=\nabla \cdot(D \nabla C)$$

  where $\mathbf{u}$ is the velocity field and $D$ the diffusivity. Write down the form this equation takes when $\nabla \cdot \mathbf{u}=0$, both $\mathbf{u}$ and $\nabla C$ are unidirectional, in the $x$-direction, and $D$ is a constant.

  (b) A solution occupies the region $x \geqslant 0$, bounded by a semi-permeable membrane at $x=0$ across which fluid passes (by osmosis) with velocity

  $$u=-k\left(C_{1}-C(0, t)\right)$$

  where $k$ is a positive constant, $C_{1}$ is a fixed uniform solute concentration in the region $x<0$, and $C(x, t)$ is the solute concentration in the fluid. The membrane does not allow solute to pass across $x=0$, and the concentration at $x=L$ is a fixed value $C_{L}$ (where $\left.C_{1}>C_{L}>0\right)$.

  Write down the differential equation and boundary conditions to be satisfied by $C$ in a steady state. Make the equations non-dimensional by using the substitutions

  $$X=\frac{x k C_{1}}{D}, \quad \theta(X)=\frac{C(x)}{C_{1}}, \quad \theta_{L}=\frac{C_{L}}{C_{1}},$$

  and show that the concentration distribution is given by

  $$\theta(X)=\theta_{L} \exp \left[\left(1-\theta_{0}\right)(\Lambda-X)\right]$$

  where $\Lambda$ and $\theta_{0}$ should be defined, and $\theta_{0}$ is given by the transcendental equation

  $$\theta_{0}=\theta_{L} e^{\Lambda-\Lambda \theta_{0}}$$

  What is the dimensional fluid velocity $u$, in terms of $\theta_{0} ?$

  (c) Show that if, instead of taking a finite value of $L$, you had tried to take $L$ infinite, then you would have been unable to solve for $\theta$ unless $\theta_{L}=0$, but in that case there would be no way of determining $\theta_{0}$.

  (d) Find asymptotic expansions for $\theta_{0}$ from equation $(*)$ in the following limits:

  (i) For $\theta_{L} \rightarrow 0, \Lambda$ fixed, expand $\theta_{0}$ as a power series in $\theta_{L}$, and equate coefficients to show that

  $$\theta_{0} \sim e^{\Lambda} \theta_{L}-\Lambda e^{2 \Lambda} \theta_{L}^{2}+O\left(\theta_{L}^{3}\right) .$$

  (ii) For $\Lambda \rightarrow \infty, \theta_{L}$ fixed, take logarithms, expand $\theta_{0}$ as a power series in $1 / \Lambda$, and show that

  $$\theta_{0} \sim 1+\frac{\log \theta_{L}}{\Lambda}+O\left(\frac{1}{\Lambda^{2}}\right)$$

  What is the limiting value of $\theta_{0}$ in the limits (i) and (ii)?

  (e) Both the expansions in (d) break down when $\theta_{L}=O\left(e^{-\Lambda}\right)$. To investigate the double limit $\Lambda \rightarrow \infty, \theta_{L} \rightarrow 0$, show that $(*)$ can be written as

  $$\lambda=\phi e^{\phi}$$

  where $\phi=\Lambda \theta_{0}$ and $\lambda$ is to be determined. Show that $\phi \sim \lambda-\lambda^{2}+\ldots$ for $\lambda \ll 1$, and $\phi \sim \log \lambda-\log \log \lambda+\ldots$ for $\lambda \gg 1$.

  Briefly discuss the implication of your results for the problem raised in (c) above.

  comment

Waves in Fluid and Solid Media

• B4.27

  Waves in Fluid and Solid Media | Part II, 2004

  A plane shock is moving with speed $U$ into a perfect gas. Ahead of the shock the gas is at rest with pressure $p_{1}$ and density $\rho_{1}$, while behind the shock the velocity, pressure and density of the gas are $u_{2}, p_{2}$ and $\rho_{2}$ respectively. Derive the Rankine-Hugoniot relations across the shock. Show that

  $$\frac{\rho_{1}}{\rho_{2}}=\frac{2 c_{1}^{2}+(\gamma-1) U^{2}}{(\gamma+1) U^{2}}$$

  where $c_{1}^{2}=\gamma p_{1} / \rho_{1}$ and $\gamma$ is the ratio of the specific heats of the gas. Now consider a change of frame such that the shock is stationary and the gas has a component of velocity $V$ parallel to the shock. Deduce that the angle of deflection $\delta$ of the flow which is produced by a stationary shock inclined at an angle $\alpha=\tan ^{-1}(U / V)$ to an oncoming stream of Mach number $M=\left(U^{2}+V^{2}\right)^{\frac{1}{2}} / c_{1}$ is given by

  $$\tan \delta=\frac{2 \cot \alpha\left(M^{2} \sin \alpha^{2}-1\right)}{2+M^{2}(\gamma+\cos 2 \alpha)}$$

  [Note that

  $$\left.\tan (\theta+\phi)=\frac{\tan \theta+\tan \phi}{1-\tan \theta \tan \phi} . \quad\right]$$

  comment